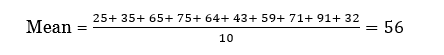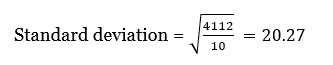Definition of Standard Deviation
Standard deviation is a measure about how much a number or a value is deviating from the mean of the whole data set. In other words, it tells us how much is data scattered. The formula for calculating the standard deviation is as follows:
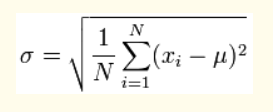
Example of Standard Deviation:
Assume that in a class 10 students have scored the following marks in math test: 25, 35, 65, 75, 64, 43, 59, 71, 91 and 32. To calculate the standard deviation, first, calculate the mean.
Then we have to take the squares of the difference between mean and each student’ score.
|
Scores |
Mean |
Mean - Score |
(Mean - Score)2 |
|
25 |
56 |
31 |
961 |
|
35 |
56 |
21 |
441 |
|
65 |
56 |
-9 |
81 |
|
75 |
56 |
-19 |
361 |
|
64 |
56 |
-8 |
64 |
|
43 |
56 |
13 |
169 |
|
59 |
56 |
-3 |
9 |
|
71 |
56 |
-15 |
225 |
|
91 |
56 |
-35 |
1225 |
|
32 |
56 |
24 |
576 |
|
|
|
|
4112 |
Using the above formula the standard deviation will be as follows:
View More Cost Accounting Definitions
Related Questions of Standard Deviation
Linda Lechner has just been severely chastised for her inventory policy.
Among the thirty largest U.S. cities, the mean
Estimate the mean and the standard deviation of the following frequency distribution
Using samples of 200 credit card statements, an auditor found the
a. How many variance terms and how many different covariance terms
Frostbite Thermalwear has a zero coupon bond issue outstanding with a face
Stock X has a 10% expected return, a beta coefficient
As part of an insurance company’s training program, participants learn how
Ned’s Natural Foods sells unshelled peanuts by the pound. Historically,
You must analyze a potential new product—a caulking compound that
Show All