Question: A sample of 1800 homes in different
A sample of 1800 homes in different neighborhood of Washington, DC, in 2017 produced the data in the table for the number of bedrooms and house price. Is the price of the house independent of whether it has 3 or more bedrooms?
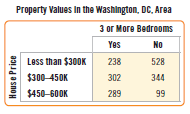
Transcribed Image Text:
Property Values In the Washington, DC, Area 3 ог Моге Вedгooms Yes No Less than $300K 238 528 $300-450K 302 344 $450-60OK 289 99 Hous e Price
> Pew Research Center publishes polls on issues important in the news and about American life at its website, www.pewinternet.org. At the end of a report about a survey you can find a paragraph such as this one: These readings come from a national survey c
> A commuter finds that she waits an average of 14.8 seconds at each of five stoplights, with a standard deviation of 9.2 seconds. Find the mean and the standard deviation of the total amount of time she waits at all five lights. What, if anything, did you
> Major League Baseball, responding to concerns about their “brand,” tests players to see whether they are using performance-enhancing drugs. Officials select a team at random, and a drug-testing crew shows up unannounced to test all 40 players on the team
> The website www.gamefaqs.com asked, as their question of the day to which visitors to the site were invited to respond, “Do you ever read the end-user license agreements when installing software or games?” Of the 98,574 respondents, 63.47% said they neve
> An investment newsletter makes general predictions about the economy to help their clients make sound investment decisions. a) Recently they said that because the stock market had been up for the past three months in a row that it was “due for a correct
> Even though commercial airlines have excellent safety records, in the weeks following a crash, airlines often report a drop in the number of passengers, probably because people are afraid to risk flying. a) A travel agent suggests that since the law of
> Respond to the following questions: a) After an unusually dry autumn, a radio announcer is heard to say, “Watch out! We’ll pay for these sunny days later on this winter.” Explain what he’s trying to say, and comment on the validity of his reasoning. b)
> Respond to the following questions: a) A casino claims that its roulette wheel is truly random. What should that claim mean? b) A reporter on Market Place says that there is a 50% chance that the NASDAQ will hit a new high in the next month. What is th
> Corporations are also very interested in the value of their brand. The following chart displays the value of the top 6 carbonated soft drink brands in 2015 as collected by Millward Brown (www.millwardbrown .com/brandz/top-global-brands/2015/brand-categor
> Multinational corporations face stiff competition in the carbonated drink category (also known as soda or pop, depending on where you live). Data were collected on the 2015 U.S. market share of leading sellers of carbonated soft drinks, summarized in the
> For each description of data in Exercises 7 to 26, identify the W’s, name the variables, specify for each variable whether its use indicates it should be treated as categorical or quantitative, and for any quantitative variable identify the units in whic
> For each description of data in Exercises 7 to 26, identify the W’s, name the variables, specify for each variable whether its use indicates it should be treated as categorical or quantitative, and for any quantitative variable identify the units in whic
> A grocery supplier believes that the mean number of broken eggs per dozen is 0.6, with a standard deviation of 0.5. You buy 3 dozen eggs without checking them. a) How many broken eggs do you expect to get? b) What’s the standard deviation? c) Is it ne
> For the cookie recipe experiment of Exercise 1, identify how Control, Randomization, and Replication were used. Exercise 1: For the following experiment, identify the experimental units, the treatments, the response, and the random assignment. A commer
> The American Veterinary Association claims that the annual cost of medical care for dogs averages $100, with a standard deviation of $30, and for cats averages $120, with a standard deviation of $35. a) What’s the expected difference in the cost of medi
> The amount of cereal that can be poured into a small bowl varies with a mean of 1.5 ounces and a standard deviation of 0.3 ounce. A large bowl holds a mean of 2.5 ounces with a standard deviation of 0.4 ounce. You open a new box of cereal and pour one la
> A newsletter for investors recently reported that the average stock price for a blue chip stock over the past 12 months was $72. No standard deviation was given. Is the standard deviation more likely to be $6, $16, or $60? Explain.
> A business publication recently released a study on the total number of years of experience in industry among CEOs. The mean is provided in the article, but not the standard deviation. Is the standard deviation most likely to be 6 months, 6 years, or 16
> The police department of a major city needs to update its budget. For this purpose, they need to understand the variation in their fines collected from motorists for speeding. As a sample, they recorded the speeds of cars driving past a location with a 2
> Two companies make batteries for cell phone manufacturers. One company claims a mean life span of 2 years, while the other company claims a mean life span of 2.5 years (assuming average use of minutes/month for the cell phone). a) Explain why you would
> A tire manufacturer believes that the tread life of its snow tires can be described by a Normal model with a mean of 32,000 miles and a standard deviation of 2500 miles. a) If you buy a set of these tires, would it be reasonable for you to hope that the
> Manufacturing and selling drugs that claim to reduce an individual’s cholesterol level is big business. A company would like to market their drug to women if their cholesterol is in the top 15%. Assume the cholesterol levels of adult American women can b
> A large philanthropic organization keeps records on the people who have contributed to their cause. In addition to keeping records of past giving, the organization buys demographic data on neighborhoods from the U.S. Census Bureau. Eighteen of these vari
> A survey of 200 middle managers showed a distribution of the number of hours of exercise they participated in per week with a mean of 3.66 hours and a standard deviation of 4.93 hours. a) According to the Normal model, what percent of managers will exer
> A company has discovered that a recent batch of batteries had manufacturing f laws, and has issued a recall. You have 10 batteries covered by the recall, and 3 are dead. You choose 2 batteries at random from your package of 10. a) Has the assumption of i
> Exercise 23 proposes modeling quarterly returns of a group of mutual funds with N(0.062, 0.018). The manager of this group of funds would like to f lag any fund whose return is unusually low for a quarter. What level of return would you consider to be un
> Suppose that job satisfaction scores can be modeled with N(100, 12). Human resource departments of corporations are generally concerned if the job satisfaction drops below a certain score. What score would you consider to be unusually low? Explain.
> Anna, a business major, took final exams in both Microeconomics and Macroeconomics and scored 83 on both. Her roommate Megan, also taking both courses, scored 77 on the Micro exam and 95 on the Macro exam. Overall, student scores on the Micro exam had a
> Each year thousands of high school students take either the SAT or ACT, standardized tests used in the college admissions process. Combined SAT scores can go as high as 1600, while the maximum ACT composite score is 36. Since the two exams use very diffe
> Every Normal model is defined by its parameters, the mean and the standard deviation. For each model described here, find the missing parameter. Don’t forget to draw a picture. a) m = 1250, 35% below 1200; s = ? b) m = 0.64, 12% above 0.70; s = ? c) s
> Every Normal model is defined by its parameters, the mean and the standard deviation. For each model described here, find the missing parameter. As always, start by drawing a picture. a) m = 20, 45% above 30; s = ? b) m = 88, 2% below 50; s = ? c) s =
> Consider the IQ model N(100, 16) one last time. a) What IQ represents the 15th percentile? b) What IQ represents the 98th percentile? c) What’s the IQR of the IQs?
> Consider the Normal model N(0.062, 0.018) for returns of mutual funds in Exercise 23 one last time. a) What value represents the 40th percentile of these returns? b) What value represents the 99th percentile? c) What’s the IQR of the quarterly returns
> In the Normal model N(100, 16) for IQ scores from Exercise 24, what cutoff value bounds the a) highest 5% of all IQs? b) lowest 30% of the IQs? c) middle 80% of the IQs? d) lowest 90% of all IQs?
> Based on the model N(0.062, 0.018) for quarterly returns from Exercise 23, what are the cutoff values for the a) highest 10% of these funds? b) lowest 20%? c) middle 40%? d) highest 80%?
> Your company bids for two contracts. You believe the probability that you get contract #1 is 0.8. If you get contract #1, the probability that you also get contract #2 will be 0.2, and if you do not get contract #1, the probability that you get contract
> Based on the Normal model N (100, 16) describing IQ scores from Exercise 24, what percent of applicants would you expect to have scores a) over 80? b) under 90? c) between 112 and 132? d) over 125?
> According to the Normal model N(0.062, 0.018) describing mutual fund returns in the 1st quarter of 2013 in Exercise 23, what percent of this group of funds would you expect to have return a) over 6.8%? b) between 0% and 7.6%? c) more than 1%? d) less
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> For Exercises 23–30, use the 68–95–99.7 Rule to approximate the probabilities rather than using technology to find the values more precisely. Answers given for probabilities or percentages from Exercise 31 on assume that a calculator or software has been
> A sporting goods manufacturer was asked to sponsor a local boy in two fishing tournaments. They claim the probability that he will win the first tournament is 0.4. If he wins the first tournament, they estimate the probability that he will also win the s
> Recently, the International Gaming Technology company issued the following press release: (LAS VEGAS, Nev.)—Cynthia Jay was smiling ear to ear as she walked into the news conference at the Desert Inn Resort in Las Vegas today, and well she should. Last n
> Insurance companies collect annual payments from homeowners in exchange for paying to rebuild houses that burn down. a) Why should you be reluctant to accept a $3000 payment from your neighbor to replace his house should it burn down during the coming y
> The following table shows a sample of property listings and values from one neighborhood (one ZIP code) in the Washington, DC, area in September 2017: a) In this sample, what proportion of homes is valued at $500K or less? b) Are the number of bedrooms
> Professional polling organizations face the challenge of selecting a representative sample of U.S. adults by telephone. This has been complicated by people who only use cell phones and by others whose landline phones are unlisted. A careful survey by Dem
> In the real estate research described in Exercise 33, 64% of homes for sale have garages, 21% have swimming pools, and 17% have both features. a) What is the probability that a home for sale has a garage, but not a pool? b) If a home for sale has a gar
> Look again at the data in the electronic communications in Exercise 54. a) If we select a respondent at random, what’s the probability that we choose a person earning less than $50 K and responded “somewhat”? b) Among those earning $50–99.9K, what is t
> Look again at the data from the coffeehouse survey in Exercise 53. a) If we select a person at random, what’s the probability we choose a person between 18 and 24 years old who is spending more time at coffeehouses? b) Among the 18- to 24-year olds, wh
> Given the table of probabilities compiled for a department store chain in Exercise 58, are age and shopping at the department store independent? Explain.
> Given the table of probabilities compiled for marketing managers in Exercise 57, are high blood pressure and high cholesterol independent? Explain.
> A consumer organization inspecting new cars found that many had appearance defects (dents, scratches, paint chips, etc.). While none had more than three of these defects, 7% had three, 11% had two, and 21% had one defect. a) Find the expected number of a
> A European department store is developing a new advertising campaign for their new U.S. location, and their marketing managers need to understand their target market better. A survey of adult shoppers found the probabilities that an adult would shop at t
> A U.S. pharmaceutical company is considering manufacturing and marketing a pill that will help to lower both an individual’s blood pressure and cholesterol. The company is interested in understanding the demand for such a product. The j
> Twenty percent of cars that are inspected have faulty pollution control systems. The cost of repairing a pollution control system exceeds $100 about 40% of the time. When a driver takes her car in for inspection, what’s the probability that she will end
> Exercises 36 and 38 discussed the challenges faced by the Red Cross in finding enough blood of various types. But blood typing also depends on the Rh factor, which can be negative or positive. Here is a table of the estimated proportions worldwide for bl
> A Mintel study asked consumers if electronic communications devices influenced whether or not they bought a certain car. The table below gives the results classified by household income: If we select a person at random from this sample: a) What is the pr
> A Mintel report on coffeehouses asked consumers if they were spending more time in coffeehouses. The table below gives the responses classified by age: a) What is the probability that a randomly selected respondent is spending more time at coffeehouses a
> Of the 2201 people on the RMS Titanic, only 711 survived. The practice of “women and children first” was first used to describe the chivalrous actions of the sailors during the sinking of the HMS Birkenhead in 1852, bu
> A Pew Research report on September 13, 2017, reported that 61% of 18–29 year olds watch TV using online streaming services. (By contrast, only 5% of those 65 and older watch online.) The table below shows the proportions within each age group using vario
> A company that manufactures and sells consumer video cameras sells two versions of their popular hard disk camera, a basic camera for $750, and a deluxe version for $1250. About 75% of customers select the basic camera. Of those, 60% purchase the extende
> As manager for a construction firm, you are in charge of bidding on two large contracts. You believe the probability you get contract #1 is 0.8. If you get contract #1, the probability you also get contract #2 will be 0.2, and if you do not get #1, the p
> A commuter must pass through five traffic lights on her way to work and will have to stop at each one that is red. After keeping a record for several months, she developed the following probability model for the number of red lights she hits: a) How ma
> Exercise 47 shows the results of a poll that asked about gun ownership. Suppose we select three adults at random from this sample. a) What is the probability that all three respond “Yes”? b) What is the probability t
> The General Social Survey, run annually, asked respondents “Do you have in your home (or garage) any guns or revolvers?” The responses are given in the table (sda.berkeley.edu/cgi-bin/hsda?harcsda+gss10). a) If we se
> More economic conditions 2017. Exercise 45 shows the results of a Gallup Poll about U.S. economic conditions. Suppose we select three adults at random from this sample. a) What is the probability that all three responded “Poor”? b) What is the probabilit
> A Gallup Poll in September 2017 asked U.S. adults to rate economic conditions in the country today as “excellent,” “good,” “only fair,” or â
> A shipment of road bikes has just arrived at The Spoke, a small bicycle shop, and all the boxes have been placed in the back room. The owner asks her assistant to start bringing in the boxes. The assistant sees 20 identical-looking boxes and starts bring
> In addition to slot machines, casinos must understand the probabilities involved in card games. Suppose you are playing at the blackjack table, and the dealer shuff les a deck of cards. The first card shown is red. So is the second and the third. In fact
> The technical support desk at a college has set up a special service for tablets. A survey shows that 54% of tablets on campus run Apple’s iOS, 43% run Google’s Android OS, and 3% run Microsoft’s Windows. Assuming that users of each of the operating syst
> A company has recently replaced their e-mail spam filter because investigations had found that the volume of spam e-mail was interrupting productive work on about 15% of workdays. To see how bad the situation was, calculate the probability that during a
> Because gambling is big business, calculating the odds of a gambler winning or losing in every game is crucial to the financial forecasting for a casino. A standard slot machine has three wheels that spin independently. Each has 10 equally likely symbols
> A recent study of IRS audits showed that, for estates worth less than $5 million, about 1 out of 7 of all estate tax returns are audited, but that probability increases to 50% for estates worth over $5 million. Suppose a tax accountant has three clients
> A small software company will bid on a major contract. It anticipates a profit of $50,000 if it gets it, but thinks there is only a 30% chance of that happening. a) What’s the expected profit? b) Find the standard deviation for the profit.
> In Exercise 36, you calculated probabilities involving various blood types. a) If you examine one donor, are the events of the donor being Type A and the donor being Type B disjoint or independent or neither? Explain your answer. b) If you examine two
> In Exercise 35, you calculated probabilities of getting various colors of M&M’s. a) If you draw one M&M, are the events of getting a red one and getting an orange one disjoint or independent or neither? b) If you draw two M&M’s one after the other, are
> The American Red Cross must track their supply and demand for various blood types. They estimate that about 45% of the U.S. population has Type O blood, 40% Type A, 11% Type B, and the rest Type AB. a) If someone volunteers to give blood, what is the pr
> The Mars company says that before the introduction of purple, yellow made up 20% of their plain M&M candies, red made up another 20%, and orange, blue, and green each made up 10%. The rest were brown. a) If you picked an M&M at random from a pre-purple
> Employment data at a large company reveal that 72% of the workers are married, 44% are college graduates, and half of the college grads are married. What’s the probability that a randomly chosen worker is: a) Neither married nor a college graduate? b)
> In a sample of real estate ads, 64% of homes for sale had garages, 21% have swimming pools, and 17% have both features. What is the probability that a home for sale has: a) A pool, a garage, or both? b) Neither a pool nor a garage? c) A pool but no ga
> Final consulting team project. You used the Multiplication Rule to calculate probabilities about the telecommunications experience of your consulting teammates in Exercise 30. a) What must be true about the groups in order to make that approach valid? b)
> You used the Multiplication Rule to calculate repair probabilities for your cars in Exercise 29. a) What must be true about your cars in order to make that approach valid? b) Do you think this assumption is reasonable? Explain.
> You are assigned to be part of a team of three analysts of a global management consulting company as described in Exercise 28. What is the probability that of your other two teammates: a) Neither has any telecommunications experience? b) Both have some t
> Consider again the auto repair rates described in Exercise 27. If you bought two new cars, what is the probability that: a) Neither will need repair? b) Both will need repair? c) At least one car will need repair? Exercise 27: In developing their warran
> The probability model below describes the number of repair calls that an appliance repair shop may receive during an hour. a) How many calls should the shop expect per hour? b) What is the standard deviation? Repair Calls 1 3 Probability 0.1 0.3 0.
> You work for a large global management consulting company. Of the entire work force of analysts, 55% have had no experience in the telecommunications industry, 32% have had limited experience (less than 5 years), and the rest have had extensive experienc
> In developing their warranty policy, an automobile company estimates that over a 1-year period 17% of their new cars will need to be repaired once, 7% will need repairs twice, and 4% will require three or more repairs. If you buy a new car from them, wha
> For a sales promotion, the manufacturer places winning symbols under the caps of 10% of all Pepsi bottles. If you buy a six-pack of Pepsi, what is the probability that you win something?
> A tire manufacturer recently announced a recall because 2% of its tires are defective. If you just bought a new set of four tires from this manufacturer, what is the probability that at least one of your new tires is defective?
> Many stores run “secret sales”: Shoppers receive cards that determine how large a discount they get, but the percentage is revealed by scratching off that black stuff (what is that?) only after the purchase has been to
> A toy company is preparing to market an electronic game for young children that “randomly” generates a color. They suspect, however, that the way the random color is determined may not be reliable, so they ask the prog
> Here are engine size (displacement, in liters) and gas mileage (estimated combined city and highway) for a random sample of 35 2016 model cars (taken from Fuel Economy 2016 and identified in the data with Sample = “Yes”). a) Make a scatterplot for these
> Since 1985, average mortgage interest rates have f luctuated from a low of nearly 3% to a high of over 14%. Is there a relationship between the amount of money people borrow and the interest rate that’s offered? Here is a scatterplot of
> The Office of Federal Housing Enterprise Oversight (www.fhfa.gov) collects data on various aspects of housing costs around the United States. Here is a scatterplot of the Housing Cost Index versus the Median Family Income for each of the 50 states. The c
> Concern over the weather associated with El Niño has increased interest in the possibility that the climate on Earth is getting warmer. The most common theory relates an increase in atmospheric levels of carbon dioxide (CO2), a greenhouse

