Question: Consider flow from a water reservoir through
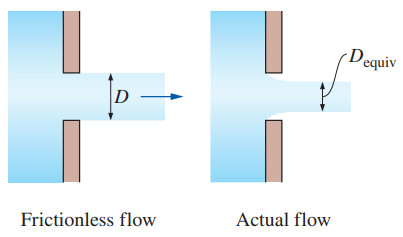
Consider flow from a water reservoir through a circular hole of diameter D at the side wall at a vertical distance H from the free surface. The flow rate through an actual hole with a sharp-edged entrance (KL = 0.5) is considerably less than the flow rate calculated assuming “frictionless†flow and thus zero loss for the hole. Disregarding the effect of the kinetic energy correction factor, obtain a relation for the “equivalent diameter†of the sharp-edged hole for use in frictionless flow relations.
> Water at 15°C is to be discharged from a reservoir at a rate of 18 L/s using two horizontal cast iron pipes connected in series and a pump between them. The first pipe is 20 m long and has a 6 cm diameter, while the second pipe is 35 m long an
> Shell-and-tube heat exchangers with hundreds of tubes housed in a shell are commonly used in practice for heat transfer between two fluids. Such a heat exchanger used in an active solar hot water system transfers heat from a water-antifreeze solution flo
> Repeat Prob. 14–92 except let the length of pipe A be three times that of pipe B. Compare this result to that of Prob. 14–92. Does the difference agree with your intuition? Explain. Data from Prob. 14-92: Two pipes of identical diameter and material ar
> Two pipes of identical diameter and material are connected in parallel. The length of pipe A is five times the length of pipe B. Assuming the flow is fully turbulent in both pipes and thus the friction factor is independent of the Reynolds number and dis
> What are the different mechanisms for transferring energy to or from a control volume?
> The velocity profile in fully developed laminar flow in a circular pipe, in m/s, is given by u(r) = 6(1 − 100r2), where r is the radial distance from the centerline of the pipe in m. Determine (a) the radius of the pipe, (b) the average velocity through
> A house built on a riverside is to be cooled in summer by utilizing the cool water of the river. A 20-m-long section of a circular stainless-steel duct of 20 cm diameter passes through the water. Air flows through the underwater section of the duct at 4
> How does surface roughness affect the pressure drop in a pipe if the flow is turbulent? What would your response be if the flow were laminar?
> The compressed air requirements of a manufacturing facility are met by a 120-hp compressor that draws in air from the outside through a 9-m-long, 22-cm-diameter duct made of thin galvanized iron sheets. The compressor takes in air at a rate of 0.27 m3/s
> In a geothermal district heating system, 10,000 kg/s of hot water must be delivered a distance of 10 km in a horizontal pipe. The minor losses are negligible, and the only significant energy loss arises from pipe friction. The friction factor is taken to
> In a laminar flow through a circular tube of radius of R, the velocity and temperature profiles at a cross section are given by u = u0(1 − r2/R2) and T(r) = A + Br2 − Cr4 where A, B, and C are positive constants. Obtain a relation for the bulk fluid temp
> Two pipes of identical length and material are connected in parallel. The diameter of pipe A is twice the diameter of pipe B. Assuming the friction factor to be the same in both cases and disregarding minor losses, determine the ratio of the flow rates i
> Water to a residential area is transported at a rate of 1.5 m3/s via 70-cm-internal-diameter concrete pipes with a surface roughness of 3 mm and a total length of 1500 m. In order to reduce pumping power requirements, it is proposed to line the interior
> Water is transported by gravity through a 10-cm diameter 550-m-long plastic pipe with an elevation gradient of 0.01 (i.e., an elevation drop of 1 m per 100 m of pipe length). Taking ρ = 1000 kg/m3 and ν = 1 × 10−6 m2/s for water, determine the flow rate
> Repeat Prob. 14–82 for cast iron pipes of the same diameter. Data from Prob. 14-82: A geothermal district heating system involves the transport of geothermal water at 110°C from a geothermal well to a city at about the same elevation for a distance of 1
> A steel rod of 0.5 cm diameter and 10 m length is stretched 3 cm. Young’s modulus for this steel is 21 kN/cm2. How much work, in kJ, is required to stretch this rod?
> A geothermal district heating system involves the transport of geothermal water at 110°C from a geothermal well to a city at about the same elevation for a distance of 12 km at a rate of 1.5 m3/s in 60-cm-diameter stainless-steel pipes. The fluid pressur
> Repeat Prob. 14–80 assuming pipe A has a halfway closed gate valve (KL = 2.1) while pipe B has a fully open globe valve (KL = 10), and the other minor losses are negligible. Data from Prob. 14-80: A certain part of cast iron piping of
> A certain part of cast iron piping of a water distribution system involves a parallel section. Both parallel pipes have a diameter of 30 cm, and the flow is fully turbulent. One of the branches (pipe A) is 1500 m long while the other branch (pipe B) is 2
> Consider laminar flow in a circular pipe. Is the wall shear stress τw higher near the inlet of the pipe or near the exit? Why? What would your response be if the flow were turbulent?
> Water at 15°C is to be pumped from a reservoir (zA = 2 m) to another reservoir at a higher elevation (zB = 9 m) through two 25-m-long plastic pipes connected in parallel. The diameters of the two pipes are 3 cm and 5 cm. Water is to be pumped
> A vented tanker is to be filled with fuel oil with ρ = 920 kg/m3 and μ = 0.045 kg/m·s from an underground reservoir using a 25-m-long, 4-cm-diameter plastic hose with a slightly rounded entrance and two 90Â
> Two water reservoirs A and B are connected to each other through a 40-m-long, 2-cm-diameter cast iron pipe with a sharp-edged entrance. The pipe also involves a swing check valve and a fully open gate valve. The water level in both reservoirs is the same
> A water tank filled with solar-heated water at 40°C is to be used for showers in a field using gravity-driven flow. The system includes 35 m of 1.5-cm-diameter galvanized iron piping with four miter bends (90°) without vanes and a wide-open globe valve.
> Reconsider Prob. 14–74E. Using appropriate software, investigate the effect of the pipe diameter on the required electric power input to the pump. Let the pipe diameter vary from 1 to 10 in, in increments of 1 in. Tabulate and plot the results, and draw
> A farmer is to pump water at 70°F from a river to a water storage tank nearby using a 125-ft-long, 5-in-diameter plastic pipe with three flanged 90° smooth bends. The water velocity near the river surface is 6 ft/s, and the pipe inlet is placed in the ri
> As a spherical ammonia vapor bubble rises in liquid ammonia, its diameter changes from 1 cm to 3 cm. Calculate the amount of work produced by this bubble, in kJ, if the surface tension of ammonia is 0.02 N/m.
> A 4-m-high cylindrical tank having a cross-sectional area of AT = 1.5 m2 is filled with equal volumes of water and oil whose specific gravity is SG = 0.75. Now a 1-cm-diameter hole at the bottom of the tank is opened, and water starts to flow out. If the
> Gasoline (ρ = 680 kg/m3 and ν = 4.29 × 10−7 m2/s) is transported at a rate of 240 L/s for a distance of 2 km. The surface roughness of the piping is 0.03 mm. If the head loss due to pipe friction is not to exceed 10 m, determine the minimum diameter of t
> Reconsider Prob. 14–70. In order to drain the tank faster, a pump is installed near the tank exit as in Fig. P14–71. Determine how much pump power input is necessary to establish an average water velocity of 4 m/s when
> A 3-m-diameter tank is initially filled with water 2 m above the center of a sharp-edged 10-cm diameter orifice. The tank water surface is open to the atmosphere, and the orifice drains to the atmosphere through a 100-m-long pipe. The friction coefficie
> Consider the flow of air and water in pipes of the same diameter, at the same temperature, and at the same mean velocity. Which flow is more likely to be turbulent? Why?
> A 2.4-m-diameter tank is initially filled with water 4 m above the center of a sharp-edged 10-cm diameter orifice. The tank water surface is open to the atmosphere, and the orifice drains to the atmosphere. Neglecting the effect of the kinetic energy cor
> Water at 70°F flows by gravity from a large reservoir at a high elevation to a smaller one through a 60-ft-long, 2-in-diameter cast iron piping system that includes four standard flanged elbows, a well-rounded entrance, a sharp-edged exit, and a fully op
> The water needs of a small farm are to be met by pumping water from a well that can supply water continuously at a rate of 5 L/s. The water level in the well is 20 m below the ground level, and water is to be pumped to a large tank on a hill, which is 58
> A semi-spherical tank of radius R is completely filled with water. Now a hole of cross-sectional area Ah and discharge coefficient Cd at the bottom of the tank is fully opened and water starts to flow out. Develop an expression for the time needed to emp
> Water at 15°C is drained from a large reservoir using two horizontal plastic pipes connected in series. The first pipe is 13 m long and has a 10-cm diameter, while the second pipe is 35 m long and has a 5-cm diameter. The water level in the re
> A damaged 1200-kg car is being towed by a truck. Neglecting the friction, air drag, and rolling resistance, determine the extra power required (a) for constant velocity on a level road, (b) for constant velocity of 50 km/h on a 30° (from horizontal) uphi
> A piping system involves two pipes of different diameters (but of identical length, material, and roughness) connected in parallel. How would you compare the (a) flow rates and (b) pressure drops in these two pipes?
> A piping system involves two pipes of different diameters (but of identical length, material, and roughness) connected in series. How would you compare the (a) flow rates and (b) pressure drops in these two pipes?
> Consider two identical 2-m-high open tanks filled with water on top of a 1-m-high table. The discharge valve of one of the tanks is connected to a hose whose other end is left open on the ground while the other tank does not have a hose connected to its
> A person filling a bucket with water using a garden hose suddenly remembers that attaching a nozzle to the hose increases the discharge velocity of water and wonders if this increased velocity would decrease the filling time of the bucket. What would hap
> For a piping system, define the system curve, the characteristic curve, and the operating point on a head versus flow rate chart.
> Which fluid at room temperature requires a larger pump to flow at a specified velocity in a given pipe: water or engine oil? Why?
> Water is pumped from a large lower reservoir to a higher reservoir. Someone claims that if the head loss is negligible, the required pump head is equal to the elevation difference between the free surfaces of the two reservoirs. Do you agree?
> A piping system equipped with a pump is operating steadily. Explain how the operating point (the flow rate and the head loss) is established.
> Water is to be withdrawn from an 8-m-high water reservoir by drilling a 2.2-cm-diameter hole at the bottom surface. Disregarding the effect of the kinetic energy correction factor, determine the flow rate of water through the hole if (a) the entrance of
> Repeat Prob. 14–55 for a slightly rounded entrance (KL = 0.12). Data from Prob. 14-55: Consider flow from a water reservoir through a circular hole of diameter D at the side wall at a vertical distance H from the free surface. The flow
> The engine of a 1500-kg automobile has a power rating of 75 kW. Determine the time required to accelerate this car from rest to a speed of 100 km/h at full power on a level road. Is your answer realistic?
> A horizontal pipe has an abrupt expansion from D1 = 5 cm to D2 = 10 cm. The water velocity in the smaller section is 8 m/s and the flow is turbulent. The pressure in the smaller section is P1 = 410 kPa. Taking the kinetic energy correction factor to b
> During a retrofitting project of a fluid flow system to reduce the pumping power, it is proposed to install vanes into the miter elbows or to replace the sharp turns in 90° miter elbows by smooth curved bends. Which approach will result in a greater redu
> A piping system involves sharp turns, and thus large minor head losses. One way of reducing the head loss is to replace the sharp turns with circular elbows. What is another way?
> Which has a greater minor loss coefficient during pipe flow: gradual expansion or gradual contraction? Why?
> The effect of rounding of a pipe exit on the loss coefficient is (a) negligible, (b) somewhat significant, or (c) very significant.
> Show that the Reynolds number for flow in a circular pipe of diameter D can be expressed as Re = 4m/(πDμ).
> Define equivalent length for minor loss in pipe flow. How is it related to the minor loss coefficient?
> What is minor loss in pipe flow? How is the minor loss coefficient KL defined?
> Consider the fully developed flow of glycerin at 40°C through a 70-m-long, 4-cm-diameter, horizontal, circular pipe. If the flow velocity at the centerline is measured to be 6 m/s, determine the velocity profile and the pressure difference acr
> A ski lift has a one-way length of 1 km and a vertical rise of 200 m. The chairs are spaced 20 m apart, and each chair can seat three people. The lift is operating at a steady speed of 10 km/h. Neglecting friction and air drag and assuming that the avera
> Liquid ammonia at −20°C is flowing through a 20-m-long section of a 5-mm-diameter copper tube at a rate of 0.09 kg/s. Determine the pressure drop, the head loss, and the pumping power required to overcome the frictional losses in the tube.
> Reconsider Prob. 14–44. Using appropriate software, investigate the effect of the pipe diameter on the pressure drop for the same constant flow rate. Let the pipe diameter vary from 1 to 10 cm in increments of 1 cm. Tabulate and plot the results, and dra
> Glycerin at 40°C with ρ = 1252 kg/m3 and μ = 0.27 kg/m·s is flowing through a 6-cm-diameter horizontal smooth pipe with an average velocity of 3.5 m/s. Determine the pressure drop per 10 m of the pipe.
> In an air heating system, heated air at 40°C and 105 kPa absolute is distributed through a 0.2 m × 0.3 m rectangular duct made of commercial steel at a rate of 0.5 m3/s. Determine the pressure drop and head loss through a 40-m-long section of the duct.
> Oil with a density of 850 kg/m3 and kinematic viscosity of 0.00062 m2/s is being discharged by a 8-mm-diameter, 40-m-long horizontal pipe from a storage tank open to the atmosphere. The height of the liquid level above the center of the pipe is 4 m. Disr
> Air at 1 atm and 60°F is flowing through a 1 ft × 1 ft square duct made of commercial steel at a rate of 1600 cfm. Determine the pressure drop and head loss per ft of the duct.
> Glycerin at 40°C with ρ = 1252 kg/m3 and μ = 0.27 kg/m·s is flowing through a 3-cm-diameter, 25-m-long pipe that discharges into the atmosphere at 100 kPa. The flow rate through the pipe is 0.075 L/s. (a) Determine the absolute pressure 25 m before the p
> Consider a person walking first in air and then in water at the same speed. For which motion will the Reynolds number be higher?
> Consider an air solar collector that is 1 m wide and 4 m long and has a constant spacing of 3 cm between the glass cover and the collector plate. Air flows at an average temperature of 45°C at a rate of 0.12 m3/s through the 1-m-wide edge of t
> Air enters a 10-m-long section of a rectangular duct of cross section 15 cm × 20 cm made of commercial steel at 1 atm and 35°C at an average velocity of 5 m/s. Disregarding the entrance effects, determine the fan power needed to o
> List the forms of energy that contribute to the internal energy of a system.
> The acceleration of high-speed aircraft is sometimes expressed in g’s (in multiples of the standard acceleration of gravity). Determine the upward force, in N, that a 90-kg man would experience in an aircraft whose acceleration is 6 g’s.
> Repeat Prob. 14–36 for turbulent flow in smooth pipes for which the friction factor is given as f = 0.184Re−0.2. What would your answer be for fully turbulent flow in a rough pipe?
> Consider laminar flow of a fluid through a square channel with smooth surfaces. Now the average velocity of the fluid is doubled. Determine the change in the head loss of the fluid. Assume the flow regime remains unchanged.
> Water at 10°C (ρ = 999.7 kg/m3 and μ = 1.307 × 10−3 kg/m·s) is flowing steadily in a 0.12-cm diameter, 15-m-long pipe at an average velocity of 0.9 m/s. Determine (a) the pressure drop, (b) the head loss, and (c) the pumping power requirement to overcome
> Repeat Prob. 14–33 for a pipe of inner radius 7 cm. Data from Prob. 14-33: The velocity profile in fully developed laminar flow in a circular pipe of inner radius R = 2 cm, in m/s, is given by u(r) = 4(1 − r2/R2). Det
> The velocity profile in fully developed laminar flow in a circular pipe of inner radius R = 2 cm, in m/s, is given by u(r) = 4(1 − r2/R2). Determine the average and maximum velocities in the pipe and the volume flow rate.
> In fully developed laminar flow in a circular pipe, the velocity at R/2 (midway between the wall surface and the centerline) is measured to be 11 m/s. Determine the velocity at the center of the pipe.
> Heated air at 1 atm and 100°F is to be transported in a 400-ft-long circular plastic duct at a rate of 12 ft3/s. If the head loss in the pipe is not to exceed 50 ft, determine the minimum diameter of the duct.
> Water at 70°F passes through 0.75-in-internal-diameter copper tubes at a rate of 0.5 lbm/s. Determine the pumping power per ft of pipe length required to maintain this flow at the specified rate.
> What is the physical significance of the Reynolds number? How is it defined for (a) flow in a circular pipe of inner diameter D and (b) flow in a rectangular duct of cross section a × b?
> Water at 15°C (ρ = 999.1 kg/m3 and μ = 1.138 × 10−3 kg/m·s) is flowing steadily in a 30-m-long and 6-cm diameter horizontal pipe made of stainless steel at a rate of 10 L/s.
> How much work, in kJ, can a spring whose spring constant is 3 kN/cm produce after it has been compressed 3 cm from its unloaded length?
> The velocity profile for the fully developed laminar flow of a Newtonian fluid between two large parallel plates is given by where 2h is the distance between the two plates, u0 is the velocity at the center plane, and y is the vertical coordinate from th
> What is the physical mechanism that causes the friction factor to be higher in turbulent flow?
> What is turbulent viscosity? What causes it?
> How is head loss related to pressure loss? For a given fluid, explain how you would convert head loss to pressure loss.
> Consider fully developed laminar flow in a circular pipe. If the viscosity of the fluid is reduced by half by heating while the flow rate is held constant, how does the head loss change?
> Consider laminar flow of air in a circular pipe with perfectly smooth surfaces. Do you think the friction factor for this flow is zero? Explain.
> Explain why the friction factor is independent of the Reynolds number at very large Reynolds numbers.
> Consider fully developed laminar flow in a circular pipe. If the diameter of the pipe is reduced by half while the flow rate and the pipe length are held constant, the head loss will (a) double, (b) triple, (c) quadruple, (d) increase by a factor of 8, o
> Consider fully developed flow in a circular pipe with negligible entrance effects. If the length of the pipe is doubled, the head loss will (a) double, (b) more than double, (c) less than double, (d) reduce by half, or (e) remain constant.
> Why are liquids usually transported in circular pipes?
> Determine the torque applied to the shaft of a car that transmits 225 hp and rotates at a rate of 3000 rpm.
> Discuss whether fully developed pipe flow is one-, two-, or three-dimensional.
> How is the friction factor for flow in a pipe related to the pressure loss? How is the pressure loss related to the pumping power requirement for a given mass flow rate?
> How does the wall shear stress τw vary along the flow direction in the fully developed region in (a) laminar flow and (b) turbulent flow?
> Someone claims that in fully developed turbulent flow in a pipe, the shear stress is a maximum at the pipe wall. Do you agree with this claim? Explain.
> Someone claims that the shear stress at the center of a circular pipe during fully developed laminar flow is zero. Do you agree with this claim? Explain.
> Someone claims that the average velocity in a circular pipe in fully developed laminar flow can be determined by simply measuring the velocity at R/2 (midway between the wall surface and the centerline). Do you agree? Explain.
> Someone claims that the volume flow rate in a circular pipe with laminar flow can be determined by measuring the velocity at the centerline in the fully developed region, multiplying it by the cross-sectional area, and dividing the result by 2. Do you ag

