Question: Construct a frequency distribution for the data
Construct a frequency distribution for the data set using the indicated number of classes. In the table, include the midpoints, relative frequencies, and cumulative frequencies. Which class has the greatest class frequency and which has the least class frequency?
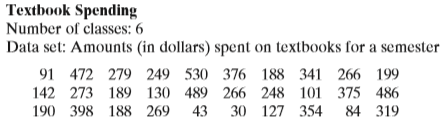
Transcribed Image Text:
Textbook Spending Number of classes: 6 Data set: Amounts (in dollars) spent on textbooks for a semester 91 472 279 249 530 376 188 341 266 199 142 273 189 130 489 266 248 101 375 486 190 398 188 269 43 30 127 354 84 319
> Given a data set, how do you know whether to calculate σ or s?
> The letters A, B, and C are marked on the horizontal axis. Describe the shape of the data. Then determine which is the mean, which is the median, and which is the mode. Justify your answers. Sick Days Used by Employees 16 14 12- 10 4 2 10+ t f14 16 1
> The table at the left shows the U.S. trade deficits (in billions of dollars) with 18 countries in 2015. a. Find the mean and the median of the trade deficits. b. Find the mean and the median without the Chinese trade deficit. Which measure of central t
> During a quality assurance check, the actual contents (in grams) of six containers of protein powder were recorded as 1525, 1526, 1502, 1516, 1529, and 1511. a. Find the mean and the median of the contents. b. The third value was incorrectly measured a
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Finishing Times Number of classes: 8 Data set: Finishing times (in seconds) of 21 participants in a 10K race 3
> At least 99% of the data in any data set lie within how many standard deviations of the mean? Explain how you obtained your answer.
> The English statistician Karl Pearson (1857–1936) introduced a formula for the skewness of a distribution. Most distributions have an index of skewness between -3 and 3. When P > 0, the data are skewed right. When P a. x = 17, s = 2
> Sample annual salaries (in thousands of dollars) for employees at a company are listed. a. Find the sample mean and the sample standard deviation. b. Each employee in the sample receives a $1000 raise. Find the sample mean and the sample standard devia
> Sample annual salaries (in thousands of dollars) for employees at a company are listed. a. Find the sample mean and the sample standard deviation. b. Each employee in the sample receives a 5% raise. Find the sample mean and the sample standard deviatio
> Another useful measure of variation for a data set is the mean absolute deviation (MAD). It is calculated by the formula a. Find the mean absolute deviation of the data set in Exercise 15. Compare your result with the sample standard deviati
> Approximate the mean of the frequency distribution. The ages (in years) of the residents of a small town in 2016 Age (in years) 0-9 Frequency 78 10-19 97 20-29 54 30-39 63 40-49 69 50–59 86 60-69 73 70–79 53 80-89 43 90–99 15
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample grade point averages for ten male students and ten female students are listed. Males 2.4 3.7 3.8 3.9 2.8 2.6 3.6 3.3 4.0 1.9 Females 2.8 3.7 2.1 3.9 3.6
> Describe the difference between the calculation of population standard deviation and that of sample standard deviation.
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample SAT scores for eight males and eight females are listed. Males 1010 1170 1410 920 1320 1100 690 1140 Females 1190 1010 1000 1300 1470 1250 840 1060
> Find the coefficient of variation for each of the two data sets. Then compare the results. The ages (in years) and weight classes (in kilograms) of all members of the 2016 Men’s U.S. Olympic wrestling team are listed. Ages Weight Cl
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Reaction Times Number of classes: 8 Data set: Reaction times (in milliseconds) of 30 adult females to an audit
> Use the frequency distribution in Exercise 15 to construct an expanded frequency distribution, as shown in Example 2. From Exercise 15: Travel Time to Work (in minutes) Class Frequency,S 0-10 188 11-21 372 22-32 264 33-43 205 44-54 83 55-65 76 66-7
> Find the coefficient of variation for each of the two data sets. Then compare the results. The ages (in years) and heights (in inches) of all members of the 2016 Women’s U.S. Olympic swimming team are listed. Ages 24 24 19 23 22 21
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample annual salaries (in thousands of dollars) for entry level software engineers in Raleigh, NC, and Wichita, KS, are listed. Raleigh 63.7 68.4 59.3 50.7 59
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample annual salaries (in thousands of dollars) for entry level architects in Denver, CO, and Los Angeles, CA, are listed. Denver 45.8 46.4 44.4 40.7 51.5 39.
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The amounts of caffeine in a sample of five-ounce servings of brewed coffee are shown in the histogram. 25 2
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The numbers of courses taught per semester by a random sample of university professors are shown in the histogra
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The distribution of the numbers of hours that a random sample of college students study per week is shown in the
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The distribution of the tuitions, fees, and room and board charges of a random sample of public 4-year degree-gr
> Make a frequency distribution for the data. Then use the table to find the sample mean and the sample standard deviation of the data set. 11 10 0 0 0 0 1 0 1 0 0 1 0 1 1 0 0 0 0 1 0 0 1 1 10 0 11 0 0 0 0 0 1 0 1 1 1 0 0 0 0 1 1 0 0 0
> Explain the relationship between variance and standard deviation. Can either of these measures be negative? Explain.
> Make a frequency distribution for the data. Then use the table to find the sample mean and the sample standard deviation of the data set. 3 3 5 3 8 0 39 6 6 7 1 6 3 2 6 9 1 8 5 0 2 3 4 9 5 8 19 7 6 9 6 706 3 8 6 8 7 3 8 9 3 7 2 4 4 1
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Pepper Pungencies Number of classes: 5 Data set: Pungencies (in thousands of Scoville units) of 24 tabasco sCO
> The mean number of runs per game scored by the Chicago Cubs during the 2016 World Series was 3.86 runs, with a standard deviation of 3.36 runs. Apply Chebychev’s Theorem to the data using k = 2. Interpret the results.
> The mean score on a Statistics exam is 82 points, with a standard deviation of 3 points. Apply Chebychev’s Theorem to the data using k = 4. Interpret the results.
> Old Faithful is a famous geyser at Yellowstone National Park. From a sample with n = 100, the mean interval between Old Faithful’s eruptions is 101.56 minutes and the standard deviation is 42.69 minutes. Using Chebychev’s Theorem, determine at least how
> You are conducting a survey on the number of pets per household in your region. From a sample with n = 40, the mean number of pets per household is 2 pets and the standard deviation is 1 pet. Using Chebychev’s Theorem, determine at least how many of the
> Use the Empirical Rule. The monthly utility bills for eight households are listed. Using the sample statistics from Exercise 30, determine which of the data entries are unusual. Are any of the data entries very unusual? Explain your reasoning. $65, $52,
> Use the Empirical Rule. The speeds for eight vehicles are listed. Using the sample statistics from Exercise 29, determine which of the data entries are unusual. Are any of the data entries very unusual? Explain your reasoning. 70, 78, 62, 71, 65, 76, 82,
> Use the Empirical Rule. Use the sample statistics from Exercise 30 and assume the number of households in the sample is 40. a. Estimate the number of households whose monthly utility bills are between $54 and $86. b. In a sample of 20 additional househ
> Use the Empirical Rule. Use the sample statistics from Exercise 29 and assume the number of vehicles in the sample is 75. a. Estimate the number of vehicles whose speeds are between 63 miles per hour and 71 miles per hour. b. In a sample of 25 additiona
> Use the Empirical Rule. The mean monthly utility bill for a sample of households in a city is $70, with a standard deviation of $8. Between what two values do about 95% of the data lie? (Assume the data set has a bell-shaped distribution.)
> Why is the standard deviation used more frequently than the variance?
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Sales Number of classes: 6 Data set: July sales (in dollars) for 21 sales representatives at a company 2114 24
> Use the Empirical Rule. The mean speed of a sample of vehicles along a stretch of highway is 67 miles per hour, with a standard deviation of 4 miles per hour. Estimate the percent of vehicles whose speeds are between 63 miles per hour and 71 miles per ho
> Construct a data set that has the given statistics. n = 6 I = 7 S = 2 s -
> Construct a data set that has the given statistics. n = 7 I = 9 s = 0
> Construct a data set that has the given statistics. N = 8 u = 6
> Construct a data set that has the given statistics. N = 6 M = 5 2
> You are asked to compare three data sets. a. Without calculating, determine which data set has the greatest sample standard deviation and which has the least sample standard deviation. Explain your reasoning. b. How are the data sets the same? How do t
> You are asked to compare three data sets. a. Without calculating, determine which data set has the greatest sample standard deviation and which has the least sample standard deviation. Explain your reasoning. b. How are the data sets the same? How do t
> You are asked to compare three data sets. a. Without calculating, determine which data set has the greatest sample standard deviation and which has the least sample standard deviation. Explain your reasoning. b. How are the data sets the same? How do t
> You are asked to compare three data sets. a. Without calculating, determine which data set has the greatest sample standard deviation and which has the least sample standard deviation. Explain your reasoning. b. How are the data sets the same? How do t
> You are applying for jobs at two companies. Company C offers starting salaries with µ = $59,000 and σ = $1500. Company D offers starting salaries with µ = $59,000 and σ = $1000. From which company are you more likely to get an offer of $62,000 or more? E
> Explain how to find the deviation of an entry in a data set. What is the sum of all the deviations in any data set?
> You are applying for jobs at two companies. Company A offers starting salaries with µ = $41,000 and σ = $1000. Company B offers starting salaries with µ = $41,000 and σ = $5000. From which company are you more likely to get an offer of $43,000 or more? E
> Both data sets shown in the stem-and-leaf plots have a mean of 165. One has a standard deviation of 16, and the other has a standard deviation of 24. By looking at the stem-and-leaf plots, which is which? Explain your reasoning. (a) 12 8 9 Key: 12|8
> Both data sets shown in the histograms have a mean of 50. One has a standard deviation of 2.4, and the other has a standard deviation of 5. By looking at the histograms, which is which? Explain your reasoning. (a) (b) 20 20 15 15 10 10 42 45 48 51 54
> Find the range, mean, variance, and standard deviation of the sample data set. The durations (in days) of pregnancies for a random sample of mothers 277 291 295 280 268 278 291 277 282 279 296 285 269 293 267 281 286 269 264 299 275
> Find the range, mean, variance, and standard deviation of the sample data set. The ages (in years) of a random sample of students in a campus dining hall 19 20 17 19 17 21 23 21 17 17 19 19 17 20 23 18 18 18 18 19
> Find the range, mean, variance, and standard deviation of the population data set. The densities (in kilograms per cubic meter) of the ten most abundant elements by weight in Earth’s crust 1.4 2330 2700 7870 1500 970 900 1740 4500 0
> Find the range, mean, variance, and standard deviation of the population data set. The numbers of alcohol-impaired crash fatalities (in thousands) per year from 2005 through 2015 14 13 13 12 11 10 10 10 10 10 10
> In Exercise 11, compare your answer to part (a) with your answer to part (b). How do outliers affect the range of a data set? From Exercise 11: The depths (in inches) at which 10 artifacts are found are listed. a. Find the range of the data set. b. Ch
> The depths (in inches) at which 10 artifacts are found are listed. a. Find the range of the data set. b. Change 38.5 to 60.5 and find the range of the new data set. 20.7 24.8 30.5 26.2 36.0 34.3 30.3 29.5 27.0 38.5
> What is the difference between class limits and class boundaries?
> Find the range of the data set represented by the graph. 75 80 85 90 95 ::
> Explain how to find the range of a data set. What is an advantage of using the range as a measure of variation? What is a disadvantage?
> Determine whether the approximate shape of the distribution in the histogram is symmetric, uniform, skewed left, skewed right, or none of these. Justify your answer. 22 20 18 16 14 12 10 8 6- 4- 2 25,000 45,000 65,000 85,000
> Construct the described data set. The entries in the data set cannot all be the same. Mean, median, and mode are the same.
> Construct the described data set. The entries in the data set cannot all be the same. Mean is not representative of a typical number in the data set.
> Construct the described data set. The entries in the data set cannot all be the same. Mean and mode are the same.
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe the shape of the histogram as symmetric, uniform, negatively skewed, positively skewed, or none of these. Number of classes: 6
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe the shape of the histogram as symmetric, uniform, negatively skewed, positively skewed, or none of these. Number of classes: 5
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe the shape of the histogram as symmetric, uniform, negatively skewed, positively skewed, or none of these. Number of classes: 6
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe the shape of the histogram as symmetric, uniform, negatively skewed, positively skewed, or none of these. Number of classes:
> Construct a frequency distribution for the data set using the indicated number of classes. In the table, include the midpoints, relative frequencies, and cumulative frequencies. Which class has the greatest class frequency and which has the l
> Approximate the mean of the frequency distribution. The populations (in thousands) of the parishes of Louisiana in 2015 Population (in thousands) 0-49 Frequency 41 50-99 100–149 150–199 2 200-249 1 250–299 2 300–349 350–399 1 400-449 2
> Approximate the mean of the frequency distribution. The gas mileages (in miles per gallon) for 24 family sedans Gas Mileage (in miles per gallon) Frequency 22–27 16 28-33 2 34–39 2 40-45 4
> Construct the described data set. The entries in the data set cannot all be the same. Median and mode are the same.
> Approximate the mean of the frequency distribution. The gas mileages (in miles per gallon) for 30 small cars Gas Mileage (in miles per gallon) Frequency 29–33 11 34–38 12 39-43 2 44-48 5
> In Exercise 46, one of the student’s B grades gets changed to an A. What is the student’s new grade point average? From Exercise 46: A student receives the grades shown below, with an A worth 4 points, a B worth 3 points, a C worth 2 points, and a D wor
> In Exercise 41, an error was made in grading your final exam. Instead of getting 93, you scored 85. What is your new weighted mean? From Exercise 41: The scores and their percents of the final grade for a statistics student are shown below. What is the
> Find the weighted mean of the data. A student receives the grades shown below, with an A worth 4 points, a B worth 3 points, a C worth 2 points, and a D worth 1 point. What is the student’s grade point average? A in 1 four-credit class B in 2 three-cred
> Find the weighted mean of the data. The mean scores for students in a statistics course (by major) are shown below. What is the mean score for the class? 9 engineering majors: 85 5 math majors: 90 13 business majors: 81
> Find the weighted mean of the data. For the month of October, a credit card has a balance of $115.63 for 12 days, $637.19 for 6 days, $1225.06 for 7 days, $0 for 2 days, and $34.88 for 4 days. What is the account’s mean daily balance for October?
> Find the weighted mean of the data. For the month of April, a checking account has a balance of $523 for 24 days, $2415 for 2 days, and $250 for 4 days. What is the account’s mean daily balance for April?
> Use the ogive in Exercise 25 to approximate a. the cumulative frequency for a weight of 201.5 pounds. b. the weight for which the cumulative frequency is 68. c. the number of black bears that weigh between 158.5 pounds and 244.5Â pounds. d
> Find the weighted mean of the data. The scores and their percents of the final grade for an archaeology student are shown below. What is the student’s mean score? Score Percent of final grade Quizzes 100 20% Midterm exam 89 30% Stud
> Find the weighted mean of the data. The scores and their percents of the final grade for a statistics student are shown below. What is the student’s mean score? Score Percent of final grade Homework 85 5% Quizzes 80 35% Project/Spee
> Without performing any calculations, determine which measure of central tendency best represents the graphed data. Explain your reasoning. Body Mass Indexes (BMI) of People in a Gym 8 3. 18 20 2 24 26 28 30 BMI kouonbosa
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. When each data class has the same frequency, the distribution is symmetric.
> Without performing any calculations, determine which measure of central tendency best represents the graphed data. Explain your reasoning. Heart Rates of a Sample of Adults 45 40- 35 15 10 5s 60 65 0 is 80 85 Heart rate (in bcats per minute) Frequenc
> Without performing any calculations, determine which measure of central tendency best represents the graphed data. Explain your reasoning. Heights of Players on Two Opposing Volleyball Teams 6. 70 71 12 73 74 75 76 7 Height (in inches) kouan basg
> Without performing any calculations, determine which measure of central tendency best represents the graphed data. Explain your reasoning. How Often Do You Change Jobs? 1250 1000 750- 500 250 Every 1-3 years Every 4-5 yearn Stayed at one job for more
> Identify any clusters, gaps, or outliers. Model Year 2017 Hybrid Electric Cars 600 1200 Annual fuel cost (in dollars) 1800 2700 3000 Frequency -N -u 900 1500 2100 2400
> Identify any clusters, gaps, or outliers. Model Year 2017 Ethanol Flexible Fuel Vehicles 20 16 12 250 300 350 400 450 500 550 600 Driving range (in miles) Frequency
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. Prices (in dollars) of Flights from Chicago to Alanta 100 140 180 220 260 300
> Use the ogive in Exercise 25 to approximate a. the cumulative frequency for a weight of 201.5 pounds. b. the weight for which the cumulative frequency is 68. c. the number of black bears that weigh between 158.5 pounds and 244.5 pounds. d. the number
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. Times (in minutes) It Takes Employees to Drive to Work 10 15 20 25 30 35 40
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. Grade Point Averages of Students in a Class o | 8 15 6 8 21 3 4 5 30 4 0 0 Key: 0|8 = 0.8
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. Weights (in pounds) of Packages on a Delivery Truck 0 5 8 1013 6 2 13 3 3 6 77 3 012 4 4 4 5 7
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. The pie chart at the left shows the responses of a sample of 352 small-business owners who were as
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. A data set can have the same mean, median, and mode.
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. The class levels of 25 students in a physics course Freshman: 2 Junior: 10 Sophomore: 5 Senior
> Find the mean, the median, and the mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. The responses of a sample of 34 young adult United Kingdom males in custodial sentences who were a

