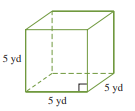
Question: Determine (a) the volume and (b) the
Determine (a) the volume and (b) the surface area of the three-dimensional figure. When appropriate, use the

key on your calculator and round your answer to the nearest hundredth.
> Arrange the quantities in order from smallest to largest. 4.3ℓ, 420 cℓ , 0.045 kℓ
> Use the figure to determine the following: //
> Arrange the quantities in order from smallest to largest. 514 hm, 62 km, 680 m
> Identify the figure as a line, half line, ray, line segment, open line segment, or half open line segment. Denote the figure by its appropriate symbol.
> Use the following information about the lion’s mane jellyfish to answer the questions. The largest species of jellyfish is the lion’s mane jellyfish. The largest lion’s mane jellyfish specimen ever di
> x2 + 5x + 4 Factor the trinomial.
> x2 + 5x – 14 Factor the trinomial.
> Use the following information about the lion’s mane jellyfish to answer the questions. The largest species of jellyfish is the lion’s mane jellyfish. The largest lion’s mane jellyfish specimen ever di
> 3x2 = 9x – 5 Solve the equation, using the quadratic formula. If the equation has no real solution, so state.
> Use the following information about the lion’s mane jellyfish to answer the questions. The largest species of jellyfish is the lion’s mane jellyfish. The largest lion’s mane jellyfish specimen ever di
> 3x2 - 6x - 5 = 0 Solve the equation, using the quadratic formula. If the equation has no real solution, so state.
> Use the following information about the lion’s mane jellyfish to answer the questions. The largest species of jellyfish is the lion’s mane jellyfish. The largest lion’s mane jellyfish specimen ever di
> 2x2 + 4x + 1 = 0 Solve the equation, using the quadratic formula. If the equation has no real solution, so state.
> Convert the given unit to the unit indicated. 0.034 mℓ to deciliters
> Solve the equation, using the quadratic formula. If the equation has no real solution, so state. 2x2 - 5x - 2 = 0
> 895 kℓ to milliliters Convert the given unit to the unit indicated.
> Use your intuition to determine whether the variation between the indicated quantities is direct or inverse. The diameter of a hose and the volume of water coming out of the hose
> Solve the equation, using the quadratic formula. If the equation has no real solution, so state. x2 - 6x - 16 = 0
> 69.3 kg to hectograms Convert the given unit to the unit indicated.
> Solve the equation, using the quadratic formula. If the equation has no real solution, so state. x2 - 11x + 24 = 0
> Use the photo taken in Canada, which shows the maximum mass for vehicles allowed on the street. What is the maximum mass in milligrams?
> 4x2 + x - 3 = 0 Solve each equation by factoring.
> Without referring to any of the tables or your notes, give the symbol and the equivalent in grams for the unit. Hectogram
> 5x2 = 14x – 8 Solve each equation by factoring.
> Without referring to any of the tables or your notes, give the symbol and the equivalent in grams for the unit. Dekagram
> 2x2 = -5x + 3 Solve each equation by factoring.
> Without referring to any of the tables or your notes, give the symbol and the equivalent in grams for the unit. Milligram
> Determine (a) the area and (b) the perimeter of the quadrilateral.
> 3x2 - 5x = 2 Solve each equation by factoring.
> Complete the following. a) 1 centiliter = _________ liter b) 1 dekaliter = _________ liters c) 1 milliliter = _________ liter d) 1 deciliter = _________ liter e) 1 kiloliter = _________ liters f) 1 hectoliter = _________ liters
> x2 + 12x + 20 = 0 Solve each equation by factoring.
> Match the given prefix with the one letter, a)–f), that gives the meaning of the prefix. Centi a) 1/100 of base unit b) 1/1000 of base unit c) 100 times base unit d) 1000 times base unit e) 10 times base unit f ) 1/10 of base unit
> x2 - 64 = 0 Solve each equation by factoring.
> Determine whether the graph represents a function. If it does represent a function, give its domain and range.
> x2 - 13x + 40 = 0 Solve each equation by factoring.
> Determine whether the graph represents a function. If it does represent a function, give its domain and range.
> x2 - 7x = -6 Solve each equation by factoring.
> U.S. Cellular Telephone Subscribers The graph below shows the number of U.S. cellular subscribers, in thousands, for the years 1993 through 2012. (a) Does the graph approximate the graph of an exponential function? (b) Estimate the number of U.S. cellula
> (a) Name the polygon. If the polygon is a quadrilateral, give its specific name. (b) State whether or not the polygon is a regular polygon.
> x2 - 9x + 18 = 0 Solve each equation by factoring.
> Radioactive Decay Strontium-90 is a radioactive isotope that decays exponentially at a rate of 2.8% per year. The amount of strontium-90, S, remaining after t years can be found by the function S1t2 = S0e-0.028t , where S0 is the original amount present.
> x2 + 5x - 6 = 0 Solve each equation by factoring.
> Value of New York City Assume the value of the island of Manhattan has grown at an exponential rate of 8% per year since 1626 when Peter Minuit of the Dutch West India Company purchased the island for $24. The value of the island, V, at any time, t, in y
> Solve each equation, using the zero-factor property. (x – 6)(5x - 42 = 0
> Value of an SUV The cost of a new SUV is $30,000. If it depreciates at a rate of 18% per year, the value of the SUV in t years can be approximated by the function V(t) = 30,000(0.82)t . Determine the value of the SUV in 4 years. Round your answer to the
> Solve each equation, using the zero-factor property. (2x + 3)(x – 1) = 0
> Adjusting for Inflation If P is the price of an item today, the price of the same item n years from today, Pn, is Pn = P(1 + r)n , where r is the constant rate of inflation. Determine the price of a movie ticket 10 years from today if the price today is
> 6x2 - 13x + 2 Factor the trinomial.
> Finding Distances The distance a car travels, d1t2, at a constant 60 mph is given by the function d1t2 = 60t, where t is the time in hours. Find the distance traveled in (a) 3 hours. (b) 7 hours.
> In which quadrants will the set of points that satisfy the equation x + y = 1 lie? Explain.
> Draw the graph of the function and state the domain and range. f (1x) = 4x+1
> 6x2 - 11x + 4 Factor the trinomial.
> Draw the graph of the function and state the domain and range. y = 2x - 1
> Recreational Vehicles The green graph shows the number of recreational vehicles sold in the United States, in thousands, for the years 2009–2014. The red dashed line can be used to approximate the number of recreational vehicles, in tho
> Draw the graph of the function and state the domain and range. y = 3x - 1
> 4x2 + 20x + 21 Factor the trinomial.
> Draw the graph of the function and state the domain and range.
> Determining a Test Grade The graph below shows the hours studied and the test grades on a biology test for 7 students. The red line on the graph can be used to approximate the test grade the average student receives for the number of hours he or she stud
> Draw the graph of the function and state the domain and range. f (x) = 4x
> x2 + 5x + 4 Factor the trinomial.
> 2x2 - 9x + 10 Factor the trinomial.
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> Earning Simple Interest When $1000 is invested in a savings account paying simple interest for a year, the interest, i, in dollars, earned can be found by the formula i = 1000r, where r is the interest rate in decimal form. (a) Graph i = 1000r, for r up
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> 2x2 - x – 3 Factor the trinomial.
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> O. Selling Chocolates Ryan sells chocolate on the Internet. His monthly profit, p, in dollars, can be estimated by p = 15n - 300, where n is the number of dozens of chocolates he sells in a month. (a) Graph p = 15n - 300, for n ( 60. (b) From the graph,
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> Factor the trinomial. 3x2 - 7x + 2
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> The radius of a balloon and its volume Use your intuition to determine whether the variation between the indicated quantities is direct or inverse.
> For what value of b will the line joining the points P and Q be parallel to the indicated axis? P(-6, 2b + 3), Q(7, -12); x-axis
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> x2 + 2x - 48 Factor the trinomial.
> (a) Determine whether the parabola will open upward or downward. (b) Find the equation of the axis of symmetry. (c) Find the vertex. (d) Find the y-intercept. (e) Find the x-intercepts if they exist. (f) Sketch the graph. (g) Find the domain and range of
> For what value of b will the line joining the points P and Q be parallel to the indicated axis? P (4, 7), Q (b, -20; y-axis
> Graph the function by using the slope and y-intercept. /
> x2 + 4x – 32 Factor the trinomial.
> Graph the function by using the slope and y-intercept. f (x) = 2x + 5
> Points A, B, and C are three vertices of a parallelogram with sides parallel to the x-axis. Plot the three points. Determine the coordinates of the fourth point, D, to complete the parallelogram. Note: There are two possible answers for point D. A (-2, 2
> Graph the function by using the slope and y-intercept. f (x) = 2x + 1
> Determine the area of the triangle.
> x2 – 64 Factor the trinomial.
> Evaluate the function for the given value of x. f (x) = -3x2 - 6x + 10, x = -2
> Points A, B, and C are three vertices of a rectangle. Plot the three points. (a) Determine the coordinates of the fourth point, D, to complete the rectangle. (b) Determine the area of the rectangle; use A = lw. A(-4, 2), B(7, 2), C(7, 8)
> f (x) = 3x2 + 2x - 5, x = -4 Evaluate the function for the given value of x.
> x2 - x - 30 Factor the trinomial.
> f (x) = -2x2 + 3x - 1, x = 3 Evaluate the function for the given value of x.
> Determine the equation of the graph.
> f (x) = x2 - 5, x = 7 Evaluate the function for the given value of x.
> x2 - 5x – 6 Factor the trinomial.
> Speed of Light Light travels at about 186,000 miles per second through space. The distance, d, in miles that light travels in t seconds can be determined by the function d(t) = 186,000t. (a) Light reaches the moon from Earth in about 1.3 sec. Determine t
> The time required to fill a pool with a hose and the volume of water coming from the hose Use your intuition to determine whether the variation between the indicated quantities is direct or inverse.
> Determine the equation of the graph.
> f(x) = 8x + 2, x = -2 Evaluate the function for the given value of x.
> Are indicated on the graph in Fig. 6.22.Write the coordinates of the indicated point.
> Appreciation of a House A house initially cost $150,000. The value, V, of the house after n years if it appreciates at a constant rate of 4% per year can be determined by the function V = f(n) = +150,000(1.04)n. (a) Determine f(8) and explain its meaning
> Graph the equation using the slope and y-intercept, as in Examples 6 and 7. 3x + 4y - 8 = 0
> Evaluate the function for the given value of x. f (x) = 4x - 1, x = 2
> Are indicated on the graph in Fig. 6.22.Write the coordinates of the indicated point.

