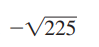Question: Evaluate the expression. /
> Find (a) the greatest common divisor (GCD) and (b) the least common multiple (LCM). 32 and 224
> Perform the indicated operation.
> Express each terminating decimal number as a quotient of two integers. If possible, reduce the quotient to lowest terms. 0.251
> Perform the indicated operation without the use of a calculator and express each answer in decimal notation. (b) Confirm your answer from part (a) by using a scientific calculator to perform the operations. If the calculator displays the answer in scient
> Find (a) the greatest common divisor (GCD) and (b) the least common multiple (LCM). 12 and 21
> Perform the indicated operation.
> Express each terminating decimal number as a quotient of two integers. If possible, reduce the quotient to lowest terms. 0.375
> 2. If a is a factor of b, then b, a is a(n) _________. 4. A natural number greater than 1 that has exactly two factors, itself and 1, is known as a(n) _________ number. 6. The largest natural number that divides (without remainder) each number in a set o
> Perform the indicated operation without the use of a calculator and express each answer in decimal notation. (b) Confirm your answer from part (a) by using a scientific calculator to perform the operations. If the calculator displays the answer in scient
> Find the prime factorization of the number. 3190
> Simplify the radical.
> Express each terminating decimal number as a quotient of two integers. If possible, reduce the quotient to lowest terms. 0.13
> Perform the indicated operation without the use of a calculator and express each answer in decimal notation. (b) Confirm your answer from part (a) by using a scientific calculator to perform the operations. If the calculator displays the answer in scient
> Find the prime factorization of the number. 663
> Simplify the radical.
> Express each rational number as terminating or repeating decimal number.
> Express the number in decimal notation. 4.6×101
> Find the prime factorization of the number. 399
> 2. A symbol that represents a specific quantity is called a(n)______. 4. The number or numbers that replace the variable to make an equation a true statement is called ________the to the equation. 6. Terms that have the same variables with the same expon
> Simplify the radical.
> Express each rational number as terminating or repeating decimal number.
> Express the number in decimal notation. 2.19 ×10-4
> Find the prime factorization of the number. 315
> Simplify the radical.
> Express each rational number as terminating or repeating decimal number.
> Express the number in decimal notation. 4.07 × 10-3
> Find the prime factorization of the number. 98
> Classify the number as a member of one or more of the following sets: the rational numbers, the integers, the natural numbers, the irrational numbers. 0.123112311123……
> Express each rational number as terminating or repeating decimal number.
> 2. The symbol ( is called the ___________ sign. 4. In the radical expression 3(5, the 3 is called the _______________ of the radical expression. 6. Any number that is the square of a natural number is called a(n) _________ square. 8. Consider the irrati
> Express the number in decimal notation. 5.3 × 104
> Determine whether the number is divisible by each of the following numbers: 2, 3, 4, 5, 6, 8, 9, and 10. Determine a number that is divisible by 3, 4, 5, 9, and 10.
> Classify the number as a member of one or more of the following sets: the rational numbers, the integers, the natural numbers, the irrational numbers. 0.123123123
> Convert each improper fraction to a mixed number.
> Express the number in scientific notation. 0.0003
> Determine whether the number is divisible by each of the following numbers: 2, 3, 4, 5, 6, 8, 9, and 10. 3,941,221
> Classify the number as a member of one or more of the following sets: the rational numbers, the integers, the natural numbers, the irrational numbers. 2.718
> Convert each improper fraction to a mixed number.
> Express the number in scientific notation. 1,260,000,000
> Determine whether the number is divisible by each of the following numbers: 2, 3, 4, 5, 6, 8, 9, and 10. 170,820
> 2. With the quotient rule for exponents, the expression can be simplified to ________. 4. With the negative exponent rule, for x ≠0, the expression x-5 can be simplified to ________. 6. A number written in scientific notation is a num
> Classify the number as a member of one or more of the following sets: the rational numbers, the integers, the natural numbers, the irrational numbers.
> Convert each improper fraction to a mixed number.
> Express the number in scientific notation. 0.00467
> Determine whether the number is divisible by each of the following numbers: 2, 3, 4, 5, 6, 8, 9, and 10. 23,800
> Classify the number as a member of one or more of the following sets: the rational numbers, the integers, the natural numbers, the irrational numbers. -5
> Write the number of inches indicated by the arrows as an improper fraction.
> Express the number in scientific notation. 0.000034
> Determine whether the statement is true or false. Modify each false statement to make it a true statement. If a number is divisible by 3 and 5, then the number is divisible by 15.
> Write the number of inches indicated by the arrows as an improper fraction.
> 2. The irrational number is known as the __________ number. 4. A rectangle whose ratio of its length to its width is equal to the golden number is known as a golden ________. 6. The proportion is called the golden _________.
> Express the number in scientific notation. 907,000,000
> Determine whether the statement is true or false. Modify each false statement to make it a true statement. If a number is not divisible by 10, then it is not divisible by 5.
> Evaluate the expression.
> Convert each mixed number to an improper fraction.
> Evaluate the expression. Assume x ≠ 0. (a)(-5)-2 (-5)-2 (b)(-1)-5 (-1)-5
> Determine whether the statement is true or false. Modify each false statement to make it a true statement. 8 is a divisor of 56.
> Evaluate the expression.
> Convert each mixed number to an improper fraction.
> Evaluate the expression. Assume x ≠0.
> Determine whether the statement is true or false. Modify each false statement to make it a true statement. 49 is a factor of 7.
> 2. The numbers that form a sequence are called its _________. 4. The amount by which each pair of successive terms differ in an arithmetic sequence is called the common _________. 6. The constant found by dividing any term in a geometric sequence by the
> Evaluate the expression.
> Convert each mixed number to an improper fraction.
> Evaluate the expression. Assume x ≠ 0. ( a) -5-2 (b) (-5)-2
> Determine whether the statement is true or false. Modify each false statement to make it a true statement. 48 is a multiple of 8.
> P = i2r; determine r when p = 62,500 and i = 5(electronics).
> Determine the volume of the block shown in Fig. 6.2, excluding the hole. The formula for the volume of a rectangular solid is V = lwh. The formula for the volume of a cylinder is V = πr2 h.
> Determine whether the number is rational or irrational.
> Volume of an Ice-Cream Cone An ice-cream cone is filled with ice cream to the top of the cone. Determine the volume, in cubic inches, of ice cream in the cone if the cone’s radius is 1.5 in. and the height is 7 in. (See the figure.) The
> Savings Account Christine borrowed $5200 from a bank at a simple interest rate of 2.5% (or 0.025) for one year. (a) Determine how much interest Christine paid at the end of 1 year. (b) Determine the total amount Christine will repay the bank at the end o
> Solve for the variable indicated. A = P(1 + rt) for t
> Solve for the variable indicated. C= 5/9(F – 32) for F
> Solve for the variable indicated.
> Solve for the variable indicated. y = mx + b for x
> Solve for the variable indicated. V = 1/3πr2 h for h
> Solve for the variable indicated. C= 2 πr for r
> Solve for the variable indicated. P= a + b + S 1 + S2 for S1
> Solve for the variable indicated. V = lwh for w
> Reduce each fraction to lowest terms.
> Solve the equation for y 10x - 3y = 0
> Solve the equation for y -9x + 4y = 11
> Solve the equation for y 4x - 2y = 10
> Determine A when P = 100, r = 6% (or 0.06) n = 1, and t = 3 (banking).
> P = nRT/V; determine V when P = 12, n = 10, R = 60, and T = 8 (chemistry).
> c =√a2 + b2; determine c when a = 5 and b = 12 (geometry).
> X= ;determine x when a = 2,b = -5, and c = -12 (mathematics).
> Determine z when x = 66, m = 60, s = 15, and n = 25 (statistics).
> C = 5/9 (F – 32); determine C when F = 77 (temperature conversion)
> V = 1/3 πr2. h; determine h when V = 47.10 and r = 3 (geometry).
> Evaluate the expression. Assume x ≠ 0. (a) 5-1 (b) 2-4
> Determine h when A = 36, b1 = 4, and b2 = 8 (geometry).
> M = a + b + c/3 ; determine a when m = 85, b = 77, and c = 92 (statistics).
> S = 4lw + 2wh; determine l when S = 336, w = 8, and h = 9 (geometry).
> A = π(R2 - r2 ); determine A when R = 5 and r = 4 (geometry).
> Evaluate the expression for the given value(s) of the variable(s). 4x - 7, x = 5
> Evaluate the expression for the given value(s) of the variable(s). -x3, x = -4
> Evaluate the expression for the given value(s) of the variable(s). -x2 , x = -1
> Evaluate the expression for the given value(s) of the variable(s). x2 , x = 8
> Does (x + y)2 = x2 + y2 ? Complete the table and state your conclusion.