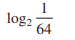[SOLVED] Evaluate the expression without using a

> Sketch a graph of each equation or pair of equations in a rectangular coordinate system. x = -3; y = 2

> Rewrite in equivalent logarithmic form.

> Rewrite in equivalent exponential form.

> solve and graph. -4 ≤ 5x + 6 < 21

> Rewrite in equivalent exponential form.

> The radioactive carbon-14 (14C) in an organism at the time of its death decays according to the equation where t is time in years and A0 is the amount of 14C present at time t = 0. (See Example 3 in Section 2.5.) Estimate the age of a skull uncovered in

> Refer to Table 4. Find a logarithmic regression model (y = a + b ln x) for the total production. Estimate (to the nearest million) the production in 2024

> Use the formula in Problem 91 (with I0 = 10-16 W/cm2 ) to find the decibel ratings of the following sounds: (A) Whisper: 10-13 W/cm2 (B) Normal conversation: 3.16 * 10-10 W/cm2 (C) Heavy traffic: 10-8 W/cm2 (D) Jet plane with afterburner: 10-1 W/cm2

> Use the models constructed in Problem 89 to find the equilibrium point. Write the equilibrium price to the nearest cent and the equilibrium quantity to the nearest unit. Data from 89:

> How many years (to two decimal places) will it take an investment of $17,000 to grow to $41,000 if it is invested at 2.95% compounded continuously?

> How many years (to two decimal places) will it take $5,000 to grow to $7,500 if it is invested at 8% compounded semiannually? Compounded monthly?

> In its first 10 years the Janus Flexible Income Fund produced an average annual return of 9.58%. Assume that money invested in this fund continues to earn 9.58% compounded annually. How long (to the nearest year) will it take money invested in this fund

> Let p(x) = log x, q(x) and r(x) = x. Use a graphing calculator to draw graphs of all three functions in the same viewing window for 1 ( x ( 16. Discuss what it means for one function to be smaller than another on an interval, and then order the three fu

> Sketch a graph of each equation or pair of equations in a rectangular coordinate system. 5x - 6y = 15

> Explain why 1 is not a suitable logarithmic base.

> Graph using a calculator and point-by-point plotting. Indicate increasing and decreasing intervals. Y = 4 ln (x-3)

> Graph using a calculator and point-by-point plotting. Indicate increasing and decreasing intervals. Y = 2 ln x + 2

> Graph using a calculator and point-by-point plotting. Indicate increasing and decreasing intervals. y = ln |x|

> Graph using a calculator and point-by-point plotting. Indicate increasing and decreasing intervals. y = - ln x

> Solve each equation to four decimal places. 1.024t = 2

> Solve each equation to four decimal places ex = 0.3059

> Solve each equation to four decimal places. 10x = 153

> Find x to four decimal places. (A) log x = 2.0832 (B) log x = -1.1577 (C) ln x = 3.1336 (D) ln x = -4.3281

> Evaluate to five decimal places using a calculator. (A) log 72.604 (B) log 0.033 041 (C) ln 40,257 (D) ln 0.005 926 3

> Find the solution set.

> What are the domain and range of the function defined by y = log (x – 1) - 1?

> Explain how the graph of the equation in Problem 56 can be obtained from the graph of y = log 3 x using a simple trans formation (see Section 2.2). Data From Problem 56:

> Graph the Problems by converting to exponential form first.

> Find x

> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If g is the inverse of a function , then  is the inverse of g.

> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. The inverse of:

> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. The graph of a one-to-one function intersects each vertical line exactly once

> Sketch a graph of each equation or pair of equations in a rectangular coordinate system.

> solve for x 31x + 62 = 5 - 21x + 12

> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. Every polynomial function of odd degree is one-to-one.

> Find x, y, or b without using a calculator.

> Write in simpler form,

> Evaluate the expression without using a calculator.

> Find the solution set.

> Evaluate the expression without using a calculator.

> Rewrite in equivalent logarithmic form.

> Graph each function in over the indicated interval.

> Match each equation with the graph of f, g, h, or k in the figure. (A) y = (Â¼) x (B) y = (0.5) x (C) y = 5x (D) y = 3x

> Use the graph of each line to find the x intercept, y intercept, and slope. Write the slope-intercept form of the equation of the line.

> From the dawn of humanity to 1830, world population grew to one billion people. In 100 more years (by 1930) it grew to two billion, and 3 billion more were added in only 60 years (by 1990). In 2016, the estimated world population was 7.4 billion with a r

> In 2015, the estimated population of Brazil was 204 million with a relative growth rate of 0.77%. (A) Write an equation that models the population growth in Brazil, letting 2015 be year 0. (B) Based on the model, what is the expected population of Brazi

> Refer to Problem 63. Light intensity I relative to depth d (in feet) for one of the clearest bodies of water in the world, the Sargasso Sea, can be approximated by I = I0e - 0.00942d where I0 is the intensity of light at the surface. What percentage of

> Table 5 shows estimates of mobile data traffic, in exabytes 11018 bytes2 per month, for years from 2015 to 2020. (A) Let x represent the number of years since 2015 and find an exponential regression model (y = abx ) for mobile data traffic. (B) Use the

> People assigned to assemble circuit boards for a computer manufacturing company undergo on-the-job training. From past experience, the learning curve for the average employee is given by N = 40 ( 1 - e - 0.12t ) where N is the number of boards assem

> Refer to Problem 57. The following rates for 60-month certificates of deposit were also taken from BanxQuote websites: (A) Oriental Bank & Trust, 1.35% compounded quarterly (B) BMW Bank of North America, 1.30% compounded monthly (C) BankFirst Corporat

> A couple just had a baby. How much should they invest now at 5.5% compounded daily in order to have $40,000 for the child’s education 17 years from now? Compute the answer to the nearest dollar.

> Suppose that $4,000 is invested at 6% compounded weekly. How much money will be in the account in (A) ½ year? (B) 10 years? Compute answers to the nearest cent.

> Find the value of an investment of $24,000 in 7 years if it earns an annual rate of 4.35% compounded continuously.

> Graph each function over the indicated interval.

> Find the Solution Set x - 2 ≥ 2(x – 5)

> Graph each function over the indicated interval.

> Solve each equation for x. (Remember: ex ( 0 and e - x ( 0 for all values of x).

> Solve each equation for x.

> Use the graph of each line to find the x intercept, y intercept, and slope. Write the slope-intercept form of the equation of the line.

> Find real numbers a and b such that a ( b but a4 = b4. Explain why this does not violate the third exponential function property in Theorem 2 on page 98.

> Graph each function over the indicated interval.

> Use the graph of ( shown in the figure to sketch the graph of each of the following. (A) y = ((x) + 2 (B) y = ((x â 3) (C) y = 2 ((x) - 4 (D) y = 4 - ((x + 2)

> describe verbally the transformations that C can be used to obtain the graph of g from the graph of ((see Section 2.2).

> describe verbally the transformations that C can be used to obtain the graph of g from the graph of f (see Section 2.2).

> Graph each function in over the indicated interval.

> Find the Solution Set -314 - x2 = 5 - 1x + 12

> For each polynomial function find the following: (A) Degree of the polynomial (B) All x intercepts (C) The y intercepts ((x) = (x – 5)2 (x + 7)2

> For each polynomial function find the following: (A) Degree of the polynomial (B) All x intercepts (C) The y intercepts ((x) = 5x6 + x4 + 4x8 + 10

> For each polynomial function find the following: (A) Degree of the polynomial (B) All x intercepts (C) The y intercepts ((x) = 30 - 3x

> For each polynomial function find the following: (A) Degree of the polynomial (B) All x intercepts (C) The y intercepts ((x) = x2 - 5x + 6

> Refer to Table 5. (A) Let x represent the number of years since 1960 and find a cubic regression polynomial for the divorce rate. (B) Use the polynomial model from part (A) to estimate the divorce rate (to one decimal place) for 2025.

> n 1917, L. L. Thurstone, a pioneer in quantitative learning theory, proposed the rational function to model the number of successful acts per unit time that a person could accomplish after x practice sessions. Suppose that for a particular person enroll

> Refer to Table 4. (A) Let x represent the number of years since 2000 and findÂ a cubic regression polynomial for the per capita consumption of eggs. (B) Use the polynomial model from part (A) to estimate (to the nearest integer) the per capi

> The financial department of a hospital, using statistical methods, arrived at the cost equation C(x) = 20x3 - 360x2 + 2,300x - 1,000 1 ( x ( 12 where C(x) is the cost in thousands of dollars for handling x thousand cases per month. The average cost per

> Financial analysts in a company that manufactures DVD players arrived at the following daily cost equation for manufacturing x DVD players per day: C(x) = x2 + 2x + 2,000 The average cost per unit at a production level of x players per day is C(x) = C(x

Question: Evaluate the expression without using a