Question: Housing Market The following table shows the
Housing Market The following table shows the five cities where the average U.S. house prices have increased the most from 2012 to 2013. The table also shows the percent change from 2006 to 2013 for those cities.
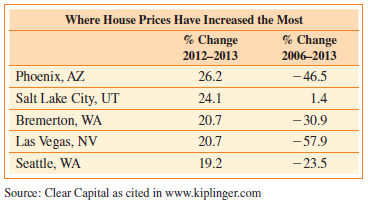
(a) If a house in Las Vegas, NV, cost $200,000 in 2012, how much did a similar house cost in 2013?
(b) If a house in Salt Lake City, UT, cost $220,000 in 2006, how much did a similar house cost in 2013?
(c) From 2006 to 2013, how much more did a $200,000 house in Bremerton, WA, decrease in price than a $200,000 house in Seattle, WA?
> E = {x|x [ N and 14 ≤ x ‹ 85}
> In Exercises 33 and 34, use the method of duplation and mediation to perform the multiplication. See Section 4.1 for Egyptian and Roman numerals. Write the answer in the numeration system in which the exercise is given. (XXVI) . (LXVII)
> The set of states in the United States that have a common border with the state of Washington
> In Exercises 33 and 34, use the method of duplation and mediation to perform the multiplication. See Section 4.1 for Egyptian and Roman numerals. Write the answer in the numeration system in which the exercise is given.
> Home Theater System Jackson wants to purchase a home theater system that sells for $2500. Either he can pay the total amount at the time of purchase or he can agree to pay $250 down and $130 a month for 18 months. How much money can he save by paying the
> The set of football players over the age of 70 who are still playing in the National Football League
> In Exercises 31 and 32, we solve a multiplication problem using Napier’s rods. (a) Determine the numbers being multiplied. Each empty box contains a single digit. (b) Determine the product.
> The set of states in the United States that have a common border with Alaska
> In Exercises 31 and 32, we solve a multiplication problem using Napier’s rods. (a) Determine the numbers being multiplied. Each empty box contains a single digit. (b) Determine the product.
> B = {x|x€N and x is even6
> In Exercises 29 and 30, we show lattice multiplications. (a) Determine the numbers being multiplied. (b) Determine the product.
> C = {x|x + 6 = 10}
> In Exercises 29 and 30, we show lattice multiplications. (a) Determine the numbers being multiplied. (b) Determine the product.
> The set of natural numbers between 10 and 178
> In Exercises 21–28, multiply using Napier’s rods. 7 ( 3456
> Answer true or false. If false, give the reason. {elm, oak, pine} ( {oak, pine, elm, maple}
> In Exercises 1–15, draw a Venn diagram to obtain the answers. Dunkin Donuts Purchases Dunkin Donuts collected the following information regarding purchases from 100 of its customers. 65 purchased coffee. 41 purchased donuts. 22 purchased both coffee an
> The set of months whose names begin with the letter J
> In Exercises 21–28, multiply using Napier’s rods. 9 ( 6742
> The set of states in the United States whose names begin with the letter H
> In Exercises 21–28, multiply using Napier’s rods. 75 ( 125
> The set of apple trees in Gro-More Farms Orchards
> In Exercises 21–28, multiply using Napier’s rods. 5 ( 125
> The set of odd numbers greater than 15
> In Exercises 21–28, multiply using Napier’s rods. 6 ( 171
> The set of fractions between 1 and 2
> In Exercises 21–28, multiply using Napier’s rods. 5 ( 79
> Subway Cost Chandler buys a monthly MetroCard, which entitles him to unlimited subway travel in New York City, for $112 per month. Without the Metrocard, each subway ride costs $2.50. How many rides per month would Chandler have to take so that the cost
> The set of odd numbers greater than 25
> In Exercises 21–28, multiply using Napier’s rods. 4 ( 58
> The set of multiples of 3 between 0 and 40
> In Exercises 21–28, multiply using Napier’s rods. 3 ( 43
> {1, 2, 3, 4, … }
> In Exercises 13–20, use lattice multiplication to determine the product. 634 ( 832
> The set of the most interesting teachers at your school
> In Exercises 13–20, use lattice multiplication to determine the product. 314 ( 652
> The set of astronauts who walked on the moon
> In Exercises 13–20, use lattice multiplication to determine the product. 47 ( 259
> Answer true or false. If false, give the reason. {cheesecake, pie} ( {pie, cookie, cheesecake, brownie}
> The set of Academy Awards winners in 2014
> In Exercises 13–20, use lattice multiplication to determine the product. 75 ( 12
> Determine whether the number used is a cardinal number or an ordinal number. Emily paid $35 for her new blouse.
> In Exercises 13–20, use lattice multiplication to determine the product. 9 ( 509
> Determine whether the number used is a cardinal number or an ordinal number. Lincoln was the sixteenth president of the United States.
> In Exercises 13–20, use lattice multiplication to determine the product. 8 ( 567
> Determine whether the number used is a cardinal number or an ordinal number. Study the chart on page 25 in the book.
> In Exercises 13–20, use lattice multiplication to determine the product. 5 ( 417
> Determine whether the number used is a cardinal number or an ordinal number J. K. Rowling has written 7 Harry Potter books.
> In Exercises 13–20, use lattice multiplication to determine the product. 4 ( 327
> Consider sets A and B below A = { x | 2 < x ≤ 5 and x ( N } and B = { x | 2 < x ≤ 5 } (a) Write a description of set A and set B. (b) Explain the difference between set A and set B. (c) Write set A in roster form. (d) Can set B be written in roster
> In Exercises 5–12, multiply using duplation and mediation. 49 ( 124
> Set-builder notation is often more versatile and efficient than listing a set in roster form. This versatility is illustrated with the following two sets. A = {x | x ( N and x > 2 } B = {x | x > 2 } a) Write a description of set A and set B. b) Explain
> In Exercises 5–12, multiply using duplation and mediation. 93 ( 93
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A is the set of states. B is the set of state capitals.
> In Exercises 5–12, multiply using duplation and mediation. 96 ( 53
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A is the set of letters in the word bank. B is the set of letters in the word post.
> In Exercises 5–12, multiply using duplation and mediation. 35 ( 236
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A is the set of Siamese cats. B is the set of cats.
> In Exercises 5–12, multiply using duplation and mediation. 138 ( 41
> Answer true or false. If false, give the reason. {book, magazine} ( {book, newspaper, journal}
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A = { grapes, apples, oranges }, B = { grapes, peaches, apples, oranges }
> Convert the given numeral to a numeral in base 10. 234
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A = { purple, green, yellow } , B = { q, r, s }
> Convert the given numeral to a numeral in base 10. 213
> Determine whether the pairs of sets are equal, equivalent, both, or neither. A = { lion, tiger, monkey } , B = { tiger, monkey, lion }
> Convert the given numeral to a numeral in base 10. 123
> Use the sets A = {2, 4, 6, 8}, B ={1, 3, 7, 9, 13, 21 }, C = { }, and D = {#, &, %, (,*} . Determine n(D) .
> Convert the given numeral to a numeral inbase 10. 57316
> Use the sets A = {2, 4, 6, 8}, B ={1, 3, 7, 9, 13, 21 }, C = { }, and D = {#, &, %, ,*} . Determine n(C) .
> Convert the given numeral to a numeral inbase 10. 100512
> Bachelor’s Degrees The circle graph below shows the percent of bachelor’s degrees awarded in 2012 in Business, Social Sciences and History, Health Professions, Psychology, Education, and other fields. In 2012, the numb
> Use the sets A = {2, 4, 6, 8}, B ={1, 3, 7, 9, 13, 21 }, C = { }, and D = {#, &, %, ,*} . Determine n(B) .
> Convert the given numeral to a numeral in base 10. 30912
> Use the sets A = {2, 4, 6, 8}, B ={1, 3, 7, 9, 13, 21 }, C = { }, and D = {#, &, %, ,*} . Determine n(A).
> Convert the given numeral to a numeral in base 10. 7658
> 2 ( {x | x is an odd natural number}
> Convert the given numeral to a numeral in base 10. 2418
> 9 ( {1, 3, 5, 7, … }
> Suppose a base 4 place-value system has its digits represented by colors as follows: (a) Determine the value of /in base 10 (b) Write 177 in the base 4 system using only the four colors given in the exercise.
> Amazon ( {rivers in the United States}
> The Price Is Right Refer to the Recreational Mathematics on page 181. Determine the correct order in which to place the digits 0, 1, 2, 3, and 8 to match the price of the 2014 Chevrolet Impala LTZ Sedan
> Answer true or false. If false, give the reason. {potato, carrot} ( {carrot, squash, cucumber, celery}
> 3 ( {x | x (N and x is odd}
> Convert the given numeral to a numeral in base 10. 324
> Mickey Mouse Є {characters created by Walt Disney }
> Computer Code The ASCII code used by most computers uses the last seven positions of an eight-bit byte to represent all the characters on a standard keyboard. How many different orderings of 0’s and 1’s (or how many different characters) can be made by u
> Boxes of Fruit There are three boxes on a table, each with a label. Thomas knows that one box contains grapes, one box contains cherries, and the third box contains both grapes and cherries. He also knows that the three labels used—grapes, cherries, and
> (a) Use the numerals 0, 1, and 2 to write the first 20 numbers in the base 3 numeration system. (b) What is the next numeral after 2223?
> Spending Money Samantha went into a store and spent half her money and then spent $20 more. Samantha then went into a second store and spent half her remaining money and then spent $20 more. After spending money in the second store, Samantha had no money
> Find d if ddd5 = 124
> Finding the Area Rectangle ABCD is made up entirely of squares. The black square has a side of 1 unit. Find the area of rectangle ABCD.
> Find b if 111b = 43
> How Americans Spend Their Money The circle graph below shows the percent of Americans’ net monthly income spent on housing, transportation, food, insurance/ retirement, healthcare, and entertainment/miscellaneous. Sammy has a net monthl
> Counting Triangles How many triangles are in the figure? Answer: 16+16+4+4+4=44
> (a) What is 112 equal to in base 10? (b) What is 118 equal to in base 10? (c) What is 1116 equal to in base 10? (d) What is 1132 equal to in base 10? (e) In general, for any base b, what is 11b equal to in base10?
> Musicians Jaquan, Cindy, and Mark are musicians. One plays the guitar, one plays the saxophone, and one plays the drums. They live in three adjacent houses on Lake View Drive. From the following information, determine who plays the drums. (Hint: A table
> (a) What is 102 equal to in base 10? (b) What is 108 equal to in base 10? (c) What is 1016 equal to in base 10? (d) What is 1032 equal to in base 10? (e) In general, for any base b, what is l0b equal to in base 10?
> Insurance Policies Ray owns two cars (a Ford Mustang and a Ford Focus), a house, and a rental apartment. He has auto insurance for both cars, a homeowner’s policy, and a policy for the rental property. The costs of the policies are Mustang: $1648 per yea
> (a) What is 04 equal to in base 10? (b) What is 14 equal to in base 10? (c) What is 24 equal to in base 10? (d) What is 34 equal to in base 10? (e) In general, if n is a digit less than the base b, and the base b is less than or equal to 10, then d
> A Grid Place five 1’s, five 2’s, five 3’s, five 4’s, and five 5’s in a 5 × 5 grid so that each digit—that is, 1, 2, 3, 4, 5—appears exactly once in each row and exactly once in each column.

