Question: In an 8-second test run, a
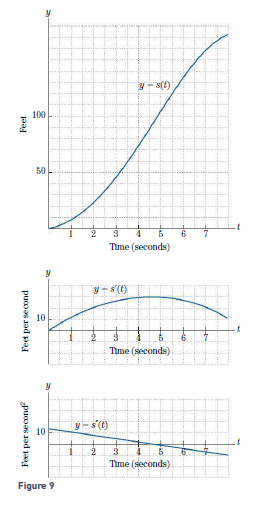
In an 8-second test run, a vehicle accelerates for several seconds and then decelerates. The function s(t) gives the number of feet traveled after t seconds and is graphed in Fig. 9.
(a) How far has the vehicle traveled after 3.5 seconds?
(b) What is the velocity after 2 seconds?
(c) What is the acceleration after 1 second?
(d) When will the vehicle have traveled 120 feet?
(e) When, during the second part of the test run, will the vehicle be traveling at the rate of 20 feet per second?
(f ) What is the greatest velocity? At what time is this greatest velocity reached? How far has the vehicle traveled at this time?
Figure 9:
Transcribed Image Text:
Feet Feet per second Feet per second- Y 100 50 10 10 Figure 9 y-s(t) Time (seconds) y-s(t) y-s(t) HEIN 6 7 Time (seconds) 3 Time (seconds)
> Differentiate. y = (x3 + x2 + 1)5
> Differentiate. y = 1 / 5x - 1
> Differentiate. y = ¾ x4/3 + 4/3 x3/4
> Differentiate. y = (3x2 - 1)8
> Differentiate. y = x4 – 4/x
> Differentiate. y = 3/x
> Differentiate. y = (x - 1)3 + (x + 2)4
> Differentiate. y = x7 + 3x5 + 1
> Differentiate. y = 6√x
> Differentiate. y = 5x8
> Differentiate. y = x7 + x3
> Find the equation and sketch the graph of the following lines. The x-axis.
> Find the equation and sketch the graph of the following lines. The y-axis.
> Find the equation and sketch the graph of the following lines. Vertical and 4 units to the right of the y-axis.
> Find the equation and sketch the graph of the following lines. Horizontal with height 3 units above the x–axis.
> Find the equation and sketch the graph of the following lines. Perpendicular to 3x + 4y = 5, passing through (6, 7).
> Find the equation and sketch the graph of the following lines. Perpendicular to y = 3x + 4, passing through (1, 2).
> Differentiate. y = 2x + (x + 2)3
> Find the equation and sketch the graph of the following lines. Through (2, 1) and (5, 1).
> Find the equation and sketch the graph of the following lines. Through (-1, 4) and (3, 7).
> What can you say about the slopes of parallel lines? Perpendicular lines?
> Describe how to find an equation for a line when you know the coordinates of two points on the graph of a line.
> What is the point–slope form of the equation of a line?
> Define the slope of a nonvertical line and give a physical description.
> How do you determine the proper units for a rate of change? Give an example.
> Describe marginal cost in your own words.
> What expression involving a derivative gives an approximation to f (a + h) - f (a)?
> Explain the relationship between derivatives and velocity and acceleration.
> Differentiate. y = 2√(x + 1)
> How is an (instantaneous) rate of change related to average rates of change?
> What is meant by the average rate of change of a function over an interval?
> Give two different notations for the first derivative of f (x) at x = 2. Do the same for the second derivative.
> State the general power rule and give an example.
> In your own words, explain the meaning of “ f (x) is differentiable at x = 2.” Give an example of a function f (x) that is not differentiable at x = 2.
> In your own words, explain the meaning of “ f (x) is continuous at x = 2.” Give an example of a function f (x) that is not continuous at x = 2.
> In your own words, explain the meaning of lim x→∞ f (x) = 3. Give an example of such a function f (x). Do the same for lim x→∞ f (x) = 3.
> Give the limit definition of f ‘(2), that is, the slope of f (x) at the point (2, f (2)).
> In your own words, explain the meaning of lim x→2 f (x) = 3. Give an example of a function with this property.
> Explain how to calculate f ‘(2) as the limit of slopes of secant lines through the point (2, f (2)).
> Differentiate. y = 3 3√(2x2 + 1)
> State the power rule, the constant-multiple rule, and the sum rule, and give an example of each.
> Explain why the derivative of a constant function is 0.
> What does f ‘(2) represent?
> Give a physical description of what is meant by the slope of f (x) at the point (2, f (2)).
> Suppose that f (x) = 4x2. (a) What is the average rate of change of f (x) over each of the intervals 1 to 2, 1 to 1.5, and 1 to 1.1? (b) What is the (instantaneous) rate of change of f (x) when x = 1?
> If f (x) = 3x2 + 2, calculate the average rate of change of f (x) over the following intervals (a) 0 ≤ x ≤ .5, (b) 0 ≤ x ≤ .1, (c) 0 ≤ x ≤ .0.01.
> If f (x) = x2 + 3x, calculate the average rate of change of f (x) over the following intervals (a) 1 …≤ x ≤ 2, (b) 1 ≤ x ≤ 1.5, (c) 1 ≤ x ≤ 1.1.
> A ball thrown straight up into the air has height s(t) = 102t - 16t2 feet after t seconds. (a) Display the graphs of s(t) and s’(t) in the window [0, 7] by [-100, 200]. Use these graphs to answer the remaining questions. (b) How high is the ball after 2
> In a psychology experiment, people improved their ability to recognize common verbal and semantic information with practice. Their judgment time after t days of practice was f (t) = .36 + .77(t - .5)-0.36 seconds. (a) Display the graphs of f (t) and f ‘(
> Differentiate. y = 4(x2 - 6)-3
> National health expenditures (in billions of dollars) from 1980 to 1998 are given by the function f (t) in Fig. 8. (a) How much money was spent in 1987? (b) Approximately how fast were expenditures rising in 1987? (c) When did expenditures reach $1 trill
> Estimate how much the function f (x) = 1 / 1 + x2 will change if x decreases from 1 to .9.
> Consider the cost function C(x) = 6x2 + 14x + 18 (thousand dollars). (a) What is the marginal cost at production level x = 5? (b) Estimate the cost of raising the production level from x = 5 to x = 5.25. (c) Let R(x) = -x2 + 37x + 38 denote the revenue i
> Let f (x) be the value in dollars of one share of a company x days since the company went public. (a) Interpret the statements f (100) = 16 and f ‘(100) = .25. (b) Estimate the value of one share on the 101st day since the company went public.
> Let P(x) be the profit (in dollars) from manufacturing and selling x cars. Interpret P(100) = 90,000 and P’(100) = 1200. Estimate the profit from manufacturing and selling 99 cars.
> Let C(x) be the cost (in dollars) of manufacturing x items. Interpret the statements C(2000) = 50,000 and C’(2000) = 10. Estimate the cost of manufacturing 1998 items.
> Let f (x) be the number (in thousands) of computers sold when the price is x hundred dollars per computer. Interpret the statements f (12) = 60 and f ‘(12) = -2. Then, estimate the number of computers sold if the price is set at $1250 per computer.
> Let f (x) be the number of toys sold when x dollars are spent on advertising. Interpret the statements f (100,000) = 3,000,000 and f ‘(100,000) = 30.
> Sales Let f (p) be the number of cars sold when the price is p dollars per car. Interpret the statements f (10,000) = 200,000 and f ‘(10,000) = -3.
> Suppose that 5 mg of a drug is injected into the bloodstream. Let f (t) be the amount present in the bloodstream after t hours. Interpret f (3) = 2 and f ‘(3) = -.5. Estimate the number of milligrams of the drug in the bloodstream after 312 hours.
> Differentiate. y = 4/x2
> Let f (t) be the temperature of a cup of coffee t minutes after it has been poured. Interpret f (4) = 120 and f ‘(4) = -5. Estimate the temperature of the coffee after 4 minutes and 6 seconds, that is, after 4.1 minutes.
> If f (25) = 10 and f ‘(25) = -2, estimate each of the following. (a) f (27) (b) f (26) (c) f (25.25) (d) f (24) (e) f (23.5)
> If f (100) = 5000 and f ‘(100) = 10, estimate each of the following. (a) f (101) (b) f (100.5) (c) f (99) (d) f (98) (e) f (99.75)
> A car is traveling from New York to Boston and is partway between the two cities. Let s(t) be the distance from New York during the next minute. Match each behavior with the corresponding graph of s(t) in Fig. 7. (a) The car travels at a positive steady
> A particle is moving in a straight line in such a way that its position at time t (in seconds) is s(t) = t2 + 3t + 2 feet to the right of a reference point, for t ≥ 0. (a) What is the velocity of the object when the time is 6 seconds? (b) Is the object m
> Table 2 gives a car’s trip odometer reading (in miles) at 1 hour into a trip and at several nearby times. What is the average speed during the time interval from 1 to 1.05 hours? Estimate the speed at time 1 hour into the trip. Table 2
> Let s(t) be the height (in feet) after t seconds of a ball thrown straight up into the air. Match each question with the proper solution. Questions A. What is the velocity of the ball after 3 seconds? B. When is the velocity 3 feet per second? C. What is
> A helicopter is rising straight up in the air. Its distance from the ground t seconds after takeoff is s(t) feet, where s(t) = t2 + t. (a) How long will it take for the helicopter to rise 20 feet? (b) Find the velocity and the acceleration of the helicop
> A toy rocket fired straight up into the air has height s(t) = 160t - 16t2 feet after t seconds. (a) What is the rocket’s initial velocity (when t = 0)? (b) What is the velocity after 2 seconds? (c) What is the acceleration when t = 3? (d) At what time wi
> Refer to Fig. 6, where s(t) represents the position of a car moving in a straight line. (a) Was the car going faster at A or at B? (b) Is the velocity increasing or decreasing at B? What does this say about the acceleration at B? (c) What happened to the
> Differentiate. y = (x2 + x)-2
> Maximum height A toy rocket is fired straight up into the air. Let s(t) = -6t2 + 72t denote its position in feet after t seconds. (a) Find the velocity after t seconds. (b) Find the acceleration after t seconds. (c) When does the rocket reach its maximum
> Liquid is pouring into a large vat. After t hours, there are 5t + √t gallons in the vat. At what rate is the liquid flowing into the vat (in gallons per hour) when t = 4?
> An analysis of the daily output of a factory assembly line shows that about 60t + t2 – 1/12 t3 units are produced after t hours of work, 0 ≤ t ≤ 8. What is the rate of production (in units per hour) when t = 2?
> After an advertising campaign, the sales of a product often increase and then decrease. Suppose that t days after the end of the advertising, the daily sales are f (t) = -3t2 + 32t + 100 units. What is the average rate of growth in sales during the fourt
> An object moving in a straight line travels s(t) kilometers in t hours, where s(t) = 2t2 + 4t. (a) What is the object’s velocity when t = 6? (b) How far has the object traveled in 6 hours? (c) When is the object traveling at the rate of 6 kilometers per
> Suppose that f (t) = 3t + 2 – 12/t. (a) What is the average rate of change of f (t) over the interval 2 to 3? (b) What is the (instantaneous) rate of change of f (t) when t = 2?
> Suppose that f (t) = t2 + 3t - 7. (a) What is the average rate of change of f (t) over the interval 5 to 6? (b) What is the (instantaneous) rate of change of f (t) when t = 5?
> Suppose that f (x) = -6/x. (a) What is the average rate of change of f (x) over each of the intervals 1 to 2, 1 to 1.5, and 1 to 1.2? (b) What is the (instantaneous) rate of change of f (x) when x = 1?
> Find the first derivatives. g(y) = y2 - 2y + 4
> Find the first derivatives. y(t) = 4t2 + 11 √t + 1
> Differentiate. y = (x3 + x2 + 1)7
> Find the first derivatives. f (P) = P3 + 3P2 - 7P + 2
> Find the first derivatives. f (t) = (t2 + 1)5
> Consider the cost function of Example 6. (a) Graph C(x) in the window [0, 60] by [-300, 1260]. (b) For what level of production will the cost be $535? (c) For what level of production will the marginal cost be $14? Cost Function of Example 6: C(x) = .0
> For the given function, simultaneously graph the functions f (x), f (x), and f (x) with the specified window setting. f (x) = x/1 + x2 , [-4, 4] by [-2, 2].
> Compute the third derivatives of the following functions: (a) f (t) = t10 (b) f (z) = 1/z + 5
> The third derivative of a function f (x) is the derivative of the second derivative f ‘(x) and is denoted by f ‘(x). Compute f ‘(x) for the following functions: (a) f (x) = x5 - x4 + 3x (b) f (x) = 4x5/2
> A toy company introduces a new video game on the market. Let S(x) denote the number of videos sold on the day, x, since the item was introduced. Let n be a positive integer. Interpret S(n), S’(n), and S(n) + S’(n).
> (a) Let A(x) denote the number (in hundreds) of computers sold when x thousand dollars is spent on advertising. Represent the following statement by equations involving A or A’: When $8000 is spent on advertising, the number of computers sold is 1200 and
> The financial analysts at the store in Example 5 corrected their projections and are now expecting the total sales for the x day of January to be (a) Let S(x) be as in Example 5. Compute T(1), T’(1), S(1), and S’(1
> (a) Compute S(10) and S’(10). (b) Use the data in part (a) to estimate the total sales on January 11. Compare your estimate to the actual value given by S(11).
> Differentiate. y = (x2 - 1)3
> Differentiate. y = 6x3
> What is the difference between a C2B and C2C?
> Explain why a business would use metrics to measure the success of strategic initiatives.
> Define critical success factors (CSFs) and key performance indicators (KPIs), and explain how managers use them to measure the success of MIS projects.
> Bug reports are an important part of software development. All bugs must be logged, fixed, and tested. There are three common types of bugs programmers look for when building a system: ■ Syntax errors: A mistake in the program’s words or symbols. ■ Runti
> How can global warming be real when there is so much snow and cold weather? That’s what some people wondered after a couple of massive snowstorms buried Washington, DC, and parts of the East Coast. Politicians across the capital made jokes and built iglo

