Question: It seems logical that the number of
It seems logical that the number of days per year that an employee is tardy is at least somewhat related to the employee’s job satisfaction. Suppose 10 employees are asked to record how satisfied they are with their job on a scale of 0 to 10, with 0 denoting completely unsatisfied and 10 denoting completely satisfied. Suppose also that through human resource records, it is determined how many days each of these employees was tardy last year. The scatter plot at the top of the next column graphs the job satisfaction scores of each employee against the number of days he or she was tardy. What information can you glean from the scatter plot? Does there appear to be any relationship between job satisfaction and tardiness? If so, how might they appear to be related?
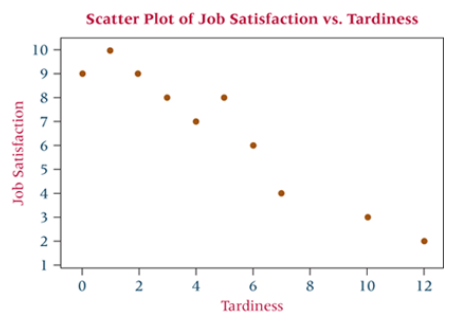
Transcribed Image Text:
Job Satisfaction 10 9 8 3 2 Scatter Plot of Job Satisfaction vs. Tardiness 2 Tardiness 8 10 12
> Classify each of the following as nominal, ordinal, interval, or ratio data. a. The time required to produce each tire on an assembly line b. The number of quarts of milk a family drinks in a month c. The ranking of four machines in your plant after they
> Southwest Airlines One night over dinner early in 1967 in San Antonio, Rollin King presented his idea for a new airline to Herb Kelleher by drawing a triangle on a napkin and labeling the three corners as Dallas, Houston, and San Antonio. King’s idea was
> The owner of a fast-food restaurant ascertains the ages of a sample of customers. From these data, the owner constructs the frequency distribution shown. For each class interval of the frequency distribution, determine the class midpoint, the relative fr
> A packaging process is supposed to fill small boxes of raisins with approximately 50 raisins so that each box will weigh the same. However, the number of raisins in each box will vary. Suppose 100 boxes of raisins are randomly sampled, the raisins counte
> The following data represent the afternoon high temperatures for 50 construction days during a year in St. Louis. a) Construct a frequency distribution for the data using 5 class intervals. b) Construct a frequency distribution for the data using 10 cla
> The following data represent the number of passengers per flight in a sample of 50 flights from Wichita, Kansas, to Kansas City, Missouri. a) Construct a dot plot for these data. b) Construct a stem-and-leaf plot for these data. What does the stem-and-
> A real estate group is investigating the price of condominiums of a given size (sq ft). The following sales prices ($1,000) were obtained in one region of a city. Construct a stem-and-leaf plot for the following data using two digits for the stem. Commen
> Construct an ogive for the following data. Class Interval _______________ Frequency 3–under 6 ………………….………….. 2 6–under 9 ………………….………….. 5 9–under 12 ………………………….. 10 12–under 15 ………………………….. 11 15–under 18 ………………………….. 17 18–under 21 …………………………... 5
> Suppose you are the owner of a medium-sized restaurant in a small city. What are some variables associated with different aspects of the business that might be helpful to you in making business decisions about the restaurant? Name four of these variables
> A call center is trying to better understand staffing requirements. It records the number of calls received during the evening shift for 78 evenings and obtains the information given below. Construct a histogram of the data and comment on the key charact
> Assembly times for components must be understood in order to “level” the stages of a production process. Construct both a histogram and a frequency polygon for the following assembly time data and comment on the key characteristics of the distribution.
> List three specific uses of cumulative frequencies in business?
> The human resources manager for a large company commissions a study in which the employment records of 500 company employees are examined for absenteeism during the past year. The business analyst conducting the study organizes the data into a frequency
> Shown here is a scatter plot of the NASDAQ 100 Index versus the Dow Jones Industrial Average on Friday closings over a period of one year (January 12, 2018 to January 4, 2019). What does the graph tell you about the relationship of the NASDAQ 100 Index t
> Suppose 100 CPA firms are surveyed to determine how many audits they perform over a certain time. The data are summarized using the Minitab stem-and-leaf plot shown here. What can you learn from this plot about the number of audits being performed by the
> Shown here is a Minitab-produced pie chart representing physician specialties. What does the chart tell you about the various specialties? Emergency Medicine 9.8% Anesthesiology 10.2% OB-GYN 10.2% Physician Specialties Pediatrics. 14.2% Internal Medi
> Study the following dot plot and comment on the general shape of the distribution. Discuss any gaps or heavy concentrations in the data. င်းငွေ ၁ ပဗ္ဗ d 9 18 ဝိဝိ 27 ထဝ 88 d 36 45 54
> Suppose 150 shoppers at an upscale mall are interviewed and one of the questions asked is household income. Study the Minitab histogram of the following data and discuss what can be learned about the shoppers. Frequency 30 20 10 50,000 100,000 Househ
> A research organization selected 50 U.S. towns from the 2010 census with populations between 4000 and 6000 as a sample to represent small towns for survey purposes. The populations of these towns follow. Construct a stem-and-leaf plot for the data, lett
> There are many types of information that might help the manager of a large department store run the business more efficiently and better understand how to improve sales. Think about this in such areas as sales, customers, human resources, inventory, supp
> A manufacturing company produces plastic bottles for the dairy industry. Some of the bottles are rejected because of poor quality. Causes of poor-quality bottles include faulty plastic, incorrect labeling, discoloration, incorrect thickness, broken handl
> Shown here is a list of the industries with the largest total toxic releases in a recent year according to the EPA. Construct both a bar chart and a pie chart to depict this information and comment on the advantages of each type of chart in depicting the
> The following figures for U.S. imports of agricultural products and manufactured goods were taken from selected years over a 30-year period (in $ billions). The source of the data is the U.S. International Trade Administration. Construct a scatter plot f
> Shown here are production unit data for a company for the first four months of the year over eight years. Construct a chart or graph that best depicts these four months of data over the eight years together in one graph. What do you visualize is happenin
> Shown here are the top seven companies from the construction, farm machinery industry in the United States and their respective revenue ($ billions). Company ____________ Revenue Caterpillar ………………………… 38.5 Deere ………………………………. 26.6 Paccar ……………………………… 17
> A consumer group surveyed food prices at 87 stores on the East Coast. Among the food prices being measured was that of sugar. From the data collected, the group constructed the frequency distribution of the prices of 4 pounds of Domino’s sugar in the sto
> Good, relatively inexpensive prenatal care often can prevent a lifetime of expense owing to complications resulting from a baby’s low birth weight. A survey of a random sample of 57 new mothers asked them to estimate how much they spent on prenatal care.
> Shipping a 40-foot container by boat from Shanghai to Chicago via the port of Los Angeles takes, on average, 16 days. However, due to several possible mitigating circumstances, shipping times can vary. Suppose a transportation analyst randomly selects 20
> In a medium-sized southern city, 86 houses are for sale, each having about 2000 square feet of floor space. The asking prices vary. The frequency distribution shown contains the price categories for the 86 houses. Construct a histogram, a frequency polyg
> The following data are shaped roughly like a normal distribution (discussed in Chapter 6). Construct a frequency distribution starting with 10 as the lowest class beginning point and use a class width of 10. Construct a histogram and a frequency polygon
> Suppose you are an operations manager for a plant that manufactures batteries. Give an example of how you could use descriptive statistics to make better managerial decisions. Give an example of how you could use inferential statistics to make better man
> Suppose 1000 commuters in New York City submit their typical daily commute time to a transportation research company which then organizes the data into the histogram shown below. Study the histogram and comment on information gleaned from the graph. Desc
> A northwestern distribution company surveyed 53 of its midlevel managers. The survey obtained the ages of these managers, which later were organized into the frequency distribution shown. Determine the class midpoint, relative frequency, and cumulative f
> The Whitcomb Company manufactures a metal ring for industrial engines that usually weighs about 50 ounces. A random sample of 50 of these metal rings produced the following weights (in ounces). a) Construct a dot plot for these data and comment on any o
> Visualize the following time-series data. Year __________ Number of Employees 1 …………………………………….. 56 2 …………………………………….. 53 3 …………………………………….. 47 4 …………………………………….. 41 5 …………………………………….. 43 6 …………………………………….. 46 7 …………………………………….. 52 8 …………………………………….. 63
> Construct a scatter plot for the following two numerical variables? x _____________ y 12 ……………………… 5 17 ……………………… 3 9 ………….………….. 10 6 ………….………….. 15 10 …………………….. 8 14 ………………….….. 9 8 ….……….………….. 8
> An examination of rejects shows at least seven problems. A frequency tally of the problems follows. Construct a Pareto chart for these data. Problem ____________ Frequency 1 ………………………………………. 673 2 ……………………………………….. 29 3 ……………………………………… 108 4 ………………………………
> Construct a bar graph from the following data. Category ___________ Frequency A ……………………………………. 7 B …………………………………… 12 C …………………………………… 14 D ……………………………………. 5 E …………………………………… 19
> Construct a pie chart from the following data. Label _____________ Value A …………………………………. 55 B ………………………………… 121 C …………………………………. 83 D …………………………………. 46
> Construct a stem-and-leaf plot for the following data. Let the leaf contain one digit? 312 324 289 335 298 314 309 294 326 317 290 311 317 301 316 306 286 308 284 324
> Construct a dot plot from the following data? 16 15 17 15 15 15 14 9 16 15 13 10 8 18 20 17 17 17 18 23 7 15 20 10 14
> Give an example of descriptive statistics in the recorded music industry. Give an example of how inferential statistics could be used in the recorded music industry. Compare the two examples. What makes them different?
> Construct a histogram, a frequency polygon, and an ogive for the following frequency distribution? Class Interval __________ Frequency 50–under 60 ……………………….. 13 60–under 70 ……………………….. 27 70–under 80 ……………………….. 43 80–under 90 ……………………….. 31 90–under 10
> For each class interval of the frequency distribution given, determine the class midpoint, the relative frequency, and the cumulative frequency. Class Interval ___________ Frequency 20–under 25 …………………………… 17 25–under 30 …………………………… 20 30–under 35 ………………
> For the following data, construct a frequency distribution with six classes? 57 23 35 18 21 26 51 47 29 21 46 43 29 23 39 50 41 19 36 28 31 42 52 29 18 28 46 33 28 20
> Shown here are quarterly data of the exports (in $ millions) of eight U.S. cities over a two-year period supplied by the U.S. Census Bureau. Using this data: a. Construct a time-series graph for one city of these data. Looking at your graph, what are som
> Shown here are the sales data (in $ millions) for U.S. furniture and home furnishing stores from 2012 through 2016 supplied by the U.S. Census Bureau. Using this data: a. Construct a time-series graph of one year of these data. Looking at your graph, w
> A customer relations expert for a retail tire company is interested in determining if there is any relationship between a customer’s level of education and his or her rating of the quality of the tire company’s service
> The human resources manager of a large chemical plant was interested in determining what factors might be related to the number of non-vacation days that workers were absent during the past year. One of the factors that the manager considered was the dis
> Are the advertising dollars spent by a company related to total sales revenue? The following data represent the advertising dollars and the sales revenues for various companies in a given industry during a recent year. Construct a scatter plot of the dat
> The U.S. National Oceanic and Atmospheric Administration, National Marine Fisheries Service, publishes data on the quantity and value of domestic fishing in the United States. The quantity (in millions of pounds) of fish caught and used for human food an
> State examples of data that can be gathered for decision-making purposes from each of the following industries: manufacturing, insurance, travel, retailing, communications, computing, agriculture, banking, and healthcare. An example in the travel industr
> An airline company uses a central telephone bank and a semiautomated telephone process to take reservations. It has been receiving an unusually high number of customer complaints about its reservation system. The company conducted a survey of customers,
> How do various currencies around the world stack up to the U.S. dollar? Shown below is a bar chart of the value of the currency of various countries in U.S. dollars as of November 2018. The currencies represented here are the Malaysia ringgit, United Ara
> The following list shows the top six pharmaceutical companies in the United States by revenue ($ billions) for a recent year as published by Forbes. Use this information to construct a pie chart and a bar graph to represent these six companies and their
> According to Bureau of Transportation statistics, the largest five U.S. airlines in scheduled system-wide (domestic and international) enplanements in 2017 (passenger numbers in millions) were: Southwest with 153.8, Delta with 120.7, American with 116.5,
> Shown here is a list published by Electronics Weekly.com of the top five semiconductor companies in the United States by revenue ($ billions). Firm ____________ Revenue ($ billions) Intel Corporation ………………….. 56.31 Qualcomm ……………………………. 15.44 Broadcom …
> A hundred or so boats go fishing every year for three or four weeks off of the Bering Strait for Alaskan king crabs. To catch these king crabs, large pots are baited and left on the sea bottom, often several hundred feet deep. Because of the investment i
> A full-service car wash has an automated exterior conveyor car wash system that does the initial cleaning in a few minutes. However, once the car is through the system, car wash workers hand clean the inside and the outside of the car for approximately 1
> Study the Minitab-produced dot plot of the number of farms per state in the United States shown below. Comment on any observations that you make from the graph. What does this graph tell you about the number of farms per state? The average number of farm
> The Airports Council International—North America (ACI) publishes data on the busiest airports in the world. Shown below is a Minitab-produced histogram constructed from ACI data on the number of passengers that enplaned and deplaned in
> Kraft Foods successfully introduced DiGiorno Pizza into the marketplace in 1996, with first-year sales of $120 million, followed by $200 million in sales in 1997. It was neither luck nor coincidence that DiGiorno Pizza was an instant success. Kraft condu
> Give a specific example of data that might be gathered from each of the following business disciplines: accounting, finance, human resources, marketing, information systems, production, and management. An example in the marketing area might be “number of
> Refer to Example 3. If labor costs $100 per unit and capital costs $200 per unit, express as a function of two variables, C(x, y), the cost of utilizing x units of labor and y units of capital.
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Consider the Cobb–Douglas production function f (x, y) = 20x1/3y2/3. Compute f (8, 1), f (1, 27), and f (8, 27). Show that, for any positive constant k, f (8k, 27k) = k f (8, 27).
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. Then, use the second-derivative test to determine, if possible, the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = 1/x + 1/
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = y ex - 3
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = x4 - 4xy
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = 2x2 - x4
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = 6xy2 - 2
> Both first partial derivatives of the function f (x, y) are zero at the given points. Use the second-derivative test to determine the nature of f (x, y) at each of these points. If the second-derivative test is inconclusive, so state. f (x, y) = 3x2 - 6x
> The function f (x, y) = 1/2 x2 + 2xy + 3y2 - x + 2y has a minimum at some point (x, y). Find the values of x and y where this minimum occurs.
> Find a formula C (x, y, z) that gives the cost of material for the rectangular enclosure in Fig. 7(b), with dimensions in feet. Assume that the material for the top costs $3 per square foot and the material for the back and two sides costs $5 per square
> The function f (x, y) = 2x + 3y + 9 - x2 - xy - y2 has a maximum at some point (x, y). Find the values of x and y where this maximum occurs.
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = x4 - 2xy - 7x2 + y2 + 3
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = x3 + x2y - y
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = x4 - 8xy + 2y2 - 3
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = 1/3 x3 - 2y3 - 5x + 6y - 5
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = 15/4 x2 + 6xy - 3y2 + 3x + 6y
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = 2x3 + 2x2y - y2 + y
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = -8y3 + 4xy + 4x2 + 9y2
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = -8y3 + 4xy + 9y2 - 2y
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = x2 - y3 + 5x + 12y + 1
> Find a formula C (x, y, z) that gives the cost of materials for the closed rectangular box in Fig. 7(a), with dimensions in feet. Assume that the material for the top and bottom costs $3 per square foot and the material for the sides costs $5 per square
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = x3 + y2 - 3x + 6y
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = 4x2 + 4xy - 3y2 + 4y - 1
> Find all points (x, y) where f (x, y) has a possible relative maximum or minimum. f (x, y) = 3x2 + 8xy - 3y2 - 2x + 4y - 1

