Question: The National Center for Health Statistics published
The National Center for Health Statistics published the following data on the leading causes of death in 2010 in National Vital Statistics Reports. Deaths are classified according to the tenth revision of the International Classification of Diseases. Rates are per 100,000 population.
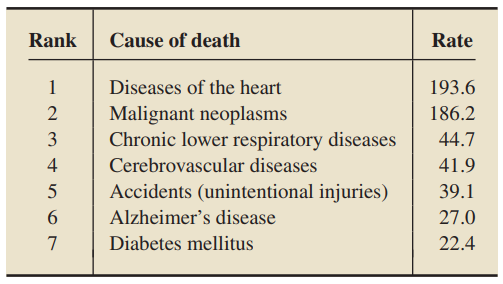
Do you think that these rates are descriptive statistics or inferential statistics? Explain your answer.
> The normal probability plot and stem-and-leaf diagram of the data are depicted in Fig. σ is unknown.
> Regarding a hypothesis test: a. What is the procedure, generally, for deciding whether the null hypothesis should be rejected? b. How can the procedure identified in part (a) be made objective and precise?
> The normal probability plot and histogram of the data are depicted in Fig; σ is known.
> College basketball, and particularly the NCAA basketball tournament, is a popular venue for gambling, from novices in office betting pools to high rollers. To encourage uniform betting across teams, Las Vegas oddsmakers assign a point spread to each game
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The carapace lengths, to the nearest hundredth of a millimeter, of a sample of 50 giant tarantulas.
> The Federal Bureau of Investigation (FBI) compiles information on robbery and property crimes by type and selected characteristic and publishes its findings in Uniform Crime Reports. According to that document, the mean value lost to purse snatching was
> The following table provides last year’s cheese consumption, in pounds, for 35 randomly selected Americans. a. At the 10% significance level, do the data provide sufficient evidence to conclude that last year’s mean cheese consumption for all Americans h
> Cheese Consumption. The null and alternative hypotheses for the hypothesis test are, respectively, H0: μ = 33 lb (mean has not increased) Ha: μ > 33 lb (mean has increased), where μ is last year’s mean cheese consumption for all Americans. Explain what
> The U.S. Department of Agriculture reports in Food Consumption, Prices, and Expenditures that the average American consumed 33 lb of cheese in 2010. Suppose that you want to decide whether last year’s mean cheese consumption is greater than the 2010 mean
> In each part, we have identified a hypothesis-testing procedure for one population mean. State the assumptions required and the test statistic used in each case. a. one-mean t-test b. one-mean z-test
> Discuss the difference between statistical significance and practical significance.
> What is meant when we say that a hypothesis test is a. exact? b. approximately corrects?
> Assess the evidence against the null hypothesis if the P-value of the hypothesis test is 0.062.
> The following statement appeared on a box of Tide laundry detergent: “Individual packages of Tide may weigh slightly more or less than the marked weight due to normal variations incurred with high speed packaging machines, but each day’s production of Ti
> State the general steps of the P-value approach to hypothesis testing.
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The gas mileages, rounded to the nearest number of miles per gallon, of all new car models.
> In each part, we have given the value obtained for the test statistic, z, in a one-mean z-test. We have also specified whether the test is two tailed, left tailed, or right tailed. Determine the P-value in each case and decide whether, at the 5% signific
> How is the P-value of a hypothesis test actually determined?
> Explain why the P-value of a hypothesis test is also referred to as the observed significance level.
> State the decision criterion for a hypothesis test, using the P-value.
> True or false: A P-value of 0.02 provides more evidence against the null hypothesis than a P-value of 0.03. Explain your answer.
> Define the P-value of a hypothesis test.
> State the general steps of the critical-value approach to hypothesis testing.
> The following graph portrays the decision criterion for a one mean z-test, using the critical-value approach to hypothesis testing. The curve in the graph is the normal curve for the test statistic under the assumption that the null hypothesis is true. D
> Determine the critical value(s) for a one-mean z-test at the 1% significance level if the test is a. right tailed. b. left tailed. c. two tailed.
> Explain the meaning of each term. a. null hypothesis b. alternative hypothesis c. test statistic d. significance level
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The number of automobiles per family.
> A variable of a population has a mean of 266 and a standard deviation of 16. Ten observations of this variable have a mean of 262.1 and a sample standard deviation of 20.4. Obtain the observed value of the a. standardized version of x¯. b. studentized v
> Suppose that you plan to apply the one-mean z-interval procedure to obtain a 90% confidence interval for a population mean, μ. You know that σ = 12 and that you are going to use a sample of size 9. a. What will be your margin of error? b. What else do yo
> A confidence interval for a population mean has a margin of error of 10.7. a. Obtain the length of the confidence interval. b. If the mean of the sample is 75.2, determine the confidence interval. c. Express the confidence interval in the form “point est
> Suppose that you intend to find a 95% confidence interval for a population mean by applying the one-mean z-interval procedure to a sample of size 100. a. What would happen to the accuracy of the estimate if you used a sample of size 50 instead but kept t
> Suppose that you have obtained a sample with the intent of performing a particular statistical inference procedure. What should you do before applying the procedure to the sample data? Why?
> If you obtained one thousand 95% confidence intervals for a population mean, μ, roughly how many of the intervals would actually contain μ?
> Must the variable under consideration be normally distributed for you to use the z-interval procedure or t-interval procedure? Explain your answer.
> The rare, booted eagle of western Europe was the focus of a study by S. Suarez et al. to identify optimal nesting habitat for this raptor. According to their paper “Nesting Habitat Selection by Booted Eagles (Hieraaetus pennatus) and Implications for Man
> In the online article “Old Faithful at Yellowstone, a Bimodal Distribution,” D. Howell examined various aspects of the Old Faithful Geyser at Yellowstone National Park. Despite its name, there is considerable variation in both the length of the eruptions
> The U.S. Department of Energy collects fuel economy information on new motor vehicles and publishes its findings in Fuel Economy Guide. The data included are the result of vehicle testing done at the Environmental Protection Agency’s National Vehicle and
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The additional sleep, to the nearest tenth of an hour, obtained by a sample of 100 patients by using a particular br
> The convict surgeonfish is a common tropical reef fish that has been found to delay metamorphosis into adults by extending its larval phase. This delay often leads to enhanced survivorship in the species by increasing the chances of finding suitable habi
> Wildfires are uncontrolled fires that usually spread quickly and are common in wilderness areas that have long and dry summers. The National Interagency Fire Center reports statistics on wildfires on their website www.nifc.gov. The following data lists t
> In a Singapore edition of Business Times, diamond pricing was explored. The price of a diamond is based on the diamond’s weight, color, and clarity. A simple random sample of 18 one-half-carat diamonds had the following prices, in dollars. a. Apply the t
> The paper “Correlations between the Intrauterine Metabolic Environment and Blood Pressure in Adolescent Offspring of Diabetic Mothers” (Journal of Pediatrics, Vol. 136, Issue 5, pp. 587–592) by N. Cho et al. presented findings of research on children of
> Refer to Problem 21. a. Find the margin of error, E. b. Explain the meaning of E as far as the accuracy of the estimate is concerned. c. Determine the sample size required to have a margin of error of 0.5 year and a 90% confidence level. d. Find a 90% co
> Researchers M. Dhami et al. discussed how people adjust to prison life in the article “Adaption to Imprisonment” (Criminal Justice and Behavior, Vol. 34, No. 8, pp. 1085–1100). A sample of 712 federally sentenced adult male prisoners had an average sente
> We know that “a 95% confidence interval for the mean age of all U.S. millionaires is from 54.3 years to 62.8 years.” Decide which of the following sentences provide a correct interpretation of the statement in quotes. Justify your answers. a. Ninety-five
> Answer true or false to the following statement and give a reason for your answer: If a 95% confidence interval for a population mean, μ, is from 33.8 to 39.0, the mean of the population must lie somewhere between 33.8 and 39.0.
> Dr. Thomas Stanley of Georgia State University has surveyed millionaires since 1973. Among other information, Stanley obtains estimates for the mean age, μ, of all U.S. millionaires. Suppose that 36 randomly selected U.S. millionaires are the following a
> For a t-curve with df = 18, obtain the t-value and illustrate your results graphically. a. The t-value having area 0.025 to its right b. t0.05 c. The t-value having area 0.10 to its left d. The two t-values that divide the area under the curve into a mid
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The ages of householders, given as a whole number.
> A random sample of size 13 is taken from a population. A normal probability plot of the sample data shows no outliers but has significant curvature. The population standard deviation is unknown. Decide whether the appropriate method for obtaining the con
> A random sample of size 128 is taken from a population. A normal probability plot of the sample data shows no outliers but has significant curvature. The population standard deviation is known. Decide whether the appropriate method for obtaining the conf
> A random sample of size 20 is taken from a population. A normal probability plot of the sample data shows three outliers but is otherwise roughly linear. Removal of the outliers is questionable. The population standard deviation is unknown. Decide whethe
> A random sample of size 25 is taken from a population. A normal probability plot of the sample data shows three outliers but is otherwise roughly linear. Checking reveals that the outliers are due to recording errors and are really not outliers. The popu
> A random sample of size 50 is taken from a population. A boxplot of the sample data reveals no outliers. The population standard deviation is known. Decide whether the appropriate method for obtaining the confidence interval is the z-interval procedure,
> A random sample of size 17 is taken from a population. A normal probability plot of the sample data is found to be very close to linear (straight line). The population standard deviation is unknown. Decide whether the appropriate method for obtaining the
> The following figure shows the standard normal curve and two t-curves. Which of the two t-curves has the larger degrees of freedom? Explain your answer.
> The paper “Are Babies Normal?” by T. Clemons and M. Pagano (The American Statistician, Vol. 53, No. 4, pp. 298–302) focused on birth weights of babies. According to the article, for babies born within the “normal” gestational range of 37–43 weeks, birth
> Explain the difference between a point estimate of a parameter and a confidence-interval estimate of a parameter.
> Edmunds.com publishes information on new car prices in Car Shopping Trends Report. During a recent year, Americans spent an average of $30,803 for a new car. Assume a standard deviation of $10,200. a. Identify the population and variable under considerat
> We have presented a “data scenario.” In each case, decide which type of grouping (single-value, limit, or cutpoint) is probably the best? The number of bedrooms per single-family dwelling.
> In 2010, the Internal Revenue Service (IRS) sampled 308,946 tax returns to obtain estimates of various parameters. Data were published in Statistics of Income, Individual Income Tax Returns. According to that document, the mean income tax per return for
> The following graph shows the curve for a normally distributed variable. Superimposed are the curves for the sampling distributions of the sample mean for two different sample sizes. a. Explain why all three curves are centered at the same place. b. Whic
> Refer to Problem 5. a. Use the answer you obtained in Problem 5(b) and Definition 3.11 on page 140 to find the mean of the variable x¯. Interpret your answer. b. Can you obtain the mean of the variable x¯ without doing the calculation in part (a)? Explai
> The following table gives the monthly salaries (in $1000s) of the six officers of a company. a. Calculate the population mean monthly salary, μ. There are 15 possible samples of size 4 from the population of six officers. They are listed in the first col
> Relative to the population mean, what happens to the possible sample means for samples of the same size as the sample size increases? Explain the relevance of this property in estimating a population mean by a sample mean.
> Provide two synonyms for “the distribution of all possible sample means for samples of a given size.”
> What is the sampling distribution of a statistic? Why is it important?
> A variable is said to be uniformly distributed or to have a uniform distribution with parameters a and b if its distribution has the shape of the horizontal line segment with equation y = 1/(b − a), for a < x < b. The mean and standard deviation of such
> The Athletic Coping Skills Inventory (ACSI) is a test to measure psychological skills believed to influence athletic performance. Researchers E. Estanol et al. studied the relationship between ACSI scores and eating disorders in dancers in the article “M
> In the paper “Cloudiness: Note on a Novel Case of Frequency” (Proceedings of the Royal Society of London, Vol. 62, pp. 287–290), K. Pearson examined data on daily degree of cloudiness, on a scale of 0 to 10, at Breslau (Wroclaw), Poland, during the decad
> Suppose that you have constructed a stem-and-leaf diagram and discover that it is only moderately useful because there are too few stems. How can you remedy the problem?
> A paint manufacturer in Pittsburgh claims that his paint will last an average of 5 years. Assuming that paint life is normally distributed and has a standard deviation of 0.5 year, answer the following questions: a. Suppose that you paint one house with
> The American Council of Life Insurers provides information about life insurance in force per covered family in the Life Insurers Fact Book. Assume that the standard deviation of life insurance in force is $50,900. a. Determine the probability that the sa
> In the article “Drinking Glucose Improves Listening Span in Students Who Miss Breakfast” (Educational Research, Vol. 43, No. 2, pp. 201–207), authors N. Morris and P. Sarll explored the relationship between students who skip breakfast and their performan
> Refer to Problem 12. a. Find the percentage of all samples of four pygmy-possums that have mean weights within 0.225 g of the population mean weight of 8.5 g. b. Obtain the probability that the mean weight of four randomly selected pygmy-possums will be
> The foraging behavior of the western pygmy-possum was investigated in the article “Strategies of a Small Nectarivorous Marsupial, the Western Pygmy-Possum, in Response to Seasonal Variation in Food Availability” (Journal of Mammalogy, Vol. 96, No. 6, pp.
> Repeat Problem 10, assuming that the number of hours worked by female marketing and advertising managers is normally distributed. Data from Problem 10: In the article “How Hours of Work Affect Occupational Earnings” (Monthly Labor Review, Vol. 121), D.
> In the article “How Hours of Work Affect Occupational Earnings” (Monthly Labor Review, Vol. 121), D. Hecker discussed the number of hours actually worked as opposed to the number of hours paid for. The study examines both full-time men and full-time wome
> Define sampling error.
> Explain the relationship between percentages for a normally distributed variable and areas under the corresponding normal curve.
> Answer true or false to each statement. Give reasons for your answers. a. Two variables that have the same mean and standard deviation have the same distribution. b. Two normally distributed variables that have the same mean and standard deviation have t
> Suppose that you have a data set that contains a large number of observations. Which graphical display is generally preferable: a histogram or a stem-and-leaf diagram? Explain your answer.
> Define a. normally distributed variable. b. normally distributed population. c. parameters for a normal curve.
> State two of the main reasons for studying the normal distribution.
> A variable has the density curve with equation y = 1 − x/2 for 0 < x < 2, and y = 0 otherwise. a. Graph the density curve of this variable. b. Show that the area under this density curve to the left of any number x between 0 and 2 equals x − x 2/4. What
> Assume that the variable under consideration has a density curve. Note that the answers required here may be only approximately correct. The area under a density curve that lies between 5 and 6 is 0.728. What percentage of all possible observations of th
> In an issue of National Mortgage News, a special report was published on publicly traded mortgage industry companies. A sample of 25 mortgage industry companies had the following numbers of employees. a. Obtain a normal probability plot of the data. b. U
> Researchers M. Kroll et al. studied the influence of paternity on rates of mortality and development in eggs and larvae of Northwest Atlantic cod in the article, “Paternal Effects on Early Life History Traits in Northwest Atlantic Cod, Gadus morhua” (Jou
> Assume that the variable under consideration has a density curve. Note that the answers required here may be only approximately correct. The area under a density curve that lies to the left of 60 is 0.364. What percentage of all possible observations of
> Refer to Problem 28, and fill in the following blanks. a. Approximately 68% of students who took the verbal portion of the GRE scored between ___ and ___. b. Approximately 95% of students who took the verbal portion of the GRE scored between ___ and ___.
> The Graduate Record Examination (GRE) is a standardized test that students usually take before entering graduate school. According to the document GRE Guide to the Use of Scores, a publication of the Educational Testing Service, the scores on the verbal
> The study “Intrathecal Sufentanil versus Fentanyl for Lower Limb Surgeries – A Randomized Controlled Trial” (Journal of Anaesthesiology Clinical Pharmacology, Vol. 27, Issue 1, pp. 67–73) by P. Motiani et al. compares two different agents, intrathecal su
> Discuss the relative advantages and disadvantages of stem-andleaf diagrams versus frequency histograms.
> The WONDER database, maintained by the Centers for Disease Control and Prevention, provides a single point of access to a wide variety of reports and numeric public health data. From that database, we obtained the following data for one year’s birth weig
> In 1903, K. Pearson and A. Lee published a paper entitled “On the Laws of Inheritance in Man. I. Inheritance of Physical Characters” (Biometrika, Vol. 2, pp. 357–462). From information presented in that paper, forearm length of men, measured from the elb
> A coffee machine is supposed to dispense 6 fluid ounces (fl oz) of coffee into a paper cup. In reality, the amounts dispensed vary from cup to cup. In fact, the amount dispensed, in fl oz, is a variable with density curve y = 2 for 5.75 < x < 6.25, and y
> For the standard normal curve, find the z-score(s) a. that has area 0.30 to its left. b. that has area 0.10 to its right. c. z0.025, z0.05, z0.01, and z0.005. d. that divide the area under the curve into a middle 0.99 area and two outside 0.005 areas.
> Determine and sketch the area under the standard normal curve that lies a. to the left of −3.02. b. to the right of 0.61. c. between 1.11 and 2.75. d. between −2.06 and 5.02. e. between −4.11 and −1.5. f. either to the left of 1 or to the right of 3.
> According to Table II, the area under the standard normal curve that lies to the left of 1.05 is 0.8531. Without further reference to Table II, determine the area under the standard normal curve that lies a. to the right of 1.05. b. to the left of −1.05
> Sketch the normal curve having the parameters a. μ = −1 and σ = 2. b. μ = 3 and σ = 2. c. μ = −1 and σ = 0.5.

