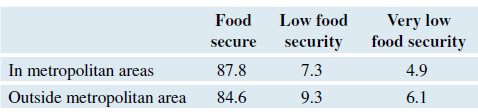
Question: The table shows the percentage of all
The table shows the percentage of all U.S. households who are food secure, have low food security, or who have very low food security. The data are reported by area of residence. Give two reasons why it would be inappropriate to do a chi-square test to determine if there is an association between food security and area of residence. (Source: 2017 World Almanac and Book of Facts)
> The 2018 Pew Research poll in exercise 10.43 also reported responses by political party. Survey results found 45% of Republicans and 69% of Democrats supported marijuana legalization. a. Use these results to fill in the following two-way table with the c
> A 2018 Pew Research poll asked a random sample of Millennials and GenXers if they supported legalization of marijuana. Survey results found 70% of Millennials and 66% of GenXers supported marijuana legalization. a. Use these results to fill in the follow
> Exercise 10.11 on artery disease in mummies indicated that 9 out of 16 mummies showed heart disease (hardening of the arteries). Test the hypothesis that the population proportion of mummies with hardening of the arteries is not the same as in the modern
> A 2017 study explored the impact of Yoga and Meditation based Lifestyle Intervention (YMLI) on cellular aging in healthy individuals (Tohlahunase et al. 2017). Ninety-six healthy individuals were enrolled in the 12-week YMLI course, which consisted of yo
> Professional musicians listened to five violins being played, without seeing the instruments. One violin was a Stradivarius, and the other four were modern-day violins. When asked to pick the Stradivarius (after listening to all five), 39 got it right an
> The table shows the results of rolling a six-sided die 120 times. Test the hypothesis that the die is not fair. A fair die should produce equal numbers of each outcome. Use the four-step procedure with a significance level of 0.05, and state your conclus
> See exercise 10.21 for an explanation of playing with the dreidel. This time the family used a plastic dreidel and got the following outcomes. The four outcomes are believed to be equally likely (that is, has a uniform probability distribution). Determin
> When playing Dreidel, (see photo) you sit in a circle with friends or relatives and take turns spinning a wobbly top (the dreidel). In the center of the circle is a pot of several foil-wrapped chocolate coins. If the four-sided top lands on the Hebrew le
> In 2018 Pew Research reported that 11% of Americans do not use the Internet. Suppose in a random sample of 200 Americans, 26 reported not using the Internet. Using a chi-square test for goodness-of-fit, test the hypothesis that the proportion of American
> A penny was spun on a hard, flat surface 50 times, and the result was 15 heads and 35 tails. Using a chisquare test for goodness of fit, test the hypothesis that the coin is biased, using a 0.05 level of significance.
> The mean age of all 118 used Toyota vans for sale (see exercise 9.16) was 3.1 years with a standard deviation of 2.7 years. The distribution of ages is right skewed. For a statistics project, a student randomly selects 35 vans from this data set and find
> One histogram shows the distribution of costs for all used Toyota Sienna vans for sale within a 100 mile radius of San Leandro, CA, for a day in 2018. The other three graphs show distributions of means from random samples taken from this population based
> According to a 2018 Money magazine article, the average income in Kansas is $53,906. Suppose the standard deviation is $3000 and the distribution of income is right skewed. Repeated random samples of 400 Kansas residents are taken, and the sample mean of
> According to a 2018 Money magazine article, Maryland has one of the highest per capita incomes in the United States, with an average income of $75,847. Suppose the standard deviation is $32,000 and the distribution is right-skewed. A random sample of 100
> In a 2017 study published in Scientific Reports, researchers (Mubanga et al. 2017) concluded that “dog ownership was associated with a lower risk of incident cardiovascular disease in single-person households and with lower cardiovascular and all-cause m
> Use the information for exercise 11.20. a. Which class had the highest sample mean number of TV hours, and which class had the lowest sample mean? (1 is for freshman, 2 is for sophomore, and so on.) b. Write out the null and alternative hypotheses for th
> Use the information for exercise 11.19. a. Which class had the highest sample mean, and which class had the lowest sample mean? (1 is for freshman, 2 is for sophomore, and so on.) b. Write out the null and alternative hypotheses for the effect of class o
> Test the hypothesis that people with different marital statuses differ in mean systolic blood pressure, using a significance level of 0.05. Refer to the Stat- Crunch output from NHANES data. a. Write the null and alternative hypotheses for the associatio
> Refer to the StatCrunch output from National Health and Nutrition Examination Survey (NHANES) data, which shows the association between marital status and cholesterol. Assume the population distributions are close enough to Normal to justify using ANOVA.
> One of the authors collected data from a class to see whether humans made selections randomly, as a random number generator would. Each of 38 students had to pick an integer from one to five. The data are summarized in the table. A true random number gen
> Suppose you had to identify the color of ink for a series of printed words that spelled out a color that did not match the ink color. For example, what color ink is used in the word RED? This might take longer than identifying the color when the ink and
> Some people believe that it is easier to read words printed on colored paper than words printed on white paper. To test this theory, statistics student Paula Smith collected data. Subjects were timed as they read a passage printed in black ink on a sheet
> Repeat the chi-square test (all four steps) from exercise 10.23, but this time assume that you got exactly 20 outcomes in each of the six categories. Refer to the figure. Explain.
> In a 2017 study, researchers investigated the effect of dietary improvement on adults with moderate to severe depression (Jacka et al. 2017). Subjects were randomly assigned to a treatment group consisting of seven individual nutritional consulting sessi
> Suppose you grow tomato plants in a greenhouse and sell the tomatoes by weight, so the amount of money you make depends on plants producing a large total weight of tomatoes. You want to determine which of two fertilizers will produce a heavier harvest of
> Phubbing is the practice of ignoring one’s companion or companions in order to pay attention to one’s phone or other mobile device. In the conclusion of a 2017 study published in Personality and Individual Differences, researchers (Wang et al. 2017) conc
> Suppose that you want to determine whether the use of one aspirin per day for people age 50 and older reduces the chance of heart attack. You have 200 people available for the study: 100 men and 100 women. You suspect that aspirin might affect men and wo
> Suppose you want to compare the effectiveness of the flu vaccine in preventing the flu using one of two different forms: nasal spray versus injection. Suppose you have 60 subjects available of different ages, and you suspect that age might have an effect
> Speed skating is a sport in which it is important to have a suit that minimizes wind drag as much as possible, as the difference between winning and losing a race can be as small as a thousandth of a second. In the 2014 Winter Olympics, U.S. speed skater
> We counted ones, twos, threes, fours, and fives from a few lines of a random number table, and we should expect to get equal numbers of each. (We ignored the sixes, sevens, eights, nines, and zeros.) There were 14 ones, 12 twos, 16 threes, 11 fours, and
> Researchers are interested in testing whether a video game that is designed to increase brain activity actually works. To test this, they plan to randomly assign subjects to either the treatment group (spend 15 minutes per day playing the game) or a cont
> Suppose an SAT tutoring company really can improve SAT scores by 10 points, on average. A competing company, however, uses a more intense tutoring approach and really can improve SAT scores by 15 points, on average. Suppose you’ve been hired by both comp
> Refer to the figure. Assume that all distributions are symmetric (therefore the sample mean and median are approximately equal) and that all the samples are the same size. Imagine carrying out two ANOVAs. The first compares the means based on samples A,
> Dravet syndrome is a complex childhood epilepsy disorder. Researchers Devinsky et al., conducted a double-blind placebo-controlled trial to determine the efficacy of the drub cannabiliol on reducing seizures in children with Dravet syndrome. One hundred
> A 2017 Pew poll asked a random sample “Are men and women basically different in how they express feelings?” The results by gender (in percentages) are shown in the table below. a. Assume the sample size for each gender
> Some sources report that the weights of full-term newborn babies have a mean of 7 pounds and a standard deviation of 0.6 pound and are Normally distributed. In the given outputs, the shaded areas (reported as p=) represent the probability that the mean w
> A random sample of 50 college first-year students (out of a total of 1000 first-years) was obtained from college records using systematic sampling. Half of those students had a campus tour with a sophomore student, and half had a tour with an instructor.
> Some sources report that the weights of full-term newborn babies have a mean of 7 pounds and a standard deviation of 0.6 pound and are Normally distributed. a. What is the probability that one newborn baby will have a weight within 0.6 pound of the mean—
> Samples of rents for one-bedroom, one-bath apartments in three cities were obtained. Assume the distribution of each population is Normal to satisfy the conditions for using ANOVA. Test the hypothesis that the mean rent for one-bedroom apartments in at l
> Based on the following output, would it be appropriate to use ANOVA to determine if the mean gas prices for one of these five cities is significantly different from that of the other cities? If so, perform the ANOVA. If not, explain why it is not appropr
> In the study referenced in exercise 10.8, researchers also asked whether or not students bought fast food at least one to two times per week. The data are reported by gender in the table. a. Find the row, column, and grand totals, and prepare a table sho
> Construct heights for 3 or more sets of twins (6 or more people). Make the twins similar, but not exactly the same, in height. Put all of the shorter twins in set A and all of the taller twins in set B. Create the numbers such that a two-sample t-test wi
> Construct two sets of body temperatures (in degrees Fahrenheit, such as 96.2 °F), one for men and one for women, such that the sample means are different but the hypothesis test shows the population means are not different. Each set should have three num
> The table shows the heights (in inches) of all the basketball players on the 2017–2018 Santa Clara University basketball team. Explain why it would be inappropriate to do a t-test with these data.
> A survey was given to StatCrunch users on the length of time for commuting and the method of commuting. Assume this is a random sample. Minitab output for one-way ANOVA is given, along with the means and standard deviations. Divide the largest standard d
> Pulse rates were taken for five people, each in three different situations: sitting, after meditation, and after exercise. Explain why it would not be appropriate to use one-way ANOVA to test whether the population mean pulse rates were associated with a
> Go back to the information in exercise 11.20. Assuming the conditions for ANOVA are met, test the hypothesis that the mean number of hours of TV varies by class, reporting the p-value and conclusion. Use the 0.05 level of significance. State your conclus
> Go back to the information in exercise 11.19. Assuming the conditions for ANOVA are met, test the hypothesis that the mean number of hours of schoolwork varies by class, reporting the p-value and conclusion. Use the 0.05 level of significance. State your
> Some software (such as SPSS) requires that ANOVA data be stacked and coded. Some software works with both stacked and unstacked data, and some (such as the TI-84) requires unstacked data. Go back to the information given in exercise 11.7. Stack and code
> The table shows the percentage of all men and women in the United States aged 18 to 44 who meet aerobic fitness guidelines. Give two reasons why a chi-square test is not appropriate for this data. (Source: 2017 World Almanac and Book of Facts)
> In a 2015 study by Nanney et al. and published in the Journal of American College Health, a random sample of community college students was asked whether they ate breakfast 3 or more times weekly. The data are reported by gender in the table. a. Find the
> A large number of surgery patients get infections after surgery, which can sometimes be quite serious. Researchers randomly assigned some surgery patients to receive a simple antibiotic ointment after surgery, others to receive a placebo, and others to r
> Suppose a polling organization asks a random sample of people if they are Democrat, Republican, or Other and asks them if they think the country is headed in the right direction or the wrong direction. If we wanted to test whether party affiliation and a
> A random sample of 12th-grade students were asked to rate the importance of reducing pollution on a scale from 0 to 1000. Responses were recorded by gender. The results of a hypothesis test are shown. Assume the conditions for using a two-sample t-test a
> State whether each of the following changes would make a confidence interval wider or narrower. (Assume that nothing else changes.) a. Changing from a 95% level of confidence to a 90% level of confidence b. Changing from a sample size of 30 to a sample s
> State whether each of the following changes would make a confidence interval wider or narrower. (Assume that nothing else changes.) a. Changing from a 90% confidence level to a 99% confidence level b. Changing from a sample size of 30 to a sample size of
> In exercise 9.31, two intervals were given for the same data, one for 95% confidence and one for 90% confidence. a. How would a 99% interval compare? Would it be narrower than both, wider than both, or between the two in width. Explain. b. If we wanted t
> Choose a t-test for each situation: one-sample t-test, two-sample t-test, paired t-test, and no t-test. a. A random sample of car dealerships is obtained. Then a student walks onto each dealer’s lot wearing old clothes and finds out how long it takes (in
> Choose a test for each situation: one-sample t-test, two-sample t-test, paired t-test, and no t-test. a. A random sample of students who transfered to a 4-year university from community colleges are asked their GPAs. Our goal is to determine whether the
> A random sample of 25 baseball players from the 2017 Major League Baseball season was taken and the sample data was used to construct two confidence intervals for the population mean. One interval was (22.0, 42.8). The other interval was (19.9, 44.0). (S
> Treatment In a 2018 study by Zhu et al. reported in The Lancet, researchers conducted an experiment to determine the efficacy and safety of the drug dorzagliatin in the treatment of patients with Type 2 diabetes. In this double-blind study, patients were
> In a 2018 study reported in The New England Journal of Medicine, Johnston et al. studied the effect of a combination of the drug clopidogrel and aspirin on reducing the rate of recurrent stroke among stroke patients. Stroke patients in the study were ran
> A 2018 Gallup poll asked college graduates if they agreed that the courses they took in college were relevant to their work and daily lives. The respondents were also classified by their field of study. If we wanted to test whether there was an associati
> A fast-food chain sells drinks that it calls HUGE. When we take a sample of 25 drinks and weigh them, we find that the mean is 36.3 ounces with a standard deviation of 1.5 ounces. a. State how you would fill in the numbers below to do the calculation wit
> A random sample of 30 12th-grade students was selected. The sample mean height was 170.7 centimeters, and the sample standard deviation was 11.5 centimeters. (Source: AMSTAT Census at School) a. State how you would fill in the numbers below to do the cal
> A researcher collects a sample of 25 measurements from a population and wants to find a 99% confidence interval. a. What value should he use for t*? (Recall that df = n - 1 for a one sample t-interval.) Use the table given for Exercise 9.25. b. Why is th
> A researcher collects one sample of 27 measurements from a population and wants to find a 95% confidence interval. What value should he use for t*? (Recall that df = n - 1 for a one-sample t-interval.)
> If you take samples of 40 lines from a random number table and find that the confidence interval for the proportion of odd-numbered digits captures 50% 37 times out of the 40 lines, is it the confidence interval or confidence level you are estimating
> Some software (such as SPSS) requires that ANOVA data be stacked and coded. Some software works with both stacked and unstacked data, and some (such as the TI-84) requires unstacked data. Go back to the information given in exercise 11.8. Stack and code
> The weights of four randomly chosen bags of horse carrots, each bag labeled 20 pounds, were 20.5, 19.8, 20.8, and 20.0 pounds. Assume that the distribution of weights is Normal. Find a 95% confidence interval for the mean weight of all bags of horse carr
> A statistics instructor randomly selected four bags of oranges, each bag labeled 10 pounds, and weighed the bags. They weighed 10.2, 10.5, 10.3, and 10.3 pounds. Assume that the distribution of weights is Normal. Find a 95% confidence interval for the me
> A researcher wants to determine whether the faculty-to-student ratio tends to be different in private colleges from that in public colleges. She has an almanac that lists this information for all accredited colleges. She creates two subgroups: one for pr
> Mark Bates, a statistics professor at Oxnard College, recorded his commuting times using three different routes from home to work. The routes are named for the streets on which he traveled, and the times are in seconds. For the boxplots given, compare th
> The following table shows the average number of vehicles sold in the United States monthly (in millions) for the years 2001 through 2018. Data on all monthly vehicle sales for these years were obtained and the average number per month was calculated. Wou
> In 2017 the Pew Research Center published a report on the demographics of the U.S. military. The following table shows the ethnic breakdown of active-duty U.S. military services and the ethnic breakdown of the U.S. population. Would it be appropriate to
> A college administrator wants to determine whether the professors at the college are doing a good job. Each professor teaches multiple classes, and so for each professor, one of his or her classes is randomly chosen, and all the students are surveyed to
> A large concert promoter that operates several hundred concert locations around the country wants to survey the managers at these locations to ask their opinions about how to improve attendance at concerts. Because the survey is rather lengthy, the promo
> Suppose a homeowner is considering replacing the grass in the front yard with drought-resistant plants such as cactus. She wants to find out whether the neighbors approve of this or not, so she inquires about this at every fifth house in the subdivision.
> Suppose a college is deciding whether or not to allocate more resources to the purchase of audio books for the college library. Explain why the college might want to use a stratified sample rather than sampling the entire college before making a decision
> Suppose a college wishes to select the location of an electric car charging station on campus based on student preference. They have 3 possible locations and are asking a random sample of students to rank the locations with 1 being the most desirable loc
> Suppose a person with access to student records at your college has a list of currently enrolled students. The person sorts the data to create two new lists. One contains all the male names, the other all the female names. The person then uses a random n
> Suppose a person with access to student records at your college has an alphabetical list of currently enrolled students. The person looks at the records of every 10th person (starting with a randomly selected person among the first 10) to see whether the
> Refer to the following figure. Assume that all data sets are symmetric and that all the samples are the same size. Imagine carrying out two ANOVAs. The first compares the means based on samples A, B, and C (above the horizontal line), and the other is ba
> According to a 2017 report by ComScore .com, the mean time spent on smartphones daily by the American adults is 2.85 hours. Assume this is correct and assume the standard deviation is 1.4 hours. a. Suppose 150 American adults are randomly surveyed and as
> Using NHANES data, we performed one-way ANOVA and a two-sample t-test. In both cases we were testing the hypothesis that the mean cholesterol values for men and women are different. Compare the output of ANOVA and the two-sample t-test by looking at the
> Using the NHANES data, we performed an ANOVA to test whether gender is associated with level of triglycerides, a form of fat, in the blood. ANOVA and t-test output from a two-sample t-test is shown. In both cases, we are testing the hypothesis that the m
> A random survey was done at a small Lutheran college, and the students were asked how many hours a week they spent watching TV. They were also asked what class they were in (1 = Freshman, 2 = Sophomore, 3 = Junior, 4 = Senior). The survey was given only
> A random survey was done at a small Lutheran college, and the students were asked how many hours a week they spent studying outside of class time. They were also asked what class they were in (1 = Freshman, 2 = Sophomore, 3 = Junior, and 4 = Senior). a.
> The mean age of all 2550 students at a small college is 22.8 years with a standard deviation is 3.2 years, and the distribution is right-skewed. A random sample of 4 students’ ages is obtained, and the mean is 23.2 with a standard deviation of 2.4 years.
> Professors of ethics (Eth), professors of philosophy (Phil), and professors in fields other than philosophy or ethics (Other) were asked how many days it had been since they had last been in contact with their mothers. Contact was defined as face-to-face
> Suppose you collect data on GPAs by classroom row in which the student chose to sit, and that there are four rows. Suppose you do multiple two-sample t-tests to compare the mean GPA of the rows, and discover that the p-value comparing the means of Row 1
> Consider the data from the happiness survey (see exercise 11.40). If you do a series of two-sample t-tests comparing mean happiness of all pairs of the seven age groups, you will find that the p-value that compares happiness of those in their forties wit
> Fill in the blank by choosing one of the options given: Chi-square goodness-of-fit tests are applicable if the data consist of ___________ (one categorical variable, two categorical variables, one numerical variable, or two numerical variables).
> Fill in the blank by choosing one of the options given: Chi-square goodness-of-fit data are often summarized with ____________ (one row or one column of observed counts—but not both, or at least two rows and at least two columns of observed counts).
> According to home-water-works.org, the average shower in the United States lasts 8.2 minutes. Assume this is correct, and assume the standard deviation of 2 minutes. a. Do you expect the shape of the distribution of shower lengths to be Normal, right-ske
> One of the histograms is a histogram of a sample (from a population with a skewed distribution) one is the distribution of many means of repeated random samples of size 5, and one is the distribution of repeated means of random samples of size 25; all th
> In a 2018 study reported in The Lancet, a randomized, double-blind controlled experiment was conducted to determine the effect of the drug upadacitinib on patients with active rheumatoid arthritis. Patients were randomly assigned to receive the drug or a
> In a 2018 study reported in The New England Journal of Medicine, Halpern et al. randomly assigned smokers to one of five groups, including four smoking cessation interventions and usual care. Usual care consisted of access to information regarding the be
> Using the information from exercise 12.4, write two headlines announcing the results of the study. Make one of the headlines imply causality and make the other one not imply causality. Clearly label each headline. Which headline is appropriate for these
> Using the information from exercise 12.3, write two headlines announcing the results of the study. Make one of the headlines imply causality and make the other one not imply causality. Clearly label each headline. Which headline is appropriate for these

