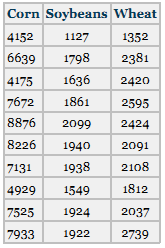
Question: The U.S. Department of Agriculture publishes
The U.S. Department of Agriculture publishes data annually on various selected farm products. Shown here are the unit production figures (in millions of bushels) for three farm products for 10 years during a 20-year period. Use these data and multiple regression analysis to predict corn production by the production of soybeans and wheat. Comment on the results.
> In testing the overall significance of a multiple regression model, the null hypothesis that each one of the β-coefficients of the x-variables in the model is equal to zero, is tested against the alternative hypothesis that __________. a) Each one of the
> The graph of a regression equation with one independent variable, y = b0 + b1 x, yields a straight line in a two-dimensional (x, y) space. To graph a regression equation with two independent variables, y = b0 + b1 x1 + b2 x2, we require a 3-dimensional s
> Which of the following categories of business analytics builds and assesses algorithmic models aimed at making empirical rather than theoretical predictions and is designed to predict future observations: a) Descriptive analytics b) Predictive analytics
> The data in the table below represent number of months spent training (x) and finish times in minutes (y) for a 5K footrace.. Interpret the relationship between the variables x and y. a) There is a strong positive correlation. b) There is a weak positi
> A runner tracks her average weekly mileage while marathon training along with her marathon finish times, given by the table below. Classify the correlation between two variables. Weekly Mileage ______ Marathon Time (mins) 55 …â&
> The proportion of the variability in the dependent variable (y) accounted for or explained by the independent variable (x) is called the __________. a) Correlation coefficient b) Regression slope coefficient c) Regression sum of squares d) Coefficient o
> For a least-squares regression line, the sum of the residuals is __________. a) Always zero b) Always positive c) Always negative d) Sometimes positive and sometimes negative 12. Residuals with large magnitudes can be used to located data points that l
> A graphical tool to illustrate the relationship between matched observations of two variables (e.g., y = the annual cost of operating a commercial airliner and x = the annual number of passengers served by the airline), is __________. a) A histogram b) A
> After establishing an overall significant difference between the means of several groups using ANOVA if we want to determine which pairs of means are significant when the sample sizes are unequal, it is appropriate to use __________. a) A series of t-tes
> A process analyst wants to conduct a study to compare five different welding techniques that have been newly developed. Fifty welders, who work in three different shifts, are randomly selected and randomly assigned to try out a welding technique (each op
> To understand how the weights of the cereal boxes vary among four different adjustable stabilizer settings of the rotary valve on the filling machine in three different production shifts, a manufacturing firm collects sample data using a structure or pla
> A manager wants to test if there is a significant difference in the variance of the output from two similar machines. It has been determined that the output of the two machines is normally distributed. To carry out the hypothesis test about the populatio
> A human resources manager wants to conduct a “before/after” study on 16 employees to determine if a motivation seminar results in an increase in their ratings of the company. The after-ratings are subtracted from the b
> Some common applications of statistics in business include which of the following: a) Predicting the outcome of an election b) Determining consumer sentiment c) Estimating salary data for various demographics d) Comparing the spending of two different gr
> To determine whether two brands of car tires differ in their performance measured in terms of the average wear over the first 10,000 miles, we should conduct __________. a) One hypothesis test for the difference in the two population means b) Two hypothe
> A nutritionist for a university wants to determine an average BMI of its students by taking a random sample of 50 students. The standard deviation of the BMIs of population of students is unknown. She is willing to accept a Type I error rate of 0.05. The
> The dean of a business school claims that the average starting salary of its graduates is more than 85 (in $000’s). It is known that the population standard deviation is 10 (in $000’s). Sample data on the starting salaries of 64 randomly selected recent
> A test of the hypotheses, H0: p = 0.18 and H1: p > 0.18 is a __________. a) One-tailed test b) Two-tailed test c) Test of two sample means d) Test of two sample proportions 14. A test of the hypotheses, H0: p = 0.18 and H1: p ≠ 0.18 is a __________. a) O
> All statistical hypotheses consist of two parts, __________ and __________. a) A null hypothesis; a full hypothesis b) A null hypothesis; a substitute hypothesis c) A null hypothesis; an alternate hypothesis d) A null hypothesis; a substantive hypothesis
> A t-distribution is similar to the z-distribution except that __________. a) It is not unimodal b) It is not symmetrical c) It has more area in the tails d) It is not bell-shaped 21. The following random sample was selected from a normal distribution: 4
> 9. In a normal distribution, what values of z should we choose if we want 90% of the area under the curve symmetrically distributed around the mean? a) ( 1.00 b) ( 1.50 c) ( 1.645 d) ( 1.96 10. In a normal distribution, if we consider the range of values
> The luggage weights for passengers for an airline are normally distributed with a mean of 25 lbs., and a standard deviation of 3 lbs. Suppose the limit on the total luggage weight is 2600 lbs. If 100 passengers are taking the airline, what is the probabi
> Suppose samples of size 100 are drawn randomly from a population that has a mean of 20 and a standard deviation of 5. What is the probability of observing a sample mean between 19 and 20.5? a) 0.3413 b) 0.6826 c) 8165 d) 0.1587 e) 0.6587 15. Suppose sa
> Selection of the winning numbers is a lottery is an example of __________. a) Convenience sampling b) Random sampling c) Nonrandom sampling d) Regulatory sampling 7. A random number generator is usually a computer program which allows computer-calculated
> A score on a 15-point multiple choices quiz measuring knowledge of statistics is an example of a(n) a) Nominal b) Ordinal c) Interval d) Ratio e) Exponential 24. Which level of data measurement allows the most or broadest application of statistical techn
> The shape of a symmetrical binomial distribution with large sample size, i.e., a large number of possible outcomes, would most likely look like a __________. a) Rectangle b) Whale c) Bell d) Hockey stick 23. In which of the following cases would the
> All normal distributions can be converted into a single distribution called the __________. a) Universal or U-distribution b) General or G-distribution c) Standard normal or the z- distribution d) Common or C-distribution 14. To convert any x value of a
> If a continuous random variable has a uniform distribution between 20 and 70, the graph of its distribution will be a rectangle with height equal to __________. a) 50 b) 1/50 c) 20 d) 70 3. The values of a random variable are uniformly distributed
> Which of the following is a characteristic of a hypergeometric distribution? a) It is a continuous distribution. b) It has undefined trials c) The outcome of each trial is a success or a failure or undefined. d) Sampling is done without replacement. e)
> In the distribution of a random variable x which is binomial with number of trials n = 30 and the probability of success p = 0.99, the largest value of x that can occur is __________. a) 24 b) 30 c) 15 d) Infinity 14. One fair coin is tossed 10 times, w
> The random variable defined as the “number of people who arrive at a store during a 15-minute interval,” is an example of __________. a) An experimental random variable b) A continuous random variable c) A discrete random variable d) Not a random variabl
> Fifty percent of all technical assistants would like to have a PC. Eighty percent of all technical assistants would like to have MAC. Fourty-five percent of all technical assistants would like to have both. If a technical assistant is randomly selected,
> A fair coin is tossed 4 times and the events A and B are defined as follows: A: {At least More than 2 heads is observed} B: {The number of heads is odd} Use the knowledge of marginal, union, intersection and conditional probabilities to describe the prob
> The method of assigning probabilities based on the laws and rules pertaining to an experiment is called the __________. a) Experimental method b) Classical method c) Relative frequency of occurrence method d) Subjective method 6. If the occurrence of on
> If the sample variance of a dataset of size 8 is 2.57, what is the population variance if considering the same dataset to be a population? a) 2.57 b) 2.28 c) 1.60 d) 1.5 24. If the sample standard deviation of a dataset consisting of 9 observations is 8,
> When you use the data gathered from a sample to generate statistics to reach conclusions about the population from which the sample was taken, you are performing __________. a) Descriptive statistics b) A census c) Inferential statistics d) Constructiv
> A toy-manufacturing company has been given a large order for small plastic whistles that will be given away by a large fast-food hamburger chain with its kid’s meal. Seven random samples of four whistles have been taken. The weight of e
> A food-processing company makes potato chips, pretzels, and cheese chips. Although its products are packaged and sold by weight, the company has been taking sample bags of cheese chips and counting the number of chips in each bag. Shown here is the numbe
> A manufacturer produces digital watches. Every two hours a sample of six watches is selected randomly to be tested. Each watch is run for exactly 15 minutes and is timed by an accurate, precise timing device. Because of the variation among watches, they
> A manufacturing company produces cylindrical tubes for engines that are specified to be 1.20 centimeters thick. As part of the company’s statistical quality control effort, random samples of four tubes are taken each hour. The tubes are
> A fruit juice company sells a glass container filled with 24 ounces of cranapple juice. Inspectors are concerned about the consistency of volume of fill in these containers. Every two hours for three days of production, a sample of five containers is ran
> Examine the Minitab output shown here for a multiple regression analysis. How many predictors were there in this model? Comment on the overall significance of the regression model. Discuss the t ratios of the variables and their significance. Analysis o
> Jensen, Solberg, and Zorn investigated the relationship of insider ownership, debt, and dividend policies in companies. One of their findings was that firms with high insider ownership choose lower levels of both debt and dividends. Shown here is a sampl
> Is there a particular product that is an indicator of per capita personal consumption for countries around the world? Shown here are data on per capita personal consumption, paper consumption, fish consumption, and gasoline consumption for 11 countries.
> Use the following data to determine the equation of the multiple regression model. Comment on the regression coefficients. Predictor _________ Coefficient Constant …………………… 31,409.5 x1 …………………….………… .08425 x2 …………………….………… 289.62 x3 …………………….………… −.0947
> Minitab residual diagnostic output from the multiple regression analysis for the data given in Problem 13.30 follows. Refer to the Problem Data 13.30: Discuss any potential problems with meeting the regression assumptions for this regression analysis
> Shown here are the data for y and three predictors, x1, x2, and x3. A multiple regression analysis has been done on these data; the Minitab results are given. Comment on the outcome of the analysis in light of the data. Regression Analysis: Y versus X1,
> The American Chamber of Commerce Researchers Association compiles cost-of-living indexes for selected metropolitan areas. Shown here are cost-of-living indexes for 25 different cities on five different items for a recent year. Use the data to develop a r
> Using the following data, determine the equation of the regression model. How many independent variables are there? Comment on the meaning of these regression coefficients. Predictor _________ Coefficient Constant ………………….. 121.62 x1 ……………………………... −.174
> The U.S. Bureau of Labor Statistics produces consumer price indexes for several different categories. Shown here are the percentage changes in consumer price indexes over a period of 20 years for food, shelter, apparel, and fuel oil. Also displayed are t
> Investment analysts generally believe the interest rate on bonds is inversely related to the prime interest rate for loans; that is, bonds perform well when lending rates are down and perform poorly when interest rates are up. Can the bond rate be predic
> The Shipbuilders Council of America in Washington, D.C., publishes data about private shipyards. Among the variables reported by this organization are the employment figures (per 1000), the number of naval vessels under construction, and the number of re
> The U.S. Bureau of Mines produces data on the price of minerals. Shown here are the average prices per year for several minerals over a decade. Use these data and multiple regression to produce a model to predict the average price of gold from the other
> Given here are the data for a dependent variable, y, and independent variables. Use these data to develop a regression model to predict y. Discuss the output.
> Use the following data to develop a multiple regression model to predict y from x1 and x2. Discuss the output, including comments about the overall strength of the model, the significance of the regression coefficients, and other indicators of model fit.
> Study the Excel regression output that follows. How many predictors are there? What is the equation of the regression model? Using the key statistics discussed in this chapter, discuss the strength of the model and its predictors.
> Study the Minitab regression output that follows. How many predictors are there? What is the equation of the regression model? Using the key statistics discussed in this chapter, discuss the strength of the model and the predictors. Regression Analysis:
> Study the Minitab residual diagnostic output that follows. Discuss any potential problems with meeting the regression assumptions for this regression analysis based on the residual graphics.
> Study the Excel output shown in Problem 13.13. Comment on the overall strength of the regression model in light of S, R2, and adjusted R2. Refer in Problem Data 13.13:
> Use a computer to develop the equation of the regression model for the following data. Comment on the regression coefficients. Determine the predicted value of y for x1 = 33, x2 = 29, and x3 = 13.
> Using the regression output obtained by working Problem 13.12, comment on the overall strength of the regression model using S, R2, and adjusted R2.
> A corporation owns several companies. The strategic planner for the corporation believes dollars spent on advertising can to some extent be a predictor of total sales dollars. As an aid in long-term planning, she gathers the following sales and advertisi
> Using the regression output obtained by working Problem 13.11, comment on the overall strength of the regression model using S, R2, and adjusted R2.
> Using the regression output obtained by working Problem 13.6, comment on the overall strength of the regression model using S, R2, and adjusted R2.
> Using the regression output obtained by working Problem 13.5, comment on the overall strength of the regression model using S, R2, and adjusted R2.
> Study the Minitab output shown in Problem 13.8. Comment on the overall strength of the regression model in light of S, R2, and adjusted R2. Refer to the Problem Data 13.8: Analysis of Variance
> Study the Minitab output shown in Problem 13.7. Comment on the overall strength of the regression model in light of S, R2, and adjusted R2. Refer to the Problem Data 13.7: Analysis of Variance
> Study the following Excel multiple regression output. How many predictors are in this model? How many observations? What is the equation of the regression line? Discuss the strength of the model in terms of F. Which predictors, if any, are significant? W
> Use the following data to develop a regression model to predict y from x1 and x2. Comment on the output. Develop a regression model to predict y from x1 only. Compare the results of this model with those of the model using both predictors. What might you
> Develop a multiple regression model to predict y from x1, x2, and x3 using the following data. Discuss the values of F and t.
> Using the data from Problem 13.6, develop a multiple regression model to predict insider ownership from debt ratio and dividend payout. Comment on the strength of the model and the predictors by examining the ANOVA table and the t tests. Refer to the Pr
> Use a computer to develop the equation of the regression model for the following data. Comment on the regression coefficients. Determine the predicted value of y for x1 = 200 and x2 = 7.
> Sketch a scatter plot from the following data, and determine the equation of the regression line.
> Study the following Minitab residual diagnostic graphs. Comment on any possible violations of regression assumptions.
> Study the following Excel regression output for an analysis attempting to predict the number of union members in the United States by the size of the labor force for selected years over a 30-year period from data published by the U.S. Bureau of Labor Sta
> Study the following Minitab output from a regression analysis to predict y from x. Regression Analysis: Y Versus X a) What is the equation of the regression model? b) What is the meaning of the coefficient of x? c) What is the result of the test of the
> Can the consumption of water in a city be predicted by air temperature? The following data represent a sample of a day’s water consumption and the high temperature for that day. Water Use (millions of gallons) ________ Temperature (degrees Fahrenheit) 21
> Is the amount of money spent by companies on advertising a function of the total revenue of the company? Shown are revenue and advertising cost data for 10 companies published by Advertising Age. Use the data to develop a regression line to predict the
> The following data represent a breakdown of state banks and all savings organizations in the United States every 5 years over a 60-year span, according to the Federal Reserve System. Develop a regression model to predict the total number of state banks
> People in the aerospace industry believe the cost of a space project is a function of the weight of the major object being sent into space. Use the following data to develop a regression model to predict the cost of a space project by the weight of the s
> Shown below are data on the total sales generated by the seafood industry and the corresponding jobs supported by the seafood industry in the top 10 states by seafood sales. The data are published by the National Marine Fisheries Service of the National
> How strong is the correlation between the inflation rate and 30-year U.S. Treasury yields? The following data published by Fuji Securities are given as pairs of inflation rates and Treasury yields for selected years over a 35-year period. Inflation Rate
> Shown here are the labor force figures (in millions) published by the World Bank for the country of Bangladesh over a 10-year period. Develop the equation of a trend line through these data and use the equation to predict the labor force of Bangladesh fo
> Sketch a scatter plot from the following data, and determine the equation of the regression line.
> It seems logical that restaurant chains with more units (restaurants) would have greater sales. This assumption is mitigated, however, by several possibilities: Some units may be more profitable than others, some units may be larger, some units may serve
> The American Research Group, Inc., conducted a telephone survey of a random sample of 1100 U.S. adults in a recent year and determined that the average amount of planned spending on gifts for the holiday season was $854 and that 40% of the purchases woul
> A manager of a car dealership believes there is a relationship between the number of salespeople on duty and the number of cars sold. Suppose the following sample is used to develop a simple regression model to predict the number of cars sold by the numb
> Determine the equation of the trend line through the following cost data. Use the equation of the line to forecast cost for year 7. Year _______ Cost ($ millions) 1 ……………………… 56 2 ……………………… 54 3 ……………………… 49 4 ……………………… 46 5 ……………………… 45
> Determine the equation of the least squares regression line to predict y from the following data. a) Construct a 95% confidence interval to estimate the mean y value for x = 60. b) Construct a 95% prediction interval to estimate an individual y value fo
> If you were to develop a regression line to predict y by x, what value would the coefficient of determination have?
> Use the following data for parts a through g. a) Determine the equation of the simple regression line to predict y from x. b) Using the x values, solve for the predicted values of y and the residuals. c) Solve for SSE. d) Calculate the standard error of
> Use the following data for parts a through f. a) Determine the equation of the least squares regression line to predict y by x. b) Using the x values, solve for the predicted values of y and the residuals. c) Solve for se. d) Solve for r2. e) Test the s
> Determine the Pearson product-moment correlation coefficient for the following data.
> E-commerce sales in the United States have been growing for many years. Shown below are quarterly adjusted e-commerce sales figures ($ billions) released by the Census Bureau for the United States over a three-year period. Use these data to determine the
> The National Safety Council released the following data on the incidence rates for fatal or lost-worktime injuries per 100 employees for several industries in three recent years. Compute r for each pair of years and determine which years are most highly
> Shown below are rental and leasing revenue figures for office machinery and equipment in the United States over a seven-year period according to the U.S. Census Bureau. Use these data to construct a trend line and forecast the rental and leasing revenue
> Determine the equation of the trend line for the data shown below provided by the U.S. Census Bureau on U.S. exports of fertilizers to Indonesia over a five-year period. Using the trend line equation, forecast the value for the year 2020. Year _________
> Construct a 99% confidence interval for the average bond rate in Problem 12.9 for a prime interest rate of 10%. Discuss the meaning of this confidence interval.
> Construct a 98% confidence interval for the average value of y for Problem 12.8 using x = 20. Construct a 98% prediction interval for a single value of y for Problem 12.8 using x = 20. Which is wider? Why?
> Construct a 90% prediction interval for a single value of y for Problem 12.7 using x = 100. Construct a 90% prediction interval for a single value of y for Problem 12.7 using x = 130. Compare the results. Which prediction interval is greater? Why?

