Question: Use the box-and-whisker plot to
Use the box-and-whisker plot to identify the five-number summary.
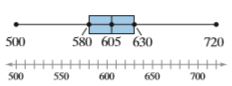
Transcribed Image Text:
500 580 605 630 720 to 700 500 550 600 650
> What is the difference between relative frequency and cumulative frequency?
> The table shows population statistics for the ages of Best Actor and Best Supporting Actor winners at the Academy Awards from 1929 to 2016. The distributions of the ages are approximately bell-shaped. Compare the z-scores for the actors. Best Actor 1982
> The table shows population statistics for the ages of Best Actor and Best Supporting Actor winners at the Academy Awards from 1929 to 2016. The distributions of the ages are approximately bell-shaped. Compare the z-scores for the actors. Best Actor 1970
> The table shows population statistics for the ages of Best Actor and Best Supporting Actor winners at the Academy Awards from 1929 to 2016. The distributions of the ages are approximately bell-shaped. Compare the z-scores for the actors. Best Actor 2005
> The table shows population statistics for the ages of Best Actor and Best Supporting Actor winners at the Academy Awards from 1929 to 2016. The distributions of the ages are approximately bell-shaped. Compare the z-scores for the actors. Best Actor 1984
> The life spans of a species of fruit fly have a bell-shaped distribution, with a mean of 33 days and a standard deviation of 4 days. a. The life spans of three randomly selected fruit flies are 34 days, 30 days, and 42 days. Find the z-score that corres
> Explain how to identify outliers using the interquartile range.
> A brand of automobile tire has a mean life span of 35,000 miles, with a standard deviation of 2250 miles. Assume the life spans of the tires have a bell-shaped distribution. a. The life spans of three randomly selected tires are 34,000 miles, 37,000 mil
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> Construct a frequency distribution and a relative frequency histogram for the data set using five classes. Which class has the greatest relative frequency and which has the least relative frequency? Fijian Banded Iguanas Data set: Lengths (in centime
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> The distribution of the ages of the winners of the Tour de France from 1903 to 2016 is approximately bell-shaped. The mean age is 27.9Â years, with a standard deviation of 3.3 years. Use the corresponding z-score to determine whether the age i
> The midpoints A, B, and C are marked on the histogram at the left. Match them with the indicated z-scores. Which z-scores, if any, would be considered unusual? z = 0.77, z = 1.54, z = -1.54 Physics Test Scores 16 14- 12 10 2 26 Score (out of 30) в с
> The midpoints A, B, and C are marked on the histogram at the left. Match them with the indicated z-scores. Which z-scores, if any, would be considered unusual? z = 0, z = 2.14, z = -1.43 Applied Statisties Test Scores 16 14 12 10 6- 4- 2 48 53 58 63
> Use the data set, which represents wait times (in minutes) for various services at a state’s Department of Motor Vehicles locations. Which wait times are between the 25th and 75th percentiles? 4. 10 13 14 17 4 27 4 8 4 5 3. 264
> A student’s IQ score is in the 91st percentile on the Weschler Adult Intelligence Scale. Make an observation about the student’s IQ score.
> Use the data set, which represents wait times (in minutes) for various services at a state’s Department of Motor Vehicles locations. Find the percentile that corresponds to a wait time of 20 minutes. 4. 10 13 14 17 4 27 4 8 4 5 3.
> Use the data set, which represents wait times (in minutes) for various services at a state’s Department of Motor Vehicles locations. Which wait time represents the 50th percentile? How would you interpret this? 4. 10 13 14 17 4 27
> Use the data set, which represents wait times (in minutes) for various services at a state’s Department of Motor Vehicles locations. Draw an ogive to show corresponding percentiles for the data. 4. 10 13 14 17 4 27 4 8 4 5 3. 264
> Construct a frequency distribution and a relative frequency histogram for the data set using five classes. Which class has the greatest relative frequency and which has the least relative frequency? Years of Service Data set: Years of service of 28 O
> Use the data set, which represents the ages of 30 executives. Which ages are below the 25th percentile? 43 57 65 47 57 41 56 53 61 54 56 50 66 56 50 61 47 40 50 43 54 41 48 45 28 35 38 43 42 44
> Use the data set, which represents the ages of 30 executives. Which ages are above the 75th percentile? 43 57 65 47 57 41 56 53 61 54 56 50 66 56 50 61 47 40 50 43 54 41 48 45 28 35 38 43 42 44
> Use the data set, which represents the ages of 30 executives. Find the percentile that corresponds to an age of 56 years old. 43 57 65 47 57 41 56 53 61 54 56 50 66 56 50 61 47 40 50 43 54 41 48 45 28 35 38 43 42 44
> Use the data set, which represents the ages of 30 executives. Find the percentile that corresponds to an age of 40 years old. 43 57 65 47 57 41 56 53 61 54 56 50 66 56 50 61 47 40 50 43 54 41 48 45 28 35 38 43 42 44
> Use the ogive, which represents the cumulative frequency distribution for quantitative reasoning scores on the Graduate Record Examination in a recent range of years. What percentile is a score of 170? How should you interpret this? Quantitative Rea
> Use the ogive, which represents the cumulative frequency distribution for quantitative reasoning scores on the Graduate Record Examination in a recent range of years. What percentile is a score of 140? How should you interpret this? Quantitative Rea
> Use the ogive, which represents the cumulative frequency distribution for quantitative reasoning scores on the Graduate Record Examination in a recent range of years. Which score represents the 40th percentile? How should you interpret this? Quantit
> A student’s score on the Fundamentals of Engineering exam is in the 89th percentile. Make an observation about the student’s exam score.
> Use the ogive, which represents the cumulative frequency distribution for quantitative reasoning scores on the Graduate Record Examination in a recent range of years. What score represents the 70th percentile? How should you interpret this? Quantita
> Refer to the data set in Exercise 26 and the box-and-whisker plot you drew that represents the data set. a. About 50% of the employees made less than what amount per hour? b. What percent of the employees made more than $23.39 per hour? c. What percen
> Construct a frequency distribution and a relative frequency histogram for the data set using five classes. Which class has the greatest relative frequency and which has the least relative frequency? Taste Test Data set: Ratings from 1 (lowest) to 10
> Refer to the data set in Exercise 23 and the box-and-whisker plot you drew that represents the data set. a. About 75% of the students studied no more than how many hours per day? b. What percent of the students studied more than 3 hours per day? c. Yo
> Use technology to draw a box-and-whisker plot that represents the data set. The hourly earnings (in dollars) of a sample of 21Â employees at a consulting firm 25.89 27.09 31.76 28.28 26.19 27.43 24.06 25.61 22.56 29.76 18.01 23.66 38.24 37
> Use technology to draw a box-and-whisker plot that represents the data set. The commuting distances (in miles) of a sample of 30 employees 7 6 7 5 2 1 1 2 3 8 9 19 12 8 15 24 3 3 11 17 45 4 4 26 10 4 21 1 5 12
> Use technology to draw a box-and-whisker plot that represents the data set. The numbers of vacation days used by a sample of 20 employees in a recent year 3 9 2 1 7 5 3 2 2 6 4 0 10 0 3 5 7 8 6 5
> Use technology to draw a box-and-whisker plot that represents the data set. The numbers of hours spent studying per day by a sample of 28 students 2 8 7 2 3 3 3 2 2 7 8 3 5 1 1 2 6 1 5 7 3 8 5 3 3 7 6 2
> Use the box-and-whisker plot to determine whether the shape of the distribution represented is symmetric, skewed left, skewed right, or none of these. Justify your answer. 100 200 300 400 500 600
> Use the box-and-whisker plot to determine whether the shape of the distribution represented is symmetric, skewed left, skewed right, or none of these. Justify your answer. 30 40 50 60 70 80 90 100 110
> Use the box-and-whisker plot to determine whether the shape of the distribution represented is symmetric, skewed left, skewed right, or none of these. Justify your answer. 20 30 40 50 60 70 80 90
> A motorcycle’s fuel efficiency represents the ninth decile of vehicles in its class. Make an observation about the motorcycle’s fuel efficiency.
> Use the box-and-whisker plot to determine whether the shape of the distribution represented is symmetric, skewed left, skewed right, or none of these. Justify your answer. 40 80 120 160 200
> Construct a frequency distribution and a frequency polygon for the data set using the indicated number of classes. Describe any patterns. Declaration of Independence Number of classes: 5 Data set: Numbers of children of those who signed the Declarati
> a. find the five-number summary, and b. draw a box-and-whisker plot that represents the data set. 2 7 1 3 1 2 8 9 9 2 5 4 7 3 7 5 4 2 3 5 9 5 6 3 9 3 4 9 8 8 2 3 9 5
> a. find the five-number summary, and b. draw a box-and-whisker plot that represents the data set. 4 7 7 5 2 9 7 6 8 5 8 4 1 5 2 8 7 6 6 9
> a. find the five-number summary, and b. draw a box-and-whisker plot that represents the data set. 171 176 182 150 178 180 173 170 174 178 181 180
> a. find the five-number summary, and b. draw a box-and-whisker plot that represents the data set. 39 36 30 27 26 24 28 35 39 60 50 41 35 32 51
> Use the box-and-whisker plot to identify the five-number summary. 8 10 01 2 3 4 5 6 7 8 9 10 11
> a. find the quartiles, b. find the interquartile range, and c. identify any outliers. 22 25 22 24 20 24 19 22 29 21 21 20 23 25 23 23 21 25 23 22
> a. find the quartiles, b. find the interquartile range, and c. identify any outliers. 56 63 51 60 57 60 60 54 63 59 80 63 60 62 65
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. It is impossible to have a z-score of 0.
> The length of a guest lecturer’s talk represents the third quartile for talks in a guest lecture series. Make an observation about the length of the talk.
> Construct a frequency distribution and a frequency polygon for the data set using the indicated number of classes. Describe any patterns. Ages of the Presidents Numbers of classes: 6 Data set: Ages of the U.S. presidents at Inauguration (Source: The
> Find the range of the data set represented by the graph. Median Annual Income by State 12 6- 3. 40 45 50 55 60 65 70 75 Income (in thousands of dollars) Frequency
> What must you know about a data set before you can use the Empirical Rule?
> Discuss the similarities and the differences between the Empirical Rule and Chebychev’s Theorem.
> The distances (in yards) for nine holes of a golf course are listed. a. Find the mean and the median of the data. b. Convert the distances to feet. Then rework part (a). c. Compare the measures you found in part (b) with those found in part (a). What d
> In an academic year, a student receives the grades shown below, with an A worth 4 points, a B worth 3 points, and a C worth 2 points. A in 2 four-credit classes and 3 three-credit classes B in 2 three-credit classes and 2 two-credit classes C in 1 two
> The letters A, B, and C are marked on the horizontal axis. Describe the shape of the data. Then determine which is the mean, which is the median, and which is the mode. Justify your answers. Hourly Wages of Employees 16 14 12 10- 6. 2 HTH 14 16 18 20
> Given a data set, how do you know whether to calculate σ or s?
> The letters A, B, and C are marked on the horizontal axis. Describe the shape of the data. Then determine which is the mean, which is the median, and which is the mode. Justify your answers. Sick Days Used by Employees 16 14 12- 10 4 2 10+ t f14 16 1
> The table at the left shows the U.S. trade deficits (in billions of dollars) with 18 countries in 2015. a. Find the mean and the median of the trade deficits. b. Find the mean and the median without the Chinese trade deficit. Which measure of central t
> During a quality assurance check, the actual contents (in grams) of six containers of protein powder were recorded as 1525, 1526, 1502, 1516, 1529, and 1511. a. Find the mean and the median of the contents. b. The third value was incorrectly measured a
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Finishing Times Number of classes: 8 Data set: Finishing times (in seconds) of 21 participants in a 10K race 3
> At least 99% of the data in any data set lie within how many standard deviations of the mean? Explain how you obtained your answer.
> The English statistician Karl Pearson (1857–1936) introduced a formula for the skewness of a distribution. Most distributions have an index of skewness between -3 and 3. When P > 0, the data are skewed right. When P a. x = 17, s = 2
> Sample annual salaries (in thousands of dollars) for employees at a company are listed. a. Find the sample mean and the sample standard deviation. b. Each employee in the sample receives a $1000 raise. Find the sample mean and the sample standard devia
> Sample annual salaries (in thousands of dollars) for employees at a company are listed. a. Find the sample mean and the sample standard deviation. b. Each employee in the sample receives a 5% raise. Find the sample mean and the sample standard deviatio
> Another useful measure of variation for a data set is the mean absolute deviation (MAD). It is calculated by the formula a. Find the mean absolute deviation of the data set in Exercise 15. Compare your result with the sample standard deviati
> Approximate the mean of the frequency distribution. The ages (in years) of the residents of a small town in 2016 Age (in years) 0-9 Frequency 78 10-19 97 20-29 54 30-39 63 40-49 69 50–59 86 60-69 73 70–79 53 80-89 43 90–99 15
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample grade point averages for ten male students and ten female students are listed. Males 2.4 3.7 3.8 3.9 2.8 2.6 3.6 3.3 4.0 1.9 Females 2.8 3.7 2.1 3.9 3.6
> Describe the difference between the calculation of population standard deviation and that of sample standard deviation.
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample SAT scores for eight males and eight females are listed. Males 1010 1170 1410 920 1320 1100 690 1140 Females 1190 1010 1000 1300 1470 1250 840 1060
> Find the coefficient of variation for each of the two data sets. Then compare the results. The ages (in years) and weight classes (in kilograms) of all members of the 2016 Men’s U.S. Olympic wrestling team are listed. Ages Weight Cl
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Reaction Times Number of classes: 8 Data set: Reaction times (in milliseconds) of 30 adult females to an audit
> Use the frequency distribution in Exercise 15 to construct an expanded frequency distribution, as shown in Example 2. From Exercise 15: Travel Time to Work (in minutes) Class Frequency,S 0-10 188 11-21 372 22-32 264 33-43 205 44-54 83 55-65 76 66-7
> Find the coefficient of variation for each of the two data sets. Then compare the results. The ages (in years) and heights (in inches) of all members of the 2016 Women’s U.S. Olympic swimming team are listed. Ages 24 24 19 23 22 21
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample annual salaries (in thousands of dollars) for entry level software engineers in Raleigh, NC, and Wichita, KS, are listed. Raleigh 63.7 68.4 59.3 50.7 59
> Find the coefficient of variation for each of the two data sets. Then compare the results. Sample annual salaries (in thousands of dollars) for entry level architects in Denver, CO, and Los Angeles, CA, are listed. Denver 45.8 46.4 44.4 40.7 51.5 39.
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The amounts of caffeine in a sample of five-ounce servings of brewed coffee are shown in the histogram. 25 2
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The numbers of courses taught per semester by a random sample of university professors are shown in the histogra
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The distribution of the numbers of hours that a random sample of college students study per week is shown in the
> Make a frequency distribution for the data. Then use the table to estimate the sample mean and the sample standard deviation of the data set. The distribution of the tuitions, fees, and room and board charges of a random sample of public 4-year degree-gr
> Make a frequency distribution for the data. Then use the table to find the sample mean and the sample standard deviation of the data set. 11 10 0 0 0 0 1 0 1 0 0 1 0 1 1 0 0 0 0 1 0 0 1 1 10 0 11 0 0 0 0 0 1 0 1 1 1 0 0 0 0 1 1 0 0 0
> Explain the relationship between variance and standard deviation. Can either of these measures be negative? Explain.
> Make a frequency distribution for the data. Then use the table to find the sample mean and the sample standard deviation of the data set. 3 3 5 3 8 0 39 6 6 7 1 6 3 2 6 9 1 8 5 0 2 3 4 9 5 8 19 7 6 9 6 706 3 8 6 8 7 3 8 9 3 7 2 4 4 1
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Pepper Pungencies Number of classes: 5 Data set: Pungencies (in thousands of Scoville units) of 24 tabasco sCO
> The mean number of runs per game scored by the Chicago Cubs during the 2016 World Series was 3.86 runs, with a standard deviation of 3.36 runs. Apply Chebychev’s Theorem to the data using k = 2. Interpret the results.
> The mean score on a Statistics exam is 82 points, with a standard deviation of 3 points. Apply Chebychev’s Theorem to the data using k = 4. Interpret the results.
> Old Faithful is a famous geyser at Yellowstone National Park. From a sample with n = 100, the mean interval between Old Faithful’s eruptions is 101.56 minutes and the standard deviation is 42.69 minutes. Using Chebychev’s Theorem, determine at least how
> You are conducting a survey on the number of pets per household in your region. From a sample with n = 40, the mean number of pets per household is 2 pets and the standard deviation is 1 pet. Using Chebychev’s Theorem, determine at least how many of the
> Use the Empirical Rule. The monthly utility bills for eight households are listed. Using the sample statistics from Exercise 30, determine which of the data entries are unusual. Are any of the data entries very unusual? Explain your reasoning. $65, $52,
> Use the Empirical Rule. The speeds for eight vehicles are listed. Using the sample statistics from Exercise 29, determine which of the data entries are unusual. Are any of the data entries very unusual? Explain your reasoning. 70, 78, 62, 71, 65, 76, 82,
> Use the Empirical Rule. Use the sample statistics from Exercise 30 and assume the number of households in the sample is 40. a. Estimate the number of households whose monthly utility bills are between $54 and $86. b. In a sample of 20 additional househ
> Use the Empirical Rule. Use the sample statistics from Exercise 29 and assume the number of vehicles in the sample is 75. a. Estimate the number of vehicles whose speeds are between 63 miles per hour and 71 miles per hour. b. In a sample of 25 additiona
> Use the Empirical Rule. The mean monthly utility bill for a sample of households in a city is $70, with a standard deviation of $8. Between what two values do about 95% of the data lie? (Assume the data set has a bell-shaped distribution.)
> Why is the standard deviation used more frequently than the variance?
> Construct a frequency distribution and a frequency histogram for the data set using the indicated number of classes. Describe any patterns. Sales Number of classes: 6 Data set: July sales (in dollars) for 21 sales representatives at a company 2114 24

