Question: Use the data in Try It Yourself
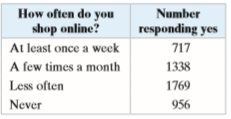
Use the data in Try It Yourself 1 to construct a 90% confidence interval for the population proportion of U.S. adults who shop online at least once a week.
From Try It Yourself 1:
Transcribed Image Text:
How often do you shop online? At least once a week Number responding yes 717 A few times a month 1338 Less often 1769 Never 956
> Exercise 12.111 described a study to determine whether viewers (older than 50) of the network news had contacted their physician to ask about one of the prescription drugs advertised during the newscast. The responses (1 = No, 2 = Yes) were recorded. Als
> Refer to Exercise 12.110. Determine whether there is enough evidence to infer thatthere are differences in the choice of Christmas tree between the three age categories. Data from Exercise 12.110: An important decision faces Christmas holiday celebrator
> Pat Statsdud is about to write a multiple- choice exam but as usual knows absolutely nothing. Pat plans to guess one of the five choices. Pat has been given one of the professor’s previous exams with the correct answers marked. The correct choices were r
> Repeat Exercise 15.1 with the following frequencies: Cell 1 2 3 4 5 Frequency 6 16 21 18 14 Data from Exercise 15.1: Consider a multinomial experiment involving n = 300 trials and k = 5 cells. The observed frequencies resulting from the e
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(72 < x < 82)
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(60 < x < 70)
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(x > 71.6)
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(x > 80)
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(x < 55)
> The random variable x is normally distributed with mean µ = 74 and standard deviation σ = 8. Find the indicated probability. P(x < 84)
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(z < 0 or z > 1.68)
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(z < -2.50 or z > 2.50)
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(0.42 < z < 3.15)
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(-2.15 < z < 1.55)
> Find the expected net gain to the player for one play of the game. It costs $25 to bet on a horse race. The horse has a 1/8 chance of winning and a 1/4 chance of placing 2nd or 3rd. You win $125 if the horse wins and receive your money back if the horse
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(z > -0.74)
> Find the indicated probability using the standard normal distribution. If convenient, use technology to find the probability. P(z < 1.28)
> The scores for the reading portion of the ACT test are normally distributed. In a recent year, the mean test score was 21.3 and the standard deviation was 6.5. The test scores of four students selected at random are 17, 29, 8, and 23. Determine whether a
> The scores for the reading portion of the ACT test are normally distributed. In a recent year, the mean test score was 21.3 and the standard deviation was 6.5. The test scores of four students selected at random are 17, 29, 8, and 23. Find the z-score th
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the left of z = 0.64 and to the right of z = 3.415
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the left of z = -1.5 and to the right of z = 1.5
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. Between z = -2.68 and z = 2.68
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. Between z = 0.05 and z = 1.71
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. Between z = -1.55 and z = 1.04
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. Between z = -1.64 and z = 0
> a. find the mean, variance, and standard deviation of the probability distribution, and b. interpret the results. A television station sells advertising in 15-, 30-, 60-, 90-, and 120-second blocks. The distribution of sales for
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the right of z = 0.015
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the left of z = -2.825
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the right of z = 3.22
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the right of z = -0.57
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the left of z = -1.95
> Find the indicated area under the standard normal curve. If convenient, use technology to find the area. To the left of z = 0.33
> Five adults who participated in the survey in Example 5 are randomly selected and asked whether they use the social media platform Instagram. Construct a binomial distribution for the number of adults who respond yes.
> A card is selected from a standard deck and replaced. This experiment is repeated a total of five times. Find the probability of selecting exactly three clubs.
> Find the area of the indicated region under the standard normal curve. If convenient, use technology to find the area. -2.35 -0.8 0
> Determine whether the experiment is a binomial experiment. If it is, specify the values of n, p, and q, and list the possible values of the random variable x. If it is not, explain why. You take a multiple-choice quiz that consists of 10 questions. Each
> In San Francisco, California, about 44% of the days in a year are clear. Find the mean, variance, and standard deviation for the number of clear days during the month of May. Interpret the results and determine any unusual events.
> At a raffle, 2000 tickets are sold at $5 each for five prizes of $2000, $1000, $500, $250, and $100. You buy one ticket. Find the expected value and interpret its meaning.
> Find the variance and standard deviation of the probability distribution constructed in Try It Yourself 2.
> Find the mean of the probability distribution you constructed in Try It Yourself 2. What can you conclude?
> Determine whether each distribution is a probability distribution. Explain your reasoning. 1. 2. 5 6 7 8 1 2 3 4. P(x) P(x) 0.09 0.36 0.49 0.10 16 4
> Verify that the distribution you constructed in Try It Yourself 2 is a probability distribution. From Try It Yourself 2: P(x) 0.16 0.19 0.15 16 1 19 2 15 3 21 0.21 4 9 0.09 5 10 0.10 8 0.08 2 n = 100 7 0.02 EP(x) =1
> A company tracks the number of sales new employees make each day during a 100-day probationary period. The results for one new employee are shown at the left. Construct a probability distribution for the random variable x. Then graph the distribution usi
> Determine whether each random variable x is discrete or continuous. Explain your reasoning. 1. Let x represent the speed of a rocket. 2. Let x represent the number of calves born on a farm in one year. 3. Let x represent the number of days of rain for
> Construct the 90% and 95% confidence intervals for the population variance and standard deviation of the medicine weights.
> Find the area of the indicated region under the standard normal curve. If convenient, use technology to find the area. 0 0.46
> Find the critical values x2R and x2L for a 90% confidence interval when the sample size is 30.
> A researcher is estimating the population proportion of people in the United States who delayed seeking medical care during the last 12 months due to costs. The estimate must be accurate within 2% of the population proportion with 90% confidence. Find th
> Use the data in Example 3 to construct a 99% confidence interval for the population proportion of 18- to 29-year-olds who get their news online. From Example 3: NEWS Percent of 18- to 29-year-olds who get news on each platform Online 50% Television
> A poll surveyed 4780 U.S. adults about how often they shop online. The results are shown in the table. Find a point estimate for the population proportion of U.S. adults who shop online at least once a week. How often do you shop online? At least onc
> You randomly select 18 adult male athletes and measure the resting heart rate of each. The sample mean heart rate is 64 beats per minute, with a sample standard deviation of 2.5 beats per minute. Assuming the heart rates are normally distributed, should
> Construct 90% and 95% confidence intervals for the population mean number of days the car model sits on the dealership’s lot in Example 3. Compare the widths of the confidence intervals.
> Construct 90% and 99% confidence intervals for the population mean temperature of coffee sold in Example 2.
> Find the critical value tc for a 90% confidence level when the sample size is 22.
> In Example 6, how many student-athletes must the researcher include in the sample to be 95% confident that the sample mean is within 0.75 hour of the population mean? Compare your answer with Example 6.
> Use the normal curves shown. Which normal curve has the greatest standard deviation? Explain your reasoning. B 80 90 100 110 120 130 140
> Construct a 90% confidence interval for the population mean age for the college students in Example 5 with the sample size increased to 30 students. Compare your answer with Example 5.
> Use the data in Example 1 and technology to construct 75%, 85%, and 90% confidence intervals for the mean number of hours spent on required athletic activities by all student-athletes in the conference. How does the width of the confidence interval chang
> Use the data in Try It Yourself 1 to construct a 95% confidence interval for the mean number of hours spent on required athletic activities by all student-athletes in the conference. Compare your result with the interval found in Example 3. From Try It
> Use the data in Try It Yourself 1 and a 95% confidence level to find the margin of error for the mean number of hours spent on required athletic activities by all student-athletes in the conference. Assume the population standard deviation is 2.3 hours.
> In Example 1, the researcher selects a second random sample of 30 student-athletes and records their numbers of hours spent on required athletic activities (see table at left). Use this sample to find another point estimate of the population mean m.
> The study in Example 5 found that 32.0% of all men in the United States ages 50 and older have arthritis. You randomly select 75 men in the United States who are at least 50 years old and ask them whether they have arthritis. What is the probability that
> In Example 4, what is the probability that at most 80 drivers will say yes, they have yelled at another driver?
> In a survey of adults in the United States, 29% said they have seen a person using a mobile device walk in front of a moving vehicle without looking. You randomly select 100 adults in the United States and ask them whether they have seen a person using a
> Use a continuity correction to convert each binomial probability to a normal distribution probability. 1. The probability of getting between 57 and 83 successes, inclusive 2. The probability of getting at most 54 successes
> A binomial experiment is listed. Determine whether you can use a normal distribution to approximate the distribution of x, the number of people who reply yes. If you can, find the mean and standard deviation. If you cannot, explain why. In a survey of a
> Determine whether you can use a normal distribution to approximate the binomial distribution. If you can, use the normal distribution to approximate the indicated probabilities and sketch their graphs. If you cannot, explain why and use a binomial distri
> Which event(s) in Exercise 6 can be considered unusual? Explain your reasoning. From Exercise 6: Basketball player Dwight Howard makes a free throw shot about 56% of the time. Find the probability that a. the first free throw shot he makes is the fourt
> A consumer price analyst claims that prices for liquid crystal display (LCD) computer monitors are normally distributed, with a mean of $190 and a standard deviation of $48. What is the probability that a randomly selected LCD computer monitor costs less
> The average sales price of a single-family house in the United States is $235,500. You randomly select 12 single-family houses. What is the probability that the mean sales price is more than $225,000? Assume that the sales prices are normally distributed
> You randomly select 100 drivers ages 16 to 19 from Example 4. What is the probability that the mean distance traveled each day is between 19.4 and 22.5 miles? Use µ = 20.7 miles and σ = 6.5 miles.
> The diameters of fully grown white oak trees are normally distributed, with a mean of 3.5 feet and a standard deviation of 0.2 foot, as shown in the figure. Random samples of size 16 are drawn from this population, and the mean of each sample is determin
> Random samples of size 64 are drawn from the population in Example 2. Find the mean and standard deviation of the sampling distribution of sample means. Then sketch a graph of the sampling distribution and compare it with the sampling distribution in Exa
> List all possible samples of size n = 3, with replacement, from the population {1, 3, 5}. Calculate the mean of each sample. Find the mean, variance, and standard deviation of the sample means. Compare your results with the mean µ = 3, variance σ2 = 8 3,
> The lengths of time employees have worked at a corporation are normally distributed, with a mean of 11.2 years and a standard deviation of 2.1 years. In a company cutback, the lowest 10% in seniority are laid off. What is the maximum length of time an em
> A researcher tests the braking distances of several cars. The braking distance from 60 miles per hour to a complete stop on dry pavement is measured in feet. The braking distances of a sample of cars are normally distributed, with a mean of 129 feet and
> A veterinarian records the weights of dogs treated at a clinic. The weights are normally distributed, with a mean of 52 pounds and a standard deviation of 15 pounds. Find the weight x corresponding to each z-score. Interpret the results. 1. z = -2.33
> Find the z-score that corresponds to each percentile. 1. P10 2. P20 3. P99
> Determine whether you can use a normal distribution to approximate the binomial distribution. If you can, use the normal distribution to approximate the indicated probabilities and sketch their graphs. If you cannot, explain why and use a binomial distri
> 1. Find the z-score that has 96.16% of the distribution’s area to its right. 2. Find the positive z-score for which 95% of the distribution’s area lies between -z and z.
> A U.S. adult who is at least 20 years old is selected at random. What is the probability that the person’s triglyceride level is between 100 and 150? Use technology to find the probability.
> What is the probability that the shopper in Example 2 will be in the supermarket between 31 and 58 minutes? When 200 shoppers enter the store, how many shoppers would you expect to be in the store between 31 and 58 minutes?
> The average speed of vehicles traveling on a stretch of highway is 67 miles per hour with a standard deviation of 3.5 miles per hour. A vehicle is selected at random. What is the probability that it is violating the speed limit of 70 miles per hour? Assu
> Find the area under the standard normal curve between z = -2.165 and z = -1.35.
> Find the area under the standard normal curve to the right of z = -2.16.
> Find the area under the standard normal curve to the left of z = 2.13.
> 1. Find the cumulative area that corresponds to a z-score of -2.19. 2. Find the cumulative area that corresponds to a z-score of 2.17.
> The scaled test scores for the New York State Grade 4 Common Core English Language Arts Test are normally distributed. The normal curve shown below represents this distribution. What is the mean test score? Estimate the standard deviation of this normal
> 1. Which normal curve has the greatest mean? 2. Which normal curve has the greatest standard deviation? B. 30 40 50 60 70
> Write the binomial probability in words. Then, use a continuity correction to convert the binomial probability to a normal distribution probability. P(54 < x < 64)
> Two thousand brown trout are introduced into a small lake. The lake has a volume of 20,000 cubic meters. Use a table to find the probability that three brown trout are found in any given cubic meter of the lake.
> What is the probability that more than four accidents will occur in any given month at the intersection?
> The study in Example 1 found that the smartphones made by a second manufacturer had a failure rate of 14%. Six smartphones made by this manufacturer are selected at random. Find the probability that the sixth smartphone is the first one to have a failure
> A recent study found that 28% of U.S. adults read an ebook in the last 12 months. You randomly select 4 adults and ask them whether they read an ebook in the last 12 months. Construct a probability distribution for the random variable x. Then graph the d
> About 5% of workers (ages 16 years and older) in the United States commute to their jobs by using public transportation (excluding taxicabs). You randomly select six workers. What is the probability that exactly two of them use public transportation to g
> The survey in Example 5 found that 27% of U.S. adults say that CNN is a major source of news for them. You randomly select five adults and ask them whether CNN is a major source of news for them. Find the probability that 1. exactly two of them respond
> A survey found that 52% of U.S. adults associate professional football with negative moral values. You randomly select 150 adults. What is the probability that exactly 65 adults associate professional football with negative moral values? Use technology t
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. The mean of the random variable of a probability distribution describes how the outcomes vary.
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. For a random variable x, the word random indicates that the value of x is determined by chance.
> Determine whether the statement is true or false. If it is false, rewrite it as a true statement. In most applications, continuous random variables represent counted data, while discrete random variables represent measured data.

