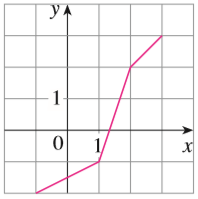
Question: Use the given graph off to sketch
> Graph the given functions on the same screen. How are these graphs related? y = tan x, -π/ 2 < x < π/ 2; y = tan-1x; y = x
> Graph the given functions on the same screen. How are these graphs related? y = sin x, -π/2 ≤ x ≤ π/2; y = sin-1x; y = x
> Simplify the expression. sin(2arccosx)
> Simplify the expression. sin(tan-1x)
> Simplify the expression. tan(sin-1x)
> Prove that cos(sin-1 x) = 1 − x2 .
> Find the exact value of each expression. a. arcsin(sin(5π /4)) b. cos(2 sin-1 (5/13))
> Evaluate the difference quotient for the given function. Simplify your answer. f(a + h) – f(a) f(x) = x', h
> Find the exact value of each expression. a. cot-1(-√3 ) b. sec-1
> Find the exact value of each expression. a. sin-1(-1/√2 ) b. cos-1(√3/2)
> Find the exact value of each expression. a. csc-1 √2 b. arcsin 1
> Find the exact value of each expression. a. tan-1 3 b. arctan(-1)
> Find the exact value of each expression. a. cos-1(-1) b. sin-1(0.5)
> When a camera flash goes off, the batteries immediately begin to recharge the flash’s capacitor, which stores electric charge given by Q(t) = Q0(1 – e-t/a) (The maximum charge capacity is Q0 and t is measured in seconds.) a. Find the inverse of this fun
> If a bacteria population starts with 100 bacteria and doubles every three hours, then the number of bacteria after t hours is n = f(t) = 100 ∙ 2t/3. a. Find the inverse of this function and explain its meaning. b. When will the population reach 50,000
> a. If g(x) = x6 + x4, x ≥ 0, use a computer algebra system to find an expression for g-1(x). b. Use the expression in part (a) to graph y = t(x), y = x, and y = g-1(x) on the same screen.
> Graph the function f(x) = x3 + x2 + x + 1 and explain why it is one-to-one. Then use a computer algebra system to find an explicit expression for f -1(x). (Your CAS will produce three possible expressions. Explain why two of them are irrelevant in this
> a. What are the values of eln 300 and ln(e300)? b. Use your calculator to evaluate eln 300 and ln(e300). What do you notice? Can you explain why the calculator has trouble?
> Evaluate the difference quotient for the given function. Simplify your answer. f(3 + h) – f(3) f(x) = 4 + 3x – x², h
> a. Find the domain of f(x) = ln(ex – 3). b. Find f -1 and its domain.
> Solve each inequality for x. a. 1 < e3x-1 < 2 b. 1 - 2 ln x < 3
> Solve each inequality for x. a. ln x < 0 b. ex > 5
> Solve each equation for x. a. ln(ln x) = 1 b. eax = Cebx, where a ≠ b
> Solve each equation for x. a. 2x-5 = 3 b. ln x + ln(x – 1) − 1
> Solve each equation for x. a. ln(x2 – 1) − 3 b. e2x - 3ex + 2 = 0
> Solve each equation for x. a. e7-4x = 6 b. ln(3x - 10) = 2
> a. What are the domain and range off? b. What is the x-intercept of the graph off? c. Sketch the graph off. f(x) = ln(x – 1) - 1
> a. What are the domain and range off? b. What is the x-intercept of the graph off? c. Sketch the graph off. f(x) = ln x + 2
> Make a rough sketch of the graph of each function. Do not use a calculator. Just use the graphs given in Figures 12 and 13 and, if necessary, the transformations of Section 1.3. a. y = ln (-x) b. y = ln|x| From Figures 12 and 13 y= log, x y= log
> A spherical balloon with radius r inches has volume V(r) = 4/3 πr3. Find a function that represents the amount of air required to inflate the balloon from a radius of r inches to a radius of r + 1 inches.
> Make a rough sketch of the graph of each function. Do not use a calculator. Just use the graphs given in Figures 12 and 13 and, if necessary, the transformations of Section 1.3. a. y = log10(x + 5) b. y = -ln x From Figures 12 and 13 y= log, x y
> Compare the functions f(x) = x0.1 and g(x) = ln x by graphing both f and g in several viewing rectangles. When does the graph off finally surpass the graph of g?
> Suppose that the graph of y = log2x is drawn on a coordinate grid where the unit of measurement is an inch. How many miles to the right of the origin do we have to move before the height of the curve reaches 3 ft?
> Use Formula 10 to graph the given functions on a common screen. How are these graphs related? y = ln x, y = log10x, y = ex, y = 10x
> Use Formula 10 to graph the given functions on a common screen. How are these graphs related? y = log1.5x, y = ln x, y = log10x, y = log50x
> Use Formula 10 to evaluate each logarithm correct to six decimal places. a. log510 b. log357
> Express the given quantity as a single logarithm. 1/3 ln(x + 2)3 + ½[ ln x – ln (x2 +3x + 2)2]
> Express the given quantity as a single logarithm. ln b + 2 ln c – 3 ln d
> Express the given quantity as a single logarithm. ln 10 + 2 ln 5
> Find the exact value of each expression. a. e-ln2 b. e ln(ln e 3)
> If f(x) = 3x2 - x + 2, find f(2), f(-2), f(a), f(-a), f(a + 1), 2f(a), f(2a), f(a2), [ f(a)]2, and f(a + h).
> Find the exact value of each expression. a. log1040 + log102.5 b. log860 - log83 - log85
> Find the exact value of each expression. a. log5 1/125 b. ln(1/e2)
> Find the exact value of each expression. a. log232 b. log82
> a. What is the natural logarithm? b. What is the common logarithm? c. Sketch the graphs of the natural logarithm function and the natural exponential function with a common set of axes.
> a. How is the logarithmic function y = logbx defined? b. What is the domain of this function? c. What is the range of this function? d. Sketch the general shape of the graph of the function y = logbx if b > 1.
> Let g(x) = 3 1 − x3 . a. Find g-1. How is it related to g? b. Graph g. How do you explain your answer to part (a)?
> Let f(x) = 1 − x2 , 0 ≤ x ≤ 1. a. Find f -1. How is it related to f ? b. Identify the graph of f and explain your answer to part (a).
> Use the given graph off to sketch the graph off -1. 1 2
> Find an explicit formula for f -1 and use it to graph f -1, f, and the line y = x on the same screen. To check your work, see whether the graphs of f and f -1 are reflections about the line. f(x) = 1 + e-x
> Researchers measured the blood alcohol concentration (BAC) of eight adult male subjects after rapid consumption of 30 mL of ethanol (corresponding to two standard alcoholic drinks). The table shows the data they obtained by averaging the BAC (in mg/mL) o
> Find an explicit formula for f -1 and use it to graph f -1, f, and the line y = x on the same screen. To check your work, see whether the graphs of f and f -1 are reflections about the line. f(x) = 4x + 3
> Find a formula for the inverse of the function. y = 1 – e-x / 1 + e-x
> Find a formula for the inverse of the function. y = ln(x + 3)
> Find a formula for the inverse of the function. y = x2 - x, x ≥ 1/2
> Find a formula for the inverse of the function. f(x) = e2x-1
> Find a formula for the inverse of the function. f(x) = 4x – 1/2x + 3
> Find a formula for the inverse of the function. f(x) = 1 + 2 + 3x
> In the theory of relativity, the mass of a particle with speed is where m0 is the rest mass of the particle and c is the speed of light in a vacuum. Find the inverse function of f and explain its meaning. mo m = f(v) V1 – v²/c²
> The formula C = 5/9(F – 32), where F ≥ -459.67, expresses the Celsius temperature C as a function of the Fahrenheit temperature F. Find a formula for the inverse function and interpret it. What is the domain of the inverse function?
> The graph off is given. a. Why is f one-to-one? b. What are the domain and range of f -1? c. What is the value of f -1(2)? d. Estimate the value of f -1(0). 1 1
> Temperature readings T (in °F) were recorded every two hours from midnight to 2:00 pm in Atlanta on June 4, 2013. The time t was measured in hours from midnight. a. Use the readings to sketch a rough graph of T as a function of t. b. Use yo
> If g(x) = 3 + x + ex, find g-1(4).
> If f(x) = x5 + x3 + x, find f -1(3) and f(f -1(2)).
> Assume that f is a one-to-one function. a. If f(6) = 17, what is f -1(17)? b. If f -1(3) = 2, what is f(2)?
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. f(t) is your height at age t.
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. f(t) is the height of a football t seconds after kickoff.
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. g(x) = 3
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. g(x) = 1 - sin x
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. f(x) = x4 - 16
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. f(x) = 2x - 3
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one.
> An airplane takes off from an airport and lands an hour later at another airport, 400 miles away. If t represents the time in minutes since the plane has left the terminal building, let x(t) be the horizontal distance traveled and y(t) be the altitude of
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one.
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one. у.
> A function is given by a table of values, a graph, a formula, or a verbal description. Determine whether it is one-to-one.
> Use the Law of Exponents to rewrite and simplify the expression. a. x2n . x3n-1 /xn+2 b. √a
> Use the Law of Exponents to rewrite and simplify the expression. a. b8(2b)4 b. (6y3)4/ 2y5
> Use the Law of Exponents to rewrite and simplify the expression. a. 84/3 b. x(3x2)3
> Use the Law of Exponents to rewrite and simplify the expression. a. 4-3 /2-8 b. 1 / 3 x4
> Graph several members of the family of functions f(x) = 1/ 1 + aebx where a > 0. How does the graph change when b changes? How does it change when a changes?
> If you graph the function you’ll see that f appears to be an odd function. Prove it. 1- e 1/1 f(x) 1+ e ,1/1
> The table gives the population of the United States, in millions, for the years 1900–2010. Use a graphing calculator with exponential regression capability to model the US population since 1900. Use the model to estimate the population
> A homeowner mows the lawn every Wednesday afternoon. Sketch a rough graph of the height of the grass as a function of time over the course of a four-week period.
> Use a graphing calculator with exponential regression capability to model the population of the world with the data from 1950 to 2010 in Table 1 on page 49. Use the model to estimate the population in 1993 and to predict the population in the year 2020.
> After alcohol is fully absorbed into the body, it is metabolized with a half-life of about 1.5 hours. Suppose you have had three alcoholic drinks and an hour later, at midnight, your blood alcohol concentration (BAC) is 0.6 mg/mL. a. Find an exponentia
> Use the graph of V in Figure 11 to estimate the half-life of the viral load of patient 303 during the first month of treatment. From Figure 11: 60 40+ 20 i (days) 10 20 30 RNA copies / mL
> An isotope of sodium, 24Na, has a half-life of 15 hours. A sample of this isotope has mass 2 g. a. Find the amount remaining after 60 hours. b. Find the amount remaining after t hours. c. Estimate the amount remaining after 4 days. d. Use a graph to
> The half-life of bismuth-210, 210Bi, is 5 days. a. If a sample has a mass of 200 mg, find the amount remaining after 15 days. b. Find the amount remaining after t days. c. Estimate the amount remaining after 3 weeks. d. Use a graph to estimate th
> A bacteria culture starts with 500 bacteria and doubles in size every half hour. a. How many bacteria are there after 3 hours? b. How many bacteria are there after t hours? c. How many bacteria are there after 40 minutes? d. Graph the population func
> A researcher is trying to determine the doubling time for a population of the bacterium Giardia lamblia. He starts a culture in a nutrient solution and estimates the bacteria count every four hours. His data are shown in the table. a. Make a scatter pl
> Use a graph to estimate the values of x such that ex > 1,000,000,000.
> Compare the functions f(x) = x10 and g(x) = ex by graphing both f and g in several viewing rectangles. When does the graph of g finally surpass the graph of f ?
> Compare the functions f(x) = x5 and g(x) = 5x by graphing both functions in several viewing rectangles. Find all points of intersection of the graphs correct to one decimal place. Which function grows more rapidly when x is large?
> You place a frozen pie in an oven and bake it for an hour. Then you take it out and let it cool before eating it. Describe how the temperature of the pie changes as time passes. Then sketch a rough graph of the temperature of the pie as a function of tim
> If is it true that f = g? x² – x S(x)· X - 1 х) — х and
> Suppose the graphs of f(x) = x2 and g(x) = 2x are drawn on a coordinate grid where the unit of measurement is 1 inch. Show that, at a distance 2 ft to the right of the origin, the height of the graph off is 48 ft but the height of the graph of t is about
> Suppose you are offered a job that lasts one month. Which of the following methods of payment do you prefer? I. One million dollars at the end of the month. II. One cent on the first day of the month, two cents on the second day, four cents on the thir
> If f(x) = 5x, show that f(x + h) – f(x) 5* – 1 5* h h
> Find the exponential function f(x) = Cbx whose graph is given. y. (-1, 3) jen