Question: even though the limit can be found
even though the limit can be found using algebraic simplification as in Section 9.1, use L’Hôpital’s rule to find the limit.
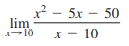
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y =(x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y =(x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y =(x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y =(x).
> use the given information to sketch the graph of . Assume that  is continuous on its domain and that all intercepts are included in the table of values
> use the given information to sketch the graph of . Assume that  is continuous on its domain and that all intercepts are included in the table of values
> use the given information to sketch the graph of . Assume that  is continuous on its domain and that all intercepts are included in the table of values
> Several experiments are simulated using the random number feature on a graphing calculator. For example, the roll of a fair die can be simulated by selecting a random integer from 1 to 6, and 50 rolls of a fair die by selecting 50 random integers from 1
> use the given information to sketch the graph of . Assume that  is continuous on its domain and that all intercepts are included in the table of values
> use the given information to sketch a possible graph of .
> use the given information to sketch a possible graph of .
> Repeat Problem 9 for the following graph of  (assume that  ″(d) Data from Problem 9: (A) the intervals on which ′(x) (B) the intervals on which ï‚&
> even though the limit can be found using algebraic simplification as in Section 9.1, use L’Hôpital’s rule to find the limit.
> even though the limit can be found using algebraic simplification as in Section 9.1, use L’Hôpital’s rule to find the limit.
> round each expression to the nearest integer without using a calculator.
> round each expression to the nearest integer without using a calculator.
> round each expression to the nearest integer without using a calculator.
> round each expression to the nearest integer without using a calculator.
> Refer to the data in the following table, obtained from a random survey of 1,000 residents of a state. The participants were asked their political affiliations and their preferences in an upcoming election. (In the table, D = Democrat, R = Republican, an
> Find all horizontal asymptotes for each function
> Find all horizontal asymptotes for each function
> n is a positive integer. Find each limit.
> n is a positive integer. Find each limit.
> Find limx→0+ (√x ln x). [Hint: Write (√x ln x = (ln x) /x-1/2 .]
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Several experiments are simulated using the random number feature on a graphing calculator. For example, the roll of a fair die can be simulated by selecting a random integer from 1 to 6, and 50 rolls of a fair die by selecting 50 random integers from 1
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> Note that L’Hôpital’s rule does not apply to every problem, and some problems will require more than one application of L’Hôpital’s rule.
> explain why L’Hôpital’s rule does not apply. If the limit exists, find it by other means.
> explain why L’Hôpital’s rule does not apply. If the limit exists, find it by other means.
> Use L’Hôpital’s rule to find the limit. Note that in these problems, neither algebraic simplification nor Theorem 4 of Section 9.2 provides an alternative to L’Hôpital&acir
> To test a new car, an automobile manufacturer wants to select 4 employees to test-drive the car for 1 year. If 12 management and 8 union employees volunteer to be test drivers and the selection is made at random, what is the probability that at least 1 u
> Use L’Hôpital’s rule to find the limit. Note that in these problems, neither algebraic simplification nor Theorem 4 of Section 9.2 provides an alternative to L’Hôpital&acir
> Use L’Hôpital’s rule to find the limit. Note that in these problems, neither algebraic simplification nor Theorem 4 of Section 9.2 provides an alternative to L’Hôpital&acir
> Use L’Hôpital’s rule to find the limit. Note that in these problems, neither algebraic simplification nor Theorem 4 of Section 9.2 provides an alternative to L’Hôpital&acir
> even though the limit can be found using Theorem 4 of Section 9.2, use L’Hôpital’s rule to find the limit.
> even though the limit can be found using Theorem 4 of Section 9.2, use L’Hôpital’s rule to find the limit.
> even though the limit can be found using Theorem 4 of Section 9.2, use L’Hôpital’s rule to find the limit.
> even though the limit can be found using Theorem 4 of Section 9.2, use L’Hôpital’s rule to find the limit.
> even though the limit can be found using algebraic simplification as in Section 9.1, use L’Hôpital’s rule to find the limit.
> Use the graph of y = g(x), assuming g″(x) > 0 if x = c or g, to identify (A) Intervals on which the graph of g is concave upward (B) Intervals on which the graph of g is concave downward (C) Intervals on which g″
> Find the probability of being dealt the given hand from a standard 52-card deck. Refer to the description of a standard 52-card deck on page 384. A 7-card hand that contains exactly 1 king and exactly 2 jacks.
> inspect the graph of the function to determine whether it is concave up, concave down, or neither, on the given interval.
> inspect the graph of the function to determine whether it is concave up, concave down, or neither, on the given interval.
> inspect the graph of the function to determine whether it is concave up, concave down, or neither, on the given interval.
> inspect the graph of the function to determine whether it is concave up, concave down, or neither, on the given interval. The identity function, (x) = x, on ( - ∞, ∞)
> One hour after x milligrams of a particular drug are given to a person, the change in body temperature T(x), in degrees Fahrenheit, is given by The rate T′1x2 at which T1x2 changes with respect to the size of the dosage x is called the
> A sporting goods chain places TV ad to promote golf club sales. The marketing director used past records to determine the following data, where x is the number of ads placed monthly and y is the number of golf clubs sold that month. (A) Enter the data
> A company estimates that it will sell N1x2 units of a product after spending $x thousand on advertising, as given by N(x) = -0.5x4 + 26x3 - 360x2 + 20,000 15 ≤ x ≤ 24 When is the rate of change of sales increasing and when is it decreasing? What is th
> A baseball cap manufacturer is planning to expand its workforce. It estimates that the number of baseball caps produced by hiring x new workers is given by T(x) = -0.25x4 + 6x3 0 ≤ x ≤ 18 When is the rate of change of baseball cap production increasin
> A national food service runs food concessions for sporting events throughout the country. The company’s marketing research department chose a particular football stadium to test market a new jumbo hot dog. It was found that the demand for the new hot dog
> Suppose that the cost equation for a company is C(x) = 830 + 396x (A) Find the local extrema for the profit function. (B) On which intervals is the graph of the profit function concave upward? Concave downward?
> An assembly plant produces 40 outboard motors, including 7 that are defective. The quality control department selects 10 at random (from the 40 produced) for testing and will shut down the plant for troubleshooting if 1 or more in the sample are found to
> The company in Problem 85 produces the same camp stove at another plant. The total cost C(x) (in dollars) of producing x camp stoves per week at plant B is shown in the figure. Discuss the graph of the marginal cost function C′(x) and
> Another commonly used measure of inflation is the annual rate of change of the Producer Price Index (PPI). A government report states that the annual rate of change of the PPI is decreasing. What does this say about the shape of the graph of the PPI?
> apply steps 1–3 of the graphing strategy to (x). Use a graphing calculator to approximate (to two decimal places) x intercepts, critical numbers, and inflection points. Summarize all the pertinent information.
> apply steps 1–3 of the graphing strategy to (x). Use a graphing calculator to approximate (to two decimal places) x intercepts, critical numbers, and inflection points. Summarize all the pertinent information.
> use the graph of y = ′(x) to discuss the graph of y = (x). Organize your conclusions in a table, and sketch a possible graph of y = (x).
> use the graph of y = ′(x) to discuss the graph of y = (x). Organize your conclusions in a table, and sketch a possible graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Find the probability of being dealt the given hand from a standard 52-card deck. Refer to the description of a standard 52-card deck on page 384. A 4-card hand that contains no face cards.
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> Summarize the pertinent information obtained by applying the graphing strategy and sketch the graph of y = (x).
> (x) is continuous on (- ∞, ∞). Use the given information to sketch the graph of .
> (x) is continuous on (- ∞, ∞). Use the given information to sketch the graph of .
> (x) is continuous on (- ∞, ∞). Use the given information to sketch the graph of .
> Use the (empirical) probabilities in Problem 81 to find the probability that a city driver selected at random (A) Drives more than 15,000 miles per year or has an accident (B) Drives 15,000 or fewer miles per year and has an accident. Data from problem
> (x) is continuous on (- ∞, ∞). Use the given information to sketch the graph of .
> use the given sign chart to sketch a possible graph of .
> use the given sign chart to sketch a possible graph of .
> Find the intervals on which the graph of  is concave upward, the intervals on which the graph of  is concave downward, and the x, y coordinates of the inflection points.
> Find the intervals on which the graph of  is concave upward, the intervals on which the graph of  is concave downward, and the x, y coordinates of the inflection points.
> Find the intervals on which the graph of  is concave upward, the intervals on which the graph of  is concave downward, and the x, y coordinates of the inflection points.
> Find the intervals on which the graph of  is concave upward, the intervals on which the graph of  is concave downward, and the x, y coordinates of the inflection points.
> Find the intervals on which the graph of  is concave upward, the intervals on which the graph of  is concave downward, and the x, y coordinates of the inflection points.
> find the x and y coordinates of all inflection points.
> find the x and y coordinates of all inflection points.
> Find the probability of being dealt the given hand from a standard 52-card deck. Refer to the description of a standard 52-card deck on page 384. A 6-card hand that contains exactly two clubs.
> find the x and y coordinates of all inflection points.
> find the indicated derivative for each function.
> find the indicated derivative for each function.
> find the indicated derivative for each function.
> find the indicated derivative for each function.

