Question: Find a power series representation for the
Find a power series representation for the function and determine the radius of convergence.
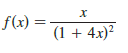
Transcribed Image Text:
f(x) = (1 + 4x)?
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= /sin 6, 0< 0 <T
> If f(n)(0) = (n + 1)! For n = 0, 1, 2, … find the Maclaurin series for f and its radius of convergence.
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = x cos(}x²)
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = cos(Tx/2)
> Use the binomial series to expand the function as a power series. State the radius of convergence. 1 (2 + x) 13
> Prove that the series obtained in Exercise 16 represents sin x for all x. Exercise 16: Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x
> Suppose you know that the series ∑∞n=0bnxn converges for |x| 00 ba Σ R-0 n + 1 n+1
> Prove that the series obtained in Exercise 7 represents sin Ï€x for all x. Exercise 7: Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) â
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x-2, a= 1
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = 1//x, a = 9
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = sin x, a = "/2
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r = sin 0, 7/3 < 0 < 2m/3
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) 3 сos x, а a = T
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = 1/x, a = -3
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.]
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x – x', a= -2
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x* – 3x? + 1, a = 1
> If f (x) = ∑∞n=0 bn (x – 5)n for all x, write a formula for bs.
> Find a power series representation for the function and determine the interval of convergence. 1 + x f(x) 1- x
> Find a power series representation for the function and determine the interval of convergence. f(x) = 2x? + 1
> Find a power series representation for the function and determine the interval of convergence. f(x) = 9 + x?
> Find a power series representation for the function and determine the interval of convergence. f(x) = x + 10
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= e /2
> Find a power series representation for the function and determine the interval of convergence.
> (a). By completing the square, show that (b). By factoring x3 + 1 as a sum of cubes, rewrite the integral in part (a). Then express 1/ (x3 + 1) as the sum of a power series and use it to prove the following formula for π: dx C1/2 Jo x -
> Find a power series representation for the function and determine the interval of convergence. 3 f(x) 1- x*
> Use the power series for tan-1x to prove the following expression for Ï€ as the sum of an infinite series: ㅠ=D 2v3 Σ (-1)" (2n + 1)3" R-0
> (a). Starting with the geometric series∑∞n=0xn, find the sum of the series (b). Find the sum of each of the following series. (c). Find the sum of each of the following series. 2 nx"-I -1 |x| (i) E nx", |x|<1
> The Bessel function of order 1 is defined by (a). Show that j1 satisfies the differential equation (b). Show that j10 (x) = -j1(x). Ji(x) = E (-1)^x²«+1 n!(n + 1)!22n+1 A-0 x³J*(x) + xJ{(x) + (x² – 1)J1(x) = 0
> (a). Show that j0 (the Bessel function of order 0 given in Example 4) satisfies the differential equation (b). Evaluate f10 j0 (x) dx correct to three decimal places. X²J®(x) + xJ¿(x) + x²J(x) = 0
> Show that the function is a solution of the differential equation f"(x) + f (x) = 0 (-1)"x2" f(x) = E %3! A-0 (2n)!
> Use the result of Example 7 to compute arctan 0.2 correct to five decimal places.
> Use a power series to approximate the definite integral to six decimal places. .2 r0.3 dx 1 + x 4 Jo
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= 0°, 0< 0 < /4
> Use a power series to approximate the definite integral to six decimal places. r0.1 "x arctan(3x) dx
> Use a power series to approximate the definite integral to six decimal places. r0.4 In(1 + x*) dx C04
> Use a power series to approximate the definite integral to six decimal places. 1 dx Jo 1+ x* r0.2
> Evaluate the indefinite integral as a power series. What is the radius of convergence? tan-(x²) dx
> Evaluate the indefinite integral as a power series. What is the radius of convergence? X - tan х — -dx .3
> Evaluate the indefinite integral as a power series. What is the radius of convergence? In(1 – t) dt t
> Evaluate the indefinite integral as a power series. What is the radius of convergence? t dt 1- 18
> Find a power series representation for f, and graph f and several partial sums sn (x) on the same screen. What happens as n increases? f(x) = tan-(2x)
> Find a power series representation for f, and graph f and several partial sums sn (x) on the same screen. What happens as n increases? 1+ x f(x) = In{ 1- x
> Find a power series representation for f, and graph f and several partial sums sn (x) on the same screen. What happens as n increases? f(x) = In(x² + 4)
> The astronomer Giovanni Cassini (1625–1712) studied the family of curves with polar equations where and are positive real numbers. These curves are called the ovals of Cassini even though they are oval shaped only for certain values o
> Find a power series representation for f, and graph f and several partial sums sn (x) on the same screen. What happens as n increases? f(x) : x? + 16
> Find a power series representation for the function and determine the radius of convergence. x? + x f(x) (1 – x)³
> Find a power series representation for the function and determine the radius of convergence. 1 + x f(x) = (1 – x)?
> Find a power series representation for the function and determine the radius of convergence. 13 f(x) = 2 - x
> Find a power series representation for the function and determine the radius of convergence. f(x) = x²tan='(x³)
> Find a power series representation for the function and determine the radius of convergence. f(x) 3 In(5 — х)
> In Exercise 31 in Section 6.4 it was shown that the length of the ellipse x = a sin θ, y = b cos θ, where a > b > 0, is where e = √a2 – b2/a is the eccentricity of the ellipse. Expand
> Use the following steps to prove (17). (a). Let g (x) = ∑∞n=0 (k/n) xn. Differentiate this series to show that (b). Let h (x) = (1 + x)-k g (x) and show that h'(x) = 0. (c). Deduce that g (x) = (1 + x)k. kg(x) 1
> (a). Use Equation 1 to find a power series representation for f (x) = ln (1 – x). What is the radius of convergence? (b). Use part (a) to find a power series for f (x) = x ln (1 – x). (c). By putting x = 1/2 in your result from part (a), express ln 2 as
> A family of curves has polar equations Investigate how the graph changes as the number changes. In particular, you should identify the transitional values of a for which the basic shape of the curve changes. 1 — а сos a cos e 1 + a cos e
> Prove Taylor’s Inequality for n = 2, that is, prove that if |f"'(x)| M | R:(x) | <x - a for |x – a|<d 6.
> Find the sum of the series. 1/ 1∙2 – 1/3∙23 + 1/5∙ 25 – 1/7∙ 27 +…
> Find the sum of the series. 3 + 9/2! + 27/3! + 81/4! +…
> Find the sum of the series. 1 – ln2 + (ln2)2/2! - (ln2)2/2! +…
> Find the sum of the series. ∑∞n=0 (-1)n π2n+1/42n+1 (2n + 1)!
> Find the sum of the series. ∑∞n=0 3n/5nn!
> Find the sum of the series. ∑∞n=0 (-1)n-1 3n/n5n
> Find the sum of the series. ∑∞n=0 (-1)n π2n/62n (2n)!
> Find the sum of the series. ∑∞n=0 (-1)n x4n/n!
> Use multiplication or division of power series to find the first three nonzero terms in the Maclaurin series for each function. y = e* In(1 + x)
> A family of curves is given by the equations r = 1 + c sin nθ, where c is a real number and n is a positive integer. How does the graph change as increases? How does it change as c changes? Illustrate by graphing enough members of the family to support y
> Use multiplication or division of power series to find the first three nonzero terms in the Maclaurin series for each function. y sin x
> Use multiplication or division of power series to find the first three nonzero terms in the Maclaurin series for each function. y = sec x
> Use multiplication or division of power series to find the first three nonzero terms in the Maclaurin series for each function. y= e cos x
> Use the series in Example 13(b) to evaluate We found this limit in Example 4 in Section 4.5 using l’Hospital’s Rule three times. Which method do you prefer? tan x - x lim .3
> Use series to evaluate the limit. limx→0 sin x – x + 1/6 x3/ x5
> Use series to evaluate the limit. limx→0 1 – cos x/1 + x - ex
> Use series to evaluate the limit. limx→0 x – 1 ln (1 + x)/x2
> Use series to approximate the definite integral to within the indicated accuracy. x²e* dx ([error|< 0.001) Jo
> Use series to approximate the definite integral to within the indicated accuracy. L" VI + x* dx (lerror| < 5 x 10-9) *0.4
> Use series to approximate the definite integral to within the indicated accuracy. *0.2 " (tan-(x) + sin(x³)] dx (five decimal places)
> (a). Investigate the family of curves defined by the polar equations r = sin nθ, where n is a positive integer. How is the number of loops related to n? (b). What happens if the equation in part (a) is replaced by r = |sin nθ|?
> Use the given graph of f (x) = x2 to find a number δ such that if |x - 1|< 8 |x² – 1|<} then y y=x² 1.5 1+ 0.5 ? 1 ?
> Use series to approximate the definite integral to within the indicated accuracy. x cos(x') dx (three decimal places)
> Evaluate the indefinite integral as an infinite series. f arctan (x2), dx
> Evaluate the indefinite integral as an infinite series. f cos x – 1/x, dx
> (a). Show that the function defined by is not equal to its Maclaurin series. (b). Graph the function in part (a) and comment on its behavior near the origin. if x + 0 f(x) : if x = 0
> (a). Expand 1/4√1 + x as a power series. (b). Use part (a) to estimate 1/4√1.1 correct to three decimal places.
> Let f (x) = ∑∞n=1xn/n2 Find the intervals of convergence for f, f', and f".
> Find the Maclaurin series of f (by any method) and its radius of convergence. Graph f and its first few Taylor polynomials on the same screen. What do you notice about the relationship between these polynomials and f? f(x) = xe¯ хе
> Find the Maclaurin series of f (by any method) and its radius of convergence. Graph f and its first few Taylor polynomials on the same screen. What do you notice about the relationship between these polynomials and f? f(x) = e* + cos x
> (a). Show that the function is a solution of the differential equation f'(x) = f (x) (b). Show that f (x) = ex. 00 f(x) = E -0 n!
> The period of a pendulum with length L that makes a maximum angle θ0 with the vertical is Where k = sin (1/2 θ0) and is the acceleration due to gravity. (In Exercise 34 in Section 5.9 we approximated this integral using Simpso
> How are the graphs of r = 1 + sin (θ – π/6) and r = 1 + sin (θ – π/3) related to the graph of r = 1 + sin θ? In general, how is the graph of r = f (θ – a) related to the graph of r = f (θ)?
> If a surveyor measures differences in elevation when making plans for a highway across a desert, corrections must be made for the curvature of the earth. (a). If R is the radius of the earth and L is the length of the highway, show that the correction is
> (a). Derive Equation 3 for Gaussian optics from Equation 1 by approximating cos ø in Equation 2 by its first-degree Taylor polynomial. (b). Show that if cos ø is replaced by its third-degree Taylor polynomial in Equation 2, then Equation 1 becomes Equati
> A car is moving with speed 20 m/s and acceleration 2 m/s at a given instant. Using a second-degree Taylor polynomial, estimate how far the car moves in the next second. Would it be reasonable to use this polynomial to estimate the distance traveled durin
> Use the Alternating Series Estimation Theorem or Taylor’s Inequality to estimate the range of values of for which the given approximation is accurate to within the stated error. Check your answer graphically. arctan x = x - 3 (Jerr
> Use the Alternating Series Estimation Theorem or Taylor’s Inequality to estimate the range of values of for which the given approximation is accurate to within the stated error. Check your answer graphically. cos x = 1 2 (lerror |<
> How many terms of the Maclaurin series for ln (1 + x) do you need to use to estimate ln 1.4 to within 0.001?
> Use Taylor’s Inequality to determine the number of terms of the Maclaurin series for ex that should be used to estimate e0.1 to within 0.00001.
> (a). Use differentiation to find a power series representation for What is the radius of convergence? (b). Use part (a) to find a power series for (c). Use part (b) to find a power series for 1 f(x) = (1 + x)? 1 f(x) = (1 + x)} 3 x? f(x) (1 + х)
> Use a computer algebra system to find the Taylor polynomials Tn centered at a for n = 2, 3, 4, 5. Then graph these polynomials and f on the same screen. f(x) = VT + x², a= 0
> Use a computer algebra system to find the Taylor polynomials Tn centered at a for n = 2, 3, 4, 5. Then graph these polynomials and f on the same screen. f(x) %3 cot x, a%3D п/4 T/4 a =
> Use a graphing device to graph the polar curve. Choose the parameter interval carefully to make sure that you produce an appropriate curve. r = cos(0/2) + cos(0/3)

