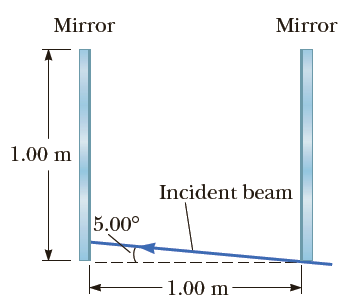
Question: How many times will the incident beam
How many times will the incident beam shown in Figure P22.17 be reflected by each of the parallel mirrors?
Figure P22.17:
> A room contains air in which the speed of sound is 343 m/s. The walls of the room are made of concrete, in which the speed of sound is 1 850 m/s. (a) Find the critical angle for total internal reflection of sound at the concrete–air boundary. (b) In whic
> A beam of laser light with wavelength 612 nm is directed through a slab of glass having index of refraction 1.78. (a) For what minimum incident angle would a ray of light undergo total internal reflection? (b) If a layer of water is placed over the glass
> Figure CQ22.4 shows light from material A with index of refraction nA entering materials B and C with indices of refraction nB and nC. Rank the three indices of refraction from largest to smallest. (a) nA, nB, nC (b) nB, nC, nA (c) nC, nA, nB (d) nB, nA,
> A light ray is incident normally to the long face (the hypotenuse) of a 45°–45°–90° prism surrounded by air, as shown in Figure 22.26b. Calculate the minimum index of refraction of the
> Determine the maximum angle θ for which the light rays incident on the end of the light pipe in Figure P22.38 are subject to total internal reflection along the walls of the pipe. Assume the light pipe has an index of refraction of 1.36 and
> A plastic light pipe has an index of refraction of 1.53. For total internal reflection, what is the minimum angle of incidence if the pipe is in (a) Air and (b) Water?
> A beam of light is incident from air on the surface of a liquid. If the angle of incidence is 30.0° and the angle of refraction is 22.0°, find the critical angle for the liquid when surrounded by air.
> Repeat Problem 34, but this time assume the quartz, polystyrene, and sodium chloride are surrounded by water. Problem 34: For light of wavelength 589 nm, calculate the critical angles for the following substances when surrounded by air: (a) Fused quartz
> For light of wavelength 589 nm, calculate the critical angles for the following substances when surrounded by air: (a) Fused quartz, (b) Polystyrene, and (c) Sodium chloride.
> A ray of light strikes the midpoint of one face of an equiangular (60°–60°–60°) glass prism (n = 1.5) at an angle of incidence of 30.0°. (a) Trace the path of the light ray through the glass and find the angles of incidence and refraction at each surface
> The prism in Figure P22.32 is made of glass with an index of refraction of 1.64 for blue light and 1.60 for red light. Find (a) (R, the angle of deviation for red light, and (b) (B, the angle of deviation for blue light, if white light is incident on the
> A light beam containing red and violet wavelengths is incident on a slab of quartz at an angle of incidence of 50.00°. The index of refraction of quartz is 1.455 at 660 nm (red light), and its index of refraction is 1.468 at 410 nm (violet light). Find t
> The index of refraction for crown glass is 1.512 at a wavelength of 660 nm (red), whereas its index of refraction is 1.530 at a wavelength of 410 nm (violet). If both wavelengths are incident on a slab of crown glass at the same angle of incidence, 60.0°
> The top row of Figure CQ23.2 shows three ray diagrams for an object O in front of a concave mirror and the bottom row shows three ray diagrams for an object O in front of a convex mirror. In each diagram, one ray is drawn correctly and the other is drawn
> The index of refraction for red light in water is 1.331 and that for blue light is 1.340. If a ray of white light enters the water at an angle of incidence of 83.00°, what are the underwater angles of refraction for the (a) Blue and (b) Red components of
> A certain kind of glass has an index of refraction of 1.650 for blue light of wavelength 430 nm and an index of 1.615 for red light of wavelength 680 nm. If a beam containing these two colors is incident at an angle of 30.00° on a piece of this glass, wh
> An opaque cylindrical tank with an open top has a diameter of 3.00 m and is completely filled with water. When the afternoon Sun reaches an angle of 28.0° above the horizon, sunlight ceases to illuminate the bottom of the tank. How deep is the tank?
> Figure P22.26 shows a light ray incident on a series of slabs having different refractive indices, where n1 Figure P22.26:
> A beam of light both reflects and refracts at the surface between air and glass, as shown in Figure P22.25. If the index of refraction of the glass is ng, find the angle of incidence, θ1, in the air that would result in the reflected ray and
> Photons with a wavelength of 589 nm in air enter a plate of crown glass with index of refraction n = 1.52. Find the (a) Speed, (b) Wavelength, and (c) Energy of a photon in the glass.
> Fingerprints left on a piece of glass such as a windowpane can show colored spectra like that from a diffraction grating. Why?
> Count the number of 180° phase reversals for the interfering rays in (a) Figure CQ24.6a, (b) Figure CQ24.6b, and (c) Figure CQ24.6c. Figure CQ24.6:
> If a Young’s experiment carried out in air is repeated under water, would the distance between bright fringes (a) Increase, (b) Decrease, or (c) Remain the same?
> A plane monochromatic light wave is incident on a double - slit as illustrated in Figure 24.4. If the viewing screen is moved away from the double slit, what happens to the separation between the interference fringes on the screen? (a) It increases. (b)
> A ray of light passes from one material into a material with a higher index of refraction. Determine whether each of the following quantities increases, decreases, or remains unchanged. Indicate your answers with I, D, or U, respectively. (a) The ray’s a
> If laser light is reflected from a phonograph record or a compact disc, a diffraction pattern appears. The pattern arises because both devices contain parallel tracks of information that act as a reflection diffraction grating. Which device, record or co
> In a single - slit diffraction experiment, as the width of the slit is made smaller, does the width of the central maximum of the diffraction pattern (a) Become smaller, (b) Become larger, or (c) Remain the same?
> Suppose Young’s experiment is carried out in air, and then, in a second experiment, the apparatus is immersed in water. In what way does the distance between bright fringes change? (a) They move farther apart. (b) They move closer together. (c) There is
> A Young’s double - slit experiment is performed with three different colors of light: red, green, and blue. Rank the colors by the distance between adjacent bright fringes, from smallest to largest. (a) Red, green, blue (b) Green, blue, red (c) Blue, gre
> If the distance between the slits is doubled in Young’s experiment, what happens to the width of the central maximum? (a) The width is doubled. (b) The width is unchanged. (c) The width is halved.
> In a two - slit interference pattern projected on a screen, are the fringes equally spaced on the screen (a) Everywhere, (b) Only for large angles, or (c) Only for small angles?
> A clear plastic sandwich bag filled with water can act as a crude converging lens in air. If the bag is filled with air and placed under water, is the effective lens (a) Converging or (b) Diverging?
> True or False: (a) The image of an object placed in front of a concave mirror is always upright. (b) The height of the image of an object placed in front of a concave mirror must be smaller than or equal to the height of the object. (c) The image of an o
> A person spearfishing from a boat sees a fish located 3 m from the boat at an apparent depth of 1 m. To spear the fish, should the person aim (a) At, (b) Above, or (c) Below the image of the fish?
> In the overhead view of Figure 23.3, the image of the stone seen by observer 1 is at C. Where does observer 2 see the image: at A, at B, at C, at D, at E, or not at all? Figure 23.3:
> A soap film is held vertically in air and is viewed in reflected light as in Figure CQ24.14. Explain why the film appears to be dark at the top. Figure CQ24.14:
> An object is placed to the left of a converging lens. Which of the following statements are true, and which are false? (a) The image is always to the right of the lens. (b) The image can be upright or inverted. (c) The image is always smaller or the same
> In Figure 23.25a, the blue object arrow is replaced by one that is much taller than the lens. How many rays from the object will strike the lens? Figure 23.25a:
> As light travels from a vacuum (n = 1) to a medium such as glass (n > 1), which of the following properties remains the same: the (a) Wavelength, (b) Wave speed, or (c) Frequency?
> A material has an index of refraction that increases continuously from top to bottom. Of the three paths shown in Figure 22.10, which path will a light ray follow as it passes through the material? Figure 22.10:
> If beam 1 is the incoming beam in Figure 22.6b, which of the other four beams are due to reflection? Which are due to refraction? Figure 22.6b:
> Which part of Figure 22.3, (a) or (b), better shows specular reflection of light from the roadway? Figure 22.3:
> A person looking into an empty container is able to see the far edge of the container’s bottom, as shown in Figure P22.23a. The height of the container is h, and its width is d. When the container is completely filled with a fluid of in
> A narrow beam of ultrasonic waves reflects off the liver tumor in Figure P22.22. If the speed of the wave is 10.0% less in the liver than in the surrounding medium, determine the depth of the tumor. Figure P22.22:
> A man shines a flashlight from a boat into the water, illuminating a rock as in Figure P22.21. What is the angle of incidence θ1? Figure P22.21:
> A laser beam is incident on a 45°–45°–90° prism perpendicular to one of its faces, as shown in Figure P22.20. The transmitted beam that exits the hypotenuse of the prism makes an angle
> Certain sunglasses use a polarizing material to reduce the intensity of light reflected from shiny surfaces, such as water or the hood of a car. What orientation of the transmission axis should the material have to be most effective?
> The light beam shown in Figure P22.19 makes an angle of 20.0° with the normal line NN' in the linseed oil. Determine the angles θ and θ'. (The refractive index for linseed oil is 1.48.) Figure P22.19:
> A ray of light strikes a flat, 2.00-cm-thick block of glass (n = 1.50) at an angle of 30.0° with respect to the normal (Fig. P22.18). (a) Find the angle of refraction at the top surface. (b) Find the angle of incidence at the bottom surface an
> Figure P22.16 shows a light ray traveling in a slab of crown glass surrounded by air. The ray is incident on the right surface at an angle of 55° with the normal and then reflects from points A, B, and C. (a) At which of these points does part
> The light emitted by a helium–neon laser has a wavelength of 632.8 nm in air. As the light travels from air into zircon, find its (a) Speed, (b) Wavelength, and (c) Frequency, all in the zircon.
> Two plane mirrors are at an angle of θ1 = 50.0° with each other as in the side view shown in Figure P22.14. If a horizontal ray is incident on mirror 1, at what angle θ2 does the outgoing reflected ray make with the s
> A ray of light is incident on the surface of a block of clear ice at an angle of 40.0° with the normal. Part of the light is reflected, and part is refracted. Find the angle between the reflected and refracted light.
> Light containing wavelengths of 400. nm, 500. nm, and 650. nm is incident from air on a block of crown glass at an angle of 25.0°. (a) Are all colors refracted alike, or is one color bent more than the others? (b) Calculate the angle of refraction in eac
> A laser beam is incident at an angle of 30.0° to the vertical onto a solution of corn syrup in water. If the beam is refracted to 19.24° to the vertical, (a) What is the index of refraction of the syrup solution? Suppose the light is red, with wavelength
> Two plane mirrors are at right angles to each other as shown by the side view in Figure P22.10. A light ray is incident on mirror 1 at an angle θ with the vertical. Using the law of reflection and geometry, show that after the ray is reflect
> Suppose reflected white light is used to observe a thin, transparent coating on glass as the coating material is gradually deposited by evaporation in a vacuum. Describe some color changes that might occur during the process of building up the thickness
> An underwater scuba diver sees the Sun at an apparent angle of 45.0° from the vertical. What is the actual direction of the Sun?
> The two mirrors in Figure P22.8 meet at a right angle. The beam of light in the vertical plane P strikes mirror 1 as shown. (a) Determine the distance the reflected light beam travels before striking mirror 2. (b) In what direction does the light beam tr
> A ray of light travels from air into another medium, making an angle of θ1 = 45.0° with the normal as in Figure P22.7. Find the angle of refraction θ2 if the second medium is (a) Fused quartz, (b) Carbon disulfide, an
> Find the speed of light in (a) Water, (b) Crown glass, and (c) Diamond.
> (a) How many minutes does it take a photon to travel from the Sun to the Earth? (b) What is the energy in eV of a photon with a wavelength of 558 nm? (c) What is the wavelength of a photon with an energy of 1.00 eV?
> (a) Find a symbolic expression for the wavelength λ of a photon in terms of its energy E, Planck’s constant h, and the speed of light c. (b) What does the equation say about the wavelengths of higher - energy photons?
> Find the energy of (a) A photon having a frequency of 5.00 x 1017 Hz and (b) A photon having a wavelength of 3.00 x 102 nm. Express your answers in units of electron volts, noting that 1 eV = 1.60 x 10-19 J.
> (a) What is the energy in joules of an x-ray photon with wavelength 1.00 x 10-10 m? (b) Convert the energy to electron volts. (c) If more penetrating x - rays are desired, should the wavelength be increased or decreased? (d) Should the frequency be incre
> A virtual image is formed 20.0 cm from a concave mirror having a radius of curvature of 40.0 cm. (a) Find the position of the object. (b) What is the magnification of the mirror?
> To fit a contact lens to a patient’s eye, a kerato-meter can be used to measure the curvature of the cornea—the front surface of the eye. This instrument places an illuminated object of known size at a known distance p from the cornea, which then reflect
> Holding your hand at arm’s length, you can readily block direct sunlight from your eyes. Why can you not block sound from your ears this way?
> A convex spherical mirror, whose focal length has a magnitude of 15.0 cm, is to form an image 10.0 cm behind the mirror. (a) Where should the object be placed? (b) What is the magnification of the mirror?
> An object 10.0 cm tall is placed at the zero mark of a meter-stick. A spherical mirror located at some point on the meter-stick creates an image of the object that is upright, 4.00 cm tall, and located at the 42.0 - cm mark of the meter-stick. (a) Is the
> A glass sphere (n = 1.50) with a radius of 15.0 cm has a tiny air bubble 5.00 cm above its center. The sphere is viewed looking down along the extended radius containing the bubble. What is the apparent depth of the bubble below the surface of the sphere
> A certain Christmas tree ornament is a silver sphere having a diameter of 8.50 cm. (a) If the size of an image created by reflection in the ornament is three - fourth’s the reflected object’s actual size, determine the object’s location. (b) Use a princi
> The lens - maker’s equation applies to a lens immersed in a liquid if n in the equation is replaced by n1/n2. Here n1 refers to the refractive index of the lens material and n2 is that of the medium surrounding the lens. (a) A certain lens has focal leng
> An observer to the right of the mirror–lens combination shown in Figure P23.62 sees two real images that are the same size and in the same location. One image is upright, and the other is inverted. Both images are 1.50 times larger than
> The lens - maker’s equation for a lens with index n1 immersed in a medium with index n2 takes the form A thin diverging glass (index = 1.50) lens with R1 = -3.00 m and R2 = -6.00 m is surrounded by air. An arrow is placed 10.0 m to the
> Find the object distances (in terms of f) for a thin converging lens of focal length f if (a) The image is real and the image distance is four times the focal length and (b) The image is virtual and the absolute value of the image distance is three times
> A dentist uses a mirror to examine a tooth that is 1.00 cm in front of the mirror. The image of the tooth is formed 10.0 cm behind the mirror. Determine (a) The mirror’s radius of curvature and (b) The magnification of the image.
> Figure P23.59 shows a converging lens with radii R1 = 9.00 cm and R2 = -11.00 cm, in front of a concave spherical mirror of radius R = 8.00 cm. The focal points (F1 and F2) for the thin lens and the center of curvature (C) of the mirror are also shown. (
> A person spear fishing from a boat sees a stationary fish a few meters away in a direction about 30° below the horizontal. To spear the fish, and assuming the spear does not change direction when it enters the water, should the person (a) Aim above where
> A “floating strawberry” illusion can be produced by two parabolic mirrors, each with a focal length of 7.5 cm, facing each other so that their centers are 7.5 cm apart (Fig. P23.58). If a strawberry is placed on the bo
> An object 2.00 cm high is placed 40.0 cm to the left of a converging lens having a focal length of 30.0 cm. A diverging lens having a focal length of -20.0 cm is placed 110 cm to the right of the converging lens. (a) Determine the final position and magn
> Consider two thin lenses, one of focal length f1 and the other of focal length f2, placed in contact with each other, as shown in Figure P23.56. Apply the thin - lens equation to each of these lenses and combine the results to show that this combination
> To work this problem, use the fact that the image formed by the first surface becomes the object for the second surface. Figure P23.55 shows a piece of glass with index of refraction n = 1.50 surrounded by air. The ends are hemispheres with radii R1 = 2.
> Two rays traveling parallel to the principal axis strike a large plano - convex lens having a refractive index of 1.60 (Fig. P23.54). If the convex face is spherical, a ray near the edge does not pass through the focal point (spherical aberration occurs)
> A parallel beam of light enters a glass hemisphere perpendicular to the flat face, as shown in Figure P23.53. The radius of the hemisphere is R = 6.00 cm, and the index of refraction is n = 1.56. Determine the point at which the beam is focused. (Assume
> The object in Figure P23.52 is midway between the lens and the mirror, which are separated by a distance d = 25.0 cm. The magnitude of the mirror’s radius of curvature is 20.0 cm, and the lens has a focal length of -16.7 cm. (a) Conside
> The lens and the mirror in Figure P23.51 are separated by 1.00 m and have focal lengths of +80.0 cm and -50.0 cm, respectively. If an object is placed 1.00 m to the left of the lens, where will the final image be located? State whether the image is uprig
> A diverging lens (n = 1.50) is shaped like that in Figure 23.25c. The radius of the first surface is 15.0 cm, and that of the second surface is 10.0 cm. (a) Find the focal length of the lens. Determine the positions of the images for object distances of
> A periscope (Fig. P23.5) is useful for viewing objects that cannot be seen directly. It can be used in submarines and when watching golf matches or parades from behind a crowd of people. Suppose the object is a distance p1 from the upper mirror and the c
> Light can travel from air into water. Some possible paths for the light ray in the water are shown in Figure CQ22.14. Which path will the light most likely follow? (a) A (b) B (c) C (d) D (e) E Figure CQ22.14:
> The magnitudes of the radii of curvature are 32.5 cm and 42.5 cm for the two faces of a biconcave lens. The glass has index of refraction 1.53 for violet light and 1.51 for red light. For a very distant object, locate (a) The image formed by violet light
> A real object’s distance from a converging lens is five times the focal length. (a) Determine the location of the image q in terms of the focal length f. (b) Find the magnification of the image. (c) Is the image real or virtual? Is it upright or inverted
> An object placed 10.0 cm from a concave spherical mirror produces a real image 8.00 cm from the mirror. If the object is moved to a new position 20.0 cm from the mirror, what is the position of the image? Is the final image real or virtual?
> An object is placed 15.0 cm from a first converging lens of focal length 10.0 cm. A second converging lens with focal length 5.00 cm is placed 10.0 cm to the right of the first converging lens. (a) Find the position q1 of the image formed by the first co
> Lens L1 in Figure P23.45 has a focal length of 15.0 cm and is located a fixed distance in front of the film plane of a camera. Lens L2 has a focal length of 13.0 cm, and its distance d from the film plane can be varied from 5.00 cm to 10.0 cm. Determine
> Two converging lenses having focal lengths of f1 = 10.0 cm and f2 = 20.0 cm are placed d = 50.0 cm apart, as shown in Figure P23.44. The final image is to be located between the lenses, at the position x = 31.0 cm indicated. (a) How far to the left of th
> A 1.00 - cm-high object is placed 4.00 cm to the left of a converging lens of focal length 8.00 cm. A diverging lens of focal length -16.00 cm is 6.00 cm to the right of the converging lens. Find the position and height of the final image. Is the image i
> A converging lens is placed at x = 0, a distance d = 10.0 cm to the left of a diverging lens as in Figure P23.42 (where FC and FD locate the focal points for the converging and the diverging lens, respectively). An object is located at x = -2.00 cm to th
> Two converging lenses, each of focal length 15.0 cm, are placed 40.0 cm apart, and an object is placed 30.0 cm in front of the first lens. Where is the final image formed, and what is the magnification of the system?
> (a) Use the thin - lens equation to derive an expression for q in terms of f and p. (b) Prove that for a real object and a diverging lens, the image must always be virtual. Hint: Set f = -|f| and show that q must be less than zero under the given conditi

