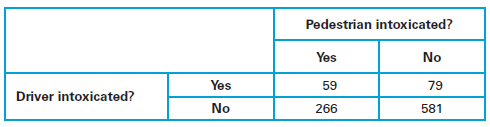
Question: If one of the pedestrian deaths is
If one of the pedestrian deaths is randomly selected, find the probability that the pedestrian was not intoxicated or the driver was not intoxicated.
> What is a scatterplot, and how is one constructed? How can we use a scatter plot to investigate a correlation?
> What is a correlation? Give three examples of pairs of variables that are correlated.
> As part of the results from the test described in Exercise 1, the P-value of 0.001 is obtained. What do you conclude?
> Listed below are the ages of female and male Academy Award winners in 15 selected years. Each pair of ages is for the same year. Construct a scatterplot; Briefly characterize the correlation in words (such as “strong positive correlati
> A nonprofit organization held a fund-raising auction attended by one of the authors of this text. He recorded the opening bids suggested by the auctioneer and the final winning bids for several items. The amounts (in dollars) are listed below. Construct
> Listed below are systolic blood pressure measurements (in mm Hg) obtained from the same woman (based on data from “Consistency of Blood Pressure Differences Between the Left and Right Arms,” by Eguchi, et al., Archives
> The table below lists brain sizes (in cm3) and Wechsler IQ scores of subjects (based on data from “Brain Size, Head Size, and Intelligence Quotient in Monozygotic Twins,” by Tramo etˆal., Neurology, Vol. 50
> Listed below are repair costs (in dollars) for cars crashed at 6 mi/h in full-front crash tests and the same cars crashed at 6 mi/h in full-rear crash tests (based on data from the Insurance Institute for Highway Safety). The cars are the Toyota Camry, M
> Listed below are altitudes (in thousands of feet) and outside air temperatures (in °F) recorded by one of the authors of this text during Delta Flight 1053 from New Orleans to Atlanta. Construct a scatterplot; Briefly characterize the correla
> Listed below are numbers of enrolled students (in thousands) and numbers of campus burglaries for randomly selected large colleges in a recent year (based on data from the New York Times). Construct a scatterplot; Briefly characterize the correlation in
> Listed below are annual data for various years. The data are weights (metric tons) of lemons imported from Mexico and U.S. car crash fatality rates per 100,000 population [based on data from “The Trouble with QSAR (or How I Learned to S
> Because the same sample values are used, the correlation coefficient remains unchanged if we rearrange the order of the x-values that are paired with the y-values.
> The correlation coefficient remains unchanged if we change the sign of all of the x-values.
> If the hypothesis test described in Exercise 9 results in a P-value of 0.001, what do you conclude about the null hypothesis?
> The correlation coefficient remains unchanged if we multiply all of the values of x by the same positive constant.
> The correlation coefficient remains unchanged if we interchange the variables x and y.
> Figure 7.8 shows a scatter plot in which the actual high temperature for the day is compared with a forecast made two days in advance. Estimate the correlation coefficient, and discuss what these data imply about weather forecasts. Do you think you would
> One classic example of a correlation involves the association between the temperature and the number of times a cricket chirps in a minute. The scatter plot in Figure 7.7 on the next page shows the relationship for eight different pairs of values of temp
> The sizes (in square feet) of homes and the prices of homes.
> The pulse rates (in beats per minute) and SAT scores of adults
> The weights of gasoline-powered cars and the distances they can travel on a gallon of gas (measured in miles per gallon)
> The distances of taxi cab rides and the costs of trips.
> Golf scores and prize money won by professional golfers (lower scores are better)
> The IQ scores and hat sizes of randomly selected adults.
> Assuming that we want to use the data in the table below to test for independence between wearing a helmet and receiving facial injuries in a bicycle accident, find the expected frequency for the cell with an observed frequency of 83.
> The weights of taxi cab passengers and the costs of trips.
> The total weight of a package you wish to mail and the cost to mail it
> The two variables I studied showed such a strong correlation that they had a correlation coefficient of r = 1.50.
> The scatter plot showed all the data points following a nearly straight diagonal line, but only a weak correlation between the two variables being plotted.
> Numerous studies have found a negative correlation between the amount of time a student spends on social media and the student’s GPA, suggesting that if you want a higher GPA, you should spend less time on social media.
> A physician finds that increasing the time spent exercising each week results in a lower resting pulse rate. Because this is a positive result, we say that there is a positive correlation.
> What does the correlation coefficient, r, tell us about the strength of a correlation? What range of values can r have?
> Let A be the event of rolling an odd number on a six-sided dice. Then event not A will be rolling an even number. Are events A and not A overlapping? Why or why not?
> A pollster randomly selects an adult for a survey. Let M denote the event of getting a male, and let R denote the event of getting a Republican. Are events M and R overlapping?
> A geneticist is working with 3 green peas and 1 yellow pea. Let G denote the event of randomly selecting one of these peas and getting one that is green. Let Y denote the event of randomly selecting one of the remaining 3 peas and getting one that is yel
> A scatterplot is a graph in which the points are scattered throughout, without any noticeable pattern.
> For the handicapper in Exercise 1, find the probability that she is correct in each of her next two predictions.
> Let A denote the event of getting a female when you randomly select a fellow student in your statistics class. Let B denote the event of getting a female when you randomly select a fellow student in your psychology class. Are events A and B independent o
> The game of BINGO involves drawing numbered and lettered buttons at random from a barrel. The B numbers are 1–15, the I numbers are 16–30, the N numbers are 31–45, the G numbers are 46–60, and the O numbers are 61–75. Buttons are not replaced after they
> A “1-Panel-THC” test for marijuana use is given to job applicants with these results: Among 143 positive results (indicating marijuana use), 24 are false positives; among 157 negative results (indicating no marijuana use), 3 are false negatives. a. Const
> Refer to the data in Exercise 29. a. If two selections are made with replacement, find the probability that both people refused to respond. b. If two selections are made without replacement, find the probability that both people refused to respond. c. Co
> Refer to the following table summarizing results from a study of 1205 people who either responded to or refused to answer survey questions (based on data from “I Hear You Knocking but You Can’t Come In,â€
> Refer to the data in Exercise 27. a. If two selections are made with replacement, find the probability that both people improved. b. If two selections are made without replacement, find the probability that both people improved. c. Compare the results fr
> In a clinical trial of an allergy medication, 120 people are treated with the medication and 100 other people are given a placebo. A control group consists of 80 people who are given neither the medication nor the placebo. The number of people in each gr
> If two different pedestrian deaths are randomly selected, find the probability that in both cases the pedestrians were intoxicated.
> If two different pedestrian deaths are randomly selected, find the probability that they both involved intoxicated drivers.
> If one of the pedestrian deaths is randomly selected, find the probability that the driver was intoxicated or the pedestrian was not intoxicated.
> If r = 1 or r = -1, then all points in the scatterplot lie directly on the best-fit line.
> If one of the pedestrian deaths is randomly selected, find the probability that the pedestrian was intoxicated or the driver was not intoxicated.
> If one of the pedestrian deaths is randomly selected, find the probability that the pedestrian was intoxicated or the driver was intoxicated.
> If a defendant is randomly selected, what is the probability that the defendant entered a guilty plea and was not sent to prison? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of
> If a defendant is randomly selected, what is the probability that the defendant entered a guilty plea and was sent to prison? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of the
> If two different defendants are randomly selected, what is the probability that they both were sentenced to prison? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of the Criminal C
> If two different defendants are randomly selected, what is the probability that they both entered guilty pleas? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of the Criminal Court
> What is the probability that a randomly selected defendant either pled not guilty or was not sent to prison? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of the Criminal Courts,&
> What is the probability that a randomly selected defendant either pled guilty or was sent to prison? Source: Brereton and Casper, “Does It Pay to Plead Guilty? Differential Sentencing and the Functioning of the Criminal Courts,â&#
> A pollster plans to call adults. She has a list of names and numbers of 100 adults, consisting of 60 males and 40 females. She will make random selections and will not call the same person more than once. a. Consider the event of getting a male on the fi
> If r = 0.200, then r2 = 0.040 and 4% of the plotted points lie on the best-fit line.
> The 50 songs on a smart phone consist of 15 that are rock, 20 that are jazz, and 15 that are country. Selections are played randomly and can be repeated. What is the probability of each of the following events? a. The first two selections are both rock.
> A study of hunting injuries and the wearing of hunter orange clothing showed that among 123 hunters injured when mistaken for game, 6 were wearing orange (based on data from the Centers for Disease Control and Prevention). If a follow-up study begins wit
> A programmer is instructed to create a password consisting of two different digits. What is the probability that the password is 12?
> A couple plans to have four children. Find the probability that the first three children are girls and the last child is a boy.
> Assume that boys and girls are equally likely and that the gender of a child is independent of the gender of any brothers or sisters. If a couple already has three girls, find the probability of getting a girl when their fourth baby is born.
> The probability of your winning your state’s lottery this week is not affected by whether you won that same lottery last week.
> The probability of rolling a die and getting an even number is 1/2, and the probability of getting an odd number is 1/2, so the probability of getting a number that is even or odd is 1/2 + 1/2 = 1.
> The probability of flipping a coin and getting heads is 1/2. The probability of rolling a die and getting a 2 is 1/6. When flipping a coin and rolling a die at the same time, the probability of getting heads or a 2 is 1/2 + 1/6 = 3/6 + 1/6 = 4/6, or 2/3.
> Because the numbers 5, 17, 18, 27, 36, and 41 were drawn in the last lottery, you should not bet on them in the next lottery because they are now less likely to be drawn.
> No one can succeed in selling a product that will kill thousands of people per year.
> Fill in the blank: If r = 0.900, then ___% of the variation in the response variable y can be explained by the linear relationship between x and y as described by the best-fit line.
> Based on recent data, a 20-year-old person in the United States has a life expectancy of about 60 years. What does that mean?
> What is life expectancy? Does a 30-year-old person have the same life expectancy as a 20-year-old person? Why or why not?
> What are vital statistics? What does it mean to say that the current U.S. birth rate is 13.2 per 1000 people?
> What is travel risk? Give an example. Can you easily compare different risk rates?
> What would the life expectancy for men be in 2100? Do you think this calculation gives a more or less realistic estimate of life expectancy in 2100 than was found in Example 5? Explain.
> What would the life expectancy for women be in 2100? Do you think this calculation gives a more or less realistic estimate of life expectancy in 2100 than was found in Example 5? Explain.
> Based on current life expectancy data, to what age is the average 80-year-old expected to live? Figure 6.13 (a) The overall U.S. death rate (deaths per 1000 people) for different ages. (b) Life expectancy (the number of additional years of life that c
> Based on current life expectancy data, to what age is the average 40-year-old expected to live? Figure 6.13 (a) The overall U.S. death rate (deaths per 1000 people) for different ages. (b) Life expectancy (the number of additional years of life that c
> Estimate the death rate for 25-year-olds. If the population of people this age is 42 million, how many people of this age can be expected to die in a year? Figure 6.13 (a) The overall U.S. death rate (deaths per 1000 people) for different ages. (b) L
> Estimate the death rate for 60-year-olds. If the population of people this age is about 14 million, how many people of this age can be expected to die in a year? Figure 6.13 (a) The overall U.S. death rate (deaths per 1000 people) for different ages.
> Refer again to the scatterplot in Figure 7.24. Does there appear to be a significant correlation between the two variables? Figure 7.24.
> If you lived in a typical city of 500,000, how many people would you expect to die due to chronic respiratory diseases in a year? Source: Centers for Disease Control and Prevention; data for 2014.
> If you lived in a typical city of 500,000, how many people would you expect to die due to a stroke in a year?
> What is the death rate due to heart disease in deaths per 100,000 of the population? Source: Centers for Disease Control and Prevention; data for 2014.
> What is the death rate due to Alzheimer’s disease in deaths per 100,000 of the population? Source: Centers for Disease Control and Prevention; data for 2014.
> Find the death rate of 20-year-olds per 100,000 people during the year. How many 20-year-olds would be expected to die out of 10,000 people?
> Find the death rate of 19-year-olds per 100,000 people during the year. How many 19-year-olds would be expected to die out of 10,000 people?
> Based on current life expectancy data, how many years is a randomly selected 18-year-old expected to live beyond his or her 18th birthday?
> Based on current life expectancy data, how many years is a randomly selected 20-year-old expected to live beyond his or her 20th birthday?
> Compare the death rates per 1000 people for California and the United States.
> Compare the death rates per 1000 people for California and Florida. Identify a reason for the difference.
> Estimate the correlation coefficient for the data points in the scatterplot in Figure 7.24. Figure 7.24.
> Find the birth rate per 1000 people for California.
> Find the birth rate per 1000 people for the United States.
> For the year 2014, find the fatality rate in deaths per passenger mile (instead of per billion passenger miles). Why don’t we report the fatality rate in units of deaths per passenger mile?
> For each of the three years, find the fatality rate in deaths per million passengers. On the basis of those rates, which year was the safest? Why?
> For each of the three years, find the fatality rate in deaths per billion passenger miles. On the basis of those rates, which year was the safest? Why?
> For each of the three years, find the fatality rate in deaths per 1000 departures. On the basis of those rates, which year was the safest? Why?
> A 60-year-old has a shorter life expectancy than a 20-year-old.
> Your life expectancy is the major factor in determining how long you live.
> The death rate for motorcycle accidents is much higher than the death rate for automobile accidents, so more people must die in motorcycle accidents than in car accidents.

