Question: Let Show that u3 + v3 + w3 - 3uvw =
Let Show that u3 + v3 + w3 - 3uvw = 1.
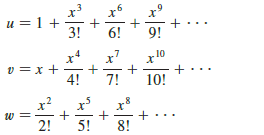
Transcribed Image Text:
.3 u = 1 + 3! 6! 9! 10 v = x + 4! 7! 10! w = 2! 5! 8! + + + +
> Find the sum of the series. ∑∞n=1 (-1)n πn/32n (2n)!
> Find the sum of the series. ∑∞n=1 (-3)n-1/23n
> Determine whether the series is convergent or divergent. ∑∞n=1 (-5)2n/n29n
> Determine whether the series is convergent or divergent. ∑∞n=1 1 ∙ 3 ∙5 ∙ ∙∙∙ (2n – 1)/5nn!
> Determine whether the series is convergent or divergent. ∑∞n=1 cos 3n/1 + (1.2)n
> Determine whether the series is convergent or divergent. ∑∞n=1 (-1)n-1 √n/n + 1
> Determine whether the series is convergent or divergent. ∑∞n=1 ln (n/3m + 1)
> Determine whether the series is convergent or divergent. ∑∞n=1 1/n √ln n
> Determine whether the series is convergent or divergent. ∑∞n=1 (-1)n/√n + 1
> Sketch the curve and find the area that it encloses. r2 = 4 cos 2θ
> Determine whether the series is convergent or divergent. ∑∞n=1 n3/5n
> Determine whether the series is convergent or divergent. ∑∞n=1 n2 +1/n3 + 1
> Determine whether the series is convergent or divergent. ∑∞n=1 n/n3 + 1
> A sequence is defined recursively by the equations a1 = 1, an+1 = 1/3(an + 4). Show that {an} is increasing and an < 2 for all n. Deduce that {an} is convergent and find its limit.
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. {(1 + 3/n)tr}
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. In n an
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. n sin n an ,2 + 1
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. an = = cos(nT/2)
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. 3 n a 1+ п? ,2
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. 94+1 an 10"
> Find the area of the shaded region. r= sin 20
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. 2 + n3 1+ 2n3
> The force due to gravity on an object with mass m at a height above the surface of the earth is F = mgR2/ (R + h)2 where R is the radius of the earth and is the acceleration due to gravity. (a). Express F as a series in powers of h/R. (b). Observe that i
> This project deals with the function 1. Use your computer algebra system to evaluate f (x) for x = 1, 0.1, 0.01, 0.001 and 0.0001. Does it appear that f has a limit as x→0? 2. Use the CAS to graph f near x = 0. Does it appear that f h
> Write the binomial series expansion of (1 + x)k. What is the radius of convergence of this series?
> (a). Write an expression for the nth-degree Taylor polynomial of f centered at a. (b). Write an expression for the Taylor series of centered at f. (c). Write an expression for the Maclaurin series of f. (d). How do you show that f (x) is equal to the sum
> Suppose f (x) is the sum of a power series with radius of convergence R. (a). How do you differentiate f? What is the radius of convergence of the series for f'? (b). How do you integrate f? What is the radius of convergence of the series for ff (x) dx?
> (a). If a series is convergent by the Integral Test, how do you estimate its sum? (b). If a series is convergent by the Comparison Test, how do you estimate its sum? (c). If a series is convergent by the Alternating Series Test, how do you estimate its s
> (a). What is an absolutely convergent series? (b). What can you say about such a series?
> Suppose ∑an = 3 and sn is the nth partial sum of the series. What is limn lim n→∞ an? What is limn lim n→∞ sn?
> (a). What is a geometric series? Under what circumstances is it convergent? What is its sum? (b). What is a p-series? Under what circumstances is it convergent?
> Find the area of the shaded region. r= 4+3 sin e
> For the limit illustrate Definition 1 by finding values of that correspond to c = 1 and c = 0.1. lim (4 + x – 3x') = 2
> (a). What is a bounded sequence? (b). What is a monotonic sequence? (c). What can you say about a bounded monotonic sequence?
> (a). What is a convergent sequence? (b). What is a convergent series? (c). What does lima→∞ an = 3 mean? (d). What does ∑∞n=1 an = 3 mean?
> Write the Maclaurin series and the interval of convergence for each of the following functions. – x) (a) 1/(1 – x) (c) sin x (e) tan-'x (b) e* (d) cos x (f) In(1 + x)
> (a). Write the general form of a power series. (b). What is the radius of convergence of a power series? (c). What is the interval of convergence of a power series?
> State the following. (a). The Test for Divergence (b). The Integral Test (c). The Comparison Test (d). The Limit Comparison Test (e). The Alternating Series Test (f). The Ratio Test
> Any object emits radiation when heated. A blackbody is a system that absorbs all the radiation that falls on it. For instance, a matte black surface or a large cavity with a small hole in its wall (like a blast furnace) is a blackbody and emits blackbody
> (a). Show that the Maclaurin series of the function f (x) = x / 1 – x – x2 is ∑∞n=1 fn xn where fn is the nth Fibonacci number, that is, f1 = 1, f2 = 1, and fn = fn-1 + fn-2 for n > 2. [Hint: Write x/ (1 – x – x2) = c0 + c1x + c2x2 + … and multiply both
> Consider the series whose terms are the reciprocals of the positive integers that can be written in base 10 notation without using the digit 0. Show that this series is convergent and the sum is less than 90.
> Right-angled triangles are constructed as in the figure. Each triangle has height 1 and its base is the hypotenuse of the preceding triangle. Show that this sequence of triangles makes indefinitely many turns around P by showing that ∑
> Find all the solutions of the equation Hint: Consider the cases x > 0 and x 1 + 2! +... = 8! 4! + +
> Find the area of the shaded region. r=1+ cos 0
> Starting with the vertices P1 (0, 1), P2 (1, 1), P3 (1, 0), P4 (0, 0) of a square, we construct further points as shown in the figure: P5 is the midpoint of P1P2,P6 is the midpoint P2P3,P7 of is the midpoint of P3P4, and so on. The polygonal spiral path
> Find the sum of the series∑∞n=1 (-1)n/(2n + 1)3n.
> A sequence {an} is defined recursively by the equations ao = aj = 1 п(п — 1)а, — (п — 1)(п — 2)а,-1 — (п — 3)а,-2 %3D
> Suppose that circles of equal diameter are packed tightly in rows inside an equilateral triangle. (The figure illustrates the case n = 4.) If A is the area of the triangle and An is the total area occupied by the rows of circles, show that An lim A
> If p > 1, evaluate the expression
> Suppose you have a large supply of books, all the same size, and you stack them at the edge of a table, with each book extending farther beyond the edge of the table than the one beneath it. Show that it is possible to do this so that the top book extend
> Find the sum of the series ∑∞n=1 ln (1 – 1/n2).
> Find the sum of the series where the terms are the reciprocals of the positive integers whose only prime factors are 2s and 3s. 1+ 3 4 6 8 12 +
> To construct the snowflake curve, start with an equilateral triangle with sides of length 1. Step 1 in the construction is to divide each side into three equal parts, construct an equilateral triangle on the middle part, and then delete the middle part (
> Find the area of the shaded region. r=
> Let {pn} be a sequence of points determined as in the figure. Thus |AP1| = 1, |PnPn+1| = 2n-1, and angle APnPn+1 is a right angle. Find limn→∞∠Pn APn+1. P4 4 P3 P2 ´A 1 P. P3
> If f (x) = sin (x3), find f(15)(0).
> 1. If limn→∞an = 0, then ∑an is convergent. 2. The series ∑∞n=1 n-sin 1 is convergent. 3.If limn→∞an = L, then limnâ†
> Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) → 0.] Also find the associated radius of convergence. f(x) = cos 3x
> Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) → 0.] Also find the associated radius of convergence. f(x): = sin 7x
> Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) → 0.] Also find the associated radius of convergence. f() %—D In(1 + х) %3D
> Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) → 0.] Also find the associated radius of convergence. f(x) = (1 – x)-2 %3D
> Find the Taylor series for f centered at 4 if What is the radius of convergence of the Taylor series? (-1)* п! fl(4) = 3(n + 1)
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = sin'x [Hint: Use sin'x = }(1 – s 2x).] cos %3D
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = x² In(1 + x³)
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= /sin 6, 0< 0 <T
> If f(n)(0) = (n + 1)! For n = 0, 1, 2, … find the Maclaurin series for f and its radius of convergence.
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = x cos(}x²)
> Use a Maclaurin series in Table 1 to obtain the Maclaurin series for the given function. f(x) = cos(Tx/2)
> Use the binomial series to expand the function as a power series. State the radius of convergence. 1 (2 + x) 13
> Prove that the series obtained in Exercise 16 represents sin x for all x. Exercise 16: Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x
> Suppose you know that the series ∑∞n=0bnxn converges for |x| 00 ba Σ R-0 n + 1 n+1
> Prove that the series obtained in Exercise 7 represents sin Ï€x for all x. Exercise 7: Find the Maclaurin series for f (x) using the definition of a Maclaurin series. [Assume that f has a power series expansion. Do not show that Rn (x) â
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x-2, a= 1
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = 1//x, a = 9
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = sin x, a = "/2
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r = sin 0, 7/3 < 0 < 2m/3
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) 3 сos x, а a = T
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = 1/x, a = -3
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.]
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x – x', a= -2
> Find the Taylor series for f (x) centered at the given value of f. [Assume that has a power series expansion. Do not show that Rn (x) →0.] f(x) = x* – 3x? + 1, a = 1
> If f (x) = ∑∞n=0 bn (x – 5)n for all x, write a formula for bs.
> Find a power series representation for the function and determine the interval of convergence. 1 + x f(x) 1- x
> Find a power series representation for the function and determine the interval of convergence. f(x) = 2x? + 1
> Find a power series representation for the function and determine the interval of convergence. f(x) = 9 + x?
> Find a power series representation for the function and determine the interval of convergence. f(x) = x + 10
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= e /2
> Find a power series representation for the function and determine the interval of convergence.
> (a). By completing the square, show that (b). By factoring x3 + 1 as a sum of cubes, rewrite the integral in part (a). Then express 1/ (x3 + 1) as the sum of a power series and use it to prove the following formula for π: dx C1/2 Jo x -
> Find a power series representation for the function and determine the interval of convergence. 3 f(x) 1- x*
> Use the power series for tan-1x to prove the following expression for Ï€ as the sum of an infinite series: ㅠ=D 2v3 Σ (-1)" (2n + 1)3" R-0
> (a). Starting with the geometric series∑∞n=0xn, find the sum of the series (b). Find the sum of each of the following series. (c). Find the sum of each of the following series. 2 nx"-I -1 |x| (i) E nx", |x|<1
> The Bessel function of order 1 is defined by (a). Show that j1 satisfies the differential equation (b). Show that j10 (x) = -j1(x). Ji(x) = E (-1)^x²«+1 n!(n + 1)!22n+1 A-0 x³J*(x) + xJ{(x) + (x² – 1)J1(x) = 0
> (a). Show that j0 (the Bessel function of order 0 given in Example 4) satisfies the differential equation (b). Evaluate f10 j0 (x) dx correct to three decimal places. X²J®(x) + xJ¿(x) + x²J(x) = 0
> Show that the function is a solution of the differential equation f"(x) + f (x) = 0 (-1)"x2" f(x) = E %3! A-0 (2n)!
> Use the result of Example 7 to compute arctan 0.2 correct to five decimal places.
> Use a power series to approximate the definite integral to six decimal places. .2 r0.3 dx 1 + x 4 Jo
> Find the area of the region that is bounded by the given curve and lies in the specified sector. r= 0°, 0< 0 < /4
> Use a power series to approximate the definite integral to six decimal places. r0.1 "x arctan(3x) dx
> Use a power series to approximate the definite integral to six decimal places. r0.4 In(1 + x*) dx C04

