Question: Prove Property 3 of integrals. Property 3
Prove Property 3 of integrals.
Property 3 of integrals:
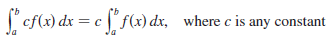
Transcribed Image Text:
cf(x) dx = c f(x) dx, where c is any constant
> Suppose that a volcano is erupting and readings of the rate r(t) at which solid materials are spewed into the atmosphere are given in the table. The time t is measured in seconds and the units for r(t) are tonnes (metric tons) per second. (a) Give uppe
> If H( θ) = θ sin θ , find H’( θ) and H’’(θ ).
> Find dy/dx by implicit differentiation. ex/y = x - y
> The velocity of a car was read from its speedometer at 10-second intervals and recorded in the table. Use the Midpoint Rule to estimate the distance traveled by the car. 1 (s) v (mi/h) t (s) v (mi/h) 60 56 10 38 70 53 20 52 80 50 30 58 90 47 40 55 1
> (a) If f (x) = ex cos x, find f ‘(x) an) f ‘’(x). (b) Check to see that your answers to part (a) are reasonable by graphing f , f ‘, and f ‘’.
> Water flows from the bottom of a storage tank at a rate of r(t) = 200 - 4t liters per minute, where 0 ≤ t ≤ 50. Find the amount of water that flows from the tank during the first 10 minutes.
> (a) If f (x) = sec x - x, find f ‘(x). (b) Check to see that your answer to part (a) is reasonable by graphing both f and f‘ for |x | , π/2.
> Find f ’ (x) and f ’’ (x). f(x) = x / x2 - 1
> The acceleration function (in m/s2 ) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time t and (b) the distance traveled during the given time interval. a(t) = 2t + 3, v(0) = 24, 0 ≤ t ≤ 3
> On May 7, 1992, the space shuttle Endeavour was launched on mission STS-49, the purpose of which was to install a new perigee kick motor in an Intelsat communications satellite. The table gives the velocity data for the shuttle between liftoff and the je
> Shown is the power consumption in the province of Ontario, Canada, for December 9, 2004 (P is measured in megawatts; t is measured in hours starting at midnight). Using the fact that power is the rate of change of energy, estimate the energy used on that
> Shown is the graph of traffic on an Internet service provider’s T1 data line from midnight to 8:00 am. D is the data throughput, measured in megabits per second. Use the Midpoint Rule to estimate the total amount of data transmitted dur
> A bacteria population is 4000 at time t = 0 and its rate of growth is 1000 . 2t bacteria per hour after t hours. What is the population after one hour?
> (a) Fin) an equation of the tangent line to the curve y = 3x + 6 cosx at the point (π/3, π + 3). (b) Illustrate part (a) by graphing the curve an) the tangent line on the same screen.
> For what values of x does the graph of f have a horizontal tangent? f (x) = x + 2 sin x
> Lake Lanier in Georgia, USA, is a reservoir created by Buford Dam on the Chattahoochee River. The table shows the rate of inflow of water, in cubic feet per second, as measured every morning at 7:30 am by the US Army Corps of Engineers. Use the Midpoint
> Find an equation of the tangent line to the curve at the given point. y = cos x - sin x, (π, -1)
> Find an equation of the tangent line to the curve at the given point. y = ex cos x, (0, 1)
> Find f ’ (x) and f ’’ (x). f(x) = x2 / 1+ex
> Find an equation of the tangent line to the curve at the given point. y = sin x + cos x, (0, 1)
> Prove, using the definition of derivative, that if f (x) = cos x, then f ‘(x) = -sin x.
> Find dy/dx by implicit differentiation. ey sin x = x + xy
> Explain exactly what is meant by the statement that “differentiation and integration are inverse processes.”
> Differentiate. f (t) = tet cot t
> The area labeled B is three times the area labeled A. Express b in terms of a. yA y. y= e* y=e* A B a
> Let (a) Find an expression for g(x) similar to the one for f (x). (b) Sketch the graphs of f and g. (c) Where is f differentiable? Where is t differentiable? if x <0 f(x) = 2 if 0 <x<1 - x if 1<x<2 if x > 2 g(x) = f(t) dt
> Justify (3) for the case h (3): f(u) < g(x + h) – g(x) < f(v) h 3.
> Differentiate. f (θ) = cosθ sinθ
> A bacteria culture initially contains 100 cells and grows at a rate proportional to its size. After an hour the population has increased to 420. (a) Find an expression for the number of bacteria after t hours. (b) Find the number of bacteria after 3 hour
> What is wrong with the equation? sec?x dx = tan. x - = 0 11
> What is wrong with the equation? sec 0 tan 0 d0= sec 0 = -3 T/3 /3
> What is wrong with the equation? 2 4 dx = 3 x? 2 -1
> Differentiate. y = cos x / 1 - sin x
> What is wrong with the equation? -3 3 -3 -2 8 -2
> The acceleration function (in m/s2 ) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time t and (b) the distance traveled during the given time interval. a(t) = t + 4, v(0) = 5, 0 ≤ t ≤ 10
> (a) Find an equation of the tangent line to the curve y = 2x sin x at the point (π/2, π). (b) Illustrate part (a) by graphing the curve and the tangent line on the same screen.
> The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval. v(t) = t2 - 2t – 3, 2 ≤ t ≤ 4
> The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval. v(t) = 3t – 5, 0 ≤ t ≤ 3
> Find an equation of the tangent line to the curve at the given point. y = x + tan x, (π , π)
> Repeat Exercise 47 for the curve y = (x2 – 1)-1 - x4. Exercise 47: Use a graph to estimate the x-intercepts of the curve y = 1 - 2x - 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> Use a graph to estimate the x-intercepts of the curve y = 1 - 2x - 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> (a) How long will it take an investment to double in value if the interest rate is 6% compounded continuously? (b) What is the equivalent annual interest rate?
> (a) If $3000 is invested at 5% interest, find the value of the investment at the end of 5 years if the interest is compounded (i) annually, (ii) semiannually, (iii) monthly, (iv) weekly, (v) daily, and (vi) continuously. (b) If A(t) is the amount of the
> If f (x) = x2 - 4, 0 ≤ x ≤ 3, find the Riemann sum with n = 6, taking the sample points to be midpoints. What does the Riemann sum represent? Illustrate with a diagram.
> If f (x) = cos x 0 ≤ x ≤ 3 π/4 evaluate the Riemann sum with n = 6, taking the sample points to be left endpoints. (Give your answer correct to six decimal places.) What does the Riemann sum represent? Illustrate with a diagram.
> Evaluate the Riemann sum for f (x) = x - 1, -6 ≤ x ≤ 4, with five subintervals, taking the sample points to be right endpoints. Explain, with the aid of a diagram, what the Riemann sum represents.
> Let f (s) = 0 and f (x) = 1/x if 0 < x ≤ 1. Show that f is not integrable on [0, 1].
> Let f (x) = 0 if x is any rational number and f (x) = 1 if x is any irrational number. Show that f is not integrable on [0, 1].
> (a) If $1000 is borrowed at 8% interest, find the amounts due at the end of 3 years if the interest is compounded (i) annually, (ii) quarterly, (iii) monthly, (iv) weekly, (v) daily, (vi) hourly, and (vii) continuously. (b) Suppose $1000 is borrowed and
> The rate of change of atmospheric pressure P with respect to altitude h is proportional to P, provided that the temperature is constant. At 15°C the pressure is 101.3 kPa at sea level and 87.14 kPa at h = 1000 m. (a) What is the pressure at an altitude o
> A freshly brewed cup of coffee has temperature 958C in a 20°C room. When its temperature is 70°C, it is cooling at a rate of 1°C per minute. When does this occur?
> When a cold drink is taken from a refrigerator, its temperature is 5°C. After 25 minutes in a 20°C room its temperature has increased to 10°C. (a) What is the temperature of the drink after 50 minutes? (b) When will its temperature be 15°C?
> In a murder investigation, the temperature of the corpse was 32.5°C at 1:30 pm and 30.3°C an hour later. Normal body temperature is 37.0°C and the temperature of the surroundings was 20.0°C. When did the murder take place?
> (a) Find the Riemann sum for f (x) = 1/x, 1 ≤ x ≤ 2, with four terms, taking the sample points to be right endpoints. (Give your answer correct to six decimal places.) Explain what the Riemann sum represents with the aid of a sketch. (b) Repeat part (a)
> A roast turkey is taken from an oven when its temperature has reached 185°F and is placed on a table in a room where the temperature is 75°F. (a) If the temperature of the turkey is 1508F after half an hour, what is the temperature after 45 minutes? (b)
> Evaluate the upper and lower sums for f (x) = 2 + sin x, 0 ≤ x ≤ π, with n = 2, 4, and 8. Illustrate with diagrams like Figure 14. Figure 14: y. a b
> A common inhabitant of human intestines is the bacterium Escherichia coli, named after the German pediatrician Theodor Escherich, who identified it in 1885. A cell of this bacterium in a nutrient-broth medium divides into two cells every 20 minutes. The
> A population of protozoa develops with a constant relative growth rate of 0.7944 per member per day. On day zero the population consists of two members. Find the population size after six days.
> Differentiate. y = sinθ cosθ
> The velocity graph of a car accelerating from rest to a speed of 120 km/h over a period of 30 seconds is shown. Estimate the distance traveled during this period. םע (km/h) 80 40 20 30 (seconds) 10
> The velocity graph of a braking car is shown. Use it to estimate the distance traveled by the car while the brakes are applied. (ft/s) 60 40 20 6 (seconds) 4 t 2.
> Dinosaur fossils are too old to be reliably dated using carbon-14. (See Exercise 11.) Suppose we had a 68-millionyear- old dinosaur fossil. What fraction of the living dinosaur’s 14C would be remaining today? Suppose the minimum detectable amount is 0.1%
> When we estimate distances from velocity data, it is sometimes necessary to use times t0, t1, t2, t3, . . . that are not equally spaced. We can still estimate distances using the time periods Δti = ti - ti-1. For example, on May 7, 1992, the
> Oil leaked from a tank at a rate of r(t) liters per hour. The rate decreased as time passed and values of the rate at two-hour time intervals are shown in the table. Find lower and upper estimates for the total amount of oil that leaked out. t (h) 2
> The table shows speedometer readings at 10-second intervals during a 1-minute period for a car racing at the Daytona International Speedway in Florida. (a) Estimate the distance the race car traveled during this time period using the velocities at the be
> The speed of a runner increased steadily during the first three seconds of a race. Her speed at half-second intervals is given in the table. Find lower and upper estimates for the distance that she traveled during these three seconds. t (s) 0.5 1.0
> (a) If f (x) = ln x, 1 ≤ x ≤ 4, use the commands discussed in Exercise 11 to find the left and right sums for n = 10, 30, and 50. (b) Illustrate by graphing the rectangles in part (a). (c) Show that the exact area under f lies between 2.50 and 2.59. Exe
> Some computer algebra systems have commands that will draw approximating rectangles and evaluate the sums of their areas, at least if xi* is a left or right endpoint. (For instance, in Maple use leftbox, rightbox, leftsum, and rightsum.) (a) If f (x) = 1
> With a programmable calculator (or a computer), it is possible to evaluate the expressions for the sums of areas of approximating rectangles, even for large values of n, using looping. (On a TI use the Is. command or a For-EndFor loop, on a Casio use Isz
> Find the point at which the line intersects the given plane. 5x = y/2 = z + 2; 10x - 7y + 3z + 24 = 0
> With a programmable calculator (or a computer), it is possible to evaluate the expressions for the sums of areas of approximating rectangles, even for large values of n, using looping. (On a TI use the Is. command or a For-EndFor loop, on a Casio use Isz
> Evaluate the upper and lower sums for f (x) = 1 + x2, -1 ≤ x ≤ 1, with n = 3 and 4. Illustrate with diagrams like Figure 14. Figure 14: y. a b
> For any vectors u and v in V3, |u × v | − |v × u |.
> Scientists can determine the age of ancient objects by the method of radiocarbon dating. The bombardment of the upper atmosphere by cosmic rays converts nitrogen to a radioactive isotope of carbon, 14C, with a half-life of about 5730 years. Vegetation ab
> Find dy/dx by implicit differentiation. cos(xy) = 1 + sin y
> Copy the vectors in the figure and use them to draw the following vectors. (a). u + v (b). u + w (c) v + w (d). u - v (e). v + u + w (f). - w - v V
> What are the projections of the point (2, 3, 5) on the xy-, yz-, and xz-planes? Draw a rectangular box with the origin and (2, 3, 5) as opposite vertices and with its faces parallel to the coordinate planes. Label all vertices of the box. Find the length
> Name all the equal vectors in the parallelogram shown. A B E D C.
> Sketch the points (1, 5, 3), (0, 2, -3), (-3, 0, 2), and (2, -2, -1) on a single set of coordinate axes.
> Find the cross product a × b and verify that it is orthogonal to both a and b. a = 2, 3, 0 , b = 1, 0,5
> Differentiate. y = x / 2 - tan x
> Find two unit vectors that are orthogonal to both j + 2k and i - 2j + 3k.
> A surface consists of all points P such that the distance from P to the plane y = 1 is twice the distance from P to the point (0, -1, 0). Find an equation for this surface and identify it.
> Identify and sketch the graph of each surface. x = y2 + z2 - 2y - 4z + 5
> A sample of tritium-3 decayed to 94.5% of its original amount after a year. (a) What is the half-life of tritium-3? (b) How long would it take the sample to decay to 20% of its original amount?
> Identify and sketch the graph of each surface. 4x2 + 4y2 - 8y + z2 = 0
> Identify and sketch the graph of each surface. y2 + z2 = 1 + x2
> Identify and sketch the graph of each surface. -4x2 + y2 - 4z2 = 4
> Identify and sketch the graph of each surface. 4x - y + 2z = 4
> Find a vector equation and parametric equations for the line. The line through the point (2, 2.4, 3.5) and parallel to the vector 3i + 2j - k
> Identify and sketch the graph of each surface. x2 = y2 + 4z2
> Identify and sketch the graph of each surface. y = z2
> If u and v are the vectors shown in the figure, find u ∙ v and |u × v |. Is u × v directed into the page or out of it? |v| = 3 45° |u|=2
> Identify and sketch the graph of each surface. x = z
> Identify and sketch the graph of each surface. x = 3
> Find the distance between the planes 3x + y - 4z = 2 and 3x + y - 4z = 24.
> The half-life of cesium-137 is 30 years. Suppose we have a 100-mg sample. (a) Find the mass that remains after t years. (b) How much of the sample remains after 100 years? (c) After how long will only 1 mg remain?
> (a). Show that the planes x + y - z = 1 and 2x - 3y + 4z = 5 are neither parallel nor perpendicular. (b). Find, correct to the nearest degree, the angle between these planes.
> Copy the vectors in the figure and use them to draw each of the following vectors. (a). a + b (b). a - b (c). 1 2 a (d). 2a + b a b
> Find an equation of the plane. The plane through (2, 1, 0) and parallel to x + 4y - 3z = 1

