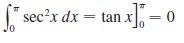Question: What is wrong with the equation? /
> Water is leaking out of an inverted conical tank at a rate of 10,000 cm3/min at the same time that water is being pumped into the tank at a constant rate. The tank has height 6 m and the diameter at the top is 4 m. If the water level is rising at a rate
> A particle moves along the curve y = 2 sins(πx/2). As the particle passes through the point (1/3 , 1), its x-coordinate increases at a rate of 10 cm/s. How fast is the distance from the particle to the origin changing at this instant?
> At noon, ship A is 100 km west of ship B. Ship A is sailing south at 35 km/h and ship B is sailing north at 25 km/h. How fast is the distance between the ships changing at 4:00 pm?
> A boat is pulled into a dock by a rope attached to the bow of the boat and passing through a pulley on the dock that is 1 m higher than the bow of the boat. If the rope is pulled in at a rate of 1 m/s, how fast is the boat approaching the dock when it is
> The altitude of a triangle is increasing at a rate of 1 cm/min while the area of the triangle is increasing at a rate of 2 cm2/min. At what rate is the base of the triangle changing when the altitude is 10 cm and the area is 100 cm2?
> A baseball diamond is a square with side 90 ft. A batter hits the ball and runs toward first base with a speed of 24 ft/s. (a) At what rate is his distance from second base decreasing when he is halfway to first base? (b) At what rate is his distance fro
> A man starts walking north at 4 ft/s from a point P. Five minutes later a woman starts walking south at 5 ft/s from a point 500 ft due east of P. At what rate are the people moving apart 15 min after the woman starts walking?
> Find dy/dx by implicit differentiation. Tan(x – y) = y / 1 + x2
> Find f ’ (x) and f ’’ (x). f (x) = (x3 + 1) ex
> A spotlight on the ground shines on a wall 12 m away. If a man 2 m tall walks from the spotlight toward the building at a speed of 1.6 m/s, how fast is the length of his shadow on the building decreasing when he is 4 m from the building?
> Two cars start moving from the same point. One travels south at 60 mi/h and the other travels west at 25 mi/h. At what rate is the distance between the cars increasing two hours later?
> (a) What quantities are given in the problem? (b) What is the unknown? (c) Draw a picture of the situation for any time t. (d) Write an equation that relates the quantities. (e) Finish solving the problem. At noon, ship A is 150 km west of ship B. Ship A
> (a) What quantities are given in the problem? (b) What is the unknown? (c) Draw a picture of the situation for any time t. (d) Write an equation that relates the quantities. (e) Finish solving the problem. A street light is mounted at the top of a 15-ft-
> For what values of x does the graph of f have a horizontal tangent? f (x) = ex cos x
> Use a graph to give a rough estimate of the area of the region that lies under the given curve. Then find the exact area. y = 2 sin x - sin 2x, 0 ≤ x ≤ π
> The graph of the acceleration a(t) of a car measured in ft/s2 is shown. Use the Midpoint Rule to estimate the increase in the velocity of the car during the six-second time interval. a 12 4 4 6 t(seconds) 2. 00
> Suppose f (π/3) = 4 and f ‘(π/3) = 22, and let g(x)d = f (x) sin x and h(x) = (cos x)/f (x). Find (a) g’(π/3) (b) h’(π/3)
> Water flows into and out of a storage tank. A graph of the rate of change r(t) of the volume of water in the tank, in liters per day, is shown. If the amount of water in the tank at time t = 0 is 25,000 L, use the Midpoint Rule to estimate the amount of
> (a) Use the Quotient Rule to differentiate the function f (x) = tan x – 1 / sec x (b) Simplify the expression for f (x) by writing it in terms of sin x and cos x, and then find f ‘(x). (c) Show that your answers to parts (a) and (b) are equivalent.
> If f (t) = sec t, find f’’ (π/4).
> Suppose that a volcano is erupting and readings of the rate r(t) at which solid materials are spewed into the atmosphere are given in the table. The time t is measured in seconds and the units for r(t) are tonnes (metric tons) per second. (a) Give uppe
> If H( θ) = θ sin θ , find H’( θ) and H’’(θ ).
> Find dy/dx by implicit differentiation. ex/y = x - y
> The velocity of a car was read from its speedometer at 10-second intervals and recorded in the table. Use the Midpoint Rule to estimate the distance traveled by the car. 1 (s) v (mi/h) t (s) v (mi/h) 60 56 10 38 70 53 20 52 80 50 30 58 90 47 40 55 1
> (a) If f (x) = ex cos x, find f ‘(x) an) f ‘’(x). (b) Check to see that your answers to part (a) are reasonable by graphing f , f ‘, and f ‘’.
> Water flows from the bottom of a storage tank at a rate of r(t) = 200 - 4t liters per minute, where 0 ≤ t ≤ 50. Find the amount of water that flows from the tank during the first 10 minutes.
> (a) If f (x) = sec x - x, find f ‘(x). (b) Check to see that your answer to part (a) is reasonable by graphing both f and f‘ for |x | , π/2.
> Find f ’ (x) and f ’’ (x). f(x) = x / x2 - 1
> The acceleration function (in m/s2 ) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time t and (b) the distance traveled during the given time interval. a(t) = 2t + 3, v(0) = 24, 0 ≤ t ≤ 3
> On May 7, 1992, the space shuttle Endeavour was launched on mission STS-49, the purpose of which was to install a new perigee kick motor in an Intelsat communications satellite. The table gives the velocity data for the shuttle between liftoff and the je
> Shown is the power consumption in the province of Ontario, Canada, for December 9, 2004 (P is measured in megawatts; t is measured in hours starting at midnight). Using the fact that power is the rate of change of energy, estimate the energy used on that
> Shown is the graph of traffic on an Internet service provider’s T1 data line from midnight to 8:00 am. D is the data throughput, measured in megabits per second. Use the Midpoint Rule to estimate the total amount of data transmitted dur
> A bacteria population is 4000 at time t = 0 and its rate of growth is 1000 . 2t bacteria per hour after t hours. What is the population after one hour?
> (a) Fin) an equation of the tangent line to the curve y = 3x + 6 cosx at the point (π/3, π + 3). (b) Illustrate part (a) by graphing the curve an) the tangent line on the same screen.
> For what values of x does the graph of f have a horizontal tangent? f (x) = x + 2 sin x
> Lake Lanier in Georgia, USA, is a reservoir created by Buford Dam on the Chattahoochee River. The table shows the rate of inflow of water, in cubic feet per second, as measured every morning at 7:30 am by the US Army Corps of Engineers. Use the Midpoint
> Find an equation of the tangent line to the curve at the given point. y = cos x - sin x, (π, -1)
> Find an equation of the tangent line to the curve at the given point. y = ex cos x, (0, 1)
> Find f ’ (x) and f ’’ (x). f(x) = x2 / 1+ex
> Find an equation of the tangent line to the curve at the given point. y = sin x + cos x, (0, 1)
> Prove, using the definition of derivative, that if f (x) = cos x, then f ‘(x) = -sin x.
> Find dy/dx by implicit differentiation. ey sin x = x + xy
> Explain exactly what is meant by the statement that “differentiation and integration are inverse processes.”
> Differentiate. f (t) = tet cot t
> The area labeled B is three times the area labeled A. Express b in terms of a. yA y. y= e* y=e* A B a
> Let (a) Find an expression for g(x) similar to the one for f (x). (b) Sketch the graphs of f and g. (c) Where is f differentiable? Where is t differentiable? if x <0 f(x) = 2 if 0 <x<1 - x if 1<x<2 if x > 2 g(x) = f(t) dt
> Justify (3) for the case h (3): f(u) < g(x + h) – g(x) < f(v) h 3.
> Differentiate. f (θ) = cosθ sinθ
> A bacteria culture initially contains 100 cells and grows at a rate proportional to its size. After an hour the population has increased to 420. (a) Find an expression for the number of bacteria after t hours. (b) Find the number of bacteria after 3 hour
> What is wrong with the equation? sec 0 tan 0 d0= sec 0 = -3 T/3 /3
> What is wrong with the equation? 2 4 dx = 3 x? 2 -1
> Differentiate. y = cos x / 1 - sin x
> What is wrong with the equation? -3 3 -3 -2 8 -2
> The acceleration function (in m/s2 ) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time t and (b) the distance traveled during the given time interval. a(t) = t + 4, v(0) = 5, 0 ≤ t ≤ 10
> (a) Find an equation of the tangent line to the curve y = 2x sin x at the point (π/2, π). (b) Illustrate part (a) by graphing the curve and the tangent line on the same screen.
> The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval. v(t) = t2 - 2t – 3, 2 ≤ t ≤ 4
> The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval. v(t) = 3t – 5, 0 ≤ t ≤ 3
> Find an equation of the tangent line to the curve at the given point. y = x + tan x, (π , π)
> Repeat Exercise 47 for the curve y = (x2 – 1)-1 - x4. Exercise 47: Use a graph to estimate the x-intercepts of the curve y = 1 - 2x - 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> Use a graph to estimate the x-intercepts of the curve y = 1 - 2x - 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> (a) How long will it take an investment to double in value if the interest rate is 6% compounded continuously? (b) What is the equivalent annual interest rate?
> (a) If $3000 is invested at 5% interest, find the value of the investment at the end of 5 years if the interest is compounded (i) annually, (ii) semiannually, (iii) monthly, (iv) weekly, (v) daily, and (vi) continuously. (b) If A(t) is the amount of the
> If f (x) = x2 - 4, 0 ≤ x ≤ 3, find the Riemann sum with n = 6, taking the sample points to be midpoints. What does the Riemann sum represent? Illustrate with a diagram.
> If f (x) = cos x 0 ≤ x ≤ 3 π/4 evaluate the Riemann sum with n = 6, taking the sample points to be left endpoints. (Give your answer correct to six decimal places.) What does the Riemann sum represent? Illustrate with a diagram.
> Evaluate the Riemann sum for f (x) = x - 1, -6 ≤ x ≤ 4, with five subintervals, taking the sample points to be right endpoints. Explain, with the aid of a diagram, what the Riemann sum represents.
> Let f (s) = 0 and f (x) = 1/x if 0 < x ≤ 1. Show that f is not integrable on [0, 1].
> Let f (x) = 0 if x is any rational number and f (x) = 1 if x is any irrational number. Show that f is not integrable on [0, 1].
> (a) If $1000 is borrowed at 8% interest, find the amounts due at the end of 3 years if the interest is compounded (i) annually, (ii) quarterly, (iii) monthly, (iv) weekly, (v) daily, (vi) hourly, and (vii) continuously. (b) Suppose $1000 is borrowed and
> Prove Property 3 of integrals. Property 3 of integrals: cf(x) dx = c f(x) dx, where c is any constant
> The rate of change of atmospheric pressure P with respect to altitude h is proportional to P, provided that the temperature is constant. At 15°C the pressure is 101.3 kPa at sea level and 87.14 kPa at h = 1000 m. (a) What is the pressure at an altitude o
> A freshly brewed cup of coffee has temperature 958C in a 20°C room. When its temperature is 70°C, it is cooling at a rate of 1°C per minute. When does this occur?
> When a cold drink is taken from a refrigerator, its temperature is 5°C. After 25 minutes in a 20°C room its temperature has increased to 10°C. (a) What is the temperature of the drink after 50 minutes? (b) When will its temperature be 15°C?
> In a murder investigation, the temperature of the corpse was 32.5°C at 1:30 pm and 30.3°C an hour later. Normal body temperature is 37.0°C and the temperature of the surroundings was 20.0°C. When did the murder take place?
> (a) Find the Riemann sum for f (x) = 1/x, 1 ≤ x ≤ 2, with four terms, taking the sample points to be right endpoints. (Give your answer correct to six decimal places.) Explain what the Riemann sum represents with the aid of a sketch. (b) Repeat part (a)
> A roast turkey is taken from an oven when its temperature has reached 185°F and is placed on a table in a room where the temperature is 75°F. (a) If the temperature of the turkey is 1508F after half an hour, what is the temperature after 45 minutes? (b)
> Evaluate the upper and lower sums for f (x) = 2 + sin x, 0 ≤ x ≤ π, with n = 2, 4, and 8. Illustrate with diagrams like Figure 14. Figure 14: y. a b
> A common inhabitant of human intestines is the bacterium Escherichia coli, named after the German pediatrician Theodor Escherich, who identified it in 1885. A cell of this bacterium in a nutrient-broth medium divides into two cells every 20 minutes. The
> A population of protozoa develops with a constant relative growth rate of 0.7944 per member per day. On day zero the population consists of two members. Find the population size after six days.
> Differentiate. y = sinθ cosθ
> The velocity graph of a car accelerating from rest to a speed of 120 km/h over a period of 30 seconds is shown. Estimate the distance traveled during this period. םע (km/h) 80 40 20 30 (seconds) 10
> The velocity graph of a braking car is shown. Use it to estimate the distance traveled by the car while the brakes are applied. (ft/s) 60 40 20 6 (seconds) 4 t 2.
> Dinosaur fossils are too old to be reliably dated using carbon-14. (See Exercise 11.) Suppose we had a 68-millionyear- old dinosaur fossil. What fraction of the living dinosaur’s 14C would be remaining today? Suppose the minimum detectable amount is 0.1%
> When we estimate distances from velocity data, it is sometimes necessary to use times t0, t1, t2, t3, . . . that are not equally spaced. We can still estimate distances using the time periods Δti = ti - ti-1. For example, on May 7, 1992, the
> Oil leaked from a tank at a rate of r(t) liters per hour. The rate decreased as time passed and values of the rate at two-hour time intervals are shown in the table. Find lower and upper estimates for the total amount of oil that leaked out. t (h) 2
> The table shows speedometer readings at 10-second intervals during a 1-minute period for a car racing at the Daytona International Speedway in Florida. (a) Estimate the distance the race car traveled during this time period using the velocities at the be
> The speed of a runner increased steadily during the first three seconds of a race. Her speed at half-second intervals is given in the table. Find lower and upper estimates for the distance that she traveled during these three seconds. t (s) 0.5 1.0
> (a) If f (x) = ln x, 1 ≤ x ≤ 4, use the commands discussed in Exercise 11 to find the left and right sums for n = 10, 30, and 50. (b) Illustrate by graphing the rectangles in part (a). (c) Show that the exact area under f lies between 2.50 and 2.59. Exe
> Some computer algebra systems have commands that will draw approximating rectangles and evaluate the sums of their areas, at least if xi* is a left or right endpoint. (For instance, in Maple use leftbox, rightbox, leftsum, and rightsum.) (a) If f (x) = 1
> With a programmable calculator (or a computer), it is possible to evaluate the expressions for the sums of areas of approximating rectangles, even for large values of n, using looping. (On a TI use the Is. command or a For-EndFor loop, on a Casio use Isz
> Find the point at which the line intersects the given plane. 5x = y/2 = z + 2; 10x - 7y + 3z + 24 = 0
> With a programmable calculator (or a computer), it is possible to evaluate the expressions for the sums of areas of approximating rectangles, even for large values of n, using looping. (On a TI use the Is. command or a For-EndFor loop, on a Casio use Isz
> Evaluate the upper and lower sums for f (x) = 1 + x2, -1 ≤ x ≤ 1, with n = 3 and 4. Illustrate with diagrams like Figure 14. Figure 14: y. a b
> For any vectors u and v in V3, |u × v | − |v × u |.
> Scientists can determine the age of ancient objects by the method of radiocarbon dating. The bombardment of the upper atmosphere by cosmic rays converts nitrogen to a radioactive isotope of carbon, 14C, with a half-life of about 5730 years. Vegetation ab
> Find dy/dx by implicit differentiation. cos(xy) = 1 + sin y
> Copy the vectors in the figure and use them to draw the following vectors. (a). u + v (b). u + w (c) v + w (d). u - v (e). v + u + w (f). - w - v V
> What are the projections of the point (2, 3, 5) on the xy-, yz-, and xz-planes? Draw a rectangular box with the origin and (2, 3, 5) as opposite vertices and with its faces parallel to the coordinate planes. Label all vertices of the box. Find the length
> Name all the equal vectors in the parallelogram shown. A B E D C.
> Sketch the points (1, 5, 3), (0, 2, -3), (-3, 0, 2), and (2, -2, -1) on a single set of coordinate axes.
> Find the cross product a × b and verify that it is orthogonal to both a and b. a = 2, 3, 0 , b = 1, 0,5