Question: Refer to the table below of the
Refer to the table below of the six basic solutions to the e-system
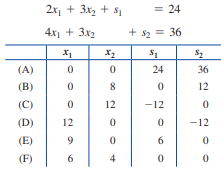
Use the basic feasible solutions to maximize P = 8x1 + 5x2.
> Solve Problem 41 with the additional restriction that the combined total number of components produced each week cannot exceed 420. Discuss the effect of this restriction on the solution to Problem 41. Data from Problem 41: A small company manufactures
> Convert the given time period to years, in reduced fraction form, assuming a 360-day year [this assumption does not affect the number of quarters (4), months (12), or weeks (52) in a year]. 6 Weeks
> There is a tie for the choice of the first pivot column. Use the simplex method to solve each problem two different ways: first by choosing column 1 as the first pivot column, and then by choosing column 2 as the first pivot column. Discuss the relations
> There is a tie for the choice of the first pivot column. Use the simplex method to solve each problem two different ways: first by choosing column 1 as the first pivot column, and then by choosing column 2 as the first pivot column. Discuss the relations
> Solve by the simplex method and also by graphing (the geometric method). Compare and contrast the results.
> first solve the linear programming problem by the simplex method, keeping track of the basic feasible solutions at each step. Then graph the feasible region and illustrate the path to the optimal solution determined by the simplex method.
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method. Repeat Problem 29 with P = 20x1 + 20x2. Data from Problem 29
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method.
> Use the continuous compound interest formula (3) to find each of the indicated values A = $23,600; P = $19,150; t = 60 months; r = ?
> Evaluate the expression. If the answer is not an integer, round to four decimal places. 10P7
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method. Repeat Problem 17 with P = x1 + 2x2. Data from Problem 17:
> Solve the linear programming problems using the simplex method.
> Solve the linear programming problems using the simplex method.
> Evaluate the expression. In how many ways can three variables be chosen from x1, x2, s1, s2, s3, s4, s5, s6, and assigned the value 0?
> Evaluate the expression. In how many ways can three variables be chosen from x1, x2, x3, s1, s2, s3 and assigned the value 0?
> Evaluate the expression.
> Evaluate the expression.
> A linear programming problem has 40 decision variables x1, x2, c , x40 and 85 problem constraints. How many rows are there in the table of basic solutions of the associated e-system? (Write the answer using scientific notation.)
> A linear programming problem has five decision variables x1, x2, x3, x4, x5 and six problem constraints. How many rows are there in the table of basic solutions of the associated e-system?
> Convert the given time period to years, in reduced fraction form, assuming a 360-day year [this assumption does not affect the number of quarters (4), months (12), or weeks (52) in a year]. 90 Days
> Explain why the linear programming problem has an optimal solution, and find it using the table method.
> Explain why the linear programming problem has no optimal solution.
> Explain why the linear programming problem has no optimal solution.
> Solve the given linear programming problem using the table method.
> Solve the given linear programming problem using the table method.
> Solve the given linear programming problem using the table method.
> Solve the given linear programming problem using the table method.
> If a1x1 + a2x2 ≤ b is one of the problem constraints in a standard maximization problem in standard form with two decision variables, and a1 and a2 are both positive, explain why the optimal value of the objective function exists.
> For a standard maximization problem in standard form, with k decision variables, x1, x2,. . xk, explain why the feasible region is not empty.
> Graph the system of inequalities from the given problem, and list the corner points of the feasible region. Verify that the corner points of the feasible region correspond to the basic feasible solutions of the associated e-system Problem 46.
> Use the continuous compound interest formula (3) to find each of the indicated values A = $32,982; P = $27,200; r = 5.93%; t = ?
> Graph the system of inequalities from the given problem, and list the corner points of the feasible region. Verify that the corner points of the feasible region correspond to the basic feasible solutions of the associated e-system Problem 44.
> Graph the system of inequalities from the given problem, and list the corner points of the feasible region. Verify that the corner points of the feasible region correspond to the basic feasible solutions of the associated e-system Problem 42.
> Convert the given i-system to an e-system using slack variables. Then construct a table of all basic solutions of the e-system. For each basic solution, indicate whether or not it is feasible.
> Convert the given i-system to an e-system using slack variables. Then construct a table of all basic solutions of the e-system. For each basic solution, indicate whether or not it is feasible.
> Convert the given i-system to an e-system using slack variables. Then construct a table of all basic solutions of the e-system. For each basic solution, indicate whether or not it is feasible.
> Convert the given i-system to an e-system using slack variables. Then construct a table of all basic solutions of the e-system. For each basic solution, indicate whether or not it is feasible.
> Refer to the partially completed table below of the 10 basic solutions to the e-system Find basic solution (J).
> Refer to the partially completed table below of the 10 basic solutions to the e-system Find basic solution (H).
> Refer to the partially completed table below of the 10 basic solutions to the e-system Which of the basic solutions (A) through (F) are feasible? Explain.
> Refer to the partially completed table below of the 10 basic solutions to the e-system In basic solution (I), which variables are basic?
> Convert the given time period to years, in reduced fraction form, assuming a 360-day year [this assumption does not affect the number of quarters (4), months (12), or weeks (52) in a year]. 9 Months
> Refer to the partially completed table below of the 10 basic solutions to the e-system In basic solution (E), which variables are nonbasic?
> Refer to the table below of the six basic solutions to the e-system Describe geometrically the set of all points in the plane such that s2
> Refer to the table below of the six basic solutions to the e-system Which of the basic solutions are not feasible? Explain.
> Refer to the table below of the six basic solutions to the e-system In basic solution (D), which variables are basic?
> Refer to the table below of the six basic solutions to the e-system In basic solution (B), which variables are nonbasic?
> write the e-system obtained via slack variables for the given linear programming problem.
> write the e-system obtained via slack variables for the given linear programming problem.
> write the e-system obtained via slack variables for the given linear programming problem.
> write the e-system obtained via slack variables for the given linear programming problem.
> Use the continuous compound interest formula (3) to find each of the indicated values
> Refer to the system Find the solution of the system for which x2 = 0, s1 = 0.
> Refer to the system Find the solution of the system for which x1 = 0, s2 = 0
> Graph the constant-cost lines through (9, 9) and (12, 12). Use a straightedge to identify the corner point where the minimum cost occurs. Confirm your answer by constructing a corner-point table.
> G raph the constant-profit lines through (3, 3) and (6, 6) . Use a straightedge to identify the corner point where the maximum profit occurs (see Explore and Discuss 1). Confirm your answer by constructing a corner-point table.
> Graph the constant-profit lines through (3, 3) and (6, 6) . Use a straightedge to identify the corner point where the maximum profit occurs (see Explore and Discuss 1). Confirm your answer by constructing a corner-point table.
> if necessary, review Theorem 1. In Problems 1–4, the feasible region is the set of points on and inside the rectangle with vertices (0, 0), (12, 0), (0, 5), and (12, 5) . Find the maximum and minimum values of the objective function Q o
> if necessary, review Theorem 1. In Problems 1–4, the feasible region is the set of points on and inside the rectangle with vertices (0, 0), (12, 0), (0, 5), and (12, 5) . Find the maximum and minimum values of the objective function Q o
> if necessary, review Theorem 1. In Problems 1–4, the feasible region is the set of points on and inside the rectangle with vertices (0, 0), (12, 0), (0, 5), and (12, 5) . Find the maximum and minimum values of the objective function Q o
> if necessary, review Theorem 1. In Problems 1–4, the feasible region is the set of points on and inside the rectangle with vertices (0, 0), (12, 0), (0, 5), and (12, 5) . Find the maximum and minimum values of the objective function Q o
> A city council voted to conduct a study on inner-city community problems using sociologists and research assistants from a nearby university. Allocation of time and costs per week are given in the table. How many sociologists and how many research assist
> convert the given interest rate to decimal form if it is given as a percentage, and to a percentage if it is given in decimal form. 0,36%
> A laboratory technician in a medical research center is asked to formulate a diet from two commercially packaged foods, food A and food B, for a group of animals. Each ounce of food A contains 8 units of fat, 16 units of carbohydrate, and 2 units of prot
> A dietitian is to arrange a special diet composed of two foods, M and N. Each ounce of food M contains 30 units of calcium, 10 units of iron, 10 units of vitamin A, and 8 units of cholesterol. Each ounce of food N contains 10 units of calcium, 10 units o
> A fast-food chain plans to expand by opening several new restaurants. The chain operates two types of restaurants, drive-through and full-service. A drive through restaurant costs $100,000 to construct, requires 5 employees, and has an expected annual re
> An investor has $24,000 to invest in bonds of AAA and B qualities. The AAA bonds yield an average of 6%, and the B bonds yield 10%. The investor requires that at least three times as much money should be invested in AAA bonds as in B bonds. How much shou
> If each van can transport 7 people and there are 35 available chaperones, show that the optimal solution found graphically involves decimals. Find all feasible solutions with integer coordinates and identify the one that minimizes the transportation cost
> An electronics firm manufactures two types of personal computers—a standard model and a portable model. The production of a standard computer requires a capital expenditure of $400 and 40 hours of labor. The production of a portable computer requires a c
> A furniture manufacturing company manufactures dining-room tables and chairs. The relevant manufacturing data are given in the table below. (A) How many tables and chairs should be manufactured each day to realize a maximum profit? What is the maximum p
> Refer to the bounded feasible region with corner points O = (0, 0) , A = (0, 5), B = (4, 3), and C = (5, 0) that is determined by the system of inequalities. If P = ax + 10y, explain why the minimum value of P cannot occur at B.
> Refer to the bounded feasible region with corner points O = (0, 0) , A = (0, 5), B = (4, 3), and C = (5, 0) that is determined by the system of inequalities. If P = ax + 10y, find all numbers a such that the minimum value of P occurs only at C.
> Refer to the bounded feasible region with corner points O = (0, 0) , A = (0, 5), B = (4, 3), and C = (5, 0) that is determined by the system of inequalities. If P = ax + 10y, find all numbers a such that the maximum value of P occurs at both A and B.
> Use the continuous compound interest formula (3) to find each of the indicated values
> Refer to the bounded feasible region with corner points O = (0, 0) , A = (0, 5), B = (4, 3), and C = (5, 0) that is determined by the system of inequalities. If P = ax + 10y, find all numbers a such that the maximum value of P occurs only at A.
> Explain why Theorem 2 cannot be used to conclude that a maximum or minimum value exists. Graph the feasible regions and use graphs of the objective function z = x - y for various values of z to discuss the existence of a maximum value and a minimum value
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> convert the given interest rate to decimal form if it is given as a percentage, and to a percentage if it is given in decimal form. 0.0019
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Solve the linear programming problems stated in Problems.
> Graph the constant-cost lines through (9, 9) and (12, 12). Use a straightedge to identify the corner point where the minimum cost occurs. Confirm your answer by constructing a corner-point table.
> Solve each system of linear inequalities graphically.
> Match the solution region of each system of linear inequalities with one of the four regions shown in the figure.
> Match the solution region of each system of linear inequalities with one of the four regions shown in the figure.
> If necessary, review Section 1.2. for below Problems refer to the following system of linear inequalities: Is the point (5, 2) in the solution region?
> If necessary, review Section 1.2. for below Problems refer to the following system of linear inequalities: Is the point (5, 3) in the solution region?
> If necessary, review Section 1.2. for below Problems refer to the following system of linear inequalities: Is the point (2, 6) in the solution region?

