Question: The truss ABC shown in the figure
The truss ABC shown in the figure supports a horizontal load P1 = 300 lb and a vertical load P2 = 900 lb. Both bars have a cross-sectional area A = 2.4 in2 and are made of steel with E = 30 × 106 psi.
(a) Determine the strain energy U1 of the truss when the load P1 acts alone (P2 = 0).
(b) Determine the strain energy U2 when the load P2 acts alone (P1 = 0).
(c) Determine the strain energy U3 when both loads act simultaneously.
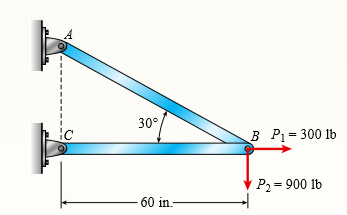
Transcribed Image Text:
30° В Р13300 1b P2 = 900 lb 60 in.
> A prismatic bar is subjected to an axial force that produces a tensile stress σU = 65 MPa and a shear stress τU = 23 MPa on a certain inclined plane (see figure). Determine the stresses acting on all faces of a stress element orient
> Acting on the sides of a stress element cut from a bar in uniaxial stress are tensile stresses of 10,000 psi and 5000 psi, as shown in the figure. (a) Determine the angle u and the shear stress Ï„u, and show all stresses on a sketch of the elem
> Two boards are joined by gluing along a scarf joint, as shown in the figure. For purposes of cutting and gluing, the angle a between the plane of the joint and the faces of the boards must be between 10° and 40°. Under a tensile loa
> A tension member constructed of an L4 × 4 × 1 2 inch angle section is subjected to a tensile load P = 12.5 kips that acts through the point where the mid-lines of the legs intersect (see figure part a). (a) Determine the maxim
> A circular brass bar with a diameter d is member AC in truss ABC that has load P = 5000 lb applied at joint C. Bar AC is composed of two segments brazed together on a plane pq, making an angle α = 36° with the axis of the bar (se
> A copper bar of rectangular cross section (b = 18 mm and h = 40 mm) is held snugly (but without any initial stress) between rigid supports (see figure). The allowable stresses on the inclined plane pq at mids pan, for which θ = 55°
> A plastic bar of rectangular cross section (b = 1.5 in. and h = 3 in.) fits snugly between rigid supports at room temperature (68°F) but with no initial stress (see figure). When the temperature of the bar is raised to 160° F, the c
> A plastic bar of diameter d = 32 mm is compressed in a testing device by a force P = 190 N that is applied as shown in the figure. (a) Determine the normal and shear stresses acting on all faces of stress elements oriented at (1) an angle θ
> The plane truss in the figure is assembled from steel C 10 × 20 shapes. Assume that L = 10 ft and b = 0.71 L. (a) If load variable P = 49 kips, what is the maximum shear stress τmax in each truss member? (b) What is the maximum pe
> A prismatic bar with a length L = 1 m and cross-sectional area A = 1200mm2 is supported at the ends. The bar is then subjected to a temperature increase of ∆T = 25° C. Calculate the complete state of stress acting on an incli
> A prismatic bar with a length L = 3 ft and cross-sectional area A = 8 in2 is compressed by an axial centroidal load P = 10 kips. Determine the complete state of stress acting on an inclined section pq that is cut through the bar at an angle θ
> A copper bar with a rectangular cross section is held without stress between rigid supports (see figure). Subsequently, the temperature of the bar is raised 50° C. (a) Determine the stresses on all faces of the elements A and B, and show these
> During a tension test of a mild-steel specimen (see figure), the extensometer shows an elongation of 0.00120 in. with a gage length of 2 in. Assume that the steel is stressed below the proportional limit and that the modulus of elasticity E = 30 Ã
> A steel bar with a diameter d = 12 mm is subjected to a tensile load P = 9.5 kN (see figure). (a) What is the maximum normal stress σmax in the bar? (b) What is the maximum shear stress τmax? (c) Draw a stress element oriented at 45
> A short column with a wide-flange shape is subjected to a compressive load that produces a resultant force P = 55 kN acting at the midpoint of one flange (see figure). (a) Determine the maximum tensile and compressive stresses σt and Ï
> A brass wire of diameter d = 1/16 in. is stretched between rigid supports with an initial tension T of 37 lb (see figure). Assume that the coefficient of thermal expansion is 10.6 × 10-6/°F and the modulus of elasticity is 15 × 106 psi. (a) If the temper
> A brass wire of diameter d = 2.42 mm is stretched tightly between rigid supports so that the tensile force is T = 98 N (see figure). The coefficient of thermal expansion for the wire is 19.5 × 10-6/°C, and the modulus of elasticit
> A standard brick (dimensions 8 in. × 4 in. × 2.5 in.) is compressed lengthwise by a force P, as shown in the figure. If the ultimate shear stress for brick is 1200 psi and the ultimate compressive stress is 3600 psi, what force
> A circular steel rod of diameter d is subjected to a tensile force P = 3.5 kN (see figure). The allowable stresses in tension and shear are 118 MPa and 48 MPa, respectively. What is the minimum permissible diameter dmin of the rod? P P = 3.5 kN
> A steel bar of rectangular cross section (1.5 in. × 2.0 in.) carries a tensile load P (see figure). The allowable stresses in tension and shear are 14,500 psi and 7,100 psi, respectively. Determine the maximum permissible load Pmax. 2.0
> The device shown in the figure consists of a prismatic rigid pointer ABC supported by a uniform translational spring of stiffness k = 950 N/m. The spring is positioned at distance b = 165 mm from the pinned end A of the pointer. The device is adjusted so
> A safety valve on the top of a tank containing steam under pressure p has a discharge hole of diameter d (see figure). The valve is designed to release the steam when the pressure reaches the value pmax. If the natural length of the spring is L and its s
> A device consists of a horizontal beam ABC supported by two vertical bars BD and CE. Bar CE is pinned at both ends but bar BD is fixed to the foundation at its lower end. The distance from A to B is 600 mm and from B to C is 350 mm. Bars BD and CE have l
> Rigid bar ACB is supported by an elastic circular strut DC having an outer diameter of 15 in. and inner diameter of 14.4 in. The strut is made of steel with a modulus elasticity of E = 29,000 ksi. Point load P = 5 kips is applied at B. Calculate the chan
> By what distance h does the cage shown in the figure move downward when the weight W is placed inside it? (See the figure.) Consider only the effects of the stretching of the cable, which has axial rigidity EA = 10,700 kN. The pulley at A has a diameter
> A short column constructed of a W12 × 35 wide-flange shape is subjected to a resultant compressive load P = 25 k having its line of action at the midpoint of one flange (see figure). (a) Determine the maximum tensile and compressive stresses
> A steel wire and an aluminum alloy wire have equal lengths and support equal loads P (see figure). The moduli of elasticity for the steel and aluminum alloy are Es = 30,000 ksi and Ea = 11,000 ksi, respectively. (a) If the wires have the same diameters,
> A steel cable with a nominal diameter of 25 mm is used in a construction yard to lift a bridge section weighing 38 kN, as shown in the figure. The cable has an effective modulus of elasticity E = 140 GPa. (a) If the cable is 14 m long, how much will it s
> The L-shaped arm ABCD shown in the figure lies in a vertical plane and pivots about a horizontal pin at A. The arm has a constant cross-sectional area and total weight W. A vertical spring of stiffness k supports the arm at point B. (a) Obtain a formula
> Rigid bar ABC is supported with a pin at A and an elastic steel rod at C. The elastic rod has a diameter of 25 mm and modulus of elasticity E = 200 GPa. The bar is subjected to a uniform load q on span AC and a point load at B. Calculate the change in le
> A 10-ft rigid bar AB is supported with a vertical translational spring at A and a pin at B. The bar is subjected to a linearly varying distributed load with maximum intensity qo. Calculate the vertical deformation of the spring if the spring constant is
> Find expressions for all support reaction forces in the plane frame with load 3P applied at C as shown in the figure. Joints A and D are pin supported, and there is a roller support at joint F. The lengths and the properties of the members are shown in t
> A trimetallic bar is uniformly compressed by an axial force P = 9 kips applied through a rigid end plate (see figure). The bar consists of a circular steel core surrounded by brass and copper tubes. The steel core has a diameter of 1.25 in., the brass tu
> Find expressions for all support reaction forces in the plane frame with load 2P applied at C, as shown in the figure. Joint A is a sliding support, joint D is pinned, and joint F is a roller support. Assume that member AC is a flat prismatic bar of leng
> A rigid bar AB of a length L = 66 in is hinged to a support at A and supported by two vertical wires attached at points C and D (see figure). Both wires have the same cross-sectional area (A = 0 .0272 in2 ) and are made of the same material (modulus E =
> A rigid bar ABCD is pinned at point B and supported by springs at A and D (see figure). The springs at A and D have stiffnesses k1 = 10 kN/m and k2 = 25 kN/m, respectively, and the dimensions a, b, and c are 250 mm, 500 mm, and 200 mm, respectively. A lo
> A bar AB supports a load P acting at the centroid of the end cross section (see figure). In the middle region of the bar the cross-sectional area is reduced by removing one-half of the bar. (a) If the end cross sections of the bar are square with sides o
> A horizontal rigid bar of weight W = 7200 lb is supported by three slender circular rods that are equally spaced (see figure). The two outer rods are made of aluminum (E1 = 10 × 106 psi)with diameter d1 = 0.4 in and length L1 = 40 in. The in
> Three-bar truss ABC (see figure) is constructed of steel pipes having a cross-sectional area A = 3500 mm2 and a modulus of elasticity E = 210 GPa. Member BC is of length L = 2.5 m, and the angle between members AC and AB is known to be 60°. Me
> A bimetallic bar (or composite bar) of square cross section with dimensions 2b × 2b is constructed of two different metals having moduli of elasticity E1 and E2 (see figure). The two parts of the bar have the same cross-sectional dimensions.
> A rigid bar of weight W = 800 N hangs from three equally spaced vertical wires (length L = 150 mm, spacing a = 50 mm): two of steel and one of aluminum. The wires also support a load P acting on the bar. The diameter of the steel wires is ds = 2 mm, and
> The aluminum and steel pipes shown in the figure are fastened to rigid supports at ends A and B and to a rigid plate C at their junction. The aluminum pipe is twice as long as the steel pipe. Two equal and symmetrically placed loads P act on the plate at
> A hollow circular pipe (see figure) supports a load P that is uniformly distributed around a cap plate at the top of the lower pipe. The inner and outer diameters of the upper and lower parts of the pipe are d1 = 50 mm, d2 = 60 mm, d3 = 57 mm, and d4 = 6
> A bungee cord that behaves linearly elastically has an unstressed length Lo = 760 mm and a stiffness k = 140 N/m. The cord is attached to two pegs, distance b = 380 mm apart, and is pulled at its midpoint by a force P = 80 N (see figure). (a) How much st
> A block B is pushed against three springs by a force P (see figure). The middle spring has a stiffness k1, and the outer springs each have stiffness k2. Initially, the springs are unstressed, and the middle spring is longer than the outer springs (the di
> A compressive load P is transmitted through a rigid plate to three magnesium-alloy bars that are identical except that initially the middle bar is slightly shorter than the other bars (see figure). The dimensions and properties of the assembly are as fol
> A slightly tapered bar AB of rectangular cross section and length L is acted upon by a force P (see figure). The width of the bar varies uniformly from b2 at end A to b1 at end B. The thickness t is constant. (a) Determine the strain energy U of the bar.
> Two cables, each carrying a tensile force P = 1200 lb, are bolted to a block of steel (see figure). The block has thickness t = 1 in and width b = 3 in. (a) If the diameter d of the cable is 0.25 in., what are the maximum tensile and compressive stresses
> The statically indeterminate structure shown in the figure consists of a horizontal rigid bar AB supported by five equally spaced springs. Springs 1, 2, and 3 have stiffnesses 3k, 1.5k, and k, respectively. When unstressed, the lower ends of all five spr
> The truss ABC shown in the figure is subjected to a horizontal load P at joint B. The two bars are identical with cross-sectional area A and modulus of elasticity E. (a) Determine the strain energy U of the truss if the angle β = 60Â&de
> Determine the strain energy per unit volume (units of psi) and the strain energy per unit weight (units of in.) that can be stored in each of the materials listed in the accompanying table, assuming that the material is stressed to the proportional limit
> The bar ABC shown in the figure is loaded by a force P acting at end C and by a force Q acting at the midpoint B. The bar has a constant axial rigidity EA. (a) Determine the strain energy U1 of the bar when the force P acts alone (Q = 0). (b) Determine t
> A three-story steel column in a building supports roof and floor loads as shown in the figure. The story height H is 10.5 ft, the cross-sectional area A of the column is 15.5 in2, and the modulus of elasticity E of the steel is 30 × 106 psi.
> A bar with a circular cross section having two different diameters d and 2d is shown in the figure. The length of each segment of the bar is L/2, and the modulus of elasticity of the material is E. (a) Obtain a formula for the strain energy U of the bar
> A prismatic bar AD of length L, cross- sectional area A, and modulus of elasticity E is subjected to loads 5P, 3P, and P acting at points B, C, and D, respectively (see figure). Segments AB, BC, and CD have lengths L/6, L/2, and L/3, respectively. (a) Ob
> A tube structure is acted on by loads at B and D, as shown in the figure. The tubes are joined using two flange plates at C that are bolted together using six 0.5-in. diameter bolts. (a) Derive formulas for the reactions RA and RE at the ends of the bar.
> The fixed-end bar ABCD consists of three prismatic segments, as shown in the figure. The end segments have a cross-sectional area A1 = 840 mm2 and length L1 = 200 mm. The middle segment has a cross-sectional area A2 = 1260 mm2 and length L2 = 250 mm. Loa
> A circular post, a rectangular post, and a post of cruciform cross section are each compressed by loads that produce a resultant force P acting at the edge of the cross section (see figure). The diameter of the circular post and the depths of the rectang
> A prismatic bar with a diameter do = 20 mm is being compared with a stepped bar of the same diameter (d1 = 2 0mm) that is enlarged in the middle region to a diameter d2 = 25 mm (see figure). The radius of the fillets in the stepped bar is 2.0 mm. (a) Doe
> Three steel cables jointly support a load of 12 kips (see figure). The diameter of the middle cable is 3/4 in. and the diameter of each outer cable is 1/2 in. The tensions in the cables are adjusted so that each cable carries one-third of the load (i.e.,
> A plastic rod AB of length L = 0.5 m has a diameter d1 = 30 mm (see figure). A plastic sleeve CD of length c = 0.3 m and outer diameter d2 = 45 mm is securely bonded to the rod so that no slippage can occur between the rod and the sleeve. The rod is made
> Repeat Problem 8, but assume that the bar is made of aluminum alloy and that BC is prismatic. Assume that P = 20 kips, L = 3 ft, t = 1/4 in, b1 = 2 in, b2 = 2.5 in., and E = 10,400 ksi. Data from Problem 8: Bar ABC is fixed at both ends (see figure) a
> Bar ABC is fixed at both ends (see figure) and has load P applied at B. Find reactions at A and C and displacement δB if P = 200 kN, L = 2 m, t = 20 mm, b1 = 100 mm, b2 = 115 mm, and E = 96 GPa. b2 3L/5 2L/5 B P A C
> Three prismatic bars, two of material A and one of material B, transmit a tensile load P (see figure). The two outer bars (material A) are identical. The cross-sectional area of the middle bar (material B) is 50% larger than the cross-sectional area of o
> A solid circular steel cylinder S is encased in a hollow circular aluminum tube A. The cylinder and tube are compressed between the rigid plates of a testing machine which applies forces P. Calculate the allowable value of the compressive force if the yi
> A horizontal rigid bar ABC is pinned at end A and supported by two cables at points B and C. A vertical load P = 10 kN acts at end C of the bar. The two cables are made of steel with a modulus elasticity E = 200 GPa and have the same cross- sectional are
> A steel bar with a uniform cross section is fixed at both ends. A load P = 2.5 kips is applied at point C. The bar has a cross-sectional area of 8 in2. Calculate the reactions at joints A and B and the displacement at joint C. Assume that the modulus of
> A cylindrical assembly consisting of a brass core and an aluminum collar is compressed by a load P (see figure). The length of the aluminum collar and brass core is 350 mm, the diameter of the core is 25 mm, and the outside diameter of the collar is 40 m
> A plain concrete wall (i.e., a wall with no steel reinforcement) rests on a secure foundation and serves as a small dam on a creek (see figure). The height of the wall is h = 6.0 ft and the thickness of the wall is t = 1.0 ft. (a) Determine the maximum t
> The assembly shown in the figure consists of a brass core (diameter d1 = 0.25 in) surrounded by a steel shell (inner diameter d2 = 0.28 in, outer diameter d3 = 0.35 in). A load P compresses the core and shell that both have a length L = 4.0 in. The modul
> Repeat Problem 29 if vertical load P at D is replaced by a horizontal load P at D (see figure). Data from Problem 29: A T-frame structure is composed of prismatic beam ABC and non prismatic column DBF that are joined at B by a frictionless pin connect
> A T-frame structure is composed of prismatic beam ABC and non prismatic column DBF that are joined at B by a frictionless pin connection. The beam has a sliding support at A and the column is pin supported at F (see figure). Beam ABC and column segment D
> A T-frame structure is composed of a prismatic beam ABC and a non prismatic column DBF. The beam and the column have a pin support at A and D, respectively. Both members are connected with a pin at B. The lengths and properties of the members are shown i
> A vertical steel bar ABC is pin-supported at its upper end and loaded by a force P1 at its lower end. A horizontal beam BDE is pinned to the vertical bar at joint B and supported at point D. Load P2 and moment M are applied at end E. Calculate the vertic
> A uniformly tapered tube AB of circular cross section and length L is shown in the figure. The average diameters at the ends are dA and dB = 2dA. Assume E is constant. Find the elongation δ of the tube when it is subjected to loads P acting
> A post AB supporting equipment in a laboratory is tapered uniformly throughout its height H (see figure). The cross sections of the post are square, with dimensions b × b at the top and 1.5 b × 1.5 b at the base. Derive a formu
> A rigid bar AB having a mass M = 1.0 kg and length L = 0.5 m is hinged at end A and supported at end B by a nylon cord BC (see figure). The cord has cross-sectional area A = 30 mm2, length b = 0.25 m, and modulus of elasticity E = 2.1 GPa. If the bar is
> A flying buttress transmits a load P = 25 kN, acting at an angle of 60° to the horizontal, to the top of a vertical buttress AB (see figure). The vertical buttress has height h = 5.0 m and rectangular cross section of thickness t = 1.5 m and w
> A weight W rests on top of a wall and is attached to one end of a very flexible cord having cross-sectional area A and modulus of elasticity E (see figure). The other end of the cord is attached securely to the wall. The weight is then pushed off the wal
> A bungee jumper having a mass of 55 kg leaps from a bridge, braking her fall with a long elastic shock cord having axial rigidity EA = 2.3 kN (see figure). If the jump off point is 60 m above the water, and if it is desired to maintain a clearance of 10
> A bumper for a mine car is constructed with a spring of stiffness k = 1120 lb/in. (see figure). If a car weighing 3450 lb is traveling at velocity v = 7 mph when it strikes the spring, what is the maximum shortening of the spring?
> A bumping post at the end of a track in a railway yard has a spring constant k = 8.0 MN/m (see figure). The maximum possible displacement δ of the end of the striking plate is 450 mm. What is the maximum velocity vmax that a railway car of w
> Solve the preceding problem if the slider has W = 100 lb, h = 45 in., A= 0.080 in2, E = 21 × 106 psi, and the allowable stress is 70 ksi. Data from Problem 8: A cable with a restrainer at the bottom hangs vertically from its upper end (se
> A cable with a restrainer at the bottom hangs vertically from its upper end (see figure). The cable has an effective cross-sectional area A = 40mm2 and an effective modulus of elasticity E = 130 GPa. A slider of mass M = 35 kg drops from a height h = 1.0
> A weight W = 4500 lb falls from a height h onto a vertical wood pole having length L = 15 ft, diameter d = 12 in., and modulus of elasticity E = 1.6 × 106 psi (see figure). If the allowable stress in the wood under an impact load is 2500 psi
> A small rubber ball (weight W = 450 Nm) is attached by a rubber cord to a wood paddle (see figure). The natural length of the cord is Lo = 200 mm, its cross-sectional area is A = 1.6 mm2, and its modulus of elasticity is E = 2.0 MPa. After being struck b
> Solve the preceding problem for W = 1.0 lb, h = 12 in., and k = 0.5 lb/in. Data from Problem 4: A block weighing W = 5.0 N drops inside a cylinder from a height h = 200 mm onto a spring having stiffness k = 90 N/m (see figure). (a) Determine the maxim
> A block weighing W = 5.0 N drops inside a cylinder from a height h = 200 mm onto a spring having stiffness k = 90 N/m (see figure). (a) Determine the maximum shortening of the spring due to the impact and (b) determine the impact factor. Block Cylin
> A cylindrical brick chimney of height H weighs w = 825 lb/ft of height (see figure). The inner and outer diameters are d1 = 3 ft and d2 = 4 ft, respectively. The wind pressure against the side of the chimney is p = 10 lb/ft2 of projected area. Determine
> Solve Problem 1 if the collar has weight W = 50 lb, the height h = 2.0 in., the length L = 3.0 ft, the cross-sectional area A = 0.25 in2, and the modulus of elasticity E = 30,000 ksi. Data from Problem 1: A sliding collar of weight W = 150 lb falls fr
> Solve the preceding problem if the collar has mass M = 80 kg, the height h = 0.5 m, the length L = 3.0 m, the cross-sectional area A = 350mm2, and the modulus of elasticity E = 170 GPa. Data from Problem 1: A sliding collar of weight W = 150 lb falls
> A sliding collar of weight W = 150 lb falls from a height h = 2.0 in. onto a flange at the bottom of a slender vertical rod (see figure). The rod has a length L = 4.0 ft, cross-sectional area A = 0.75 in2, and modulus of elasticity E = 30 ×
> A long, slender bar in the shape of a right circular cone with length L and base diameter d hangs vertically under the action of its own weight (see figure). The weight of the cone is W and the modulus of elasticity of the material is E. Derive a formula
> A circular aluminum alloy bar of length L = 1.8 m has a slot in the middle half of its length (see figure). The bar has a radius 36 mm r 5 and modulus of elasticity E = 72 GPa. The slot has a height 2a = r/4. Calculate the elongation of the bar due to fo
> A slightly tapered bar AB of solid circular cross section and length L is supported at end B and subjected to a tensile load P at the free end A. The diameters of the bar at ends A and B are dA and dB, respectively. Determine the length of the bar if the
> Repeat Problem 18, but assume that the bar is made of aluminum alloy. If P2 = 200 kN, what is P1 so that displacement δC = 0? What is displacement δB? Assume that L = 2 m, t = 20 mm, b1 = 100 mm, b2 = 115 mm, and E = 72 GPa. D
> Repeat Problem 18, but assume that the bar is made of copper alloy. Calculate the displacements δB and δC if P = 50 kips, L = 5 ft, t = 3/8 in., b1 = 2.75 in, b2 = 3in., and E = 16,000 ksi. Data from Problem 18: A flat brass
> A flat brass bar has length L, constant thickness t, and a rectangular cross section whose width varies linearly between b2 at the fixed support to b1 at the free end (see figure). Assume that the taper of the bar is small. The bar has modulus of elastic
> A steel bracket of solid circular cross section is subjected to two loads, each of which is P = 4.5 kN at D (see figure). Let the dimension variable be b = 240 mm. (a) Find the minimum permissible diameter dmin of the bracket if the allowable normal stre
> A prismatic bar AB of length L, cross- sectional area A, modulus of elasticity E, and weight W hangs vertically under its own weight (see figure). (a) Derive a formula for the downward displacement δC of point C, located at distance h from t

