Question: The velocity function (in meters per second)
The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval.
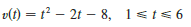
> Evaluate the indefinite integral. f x(2x + 5)8 dx
> Evaluate the indefinite integral. f x2 √2 + x dx
> Evaluate the integral by making the given substitution. f x2 √x3 + 1 dx, u = x3 + 1
> Evaluate the indefinite integral. f sec3x tan x dx
> Evaluate the indefinite integral. f dt/cos2t√1 + tan t
> Evaluate the indefinite integral. f dx/√1 – x2, sin-1x
> Find the area bounded by the loop of the curve with parametric equations x = t2, y – t3 – 3t.
> Evaluate the indefinite integral. f cos (π/x)/x2 dx
> Investigate the family of curves given by f (x) = x4 + x3 + cx2 In particular you should determine the transitional value of c at which the number of critical numbers changes and the transitional value at which the number of inflection points changes. Il
> Evaluate the indefinite integral. f √cot x, csc2x dx
> Evaluate the indefinite integral. f sin (ln x)/x dx
> Evaluate the indefinite integral. f (x2 + 1) (x3 + 3x)4 dx
> Evaluate the indefinite integral. f tan-1x/1 + x2 dx
> Evaluate the indefinite integral. f cos x/sin2x, dx
> Evaluate the indefinite integral. f sec 2θ tan 2θ dθ
> Evaluate the integral by making the given substitution. f x3 (2 + x4)5 dx, u = 2 + x
> Evaluate the indefinite integral. f ex √1 + ex dx
> Evaluate the indefinite integral. f z2/z3 + 1 dz
> A vertical plate is submerged (or partially submerged) in water and has the indicated shape. Explain how to approximate the hydrostatic force against one side of the plate by a Riemann sum. Then express the force as an integral and evaluate it.
> (a). If f (x) = 0.1 ex + sin x, -4 < x < 4 use a graph of f to sketch a rough graph of the antiderivative F of f that satisfies F (0) = 0. (b). Find an expression for F (x). (c). Graph F using the expression in part (b). Compare with your sketch in part
> Evaluate the indefinite integral. f a + bx2 / √3ax + bx3 dx
> Evaluate the indefinite integral. f sin √x/ √x dx
> Evaluate the indefinite integral. f dx/ 5 – 3x
> Evaluate the indefinite integral. f x/ (x2 + 1)2 dx
> Evaluate the indefinite integral. f (ln x)2/x dx
> Evaluate the indefinite integral. f ex cos (ex) dx
> Evaluate the indefinite integral. f sin πt dt
> Evaluate the indefinite integral. f (3t + 2)2.4 dt
> Evaluate the integral by making the given substitution. f e-x dx, u = -x
> Use Part 1 of the Fundamental Theorem of Calculus to find the derivative of the function.
> (a). How would you evaluate fx5e-2x dx by hand? (Don’t actually carry out the integration.) (b). How would you evaluate fx5e-2x dx using tables? (Don’t actually do it.) (c). Use a CAS to evaluate fx5e-2x dx. (d). Graph the integrand and the indefinite in
> A vertical plate is submerged (or partially submerged) in water and has the indicated shape. Explain how to approximate the hydrostatic force against one side of the plate by a Riemann sum. Then express the force as an integral and evaluate it.
> (a). Explain why the function whose graph is shown is a probability density function. (b). Use the graph to find the following probabilities: (c). Calculate the mean.
> Use Part 1 of the Fundamental Theorem of Calculus to find the derivative of the function.
> (a). Show that for cos (x2) > cos x for 0 < x < 1. (b). Deduce that for fπ/60 cos (x2) dx > 1/2.
> (a) Show that 1 < √1 + x3 < 1 + x3 for x > 0. (a) Show that 1 < f10 √1 + x3 dx < 1.25.
> A car dealer sells a new car for $18000. He also offers to sell the same car for payments of $375 per month for five years. What monthly interest rate is this dealer charging? To solve this problem, you will need to use the formula for the present value
> Use Part 1 of the Fundamental Theorem of Calculus to find the derivative of the function.
> In the figure, the length of the chord AB is 4 cm and the length of the arc AB is 5 cm. Find the central angle θ, in radians, correct to four decimal places. Then give the answer to the nearest degree.
> Water flows into and out of a storage tank. A graph of the rate of change r (t) of the volume of water in the tank, in liters per day, is shown. If the amount of water in the tank at time t = 0 is 25,000 L, use the Midpoint Rule to estimate the amount of
> The marginal cost of manufacturing x yards of a certain fabric is C'(x) = 3 – 0.01x + 0.000006x2 (in dollars per yard). Find the increase in cost if the production level is raised from 2000 yards to 4000 yards.
> (a). If f (x) = sin (sin x), use a graph to find an upper bound for f(4) (x). (b). Use Simpson’s Rule with n = 10 to approximate fπ0f (x) dx and use part (a) to estimate the error. (c). How large should n be to guarantee that the size of the error Sn in
> Suppose that a volcano is erupting and readings of the rate r (t) at which solid materials are spewed into the atmosphere are given in the table. The time is measured in seconds and the units for r (t) are tonnes (metric tons) per second. (a). Give upp
> The velocity of a car was read from its speedometer at 10-second intervals and recorded in the table. Use the Midpoint Rule to estimate the distance traveled by the car.
> A vertical plate is submerged (or partially submerged) in water and has the indicated shape. Explain how to approximate the hydrostatic force against one side of the plate by a Riemann sum. Then express the force as an integral and evaluate it.
> Water flows from the bottom of a storage tank at a rate of r (t) = 200 – 4t liters per minute, where 0 < t < 50. Find the amount of water that flows from the tank during the first 10 minutes.
> The linear density of a rod of length 4 m is given by ρ (x) = 9 + 2√x measured in kilograms per meter, where is measured in meters from one end of the rod. Find the total mass of the rod.
> The acceleration function (in m/s2) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time and (b) the distance traveled during the given time interval.
> The acceleration function (in m/s2) and the initial velocity are given for a particle moving along a line. Find (a) the velocity at time and (b) the distance traveled during the given time interval.
> Sketch the area represented by g (x). Then find g'(x) in two ways: (a) by using Part 1 of the Fundamental Theorem and (b) by evaluating the integral using Part 2 and then differentiating.
> The velocity function (in meters per second) is given for a particle moving along a line. Find (a) the displacement and (b) the distance traveled by the particle during the given time interval.
> A tank is full of water. Find the work required to pump the water out of the spout. In Exercises 21 and 22 use the fact that water weighs 62.5 lb/ft3.
> Use Newton’s method to find all the roots of the equation correct to eight decimal places. Start by drawing a graph to find initial approximations.
> Use Newton’s method to find all roots of the equation correct to six decimal places. 1/x = 1 + x3
> If f (x) is the slope of a trail at a distance of x miles from the start of the trail, what does f35 f (x) dx represent?
> (a). Graph the epitrochoid with equations x = 11 cos t – 4 cos (11t/2), x = 11 sin t – 4 sin (11t/2) What parameter interval gives the complete curve? (b). Use your CAS to find the approximate length of this curve.
> In Section 4.6 we defined the marginal revenue function R'(x) as the derivative of the revenue function R(x), where is the number of units sold. What does f10005000 R'(x) dx represent?
> A honeybee population starts with 100 bees and increases at a rate of n'(t) bees per week. What does 100 + f015 n'(t) dt represent?
> Express the limit as a definite integral
> The current in a wire is defined as the derivative of the charge: I (t) = Q'(t). (See Example 3 in Section 3.8.) What does fab I (t) dt represent?
> Use the properties of integrals to verify that 2 < f-11 √1 + x2 dx < 2 √2
> The boundaries of the shaded region are the y-axis, the line y = 1, and the curve y = 4√x. Find the area of this region by writing x as a function of y and integrating with respect to y
> Estimate the errors involved in Exercise 47, parts (a) and (b). How large should n be in each case to guarantee an error of less than 0.00001? Exercise 47: Use (a) the Trapezoidal Rule, (b) the Midpoint Rule, and (c) Simpson’s Rule wi
> Sketch the area represented by g (x). Then find g'(x) in two ways: (a) by using Part 1 of the Fundamental Theorem and (b) by evaluating the integral using Part 2 and then differentiating.
> The area of the region that lies to the right of the -axis and to the left of the parabola x = 2y – y2 (the shaded region in the figure) is given by the integral f02(2y – y2) dy. (Turn your head clockwise and think of
> Find the general indefinite integral. f sin 2x/sin x, dx
> Find the general indefinite integral. f sin x/1 – sin 2x, dx
> The curves with equations xn + yn = 1, n = 4, 6, 8, . . . , are called fat circles. Graph the curves with n = 2, 4, 6, 8, and 10 to see why. Set up an integral for the length L2k of the fat circle with n = 2k. Without attempting to evaluate this integral
> Find the general indefinite integral. f sec t (sec t + tan t) dt
> Find the general indefinite integral. f (1 + tan2a) da
> Find the general indefinite integral. f v (v2 + 2)2 dv
> Find the general indefinite integral. f (1 – t) (2 + t2) dt
> Find the general indefinite integral. Illustrate by graphing several members of the family on the same screen.
> A manufacturer determines that the cost of making units of a commodity is C (x) = 1800 + 25x – 0.2 x2 + 0.001 x3 and the demand function is p (x) = 48.2 – 0,03x. (a). Graph the cost and revenue functions and use the graphs to estimate the production leve
> Find the general indefinite integral. Illustrate by graphing several members of the family on the same screen.
> Evaluate the integral. f01 (1 + 1/2u4 – 2/5u9) du
> Evaluate the integral and interpret it as a difference of areas. Illustrate with a sketch.
> Evaluate the integral and interpret it as a difference of areas. Illustrate with a sketch.
> Repeat Exercise 35 for the curve y = (x2 + 1)-1 – x4. Exercise 35: Use a graph to estimate the x-intercepts of the curve y = 1 – 2x – 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> A vertical plate is submerged (or partially submerged) in water and has the indicated shape. Explain how to approximate the hydrostatic force against one side of the plate by a Riemann sum. Then express the force as an integral and evaluate it.
> Use a graph to estimate the x-intercepts of the curve y = 1 – 2x – 5x4. Then use this information to estimate the area of the region that lies under the curve and above the x-axis.
> Use a graph to give a rough estimate of the area of the region that lies beneath the given curve. Then find the exact area.
> A manufacturing company owns a major piece of equip ment that depreciates at the (continuous) rate f = f (t), where is the time measured in months since its last overhaul. Because a fixed cost A is incurred each time the machine is overhauled, the compan
> A high-tech company purchases a new computing system whose initial value is V. The system will depreciate at the rate f (t) and will accumulate maintenance costs at the rate g = g (t), where is the time measured in months. The company wants to determine
> A hockey team plays in an arena with a seating capacity of 15,000 spectators. With the ticket price set at $12, average attendance at a game has been 11,000. A market survey indicates that for each dollar the ticket price is lowered, average attendance w
> Find a function f and a number a such that 6 + fxaf (t)/t2 dt = 2√x for all x > 0.
> Let (a). Find an expression for g (x) similar to the one for f (x). (b). Sketch the graphs of f and g. (c). Where f is differentiable? Where is g differentiable?
> Let g (x) = fx0 f(t) dt, where f is the function whose graph is shown. (a). Evaluate g (x), g (1), g (2), g (3), and g (6). (b). On what interval is g increasing? (c). Where does g have a maximum value? (d). Sketch a rough graph of g.
> Find a function f such that f (1) = 0 and f'(x) = 2x/x.
> Evaluate the integral. f02 |2x – 1| dx
> Evaluate the integral. f01/√3 t2 – 1/t4 - 1 dt
> The figure shows graphs of the marginal revenue function R' and the marginal cost function C' for a manufacturer. [Recall from Section 4.6 that R (x) and C (x) represent the revenue and cost when x units are manufactured. Assume that R and C are measured
> TriStateCo, an Idaho corporation, has operated landfills in Washington (WA), Idaho (JD), and Oregon (OR) for the past 20 years. The states are changing their environmental Laws governing landfills, and it is cumbersome for each landfill to meet the req
> Ten years ago, Xio and Xandra each invested $300,000 to create Xava Corporation. Xava develops and manufactures rock climbing and bungee jumping equipment. The business has become very profitable (it now is valued at $3 million), and Xandra would like to
> Calabra, S.A., a Peruvian corporation, manufactures inventory in Peru. The inventory is sold to independent distributors in the United States, with title passing to the purchaser in the United States. Calabra has no employees or operations within the Uni
> CL LLC is a manufacturing business and reported taxable income of $40 million before interest expense, taxes, depreciation, and amortization ("tax EBITDA"), plus $1.5 million of separately stated investment income. CL incurred interest expense of $1 mill
> You are the head tax accountant for Venture Company, a U.S. corporation. The board of directors is considering expansion overseas and asks you to present a summary of the U.S. tax consequences of investing overseas through a foreign subsidiary. Prepare a
> The Giant consolidated group includes SubTwo, which was acquired as part of a § 382 ownership change. SubTwo brought with it to the group a large NOL carryforward, $3 million of which is available this year under the SRLY rules due to SubTwo's positive c
> Browne and Red, both C corporations, formed the BR Partnership on January 1, 2018. Neither Browne nor Red is a personal service corporation, and BR is not a tax shelter. BR's gross receipts were $22 million, $25 million, $31 n1illion, and S32 million, re
> Determine consolidated taxable income for the calendar year Yeti Group, which elected consolidated status immediately upon the creation of the two member corporations on January 1 of year 1. All recognized income is ordinary in nature, and no intercomp

