Question: Two curves are orthogonal if their tangent
Two curves are orthogonal if their tangent lines are perpendicular at each point of intersection. Show that the given families of curves are orthogonal trajectories of each other, that is, every curve in one family is orthogonal to every curve in the other family. Sketch both families of curves on the same axes.
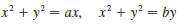
Transcribed Image Text:
x² + y² = ax, x² + y² = by ах,
> (a). Show that f (x) = 2x + cos x is one-to-one. (b). What is the value of f-1(1)? (c). Use the formula from Exercise 41(a) to find (f-1)'(1). Exercise 41(a): (a). Suppose f is a one-to-one differentiable function and its inverse function f-1 is also d
> (a). Suppose f is a one-to-one differentiable function and its inverse function f-1 is also differentiable. Use implicit differentiation to show that (f-1)'(x) = 1/f'(f-1(x)) provided that the denominator is not 0. (b). If f (4) = 5 and f'(4) = 2/3, find
> If f and g are the functions whose graphs are shown, let u (x) = f (x) g (x) and v (x) = f (x) /g (x). (a). Find u'(1) (b). Find v'(5)
> Find the limit. limx→0+ tan-1 (ln x)
> Find the exact value of each expression. (a). tan-1 (tan3π/4) (b). cos (arccos ½)
> Find the limit. limx→∞+ arctan (ex)
> Find the limit. limx→∞ arccos (1 + x2/1 + 2x2)
> Find the limit. Limx→-1+ sin-1 x
> Find f'(x). Check that your answer is reasonable by comparing the graphs of f and f'. f (x) = arctan (x2 -x)
> Find f'(x). Check that your answer is reasonable by comparing the graphs of f and f'. f (x) = √1 - x2 arcsin x
> Find an equation of the tangent line to the curve y = 3 arccos (x/2) at the point (1, π).
> If g (x) = x sin (x/4) + √16 – x2, find g'(2).
> Find y' if tan-1 (xy) = 1 + x2y.
> The figure shows the graphs of four functions. One is the position function of a car, one is the velocity of the car, one is its acceleration, and one is its jerk. Identify each curve, and explain your choices. у. a b
> Find the derivative of the function. Find the domains of the function and its derivative. g (x) = c0s-1 (3 – 2x)
> Find the derivative of the function. Find the domains of the function and its derivative. f (x) = arcsin (ex)
> Find the exact value of each expression. (a). arctan 1 (b). sin-1 (1/√2)
> Find the derivative of the function. Simplify where possible. y = arccos (b + a cos x/a + b cos x), 0 < x < π, a > b > 0
> Find the derivative of the function. Simplify where possible. y = arctan √1-x/1+x
> Find the derivative of the function. Simplify where possible. y = x sin-1 x + √1 – x2
> Find the derivative of the function. Simplify where possible. y = cos-1 (sin-1 t)
> Find the derivative of the function. Simplify where possible. y = arctan (cos θ)
> Find the derivative of the function. Simplify where possible. y = tan-1 (x – √1 + x2)
> Find the derivative of the function. Simplify where possible. y = cos-1(e2x)
> The figure shows the graphs of three functions. One is the position function of a car, one is the velocity of the car, and one is its acceleration. Identify each curve, and explain your choices. y b
> Find the derivative of the function. Simplify where possible. f (x) = x ln (arctan x)
> Find the derivative of the function. Simplify where possible. G (x) = √1 – x2 arccos x
> Find the derivative of the function. Simplify where possible. F (θ) = arcsin √sin θ
> Find the exact value of each expression. (a). tan-1 (1/√3) (b). sec-1 (2)
> Find the derivative of the function. Simplify where possible. y = sin-1 (2x + 1)
> Find the derivative of the function. Simplify where possible. y = tan-1 (x2)
> Find the derivative of the function. Simplify where possible. y = (tan-1 x)2
> (a). Prove that sin-1 x = π/2. (b). Use part (a) to prove Formula 2. sin1x cos1x
> Prove Formula 2 by the same method as for Formula 1.
> Graph the given functions on the same screen. How are these graphs related? y = tan x, - 7/2 < x < m/2; y= tan='x; y = x
> The figure shows graphs of f, f', f'' and f'''. Identify each curve, and explain your choices. a b c d
> Graph the given functions on the same screen. How are these graphs related? y = sin x, -7/2 sIS /2; y= sin-'x; y = x
> Simplify the expression. cos (2 tan-1 x)
> Simplify the expression. sin (tan-1 x)
> Simplify the expression. tan (sin-1 x)
> Find the exact value of each expression. (a). sin-1 (√3/2) (b). cos-1 (-1)
> Find dy/dx by implicit differentiation. x2y2 + x sin y = 4
> Find dy/dx by implicit differentiation. y5 + x2y3 = 1+ yex2
> Find dy/dx by implicit differentiation. x4 (x + y) = y2 (3x – y)
> Find dy/dx by implicit differentiation. 2x3 + x2y – xy3 = 2
> Find dy/dx by implicit differentiation. x2 + xy – y2 = 4
> Two curves are orthogonal if their tangent lines are perpendicular at each point of intersection. Show that the given families of curves are orthogonal trajectories of each other, that is, every curve in one family is orthogonal to every curve in the oth
> Two curves are orthogonal if their tangent lines are perpendicular at each point of intersection. Show that the given families of curves are orthogonal trajectories of each other, that is, every curve in one family is orthogonal to every curve in the oth
> Two curves are orthogonal if their tangent lines are perpendicular at each point of intersection. Show that the given families of curves are orthogonal trajectories of each other, that is, every curve in one family is orthogonal to every curve in the oth
> Find an equation of the tangent line to the curve at the given point. y = (1 + 2x)10, (0, 1)
> Show by implicit differentiation that the tangent to the ellipse x2/a2 + y2/b2 = 1 at the point (x0, y0) is x0x/a2 + y0y/b2 = 1
> Find dy/dx by implicit differentiation. 2√x + √y = 3
> Find y' and y". y = eax sin βx
> Find y' and y". y = cos2x
> Find y' and y". y = cos (x2)
> Find the derivative of the function. y = 23x2
> Zoom in toward the points (1, 0), (0, 1), and (-1, 0) on the graph of the function g (x) = (x2 – 1)2/3. What do you notice? Account for what you see in terms of the differentiability of g.
> A particle moves according to a law of motion s = f (t), t > 0, where is measured in seconds and in feet. (a). Find the velocity at time t. (b). What is the velocity after 3 s? (c). When is the particle at rest? (d). When is the particle moving in the po
> Find the derivative of the function. y = cos √sin (tan πx)
> Find y" by implicit differentiation. x4 + y4 = a4
> Find y" by implicit differentiation. x3 + y3 = 1
> Find y" by implicit differentiation. √x + √y = 1
> Find y" by implicit differentiation. 9x2 + y2 = 9
> Find the derivative of the function. f (t) = √t/t2 + 4
> Find dy/dx by implicit differentiation. x3 + y3 = 1
> (a). The curve with equation y2 = 5x4 – x2 is called a kampyle of Eudoxus. Find an equation of the tangent line to this curve at the point (1, 2). (b). Illustrate part (a) by graphing the curve and the tangent line on a common screen. (If your graphing d
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. у? - 4) — х"({? - 5) К0, —2) (devil's curve) y
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. 2(x? + y?)? = 25(x² – y²) (3, 1) (lemniscate) y4
> Find the points on the lemniscate in Exercise 27 where the tangent is horizontal. Exercise 27: Use implicit differentiation to find an equation of the tangent line to the curve at the given point. 2(x? + y?)? = 25(x² – y²) (3, 1) (lemniscate) y4
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. x2/3 + y2/3 = 4 (-3 √3, 1) (astroid) 8
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. x2 + y2 = (2x2 + 2y2) – x)2 (0, 1 /2) (cardioid) y.
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. x² + 2xy – y² + x = 2, (1, 2) (hyperbola)
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. x² + xy + y? = 3, (1, 1) (ellipse)
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. sin(x + y) = 2x – 2y, (7, 7) %3D
> Use implicit differentiation to find an equation of the tangent line to the curve at the given point. y sin 2x = x cos 2y, (7/2, 7/4)
> Regard as the independent variable and as the dependent variable and use implicit differentiation to find dx/dy. y sec x = x tan y
> Write the composite function in the form f (g (x)). [Identify the inner function u = g (x) and the outer function y = f (u).] Then find the derivative dy/dx. y = (2x3 + 5)4
> Regard as the independent variable and as the dependent variable and use implicit differentiation to find dx/dy. x4y2 - x3y + 2xy3 = 0
> If g (x) + x sin g (x) = x2, find g'(0).
> (a). The curve with equation has been likened to a bouncing wagon. Use a computer algebra system to graph this curve and discover why. (b). At how many points does this curve have horizontal tangent lines? Find the x-coordinates of these points.
> If f (x) + x2 [f (x)]3 = 10 and f (1) = 2, find f'(1).
> Find dy/dx by implicit differentiation. sin x + cos y = sin x cos y
> Find dy/dx by implicit differentiation. ey cos x = 1 + sin (xy)
> Find dy/dx by implicit differentiation. tan (x – y) = y/1 + xy2
> Find dy/dx by implicit differentiation. ex/y = x - y
> Find dy/dx by implicit differentiation. y sin (x2) = x sin (y2)
> Find dy/dx by implicit differentiation. 4 cos x sin y = 4
> Find dy/dx by implicit differentiation. 1 + x = sin (xy2)
> (a). Find y' by implicit differentiation. (b). Solve the equation explicitly for and differentiate to get y' in terms of x. (c). Check that your solutions to parts (a) and (b) are consistent by substituting the expression for into your solution for part
> Find the derivative of the function. F (x) = √1 - 2x
> Fanciful shapes can be created by using the implicit plotting capabilities of computer algebra systems. (a). Graph the curve with equation At, how many points does this curve have horizontal tangents? Estimate the -coordinates of these points. (b). Fin
> Find the derivative of the function. F (x) = (4x - x2)100
> Find the derivative of the function. F (x) = (x4 + 3x2 – 2)5
> Write the composite function in the form f (g (x)). [Identify the inner function u = g (x) and the outer function y = f (u).] Then find the derivative dy/dx. y = √2 - ex
> Find R'(0), R(x) = x + 3x3 + 5x5/1 + 3x3 + 6x6 + 9x9 where Hint: Instead of finding R’(x) first, let f (x) be the numerator and g (x) the denominator of R (x) and compute R' (0) from f (0), f' (0), g (0), and g' (0).
> Let f and be the functions in Exercise 53. Exercise 53: A table of values for f, g, f', and g' is given. (a). If F (x) = f (f (x)), find F'(2). (b). If G (x) = g (g (x)), find G'(3).
> A table of values for f, g, f', and g' is given. (a). If h (x) = f (g (x)), find h'(1). (b). If H (x) = g (f (x)), find H'(1).
> Find the x-coordinates of all points on the curve y = sin 2x – 2 sin x at which the tangent line is horizontal.
> Write the composite function in the form f (g (x)). [Identify the inner function u = g (x) and the outer function y = f (u).] Then find the derivative dy/dx. y = e√x
> (a). If f (x) = x√2 – x2, find f'(x). (b). Check to see that your answer to part (a) is reasonable by comparing the graphs of f and f'.

