Question: Using your results from Problem 4.8,
Using your results from Problem 4.8, find the heat flow at the base of the fin for the following conditions.
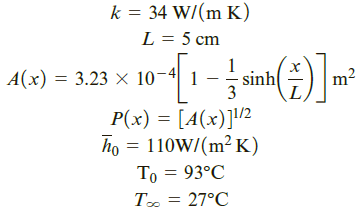
Use a grid spacing of 0.5 cm.
> A thin, flat plate 15 cm square is tested for drag in a wind tunnel with air at 30 m/s, 100 kPa (abs), and 16°C flowing across and parallel to the top and bottom surfaces. The observed total drag force is 0.06 N. Using the definition of friction coeffici
> It has been proposed to tow icebergs from the polar region to the Middle East in order to supply potable water to arid regions there. A typical iceberg suitable for towing should be relatively broad and flat. Consider an iceberg 0.25 km thick and 1 km sq
> A spherical interplanetary probe with a 30-cm diameter contains electronic equipment that dissipates 100 W. If the probe surface has an emissivity of 0.8, what is its surface temperature in outer space? State your assumptions in the calculations.
> The wing of an airplane has a polished aluminum skin. At a 1500 m altitude, it absorbs 100 W/m2 by solar radiation. Assuming that the interior surface of the wing’s skin is well insulated and the wing has a chord of 6-m length (i.e., L
> Twenty-five square computer chips, each 10 mm * 10 mm in size and 1 mm thick, are mounted 1 mm apart on an insulating plastic substrate as shown below. The chips are to be cooled by nitrogen flowing along the length of the row at -40°C and atm
> The air-conditioning system in a Chevrolet van for use in desert climates is to be sized. The system is to maintain an interior temperature of 20°C when the van travels at 100 km/h through dry air at 30°C at night. If the top of th
> At the equator, where the sun is approximately overhead at noon, a near optimum orientation for a flat-plate solar hot-water heater is in the horizontal position. Suppose a 4-m ï€ªï€ 4-m solar collector for domestic hot wate
> A refrigeration truck is traveling at 130 km/h on a desert highway where the air temperature is 50°C. The body of the truck is idealized as a rectangular box 3 m wide, 2.1 m high, and 6 m long, at a surface temperature 10°C. Assume
> Air at 320 K with a free-stream velocity of 10 m/s is used to cool small electronic devices mounted on a printed circuit board as shown in the sketch. Each device is 5 mm * 5 mm square in planform and dissipates 60 mW. A turbulator is located at the lead
> A flat-plate heat exchanger operates in a nitrogen atmosphere at a pressure of about 104 N/m2 and 38°C. The flat-plate heat exchanger was originally designed to operate in air at 1 atm and 38°C turbulent flow. Estimate the ratio of the heat transfer coef
> In a sheet-metal rolling and stamping factory, a 2.5- mm thick strip of stainless steel is hot rolled, as is schematically depicted in the figure. The strip emerges from the furnace and the rolling mill with a temperature of 900°C with a speed
> Evaluate the Stanton number for flow over a tube from the following data /
> In a manufacturing operation, a long strip of sheet metal is transported on a conveyor at a velocity of 2 m/s while a coating on its top surface is to be cured by radiant heating. Suppose that infrared lamps mounted above the conveyor provide a radiant f
> The sun has a radius of 7 * 108 m and approximates a blackbody with a surface temperature of about 5800 K. Calculate the total rate of radiation from the sun and the emitted radiation flux per square meter of surface area.
> A 2.5-cm-diameter, 15-cm-long transit rod (k = 0.97 on the end of a 2.5-cm-diameter wood rod at a uniform temperature of 100°C is suddenly placed into a 16°C, 30 m/s air stream flowing parallel to the axis of the rod. Estimate the
> The six identical aluminum fins shown in the sketch are attached to an electronic device for cooling. Cooling air is available at a velocity of 5 m/s from a fan at 20°C. If the average temperature at the base of a fin is not to exceed 100&Acir
> An electronic device is to be cooled by air flowing over aluminum fins attached to lower surface as shown. The device dissipates 5 W, and the thermal contact resistance between the lower surface of the device and the upper surface of the cooling fin asse
> An array of 16 silicon chips arranged in 2 rows is insulated at the bottom and cooled by air flowing in forced convection over the top. The array is located either with its long side or its short side facing the cooling air. If each chip is 10 mm* 10 mm
> A thin, flat plate is placed in an atmospheric pressure air stream that flows parallel to it at a velocity of 5 m/s. The temperature at the surface of the plate is maintained uniformly at 200°C, and that of the main air stream is 30°C. Calculate the temp
> The integral method also can be applied to turbulent-flow conditions if experimental data for the wall shear stress are available. In one of the earliest attempts to analyze turbulent flow over a flat plate, Ludwig Prandtl proposed in 1921 the following
> A heat exchanger for heating liquid mercury is under development. The exchanger is visualized as a 15-cm-long and 0.3-m-wide flat plate. The plate is maintained at 70°C, and the mercury flows parallel to the short side at 15°C with
> For liquid metals with Prandtl numbers much less than unity, the hydrodynamic boundary layer is much thinner than the thermal boundary layer. As a result, one may assume that the velocity in the boundary layer is uniform / Starting with Eq. (5.7b)
> Air at 1000°C flows at an inlet velocity of 2 m/s between two parallel flat plates spaced 1 cm apart. Estimate the distance from the entrance to the point where the boundary layers meet.
> Evaluate the Nusselt number for flow over a sphere for the following conditions: / /
> Determine the rate of radiant heat emission in watts per square meter from a blackbody at (a) 150°C, (b) 600°C, and (c) 5700°C.
> Air at 20°C flows at 1 m/s between two parallel flat plates spaced 5 cm apart. Estimate the distance from the entrance to the point at which the hydrodynamic boundary layers meet.
> For flow over a slightly curved isothermal surface, the temperature distribution inside the boundary layer t can be approximated by the polynomial /where y is the distance normal to the surface. (a) By applying appropriate boun
> Experimental measurements of the temperature distribution during the flow of air at atmospheric pressure over the wing of an airplane indicate that the temperature distribution near the surface can be approximated by a linear equation: where a = a consta
> The average friction coefficient for flow over a 0.6-m long plate is 0.01. What is the value of the drag force in newtons per meter width of the plate for the following fluids: (a) air at 15°C, (b) steam at 100°C and atmospheric pressure, (c) water at
> Assuming a linear velocity distribution and a linear temperature distribution in the boundary layer over a flat plate, derive a relation between the thermal and hydrodynamic boundary layer thicknesses and the Prandtl number.
> Engine oil at 100°C flows over and parallel to a flat sur- face at a velocity of 3 m/s. Calculate the thickness of the hydrodynamic boundary layer at a distance 0.3 m from the leading edge of the surface.
> A journal bearing has a clearance of 0.5 mm. The journal has a diameter of 100 mm and rotates at 3600 rpm within the bearing. It is lubricated by an oil having a density of 800 kg/m3, a viscosity of 0.01 kg/m s, and a thermal conductivity of 0.14 W/m K.
> A journal bearing has a clearance of 0.5 mm. The journal has a diameter of 100 mm and rotates at 3600 rpm within the bearing. It is lubricated by an oil having a density of 800 kg/m3, a viscosity of 0.01 kg/m s, and a thermal conductivity of 0.14 W/m K.
> A journal bearing is idealized as a stationary flat plate and a moving flat plate that moves parallel to it. The space between the two plates is filled by an incompressible fluid. Consider such a bearing in which the stationary and moving plates are at 1
> When viscous dissipation is appreciable, conservation of energy [Eq. (5.6)] must take into account the rate at which mechanical energy is irreversibly converted to thermal energy due to viscous effects in the fluid. This gives rise to an additional term,
> Repeat Problem 1.25 but assume that the surface of the storage vessel has an absorbance (equal to the emittance) of 0.1. Then determine the rate of evaporation of the liquid oxygen in kilograms per second and pounds per hour, assuming that convection can
> Evaluate the Prandtl number from the following data: / kg/m s.
> Suppose that the graph below shows measured values of / for air in forced convection over a cylinder of diameter D, plotted on a logarithmic graph of / as a function of ReD Pr. Write an appropriate dimensionless correlation for the average Nusselt number
> The drag on an airplane wing in flight is known to be a function of the density of air () , the viscosity of air (() , the free-stream velocity (U() , a characteristic dimension of the wing (s), and the shear stress on the surface of th
> A turbine blade with a characteristic length of 1 m is cooled in an atmospheric pressure wind tunnel by air at 40°C with a velocity of 100 m/s. For a surface temperature of 500 K, the cooling rate is found to be 10,000 watts. Use these results
> The test data tabulated on the next page were reduced from measurements made to determine the heat trans- fer coefficient inside tubes at Reynolds numbers only slightly above transition and at relatively high Prandtl numbers (as associated with oils). Te
> The experimental data shown tabulated were obtained by passing n-butyl alcohol at a bulk temperature of 15°C over a heated flat plate 0.3-m long, 0.9-m wide, and with a surface temperature of 60°C. Correlate the experimental data us
> A series of tests in which water was heated while flowing through a 1-m-long electrically heated tube of 1.3-cm ID yielded the experimental pressure-drop data shown next. Isothermal pressure-drop data for the same tube are given below in terms of the dim
> The torque due to the frictional resistance of the oil film between a rotating shaft and its bearing is found to be dependent on the force F normal to the shaft, the speed of rotation N of the shaft, the dynamic viscosity m of the oil, and th
> Replot the data points of Fig. 5.9(b) on log-log paper and find an equation approximating the best correlation line. Compare your results with Fig. 5.10. Then suppose that steam at 1 atm and 100°C is flowing across a 5-cm-OD pipe at a velocity of 1 m/s.
> The convection equations relating the Nusselt, Reynolds, and Prandtl numbers can be rearranged to show that for gases the local heat transfer coefficient hc,x depends on the absolute temperature / This formulation is of the form / where n and C are
> A spherical vessel, 0.3 m in diameter, is located in a large room whose walls are at 27°C (see sketch). If the vessel is used to store liquid oxygen at 2183°C and both the surface of the storage vessel and the walls of the room are
> Experiments have been performed on the temperature distribution in a homogeneous long cylinder (0.1 m diameter, thermal conductivity of 0.2 W/m K) with uniform internal heat generation. By dimensional analysis, determine the relation between the steady-
> Evaluate the Reynolds number for flow over a tube from the following data: / /
> Develop the control volume difference equation for one-dimensional steady conduction in a fin with variable cross-sectional area A(x) and perimeter P(x). The heat transfer coefficient from the fin to ambient is a constant / and the fin tip is adiabatic.
> Solve the system of equations: by Jacobi and Gauss-Seidel iteration. Use as a convergence criterion Compare the rate of convergence for the two methods.
> Discuss the advantages and disadvantages of two methods for solving one-dimensional steady conduction problems.
> Determine the stability criterion for an explicit solution of three-dimensional transient conduction in a rectangular geometry.
> Derive the energy balance equation for a corner control volume in a three-dimensional steady conduction problem with heat generation in a rectangular coordinate system. Assume an adiabatic boundary condition and equal node spacing in all three dimensions
> Derive the control volume energy balance equation for three-dimensional transient conduction with heat generation in a rectangular coordinate system.
> Consider two-dimensional steady conduction near a curved boundary. Determine the difference equation for an appropriate control volume near the node (i, j). The boundary experiences convection heat transfer through a coefficient h to ambient temperature
> Two large parallel plates with surface conditions approximating those of a blackbody are maintained at 816°C and 260°C, respectively. Determine the rate of heat transfer by radiation between the plates in W/m2 and the radiative heat transfer coefficient
> A circumferential fin with a rectangular cross section is made of mild steel has an outer diameter of 3.7 cm OD, and a thickness of 0.3 cm. An array of these circular fins 5 are attached to a circular tube with an outer diameter of 2.5 cm as shown. Cooli
> Solve the set of difference equations derived in Problem 4.52, given the following values of the problem parameters: Determine the temperature distribution in the disk when the maximum temperature is 300°C.
> It has been proposed that a highly concentrating solar collector such as the one that follows can be used to process materials economically when it is desirable to heat the material surface rapidly without significantly heating the bulk. In one such proc
> Hot flue gases from a combustion furnace flow through a chimney, which is 7 m tall and has a hollow cylindrical cross section with inner diameter di = 30 cm and outer diameter do = 50 cm. The flue gases flow with an average temperature of Tg = 300°C and
> For the geometry shown in the sketch, determine the layout of nodes and control volumes. Provide a scale drawing showing the problem geometry overlaid with the nodes and control volumes. Explain how to derive the energy balance equation for all the bound
> For one-dimensional conduction, why are the boundary control volumes half the size of the interior control volumes?
> How would the results of Problem 4.47 be modified if the problem were not axisymmetric?
> Determine the appropriate difference equations for an axisymmetric, steady, spherical geometry with volumetric heat generation. Explain how to solve the equations.
> Determine the difference equations applicable at the centerline and at the surface of an axisymmetric cylindrical geometry with volumetric heat generation and convection boundary condition. Assume steady- state conditions.
> A steel billet is to be heat treated by immersion in a molten salt bath. The billet is 5 cm square and 1 m long. Prior to immersion in the bath, the billet is at a uniform temperature of 20°C, The bath is 600°C, and the heat transfer coefficient at the b
> Using the information in Problem 1.22, estimate the ambient air temperature that could cause frostbite on a calm day on the ski slopes.
> A long concrete beam is to undergo a thermal test to determine its loss of strength in the event of a building fire. The beam cross section is triangular as shown in the sketch. Initially, the beam is at a uniform temperature of 20°C. At the s
> Derive the stability criterion for an inside-corner boundary control volume for two-dimensional steady conduction when a convection boundary condition exists.
> Derive Eq. (4.28).
> Derive the stability criterion for the explicit solution of two-dimensional transient conduction.
> Show that in the limit as / and the difference equation, Eq. (4.23), is equivalent to the two-dimensional version of the differential equation, Eq. (2.6).
> Repeat Problem 4.39 by considering, instead of the trapezoidal microchannel, the two geometries shown in the following figure (a) rectangular channel of dimension a = b = 200 mm, d = 200 mm, and p = 150 mm and the coolant flow heat transfer coefficient
> Discuss the advantages and disadvantages of using a large control volume.
> A novel method to cool high-powered microelectronic chips and chip modules is to etch the liquid coolant flow channels in silicon (Si) substrate itself [9–12]. A typical such arrangement with channels of trapezoidal cross section is sho
> Consider a band saw blade that is to cut steel bar stock. The blade thickness is 2 mm, its height is 20 mm, and it has penetrated the steel workpiece to a depth of 5 mm (see the accompanying sketch). Exposed surfaces of the blade are cooled by an ambient
> A long, steel beam with a rectangular cross section of 40 cm * 60 cm is mounted on an insulating wall as shown in the following sketch. The rod is heated by radiant heaters that maintain the top and bottom surfaces at 300°C. A stream of air at
> In order to prevent frostbite to skiers on chair lifts, the weather report at most ski areas gives both an air temperature and the wind-chill temperature. The air temperature is measured with a thermometer that is not affected by the wind. However, the r
> Determine (a) the temperature at the 16 equally spaced points shown in the accompanying sketch to an accuracy of three significant figures and (b) the rate of heat flow per meter thickness. Assume two-dimensional heat flow and k = 1 W/m K.
> The plate in Problem 4.34 gradually oxidizes over time so that the surface emissivity increases to 0.5. Calculate the resulting temperature in the plate, including radiation heat transfer to the surroundings at the same temperature as the ambient t
> A 1-cm-thick, 1-m-square steel plate is exposed to sunlight and absorbs a solar flux of 800 W/m2. The bottom of the plate is insulated, the edges are maintained at 20°C by water-cooled clamps, and the exposed face is cooled by a convection coefficie
> Repeat Problem 4.32 if the temperature distribution on the top surface of the bar varies sinusoidally from 40°C at the left edge to a maximum of 250°C in the center and back to 40°C at the right edge.
> In the long, 30-cm-square bar shown in the accompanying sketch, the left face is maintained at 40°C and the top face is maintained at 250°C. The right face is in contact with a fluid at 40°C through a heat transfer coeffi
> The horizontal cross section of an industrial chimney is shown in the accompanying sketch. Flue gases maintain the interior surface of the chimney at 300°C, and the outside is exposed to an ambient temperature of 0°C through a heat
> Determine the temperature at the four nodes shown in the sketch. Assume steady conditions and two- dimensional heat conduction. The four faces of the square shape are at different temperatures as shown.
> Give an example of a practical problem in which the variation of thermal conductivity with temperature is significant and for which a numerical solution is therefore the only viable solution method.
> Develop a reasonable layout of nodes and control volumes for the geometry shown in the sketch. Provide a scale drawing showing the problem geometry overlaid with the nodes and control volumes. Identify each type of control volume used.
> Develop a reasonable layout of nodes and control volumes for the geometry shown in the sketch. Provide a scale drawing showing the problem geometry overlaid with the nodes and control volumes.
> In an experimental set up in a laboratory, a long cylinder with a 5-cm diameter, and an electrical resistance heater inside its entire length is cooled with water flowing crosswise over the cylinder at 25°C and a velocity of 0.8 m/s. For these flow condi
> A long cylindrical rod, 8 cm in diameter, is initially at a uniform temperature of 20°C. At time t = 0, the rod is exposed to an ambient temperature of 400°C through a heat transfer coefficient of 20 W/m2 K. The thermal conductivity of the rod is 0.8 W/m
> An interior wall of a cold furnace, initially at 0°C, is suddenly exposed to a radiant flux of 15 kW/m2 when the furnace is brought on line. The outer sur- face of the wall is exposed to ambient air at 20°C through a heat transfer c
> To more accurately model the energy input from the sun, suppose the absorbed flux in Problem 4.23 is given by where t is in hours and qabs is in W/m2. (This time variation of qabs gives the same total heat input to the wall as in Problem 4.23, i.e., 2000
> Repeat the numerical calculations of Problem 4.23 (Trombe wall) in order to obtain results to graph the temperature distribution in the Trombe wall after 2, 4, 6, and 8 hours of exposure.
> A Trombe wall is a masonry wall often used in passive solar homes to store solar energy. Suppose that such a wall, fabricated from 20-cm-thick solid concrete blocks / is initially at 15°C in equilibrium with the room in which it is located. I
> A 3-m-long steel rod / / is initially at 20°C and is insulated completely except for its end faces. One end is suddenly exposed to the flow of combustion gases at 1000°C through a heat transfer coefficient of 250 W/m2 K an
> Equation (4.16) is often called the fully-implicit form of the one-dimensional transient conduction difference equation because all quantities in the equation, except for the temperatures in the energy storage term, are evaluated at the new time step, m
> What are the advantages and disadvantages of using explicit and implicit difference equations?
> What is the physical significance of the statement that the temperature of each node is just the average of its neighbors if there is no heat generation [with reference to Eq. (4.3)]?
> Consider one-dimensional transient conduction with a convection boundary condition in which the ambient temperature near the surface is a function of time. Determine the energy balance equation for the boundary control volume. How would the solution meth

