Question: We arrived at Formula 6.3.2, /
We arrived at Formula 6.3.2, / by using cylindrical shells, but now we can use integration by parts to prove it using the slicing method of Section 6.2, at least
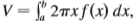
for the case where f is one to one and therefore has an inverse function t. Use the figure to show that
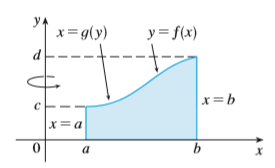
Make the substitution y = f(x) and then use integration by parts on the resulting integral to prove that
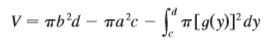
Transcribed Image Text:
V = 2xf(x) dx, yイ x= g(y) y= f(x) d x=b x= a a b V = mb’d – wa’c - [' "[g(y)l²dy
> Evaluate the integral. tan 2y dy
> Determine whether each integral is convergent or divergent. Evaluate those that are convergent. 1 3 — 4х 00
> (a) Show that the function defined by is not equal to its Maclaurin series. (b) Graph the function in part (a) and comment on its behavior near the origin. 1/22 if x + 0 f(x) = if x- 0
> The meaning of the decimal representation of a number 0.d1d2d3 ... (where the digit di is one of the numbers 0, 1, 2, . . . , 9) is that Show that this series always converges. di 0.d,d;dzd4 . .. 10 dz dz d4 102 103 104
> Use the sum of the first 10 terms to approximate the sum of the series. Estimate the error. 1 -1 3" + 4"
> Experiment with values of k between 3.4 and 3.5. What happens to the terms?
> Use the sum of the first 10 terms to approximate the sum of the series. Estimate the error. E 5 "cos’n cos'n 2.
> Use the sum of the first 10 terms to approximate the sum of the series. Estimate the error. 1/m nº
> Match the differential equation with its direction field (labeled I–IV). Give reasons for your answer. y' = x(2 – y) II yA --
> Determine whether the sequence converges or diverges. If it converges, find the limit. tan 'n an n
> Use the sum of the first 10 terms to approximate the sum of the series. Estimate the error. 1 5 + n*
> Determine whether the series converges or diverges. Σ 1+1/m
> Determine whether the series converges or diverges. Σ sin
> Determine whether the series converges or diverges. n! n"
> Determine whether the series converges or diverges. n! -1
> Explain why the Integral Test can’t be used to determine whether the series is convergent. cos'n Σ + n?
> Determine whether the series converges or diverges.
> Calculate terms of the sequence for a value of k between 3 and 3.4 and plot them. What do you notice about the behavior of the terms?
> Determine whether the series converges or diverges. 1 Σ -2 n Vn? – 1
> Evaluate the integral. (In x)² 2
> Determine whether the series converges or diverges. е" + 1 -1 пе" + 1
> Determine whether the series converges or diverges. n + 3" -i n + 2"
> Determine whether the series converges or diverges. 5 + 2n Σ (1 + n²)² 2)2
> Determine whether the series converges or diverges. n + 2 Σ (n + 1)3 A-3
> Determine whether the series converges or diverges. V1 + n Σ 2 + n n-1
> Determine whether the series converges or diverges. n2 + n + 1 n* + n? 2
> Determine whether the series converges or diverges. n п +1 -i n + n
> Determine whether the series converges or diverges. Σ Vn + 2
> If f(x) = (1 + x3)30, what is f(58)(0)?
> Use the Ratio Test to determine whether the series is convergent or divergent. (-2)" Σ n2 -1
> Evaluate the integral. x² + 2x) cos x dx
> Determine whether the series converges or diverges. 1 Σ Vn² + 1 n-1
> Determine whether the series converges or diverges. i n"
> Determine whether the series converges or diverges. 4"11 Σ 3" – 2
> Determine whether the series converges or diverges. Σ V3n4 + 1
> Determine whether the series converges or diverges. 1 + cos n e"
> Determine whether the series converges or diverges. (2k – 1)(k² – 1) Σ (k + 1)(k² + 4)²
> Determine whether the series converges or diverges. Σ k3 + 4k + 3 k-1
> Determine whether the series converges or diverges. k sin?k 1 1 + k³ WI
> Determine whether the series converges or diverges. In k k k-1
> Determine whether the series converges or diverges. 6" -i 5" - 1
> Use the Table of Integrals on Reference Pages 6–10 to evaluate the integral. et dx 4 - e2*
> Show that if p is an nth-degree polynomial, then p(x + 1) = ' Σ p®(x) i!
> Determine whether the series converges or diverges. 9" Σ 3 + 10"
> Determine whether the series converges or diverges. n - 1 AI n' + 1
> Determine whether the series converges or diverges. n + 1 n/n
> Determine whether the series converges or diverges. Σ In - 1 -2
> Determine whether the series converges or diverges. 1 Σ n' + 8 n-1
> Set up an integral that represents the length of the curve. Then use your calculator to find the length correct to four decimal places. x = y? – 2y, 0 < y< 2
> For what values of p is each series convergent? (In n)" 2 (-1)* 1 n-2
> Use any test to determine whether the series is absolutely convergent, conditionally convergent, or divergent. (-9)" Σ n10*+1 n-1
> Find the Taylor polynomial T3(x) for the function f centered at the number a. Graph f and T3 on the same screen. f(x) — х сos x,
> For what values of p is each series convergent? (-1)" -1 n + p
> For what values of p is each series convergent? (-1)* -1 Σ n" n-1
> Approximate the sum of the series correct to four decimal places. (-1)"-1 Σ n 4"
> Approximate the sum of the series correct to four decimal places. E (-1)"ne 2n
> Determine whether the differential equation is linear. — х y tan x
> Approximate the sum of the series correct to four decimal places. i(-1)** -1 ク
> Approximate the sum of the series correct to four decimal places. (-1)" Σ (2n)!
> (a) Use integration by parts to show that (b) If f and t are inverse functions and f9 is continuous, prove that (c) In the case where f and t are positive functions and b > a > 0, draw a diagram to give a geometric interpretation of part (b). (d) U
> Show that the series is convergent. How many terms of the series do we need to add in order to find the sum to the indicated accuracy? (lerror|< 0.00005) n-
> Show that the series is convergent. How many terms of the series do we need to add in order to find the sum to the indicated accuracy? (-1)* Σ n2 2" -' (lerror |< 0.0005) -1
> Show that the series is convergent. How many terms of the series do we need to add in order to find the sum to the indicated accuracy? Σ (-)" (|error|< 0.0005) -1 n
> Use any test to determine whether the series is absolutely convergent, conditionally convergent, or divergent. 2 + 3n
> Show that the series is convergent. How many terms of the series do we need to add in order to find the sum to the indicated accuracy? (-1)*+1 Σ n° (|error|< 0.00005) n-1
> Graph both the sequence of terms and the sequence of partial sums on the same screen. Use the graph to make a rough estimate of the sum of the series. Then use the Alternating Series Estimation Theorem to estimate the sum correct to four decimal places.
> Graph both the sequence of terms and the sequence of partial sums on the same screen. Use the graph to make a rough estimate of the sum of the series. Then use the Alternating Series Estimation Theorem to estimate the sum correct to four decimal places.
> Determine whether the differential equation is linear. y' + x/y = x?
> Test the series for convergence or divergence. E (-1)"(/n + 1 – Vñ)
> Test the series for convergence or divergence. E(-1)". E(-1)* "". n!
> Test the series for convergence or divergence. E (-1)" cos n-1
> Evaluate the indefinite integral. Illustrate, and check that your answer is reasonable, by graphing both the function and its antiderivative (take C − 0). |x² sin 2x dx
> Show that cosh x ≥1+ 1 2 x2 for all x.
> Evaluate the integral. w/4 (" tan'0 sec?0 do Jo
> Find the area enclosed by the curve in Exercise 29. Data from Exercise 29: At what points does the curve have vertical or horizontal tangents? Use this information to help sketch the curve. X= 2a cos t - a cos 21 y = 2a sin t – a sin 2t
> Find the sum of the series. E [tan '(n + 1) – tan 'n] -1
> Find the sum of the series. 1 Σ п(п + 3)
> Evaluate the indefinite integral. Illustrate, and check that your answer is reasonable, by graphing both the function and its antiderivative (take C = 0). x3/2 In x dx
> Find the sum of the series. (-3)' Σ "-1 3n n-1
> Determine whether the series is conditionally convergent, absolutely convergent, or divergent. (-1)"/n Σ In n n-2
> Determine whether the series is conditionally convergent, absolutely convergent, or divergent. (-1)"(n Σ 22a+1 (-17(л + 1)3" 2n1
> Evaluate the integral. *w/2 · dx m/2 1 + cos'x
> Find the slope of the tangent line to the given curve at the point corresponding to the specified value of the parameter. r3 3 + сos 30; ө — п/2
> Determine whether the series is conditionally convergent, absolutely convergent, or divergent. E (-1) 'n-1/3
> Determine whether the series is convergent or divergent. Vn + 1 – Vn – 1 п — R-1
> Determine whether the series is convergent or divergent. E (-1)"-1. n + 1 n-1
> Determine whether the series is convergent or divergent. (-5)2n Σ n²9"
> Determine whether the series is convergent or divergent. 1.3. 5. (2n – 1) R-1 5"n!
> Determine whether the series is absolutely convergent or conditionally convergent. (-1)" Σ n-1 n' + 1 3
> Determine whether the series is convergent or divergent. 2n n° Σ | (1 + 2n²)"
> Determine whether the series is convergent or divergent. cos 3n Σ 1 + (1.2)" n-
> Determine whether the series is convergent or divergent. n Σ E In Зп + 1 n-1
> Evaluate the integral. 1 + 12t dt 1+ 3t
> Determine whether the sequence converges or diverges. If it converges, find the limit. (-1)" - an 2n
> Determine whether the series is convergent or divergent. 1 Σ n=2 n/In n
> Determine whether the series is convergent or divergent. (-1)" Σ Vn + 1
> Determine whether the series is convergent or divergent. n? + 1 Σ n3 + 1
> Evaluate the indefinite integral. Illustrate, and check that your answer is reasonable, by graphing both the function and its antiderivative (take C − 0). 2% dx Sxe
> A sequence is defined recursively by the equations a1 = 1, an+1 = 1 3 (a n +4). Show that {an} is increasing and an < 2 for all n. Deduce that / is convergent and find its limit.
> Determine whether the sequence is convergent or divergent. If it is convergent, find its limit. {(-10)"/n!}

