Question: A rubber sheet in biaxial stress is
A rubber sheet in biaxial stress is subjected to tensile stresses σx = 270 Pa and σy = 144 Pa. The corresponding strains in the sheet are εx = 0.0002 and εy = 0.000015. Determine Poisson’s ratio and the modulus elasticity of the material.
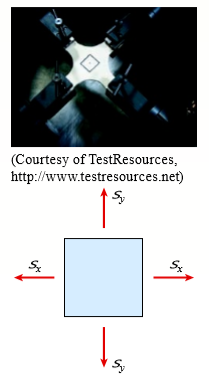
Transcribed Image Text:
(Courtesy of TestResources, http://www.testresources.net)
> At a point on the web of a girder on a gantry crane, the stresses acting on the x face of a stress element are σx = 6250 psi and τxy = 1425 psi (see figure). What is the allowable range of values for the stress σy if the
> σx = -108 MPa, σy = 58MPa, τxy = -58MPa Data for Problem 22: An element in plane stress (see figure) is subjected to stresses σx, σy, and τxy. (a) Determine the principal stresses and s
> σx = -3300 psi, σy = -11,000psi, τxy = 4500 psi. Data for Problem 21: An element in plane stress (see figure) is subjected to stresses σx, σy, and τxy. (a) Determine the principal stres
> σx = 16.5 MPa, σy = -91 MPa, τxy = -39 MPa. Data for Problem 20: An element in plane stress (see figure) is subjected to stresses σx, σy, and τxy. (a) Determine the principal stresses a
> σx = 14,500 psi, σy = 1070 psi, τxy = 1900 psi. Data for Problem 19: An element in plane stress (see figure) is subjected to stresses σx, σy, and τxy. (a) Determine the principal stress
> σx = 2150 kPa, σy = 375 kPa, τxy = -460 kPa. Data for Problem 18: An element in plane stress (see figure) is subjected to stresses σx, σy, and τxy. (a) Determine the principal stresses
> The stresses at a point along a beam supporting a sign (see figure) are σx = 2250 psi, σy = 1175 psi, and τxy = -820 psi. (a) Find the principal stresses. Show them on a sketch of a properly oriented element. (b) Find the
> A propeller shaft subjected to combined torsion and axial thrust is designed to resist a shear stress of -57 MPa and a compressive stress of -105 MPa (see figure). (a) Determine the principal stresses and show them on a sketch of a properly oriented elem
> Repeat the preceding problem using σy = -750 psi. Data from Problem 14: The state of stress on an element along the hydraulic lift cylinder on a truck is σy = -25 MPa. Find the maximum shear stress on the element and show the stat
> A steel pipe is subjected to a quadratic distributed load over its height with the peak intensity qo at the base (see figure). Assume the following pipe properties and dimensions: height L, outside diameter d = 200 mm, and wall thickness t = 10 mm. Allow
> The state of stress on an element along the hydraulic lift cylinder on a truck is σy = -5 MPa. Find the maximum shear stress on the element and show the state of stress on a sketch of a properly oriented element. Andrey N Bannov/Shutterst
> A shear wall in a reinforced concrete building is subjected to a vertical uniform load of intensity q and a horizontal force H, as shown in the first part of the figure. (The force H represents the effects of wind and earthquake loads.) As a consequence
> A simply supported beam is subjected to two point loads as shown in the figure. The stresses on element A are τxy = –20 kPa. Find the principal stresses on element A and show them on a sketch of a properly oriented element.
> The stresses on an element are σx = -300 psi and σy = -600 psi. Find the maximum shear stresses on the element and show them on a sketch of a properly oriented element.
> The normal and shear stresses acting on element B are σx = -46 MPa, σy = -13 MPa, and τxy = 21 MPa (see figure for Problem 10). Determine the maximum shear stresses and associated normal stresses and show them on a sketch
> The stresses acting on element B in the web of a wide-flange beam are found to be -14,000 psi compression in the horizontal direction and 2600 psi compression in the vertical direction. Also, shear stresses of magnitude -3800 psi act in the directions sh
> An element in plane stress from the fuselage of an airplane is subjected to compressive stresses of magnitude -35 MPa in the horizontal direction and tensile stresses of magnitude 6.5 MPa in the vertical direction. Also, shear stresses of magnitude -12.5
> The normal and shear stresses acting on element A are 6500 psi, 17,300 psi, and 2900 psi (see the figure b for Problem 5). Determine the maximum shear stresses and associated normal stresses and show them on a sketch of a properly oriented element. Data
> The stresses acting on element A in the web of a train rail are found to be 40 MPa tension in the horizontal direction and -160 MPa compression in the vertical direction. Also, shear stresses of magnitude -54 MPa act in the directions shown (see the figu
> An element in plane stress is subjected to stresses σx = 25500 psi, σy = 22000 psi, and τxy = 1900 psi (see the figure for Problem 1). Determine the principal stresses and show them on a sketch of a properly oriented elem
> A sign for an automobile service station is supported by two aluminum poles of hollow circular cross section, as shown in the figure. The poles are being designed to resist a wind pressure of 75 lb/ft2 against the full area of the sign. The dimensions of
> An element in plane stress is subjected to stresses σx = 105 MPa, σy = 75 MPa, and τxy = 25 MPa (see the figure for Problem 1). Determine the principal stresses and show them on a sketch of a properly oriented element. D
> An element in plane stress is subjected to stresses σx = 5750 psi, σy = 1100 psi, and τxy = 750 psi (see the figure for Problem 1). Determine the principal stresses and show them on a sketch of a properly oriented element
> Repeat the preceding problem using σx = 5.5 MPa, σy = 4MPa, and τxy = 3.2 MPa. Data from Problem 1: The stresses acting on an element are σx = 750 psi, σy = 600 psi, and τxy = 400 psi.
> σx = 7300 psi, σy = 0 psi, τxy = 1300psi Data for Problem 25: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle, det
> σx = 50MPa, σy = -23.4 MPa, τxy = -9.6 MPa Data for Problem 24: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle, d
> σx = 2050 psi, σy = 6100 psi, τxy = 2750 psi Data for Problem 23: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle,
> σx =-29.5 MPa, σy = 29.5 MPa, τxy = 27 MPa Data for Problem 22: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle, d
> σx = -11,500psi, σy = -18,250 psi, τxy = -7200 psi Data for Problem 21: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s c
> σx = -3.3MPa, σy = 8.9MPa, τxy = -14.1 MPa Data for Problem 20: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle, d
> σx = 800psi, σy = -2200 psi, τxy = 2900 psi Data for Problem 19: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle,
> A circular pole is subjected to linearly varying distributed force with maximum intensity qo. Calculate the diameter do of the pole if the maximum allowable shear stress for the pole is 75 MPa. do 2 m 90 = 100 kN/m
> σx = 2900 kPa, σy = 9100 kPa, τxy = -3750 kPa Data for Problem 18: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr’s circle
> σx = -5700 psi, σy = 950 psi, τxy = -2100 psi, θ = 65° Data for Problem 17: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Usin
> σx = 33 MPa, σy = -9 MPa, τxy = -9 MPa, θ = 35° Data for Problem 16: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using Mohr&
> σx = -1720 psi, σy = -680psi, τxy = 320 psi, θ = 14 ° Data for Problem 15: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using
> σx = -47 MPa, σy = -186 MPa, τxy = -29 MPa, θ = -33° Data for Problem 14: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). Using
> σx = 3500 psi, σy = 12,200 psi, τxy = -3300 psi, θ = -51° Data for Problem 13: An element in plane stress is subjected to stresses σx, σy, and τxy (see figure). U
> An element in pure shear is subjected to stresses τxy = 3750 psi, as shown in the figure. Using Mohr’s circle, determine the following: (a) The stresses acting on an element oriented at a slope of 3 on 4 (see figure). (b) The
> The rotor shaft of a helicopter (see figure part a) drives the rotor blades that provide the lifting force and is subjected to a combination of torsion and axial loading (see figure part b). It is known that normal stress σy = 68 MPa and shear
> A specimen used in a coupon test is shown in the figure. The stresses on element A are known to be σy = -1500 psi. Use Mohr’s circle to: (a) Find the stresses acting on the element oriented at an angle θ = -35&Ac
> A vertical pole consisting of a circular tube of outer diameter 5 in. and inner diameter 4.5 in. is loaded by a linearly varying distributed force with maximum intensity of qo. Find the maximum shear stress in the pole. 10 ft 90 = 400 lb/ft
> A specimen used in a coupon test has normal stress σy = 15 MPa (see figure). Using Mohr’s circle, find the state of stress on the element oriented at angle θ = 20° and show the full stress state on a s
> An element on the surface of a drive shaft is in pure shear and is subjected to stresses τxy = 2700 psi, as shown in the figure. Using Mohr’s circle, determine the following. (a) The stresses acting on an element oriented at
> An element in biaxial stress is subjected to stresses σx = -29 MPa and σy = 57 MPa, as shown in the figure. Using Mohr’s circle, determine the following. (a) The stresses acting on an element oriented at a slope of
> An element on the top surface of the fuel tanker in Problem 1 is in biaxial stress and is subjected to stresses σx = 6250 psi and σy = -1750 psi, as shown in the figure. Using Mohr’s circle, determine the following
> An element on the top surface of the fuel tanker in Problem 1 is in biaxial stress and is subjected to stresses σx = -48 MPa and σy = 19 MPa, as shown in the figure. Using Mohr’s circle, determine the following. (a
> An element on the gusset plate in Problem 23 in uniaxial stress is subjected to compressive stresses of magnitude -6750 psi, as shown in the figure. Using Mohr’s circle, determine the following. (a) The stresses acting on an element ori
> An element in uniaxial stress is subjected to tensile stresses σx = 57 MPa, as shown in the figure. Using Mohr’s circle, determine the following. (a) The stresses acting on an element oriented at an angle θ = -33
> The normal stress on an elastomeric rubber pad in a test machine is σy = -100 psi (see figure). Assume E = 312 psi and shear modulus G = 105 psi. (a) Calculate the strains in the pad in the x, y, and z directions. (b) Calculate the unit volume
> A circle of a diameter d = 200 mm is etched on a brass plate (see figure). The plate has dimensions of 400 × 400 × 20 mm. Forces are applied to the plate, producing uniformly distributed normal stresses σx = 59 MPa an
> A simple log bridge in a remote area consists of two parallel logs with planks across them (see figure). The logs are Douglas fir with an average diameter 300Â mm. A truck moves slowly across the bridge, which spans 2.5 m. Assume that the weig
> A rectangular beam with semicircular notches, as shown in part b of the figure, has dimensions h = 0.88 in. and h1 = 0.80 in. The maximum allowable bending stress in the metal beam is σmax = 60 ksi, and the bending moment is M = 600 lb-in. Det
> Solve the preceding problem for an aluminum plate with b = 10 in, t = 0.75 in., E = 10,600 ksi, v = 0.33, Px = 96 kips, Py = 24 kips, and V = 18 kips. For part (b) of Problem 12, assume that the required strain energy stored is 640 in.-lb. In part (c), t
> A square plate of a width b and thickness t is loaded by normal forces Px and Py and by shear forces V, as shown in the figure. These forces produce uniformly distributed stresses acting on the side faces of the plate. (a) Calculate the change â
> A 4.0 in. cube of concrete (E = 4.5 × 106 psi, v = 0.2) is compressed in biaxial stress by means of a framework that is loaded as shown in the figure. Assuming that each load F equals 25 kips, determine the change âˆ&#
> A brass cube of 48 mm on each edge is compressed in two perpendicular directions by forces P = 160 kN (see figure). (a) Calculate the change ∆V in the volume of the cube and the strain energy U stored in the cube, assuming E = 100 GPa a
> Solve the preceding problem for an aluminum plate with σx = 12,000 psi (tension), σy = 23000 psi (compression), dimensions 20 × 30 × 0.5 in, E = 10.5 × 106 psi, and v = 0.33. Data from Proble
> A rectangular plate in biaxial stress (see figure) is subjected to normal stresses σx = 67 MPa (tension) and σy = -23 MPa (compression). The plate has dimensions 400 × 550 × 20 mm and is made of steel with
> Solve the preceding problem for a steel plate with σx = 11,600 psi (tension), σy = -5700 psi (compression), εx = 450 × 1026 (elongation), and εy = -310 × 1026 (shortening). Data
> A cast-iron plate in biaxial stress is subjected to tensile stresses σx = 31 MPa and σy = 17 MPa (see figure). The corresponding strains in the plate are εx = 240 × 1026 and εy = 85 Ã
> Assume that the normal strains εx and εy for an element in plane stress (see figure) are measured with strain gages. (a) Obtain a formula for the normal strain εz in the z direction in terms of εx, &Ici
> An element of a material is subjected to plane stresses as shown in the figure. The stresses σx, σy, and τxy are 10 MPa, –15 MPa, and 5 MPa, respectively. Assume E = 200 GPa and v = 0.3. (a) Calculate th
> Calculate the maximum shear stress Ï„max in the web of the T-beam shown in the figure if b = 10 in, t = 0.5 in, h = 7 in., h1 = 6.2 in., and the shear force V = 5300 lb. hi b-
> The state of stress on an element of material is shown in the figure. Calculate the unit volume change of the element if the stresses σx and σy are –20 ksi and 10 ksi, respectively. Assume E = 10,600 ks
> Solve the preceding problem if the thickness of the steel plate is t = 12 mm , the gage readings are εx = 530 × 10-6− (elongation) and εy – 2210 × 10-6 (shortening),
> A solid bronze sphere (volume modulus of elasticity K = 14.5 × 106 psi) is suddenly heated around its outer surface. The tendency of the heated part of the sphere to expand produces uniform tension in all directions at the center of the sphere. If the st
> A solid steel sphere (E = 210 GPa, v = 0.3) is subjected to hydrostatic pressure p such that its volume is reduced by 0.4%. (a) Calculate the pressure p. (b) Calculate the volume modulus of elasticity K for the steel. (c) Calculate the strain energy U st
> A solid spherical ball of magnesium alloy (E = 6.5 × 1026 psi, v = 0.35) is lowered into the ocean to a depth of 8000 ft. The diameter of the ball is 9.0 in. (a) Determine the decrease ∆d in diameter, the decrease ∆V in volume, and the strain energy U of
> A copper bar with a square cross section is inserted into a square rigid tube as shown in the figure. The length of the copper bar is 1.2 m and the area of the cross section is 300 mm2. The bar is subjected to a force P that applies a uniformly distribut
> A rubber cube R of a side L = 3 in and cross- sectional area A = 9 in2 is compressed inside a steel cube S by a force F = 5 lb that applies uniformly distributed pressure to the rubber. Assume E = 0.3 ksi and n=v = 0.45. (a) Calculate the lateral pressur
> A block R of rubber is confined between plane parallel walls of a steel block S (see figure). A uniformly distributed pressure p0 is applied to the top of the rubber block by a force F. (a) Derive a formula for the lateral pressure p between the rubber a
> A rubber cylinder R of length L and cross- sectional area A is compressed inside a steel cylinder S by a force F that applies a uniformly distributed pressure to the rubber (see figure). (a) Derive a formula for the lateral pressure p between the rubber
> Solve the preceding problem if the material is nylon. (a) Find the bulk modulus K for the nylon if the following stress and strain data is known: normal stresses are σx = 3.9 MPa, σy = 3.2MPa, and σz = 1.8 MPa ; and norma
> The T-beam shown in the figure has cross-sectional dimensions: b = 210 mm, t = 16 mm, h = 300 mm, and h1 = 280 mm. The beam is subjected to a shear force V = 68 kN. Determine the maximum shear stress Ï„max in the web of the beam. hi b-
> An element of aluminum is subjected to triaxial stress (see figure). (a) Find the bulk modulus K for the aluminum if the following stress and strain data are known: normal stresses are σx = 5200 psi (tension), σy = 4750 psi (compres
> Solve the preceding problem if the cube is granite (E = 80 GPa, v = 0.25) with dimensions a = 89 mm and compressive strains εx = 690 × 1026 and εy = εz = 255 × 1026. For part (e) of Problem
> A cube of cast iron with sides of length a = 4.0 in. (see figure) is tested in a laboratory under triaxial stress. Gages mounted on the testing machine show that the compressive strains in the material are εx = -225 × 1026 and &
> An element of aluminum in the form of a rectangular parallelepiped (see figure) of dimensions a = 5.5 in, b = 4.5 in, and c = 3.5 in is subjected to triaxial stresses σx = 12,500 psi, σy = 25000 psi, and sz = 21400 psi acting on the
> An element of aluminum is subjected to triaxial stresses. Calculate the strains in the element in x, y, and z directions if the stresses σx, σy, and σz are –20 MPa, 28 MPa, and –18 MPa,
> Solve Problem 14 by using Mohr’s circle for plane strain. Data from Problem 14: Solve the preceding problem for the following data: εx = 21120 × 1026, εy = 2430 × 1026 , γ
> Solve Problem 13 by using Mohr’s circle for plane strain. Data from Problem 13: An element of material in plane strain (see figure) is subjected to strains εx = 480 × 1026, εy = 70 ×
> Solve Problem 12 by using Mohr’s circle for plane strain. Data from Problem 12: Solve the preceding problem for the following strains: εx = 120 × 1026, εy = -450 × 1026, and Î&
> Solve Problem 11 by using Mohr’s circle for plane strain. Data from Problem 11: The strains for an element of material in plane strain (see figure) are as follows: εx = 480 × 1026, εy = 140 &Atil
> A hollow aluminum box beam has the square cross section shown in the figure. Calculate the maximum and minimum shear stresses Ï„max and Ï„min in the webs of the beam due to a shear force V = 28 k. 1.0 in. 1.0 in. 12 in.
> Solve Problem 10 by using Mohr’s circle for plane strain. Data from Problem 10: Solve the preceding problem for the following data: εx = 190 × 1026, εy = -230 × 1026, γxy
> Solve Problem 9 by using Mohr’s circle for plane strain. Data from Problem 9: An element of material subjected to plane strain (see figure) has strains of εx = 280 × 1026, εy = 420 ×
> The strains on the surface of an experimental device made of pure aluminum (E = 70 GPa, v = 0.33) and tested in a space shuttle were measured by means of strain gages. The gages were oriented as shown in the figure, and the measured strains were Î&
> On the surface of a structural component in a space vehicle, the strains are monitored by means of three strain gages arranged as shown in the figure. During a certain maneuver, the following strains were recorded: εa = 1100 × 1
> A 60° strain rosette, or delta rosette, consists of three electrical-resistance strain gages arranged as shown in the figure. Gage A measures the normal strain εa in the direction of the x axis. Gages B and C measure the strains &
> A solid circular bar with a diameter of d = 1.25 in is subjected to an axial force P and a torque T (see figure). Strain gages A and B mounted on the surface of the bar give readings εA = 140 × 1026 and εB = 260 &At
> A 45° strain rosette (see figure) mounted on the surface of an automobile frame gives the following readings: gage A = 310 × 1026; gage B = 180 × 1026; and gage C = -160 × 1026. Determine the principal st
> During a test of an airplane wing, the strain gage readings from a 45° rosette (see figure) are as follows: gage A = 520 × 1026 ; gage B = 360 × 1026; and gage C = -80 × 1026. Determine the principal stra
> A hollow steel box beam has the rectangular cross section shown in the figure. Determine the maximum allowable shear force V that may act on the beam if the allowable shear stress is 36 MPa. 20 mm 10 mm 10 mm 450 20 Įmm 200 mm
> Solve the preceding problem for the following data: σx = -150 MPa, σy = -210 MPa, τxy = -16 MPa, and θ = 50°. The material is brass with E = 100 GPa and v = 0.34. Data from Problem 17: An elemen
> An element in plane stress is subjected to stresses σx = -8400 psi, σy = 1100 psi, and τxy = -1700 psi (see figure). The material is aluminum with modulus of elasticity E = 10,000 ksi and Poisson’s ratio
> Solve the preceding problem if the plate is made of aluminum with E = 72 GPa and Poisson’s ratio v = 0.33. The plate is loaded in biaxial stress with normal stress σx = 79 MPa, angle ∅ = 18°, and
> A brass plate with a modulus of elasticity E = 16 × 106 psi and Poisson’s ratio v = 0.34 is loaded in biaxial stress by normal stresses σx and σy (see figure). A strain gage is bonded to the plate at a
> Solve the preceding problem for the following data: εx = 21120 × 1026, εy = 2430 × 1026 , γxy = 780 × 1026, and θ = 45°. Data from Problem 13: An elem
> An element of material in plane strain (see figure) is subjected to strains εx = 480 × 1026, εy = 70 × 1026, and γxy = 420 × 1026. Determine the following quantities: (a) the st

