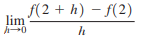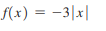Question: Compute the following limit for each function
> Theorem 3 also states that. What conditions must n and an satisfy for the limit to be ∞? For the limit to be - ∞?
> In a lottery game, a single ball is drawn at random from a container that contains 25 identical balls numbered from 1 through 25. Compute the probability that the number drawn is: Less than 10 or greater than 10.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. The graph of a rational function cannot cross a horizontal asymptote.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. A rational function has at most one horizontal asymptote.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. A rational function has at most one vertical asymptote.
> Give a limit expression that describes the left end behavior of the function.
> Give a limit expression that describes the left end behavior of the function.
> Give a limit expression that describes the right end behavior of the function.
> Give a limit expression that describes the right end behavior of the function.
> Find all horizontal and vertical asymptotes.
> Find all horizontal and vertical asymptotes.
> Find all horizontal and vertical asymptotes.
> Using the probability assignments in Problem 27C, what is the probability that a random customer will not choose brand S? Data from problem 27C,:
> Find all horizontal and vertical asymptotes.
> Find all horizontal and vertical asymptotes.
> Find all horizontal and vertical asymptotes.
> Find all horizontal and vertical asymptotes.
> Use - ∞ or ∞ where appropriate to describe the behavior at each zero of the denominator and identify all vertical asymptotes.
> Use - ∞ or ∞ where appropriate to describe the behavior at each zero of the denominator and identify all vertical asymptotes.
> Use - ∞ or ∞ where appropriate to describe the behavior at each zero of the denominator and identify all vertical asymptotes.
> Use - ∞ or ∞ where appropriate to describe the behavior at each zero of the denominator and identify all vertical asymptotes.
> Use - ∞ or ∞ where appropriate to describe the behavior at each zero of the denominator and identify all vertical asymptotes.
> Find each function value and limit. Use - ∞ or ∞ where appropriate.
> In a lottery game, a single ball is drawn at random from a container that contains 25 identical balls numbered from 1 through 25. Compute the probability that the number drawn is: Even or a multiple of 7.
> Find each function value and limit. Use - ∞ or ∞ where appropriate.
> Find each function value and limit. Use - ∞ or ∞ where appropriate.
> Find each function value and limit. Use - ∞ or ∞ where appropriate.
> Find (A) the leading term of the polynomial, (B) the limit as x approaches ∞, and (C) the limit as x approaches - ∞.
> Find (A) the leading term of the polynomial, (B) the limit as x approaches ∞, and (C) the limit as x approaches - ∞.
> Find (A) the leading term of the polynomial, (B) the limit as x approaches ∞, and (C) the limit as x approaches - ∞.
> Find (A) the leading term of the polynomial, (B) the limit as x approaches ∞, and (C) the limit as x approaches - ∞.
> Find each limit. Use - ∞ and ∞ when appropriate.
> Find each limit. Use - ∞ and ∞ when appropriate.
> Find each limit. Use - ∞ and ∞ when appropriate.
> In a family with 2 children, excluding multiple births, what is the probability of having 2 girls? Assume that a girl is as likely as a boy at each birth.
> Find each limit. Use - ∞ and ∞ when appropriate.
> Refer to the following graph of y = f (x).
> Refer to the following graph of y = f (x).
> Refer to the following graph of y = f (x).
> Factor each polynomial into the product of first-degree factors with integer coefficients.
> Factor each polynomial into the product of first-degree factors with integer coefficients.
> Factor each polynomial into the product of first-degree factors with integer coefficients.
> Factor each polynomial into the product of first-degree factors with integer coefficients.
> Refer to Problem 97. The average fee per ton of pollution is given by A(x) = F(x) >x. Write a piecewise definition of A(x). What is the limit of A(x) as x approaches 4,000 tons? As x approaches 8,000 tons? Data from Problem 97: A state charges polluters
> Assume that the volume discounts in Table 1 apply only to that portion of the volume in each interval. That is, the discounted price for a $4,000 purchase would be computed as follows: 300 + 0.9717002 + 0.9512,0002 + 0.9311,0002 = 3,809 (A) If x is the
> A single card is drawn from a standard 52-card deck. Let D be the event that the card drawn is a diamond, and let F be the event that the card drawn is a face card. find the indicated probabilities. Answer:
> Refer to Problems 91 and 92. Write a brief verbal comparison of the two services described for customers who use a car for more than 10 hours in a month. A company sells custom embroidered apparel and promotional products. Table 1 shows the volume disco
> A car sharing service offers a membership plan with no monthly fee. Members who use a car for at most 10 hours are charged $15 per hour. Members who use a car for more than 10 hours are charged $10 per hour.
> Let Æ’ be defined by.
> Compute the following limit for each function.
> Compute the following limit for each function.
> Compute the following limit for each function.
> Is the limit expression a 0>0 indeterminate form? Find the limit or explain why the limit does not exist.
> Is the limit expression a 0>0 indeterminate form? Find the limit or explain why the limit does not exist.
> Is the limit expression a 0>0 indeterminate form? Find the limit or explain why the limit does not exist.
> Refer to the description of a standard deck of 52 cards and Figure 4 on page 384. An experiment consists of drawing 1 card from a standard 52-card deck , what is the probability of drawing. Figure 4: A six or club.
> Is the limit expression a 0>0 indeterminate form? Find the limit or explain why the limit does not exist.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample.
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> A single card is drawn from a standard 52-card deck. Let D be the event that the card drawn is a diamond, and let F be the event that the card drawn is a face card. find the indicated probabilities
> Find each indicated quantity if it exists.
> Find each indicated quantity if it exists.
> Sketch a possible graph of a function that satisfies the given conditions.
> Sketch a possible graph of a function that satisfies the given conditions.
> Find the indicated limits.
> Find the indicated limits.
> Find the indicated limits.
> Find the indicated limits. Answer:
> Find each limit if it exists.
> Find each limit if it exists.
> Refer to the description of a standard deck of 52 cards and Figure 4 on page 384. An experiment consists of drawing 1 card from a standard 52-card deck , what is the probability of drawing. Figure 4: A red queen.
> Find each limit if it exists.
> Find each limit if it exists.
> Find each limit if it exists.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> Use the graph of the function g shown to estimate the indicated limits and function values.
> Use the graph of the function g shown to estimate the indicated limits and function values.
> Use the graph of the function g shown to estimate the indicated limits and function values.
> Use the graph of the function g shown to estimate the indicated limits and function values.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> A single card is drawn from a standard 52-card deck. Let D be the event that the card drawn is a diamond, and let F be the event that the card drawn is a face card. find the indicated probabilities.
> Write the expression as a quotient of integers, reduced to lowest terms.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> Use the graph of the function f shown to estimate the indicated limits and function values.
> You draw and keep a single bill from a hat that contains a $1, $10, $20, $50, and $100 bill. What is the expected value of the game to you?
> If the probability distribution for the random variable X is given in the table, what is the expected value of X?
> Find the average (mean) of the exam scores in Problem 2, if each score is divided by 2.
> Find the average (mean) of the exam scores in Problem 2, if 3 points are subtracted from each score.
> Find the average (mean) of the exam scores 78, 64, 97, 60, 86, and 83.
> Repeat Problem 55, assuming that the Grand Prize is currently $400,000,000. Data from Problem 55: A $2 Powerball lottery ticket has a 1/27.05 prob- 2. (A) ability of winning $4, a 1/317.39 probability of winning $7, a 1/10,376.47 probability of winning
> A pink-flowering plant is of genotype RW. If two such plants are crossed, we obtain a red plant (RR) with probability .25, a pink plant (RW or WR) with probability .50, and a white plant (WW) with probability .25, as shown in the table. What is the expec