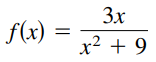Question: Find the vertical asymptotes (if any) of
> Determine whether the statement is true or false. If it is false, explain why or give an example that shows it is false. The graph of a function cannot cross a vertical asymptote.
> A crossed belt connects a 20-centimeter pulley (10-cm radius) on an electric motor with a 40-centimeter pulley (20-cm radius) on a saw arbor (see figure). The electric motor runs at 1700 revolutions per minute. a. Determine the number of revolutions pe
> Consider the shaded region outside the sector of a circle of radius 10 meters and inside a right triangle (see figure). a. Write the area A = f(θ) of the region as a function of θ. Determine the domain of the function. b. Use
> On a trip of d miles to another city, a truck driver’s average speed was x miles per hour. On the return trip, the average speed was y miles per hour. The average speed for the round trip was 50 miles per hour. a. Verify that What is th
> A 25-foot ladder is leaning against a house (see figure). If the base of the ladder is pulled away from the house at a rate of 2 feet per second, then the top will move down the wall at a rate of where x is the distance between the base of the ladder and
> For a quantity of gas at a constant temperature, the pressure P is inversely proportional to the volume V. What is the limit of P as V approaches 0 from the right? Explain what this means in the context of the problem.
> Determine whether f(x) approaches ∞ or −∞ as x approaches −2 from the left and from the right. f(x) = sec (πx / 4)
> Use a graphing utility to complete the table for each function and graph each function to estimate the limit. What is the value of the limit when the power of x in the denominator is greater than 3?
> According to the theory of relativity, the mass m of a particle depends on its velocity v. That is, / where m0 is the mass when the particle is at rest and c is the speed of light. Find the limit of the mass as v approaches c from the left.
> Use the graph of the function f (see figure) to sketch the graph of g(x) = 1f(x) on the interval [−2, 3]. To print an enlarged copy of the graph, go to MathGraphs.com.
> Does the graph of every rational function have a vertical asymptote? Explain.
> Write a rational function with vertical asymptotes at x = 6 and x = −2, and with a zero at x = 3. (a) / (b) / (c) /
> Use the information to determine the limits. (a) / (b) / (c) /
> Use the information to determine the limits. (a) / (b) / (c) /
> Use a graphing utility to graph the function and determine the one-sided limit.
> Use a graphing utility to graph the function and determine the one-sided limit.
> Find the one-sided limit (if it exists).
> Determine whether f(x) approaches ∞ or −∞ as x approaches −2 from the left and from the right. f(x) = tan (πx / 4)
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Determine whether f(x) approaches ∞ or −∞ as x approaches −2 from the left and from the right. f(x) = 1 / x + 2
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Determine whether the graph of the function has a vertical asymptote or a removable discontinuity at x = −1. Graph the function using a graphing utility to confirm your answer. f(x) = sin (x + 1) / x + 1
> Determine whether the graph of the function has a vertical asymptote or a removable discontinuity at x = −1. Graph the function using a graphing utility to confirm your answer. f(x) = cos (x2 – 1) / x + 1
> Determine whether the graph of the function has a vertical asymptote or a removable discontinuity at x = −1. Graph the function using a graphing utility to confirm your answer. f(x) = x2 – 2x - 8 / x + 1
> Determine whether the graph of the function has a vertical asymptote or a removable discontinuity at x = −1. Graph the function using a graphing utility to confirm your answer. f(x) = x2 - 1 / x + 1
> Find the vertical asymptotes (if any) of the graph of the function. g(θ) = tan θ / θ
> Find the vertical asymptotes (if any) of the graph of the function. s(t) = t / sin t
> Find the vertical asymptotes (if any) of the graph of the function. f(x) = tan πx
> Determine whether f(x) approaches ∞ or −∞ as x approaches −2 from the left and from the right. f(x) = 2 │x│ / x2 – 4
> Find the vertical asymptotes (if any) of the graph of the function. f(x) = csc πx
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> In your own words, describe what is meant by a vertical asymptote of a graph.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> A utility company burns coal to generate electricity. The cost C in dollars of removing p% of the air pollutants in the stack emissions is Find the cost of removing 50% of the pollutants. Find the cost of removing 90% of the pollutants. Find the limit
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the one-sided limit (if it exists).
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the one-sided limit (if it exists).
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Find the vertical asymptotes (if any) of the graph of the function.
> Determine whether f (x) approaches ∞ or −∞ as x approaches 6 from the left and from the right.
> Determine whether f (x) approaches ∞ or −∞ as x approaches 6 from the left and from the right.
> Verify that the Intermediate Value Theorem applies to the indicated interval and find the value of c guaranteed by the theorem. has at least two zeros in the interval [−3, 3].
> Create a table of values for the function and use the result to determine whether f (x) approaches ∞ or −∞ as x approaches −3 from the left and from the right. Use a graphing utility to graph the function to confirm your answer. f(x) = tan (πx / 6)
> Verify that the Intermediate Value Theorem applies to the indicated interval and find the value of c guaranteed by the theorem. has at least two zeros in the interval [−3, 3].
> Use the Intermediate Value Theorem to show that has at least two zeros in the interval [−3, 3].
> Use the Intermediate Value Theorem to show that has a zero in the interval [1, 2].
> Describe the intervals on which the function is continuous.
> Describe the intervals on which the function is continuous.
> Describe the intervals on which the function is continuous.
> Describe the intervals on which the function is continuous.
> Describe the intervals on which the function is continuous.
> Describe the intervals on which the function is continuous.
> Find the values of b and c such that the function is continuous on the entire real number line.
> Create a table of values for the function and use the result to determine whether f (x) approaches ∞ or −∞ as x approaches −3 from the left and from the right. Use a graphing utility to graph the function to confirm your answer. f(x) = cot (πx / 3)
> Find the value of c such that the function is continuous on the entire real number line.
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Find the x-values (if any) at which f is not continuous. Which of the discontinuities are removable?
> Discuss the continuity of the function on the closed interval.
> Discuss the continuity of the function on the closed interval.
> Find the limit (if it exists). If it does not exist, explain why.
> Create a table of values for the function and use the result to determine whether f (x) approaches ∞ or −∞ as x approaches −3 from the left and from the right. Use a graphing utility to graph the function to confirm your answer. f(x) = -1 / 3 + x
> Find the limit (if it exists). If it does not exist, explain why.
> Find the limit (if it exists). If it does not exist, explain why.
> Find the limit (if it exists). If it does not exist, explain why.
> Find the limit (if it exists). If it does not exist, explain why.