Question: Refer to Exercise 7.59. a. Determine
Refer to Exercise 7.59.
a. Determine the probability distribution of smoke detectors.
b. What is the mean, variance, and standard deviation of the number of smoke detectors?
Data from Exercise 7.59:
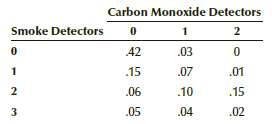
A fire inspector has conducted an extensive analysis of the number of smoke detectors and the number of carbon monoxide detectors in the homes in a large city. The analysis led to the creation of the following bivariate probability distribution.
Transcribed Image Text:
Carbon Monoxide Detectors Smoke Detectors 1 2 42 .03 1 .15 .07 .01 2 .06 .10 .15 .05 .04 .02 3.
> Refer to the example of HTSM Corp. in Appendix 19A and assume it is now 2018, three years after the defined benefit pension plan was initiated. In December 2018, HTSM’s actuary provided the company with an actuarial revaluation of the plan. The actuary’s
> Hass Foods Inc. sponsors a post-retirement medical and dental benefit plan for its employees. The company adopted the provisions of IAS 19 beginning January 1, 2017. The following balances relate to this plan on January 1, 2017: Plan assets…………………………………
> You are the auditor of Beaton and Gunter Inc., the Canadian subsidiary of a public multinational engineering company that offers a defined benefit pension plan to its eligible employees. Employees are permitted to join the plan after two years of employm
> Etienne Inc., a Canadian company traded on the Venture Exchange of the Toronto Stock Exchange, has sponsored a non-contributory defined benefit pension plan for its employees since 1992. Relevant information about the pension plan on January 1, 2017 is a
> Refer to the data in P19-9, except now assume Dela Corporation reports under IFRS. Depending on what your instructor assigns, do either parts (a), (b), (c), and (e) or parts (d) and (e). Data from P19-9: Dela Corporation initiated a defined benefit pen
> RWL Limited provides a long-term disability program for its employees through an insurance company. For an annual premium of $20,000, the insurance company is responsible for providing salary continuation to disabled employees on a long-term basis after
> The following are two independent situations related to future taxable and deductible amounts that resulted from temporary differences at December 31, 2017. In both situations, the future taxable amounts relate to property, plant, and equipment depreciat
> You, the ethical accountant, are the new controller at Pro Vision Corporation. It is January 2018 and you are currently preparing the December 31, 2017 financial statements. Pro Vision manufactures household appliances. It is a private company and has be
> Sarah Corp. reported the following differences between statement of financial position carrying amounts and tax bases at December 31, 2016: The differences between the carrying amounts and tax bases were expected to reverse as follows: Tax rates enac
> Andrew Weiman and Mei Lee are discussing accounting for income taxes. They are currently studying a schedule of taxable and deductible amounts that will arise in the future as a result of existing temporary differences. The schedule applies to a company
> The accounting records of Steven Corp., a real estate developer, indicated income before income tax of $850,000 for its year ended December 31, 2017, and of $525,000 for the year ended December 31, 2018. The following data are also available. 1. Steven C
> The following information applies to Edward Corporation, which reports under IFRS. 1. Prior to 2016, taxable income and accounting income were identical. 2. Accounting income was $1.7 million in 2016 and $1.4 million in 2017. 3. On January 1, 2016, equip
> The accounting income of Grace Corporation and its taxable income for the years 2017 to 2020 are as follows: The change in the tax rate from 25% to 30% was not enacted until early in 2018. Accounting income for each year includes an expense of $40,000
> Eloisa Corporation applies IFRS. Information about Eloisa Corporation’s income before income tax of $633,000 for its year ended December 31, 2017 includes the following: 1. CCA reported on the 2017 tax return exceeded depreciation reported on the income
> At December 31, 2016, Wright Corporation had a temporary difference (related to pensions) and reported a related deferred tax asset of $30,000 on its balance sheet. At December 31, 2017, Wright has five temporary differences. An analysis reveals the foll
> On December 31, 2016, Haley Inc. has taxable temporary differences of $2.2 million and a deferred tax liability of $616,000. These temporary differences are due to Haley having claimed CCA in excess of book depreciation in prior years. Haley’s year end i
> Aaron Engines Ltd. operates small engine repair outlets and is a tenant in several of Tran Holdings Inc.’s strip shopping malls. Aaron signed several lease renewals with Tran that each called for a three-month rent-free period. The leas
> Chen Corporation reported income before income tax for the year ended December 31, 2017 of $1,645,000. In preparing the 2017 financial statements, the accountant discovered an error that was made in 2016. The error was that a piece of land with a cost of
> The consolidated financial statements of Deutsche Lufthansa AG for the year ended December 31, 2014 are available in the company’s 2014 Annual Report on the www.lufthan sagroup.com website. Instructions: (a) What is included in the current liabilities f
> Access the annual report for Air Canada for its December 31, 2014 fiscal year end from SEDAR or the company’s website (www.aircanada.com). Also, access the annual report for the year ended December 31, 2014 for British Airways plc from the company’s pare
> Calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown in parentheses. a. Bank of Nova Scotia (BNS): 25%, Sun Energy (SU): 25%, Telus (T): 25%, George Weston (WN): 25% b. BNS: 10%, SU: 10%, T: 70%, WN
> Calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown in parentheses. a. Bank of Montreal (BMO): 25%, Magna International (MG): 25%, Power (POW): 25%, Rogers Communication (RCL.B): 25% b. BMO: 20%, M
> Calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown in parentheses. a. Canadian National Railway (CNR): 10%, Enbridge (ENB): 40%, Loblaw (L): 40%, Manulife Financial (MFC): 10% b. CNR: 50%, ENB: 30
> Calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown in parentheses. a. Agnico Eagle (AEM): 25%, Bell Canada Enterprises (BCE): 25%, Bank of Montreal (BMO: 25%, Dollarama (DOL): 25% b. AEM: 30%, BCE
> Refer to Exercise 7.85. Compute the correlation matrix of the returns of the four banks. Briefly describe what the correlations tell you. Data from Exercise 7.85: An analyst recommends that you invest in a portfolio made up of Bank of Montreal (BMO), Ban
> An analyst recommends that you invest in a portfolio made up of Bank of Montreal (BMO), Bank of Nova Scotia (BNS), Canadian Imperial Bank of Commerce (CM), and Royal Bank (RY). Why would it not useful in diversification?
> Refer to Exercise 6.83. Respondents in Greece, Hungary, and Poland were asked whether they approved or disapproved of the way the EU was dealing with the refugee issue. The number of respondents and the percentage opting for disapprove are listed here.
> In June 2016, Britons were heading to the polls to vote in a referendum to decide whether the United Kingdom would leave the European Union. Pew Research Center conducted surveys in European countries to determine opinions about the possible â€
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. United Health (UNH): 25%, United Technologies (UTX): 25%, Verizon (VZ): 25%, Walmart (WMT): 25% b. UNH: 10%, UTX: 20%, VZ: 30%, WMT: 40% c. U
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. Chevron (CVX): 25%, du Pont (DD): 25%, Procter & Gamble (PG): 25%, Travelers (TRV): 25% b. CVX: 50%, DD: 20%, PG: 15%, TRV: 15% c. CVX: 10%,
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. Coca Cola (KO): 40%, Pfizer (PFE): 20%, Verizon Communications (VZ): 40% b. KO: 60% PFE: 20%, (VZ): 20% c. KO: 10%, PFE: 30%, VZ: 60% d. Whic
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. General Electric (GE): 25%, Johnson & Johnson (JNJ): 25%, McDonald’s (MCD): 25%, Merck (MRK): 25% b. GE: 5%, JNJ: 30%, MCD: 40%, MRK: 25% c.
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. Chevron (CVX): 25%, Coca Cola (KO): 25%, Disney (DIS): 25%, Exxon Mobil (XOM): 25% b. CVX: 10%, KO: 20%, DIS: 30%, XOM: 40% c. CVX: 55%, KO:
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. 3M (MMM): 25%, Boeing (BA): 25%, Home Depot (HD): 25%, Travelers (TRV): 25% b. MMM: 10%, HD: 50%, IBM: 20%, TRV: 20% c. MMM: 30%, HD: 20%, IB
> calculate the mean and standard deviation of the portfolio. The proportions invested in each stock are shown. a. American Express (AXP): 20%, Goldman Sachs (GS): 30%, JP Morgan Chase (JPM): 50% b. AXP: 20%, GS: 60%, JPM: 20% c. AXP: 50%, GS: 30%, JPM: 20
> Researchers at the University of Pennsylvania School Of Medicine have determined that children under 2 years old who sleep with the lights on have a 36% chance of becoming myopic before they are 16 Children who sleep in darkness have a 21% of becoming my
> Refer to Exercise 7.73. Compute the expected value and standard deviation of the portfolio composed of 30% stock 1 and 70% stock 2. Data from Exercise 7.73: An investor is given the following information about the returns on two stocks: Stock 1 2 Mea
> Refer to Exercise 7.73. Compute the expected value and standard deviation of the portfolio composed of 60% stock 1 and 40% stock 2. The coefficient of correlation is .4. Data from Exercise 7.73: An investor is given the following information about the re
> An investor is given the following information about the returns on two stocks: a. If he is most interested in maximizing his returns, which stock should he choose? b. If he is most interested in minimizing his risk, which stock should he choose? St
> A portfolio is composed of two stocks. The proportion of each stock, their expected values, and standard deviations are listed next. For each of the following coefficients of correlation, calculate the expected value and standard deviation of the portfo
> Describe what happens to the expected value and standard deviation of the portfolio returns when the coefficient of correlation decreases.
> A professor of business statistics is about to begin work on a new research project. Because his time is quite limited, he has developed a PERT/CPM critical path, which consists of the following activities: 1. Conduct a search for relevant research artic
> In preparing to launch a new product, a marketing manager has determined the critical path for her department. The activities and the mean and variance of the completion time for each activity along the critical path are shown in the accompanying table.
> The operations manager of a large plant wishes to overhaul a machine. After conducting a PERT/CPM analysis he has developed the following critical path. 1. Disassemble machine 2. Determine parts that need replacing 3. Find needed parts in inventory 4. Re
> There are four activities along the critical path for a project. The expected values and variances of the completion times of the activities are listed here. Determine the expected value and variance of the completion time of the project. Expected Co
> Refer to Exercise 7.63. a. Determine the probability distribution of the total scores for both teams. b. Calculate the mean, variance, and standard deviation of the total scores for both teams. c. Calculate the covariance and coefficient of correlation o
> Refer to Exercise 7.63. a. Determine the probability distribution of the visiting team scores. b. Calculate the mean, variance, and standard deviation of the visiting team scores. Data from Exercise 7.63: After watching several seasons of soccer a statis
> Refer to Exercise 7.63. a. Determine the probability distribution of the home team scores. b. Calculate the mean, variance, and standard deviation of the home team scores. Data from Exercise 7.63: After watching several seasons of soccer a statistician p
> After watching several seasons of soccer a statistician produced the following bivariate distribution of scores. a. What is the probability that the home team wins? b. What is the probability of a tie? c. What is the probability that the visiting team w
> Refer to Exercise 7.59. a. Determine the probability distribution of carbon monoxide detectors. b. What is the mean, variance, and standard deviation of the number of carbon monoxide detectors? Data from Exercise 7.59: A fire inspector has conducted an e
> The mark on a statistics exam that consists of 100 multiple-choice questions is a random variable. a. What are the possible values of this random variable? b. Are the values countable? Explain. c. Is there a finite number of values? Explain. d. Is the ra
> The amount of money students earn on their summer jobs is a random variable. a. What are the possible values of this random variable? b. Are the values countable? Explain. c. Is there a finite number of values? Explain. d. Is the random variable discrete
> The distance a car travels on a tank of gasoline is a random variable. a. What are the possible values of this random variable? b. Are the values countable? Explain. c. Is there a finite number of values? Explain. d. Is the random variable discrete or co
> The number of accidents that occur on a busy stretch of highway is a random variable. a. What are the possible values of this random variable? b. Are the values countable? Explain. c. Is there a finite number of values? Explain. d. Is the random variable
> Refer to Exercise 7.59. a. What proportions of homes have one carbon monoxide detector and two smoke detectors? b. What proportion of homes with one carbon monoxide detector have two smoke detectors? c. What proportion of homes with two smoke detectors h
> Suppose that there are two people in a room. The probability that they share the same birthday (date, not necessarily year) is 1/365, and the probability that they have different birthdays is 364/365. To illustrate, suppose that you’re in a room with one
> In the last part of the 20th century, scientists developed the theory that the planet was warming and the primary cause was the increasing amounts of carbon dioxide (CO2), which is the product of burning oil, natural gas, and coal (fossil fuels). Althoug
> Did you conclude in Case 4.1 that the earth has warmed since 1880 and that there is some linear relationship between CO2 and temperature anomalies? If so, here is another look at the same data. C04-02a lists the temperature anomalies from 1880 to 1940, C
> Now that we have presented techniques that allow us to conduct more precise analyses, we’ll return to Case 3.1. Recall that there are two issues in this discussion. First, is there global warming and, second, if so, is carbon dioxide the cause? The only
> Since the 1960s, Québécois have been debating whether to separate from Canada and form an independent nation. A referendum was held on October 30, 1995, in which the people of Quebec voted not to separate. The vote was extremely close, with the “no” side
> The 2004–2005 hockey season was cancelled because of a player strike. The key issue in this labor dispute was a “salary cap.” The team owners wanted a salary cap to cut their costs. The owners of small-market teams wanted the cap to help their teams be c
> Adam Smith published The Wealth of Nations in 1776 in which he argued that when institutions protect the liberty of individuals, greater prosperity results for all. Since 1995, the Wall Street Journal and the Heritage Foundation, a think tank in Washingt
> Pregnant women are screened for a birth defect called Down syndrome. Down syndrome babies are mentally and physically challenged. Some mothers choose to abort the fetus when they are certain that their baby will be born with the syndrome. The most common
> Refer to Case 6.2. Another baseball strategy is to attempt to steal second base. Historically the probability of a successful steal of second base is approximately 68%. The probability of being thrown out is 32%. (We’ll ignore the relat
> No sport generates as many statistics as baseball. Reporters, managers, and fans argue and discuss strategies on the basis of these statistics. An article in Chance (“A Statistician Reads the Sports Page,” Hal S. Stern
> A fire inspector has conducted an extensive analysis of the number of smoke detectors and the number of carbon monoxide detectors in the homes in a large city. The analysis led to the creation of the following bivariate probability distribution. a. What
> A number of years ago, there was a popular television game show called Let’s Make a Deal. The host, Monty Hall, would randomly select contestants from the audience and, as the title suggests, he would make deals for prizes. Contestants would be given rel
> Refer to Exercises 7.82 and 7.83. a. Compute the expected value and variance of this portfolio: UNH: .191, UTX: .213, VZ: .370, WMT: .226 b. Can you do better? That is, can you find a portfolio whose expected value is greater than or equal to .0100 and w
> The number of magazine subscriptions per household is represented by the following probability distribution. a. Calculate the mean number of magazine subscriptions per household. b. Find the standard deviation. Magazine subscriptions per household 0
> Refer to Exercise 4.140. Suppose that in addition to recording the coffee sales, the manager also recorded the average temperature (measured in degrees Fahrenheit) during the game. These data together with the number of cups of coffee sold were recorded.
> Compute the coefficient of determination and the least squares line for Exercise 3.64. Compare this information with that developed by the scatter diagram alone. Data from Exercise Data from Exercise.64: One general belief held by observers of the busine
> The best way of winning at blackjack is to “case the deck,” which involves counting 10s, non-10s, and aces. For card counters, the probability of winning a hand may increase to 52%. Repeat Exercise 7.102 for a card counter. Data from Exercise 7.102: Repe
> Several books teach blackjack players the “basic strategy,” which increases the probability of winning any hand to 50%. Repeat Exercise 7.102, assuming the player plays the basic strategy. Data from Exercise 7.102: Repeat Exercise 7.100 using Excel. Data
> According to the American Academy of Cosmetic Dentistry, 75% of adults believe that an unattractive smile hurts career success. Suppose that 25 adults are randomly selected. a. What is the probability that 15 or more of them would agree with the claim? b
> The leading brand of dishwasher detergent has a 30% market share. A sample of 25 dishwasher detergent customers was taken. a. What is the probability that 10 or fewer customers chose the leading brand? b. What is the probability that 11 or more customers
> Repeat Exercise 7.106 using Excel. Data from Exercise 7.106: Suppose X is a binomial random variable with n = 25 and p = .7. Use Table 1 to find the following. a. P(X = 18) b. P(X = 15) c. P(X ≤ 20) d. P(X ≥ 16)
> Refer to Exercise 7.56. Find the following conditional probabilities. a. P(1 refrigerator ∣ 0 stoves) b. P(0 stoves ∣ 1 refrigerator) c. P(2 refrigerators ∣ 2 stoves) Data from Exercise 7.56: After analyzing several months of sales data, the owner of an
> Suppose X is a binomial random variable with n = 25 and p = .7. Use Table 1 to find the following. a. P(X = 18) b. P(X = 15) c. P(X ≤ 20) d. P(X ≥ 16)
> Repeat Exercise 7.103 using Excel. Data from Exercise 7.103: Given a binomial random variable with n = 6 and p = .2, use the formula to find the following probabilities. a. P(X = 2) b. P(X = 3) c. P(X = 5)
> Repeat Exercise 7.103 using Table 1 in Appendix B. Data from Exercise 7.103: Given a binomial random variable with n = 6 and p = .2, use the formula to find the following probabilities. a. P(X = 2) b. P(X = 3) c. P(X = 5)
> Repeat Exercise 7.100 using Excel. Data from Exercise 7.100: Given a binomial random variable with n = 10 and p = .3, use the formula to find the following probabilities. a. P(X = 3) b. P(X = 5) c. P(X = 8)
> Repeat Exercise 7.100 using Table 1 in Appendix B. Data from Exercise 7.100: Given a binomial random variable with n = 10 and p = .3, use the formula to find the following probabilities. a. P(X = 3) b. P(X = 5) c. P(X = 8)
> Refer to Exercise 7.97. a. Compute the expected value and variance of the portfolio described next. INTC: 20.9%, ORCL: 7.4%, SIRI: 11.9%, SBUX: 59.8% b. Can you do better? That is, can you find a portfolio whose expected value is greater than or equal to
> Refer to Exercise 7.92. a. Compute the expected value and variance of the portfolio described next. BNS: 44.0%, CNR: 27.5%, CTC.A: 21.9%, MG: 6.6% b. Can you do better? That is, can you find a portfolio whose expected value is greater than or equal to 1%
> During 2002 in the state of Florida, a total of 365,474 drivers were involved in car accidents. The accompanying table breaks down this number by the age group of the driver and whether the driver was injured or killed. (There were actually 371,877 accid
> Coin collecting is big business around the world. As an illustration, there are more than 500,000 American coins and more than 100,000 Canadian coins for sale/auction on Ebay. Moreover, there are dozens of other coin auctions every month. There are three
> One general belief held by observers of the business world is that taller men earn more money than shorter men. In a University of Pittsburgh study, 250 MBA graduates, all about 30 years old, were polled and asked to report their height (in inches) and t
> Canadians who visit the United States often buy liquor and cigarettes, which are much cheaper in the United States. However, there are limitations. Canadians visiting in the United States for more than 2 days are allowed to bring into Canada one bottle o
> In attempt to determine the factors that affect the amount of energy used, 200 households were analyzed. The number of occupants and the amount of electricity used were measured for each household. Produce a scatter diagram. What does the graph tell you
> Because inflation reduces the purchasing power of the dollar, investors seek investments that will provide higher returns when inflation is higher. It is frequently stated that common stocks provide just such a hedge against inflation. The annual percent
> a. Calculate the violent crime and property crime rates per 100,000 of population. b. Draw a line chart of the violent crime rate per 100,000 of population. c. Draw a line chart of the property crime rate per 100,000 of population. d. Describe your findi
> The number of customers entering a bank in the first hour of operation for each of the last 200 days was recorded. Draw a histogram and describe its shape.
> Refer to Exercise 6.11. a. What is the probability that a customer does not use a credit card? b. What is the probability that a customer pays in cash or with a credit card? c. Which method did you use in part (b)? Data from Exercise 6.11: Shoppers can p
> The distribution of the number of home runs in soft-ball games is shown here. a. Calculate the mean number of home runs. b. Find the standard deviation. Number of home runs 0 1 2 3 4 5 Probability .05 .16 41 .27 .07 .04
> How long does it take for someone to be deeply in debt? If it takes a long time we would expect AGE and DEBT to be related. Determine if they are by using a graphical technique. What have you learned?
> Are younger Americans more educated than older Americans? Answer the question by using a graphical technique to examine the relationship between AGE and EDUC. What does the graph tell you?
> It seems reasonable to believe that as one grows older one accumulates more money. To see if this is true use a graphical method to determine whether AGE and ASSETS are related. What did you discover?
> We expect that older respondents will have few, if any, children living in the household. Perform a statistical analysis to determine whether the age (AGE) of the respondent is linearly related to the number of children in the household (KIDS). Estimate
> After analyzing several months of sales data, the owner of an appliance store produced the following joint probability distribution of the number of refrigerators and stoves sold daily. a. Find the marginal probability distribution of the number of refr
> Repeat Exercise 4.152 using wage and salary income (WAGEINC) instead of income. Data from Exercise 4.152: Investigate the relationship between total household income (INCOME) and total value of household assets (ASSET). Conduct a statistical analysis to

