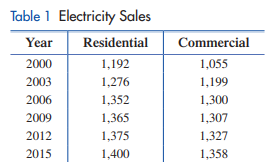
Question: Refer to the data in Table 1. (
Refer to the data in Table 1.
(A) Let x represent time (in years) with x = 0 corresponding to 2000, and let y represent the corresponding commercial sales. Enter the appropriate data set in a graphing calculator and find a quadratic regression equation for the data.
(B) If y = C(x) denotes the regression equation found in part (A), find C(30) and C′1302, and write a brief verbal interpretation of these results. Round answers to the nearest tenth of a billion.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. The derivative of a constant times a function is 0.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. The derivative of a quotient is the quotient of the derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives. y′ if y = (2x – 5)2
> Compute the probability of event E if the odds in favor of E are. Answer:
> Can a cubic polynomial function have more than two horizontal tangents? Explain.
> Now that you know how to find derivatives, explain why it is no longer necessary for you to memorize the formula for the x coordinate of the vertex of a parabola.
> Require the use of a graphing calculator. For each problem, find ’(x) and approximate (to four decimal places) the value(s) of x where the graph of f has a horizontal tangent line.
> Require the use of a graphing calculator. For each problem, find ’(x) and approximate (to four decimal places) the value(s) of x where the graph of f has a horizontal tangent line.
> Require the use of a graphing calculator. For each problem, find ’(x) and approximate (to four decimal places) the value(s) of x where the graph of f has a horizontal tangent line.
> Require the use of a graphing calculator. For each problem, find ’(x) and approximate (to four decimal places) the value(s) of x where the graph of f has a horizontal tangent line.
> If an object moves along the y axis (marked in feet) so that its position at time x (in seconds) is given by the indicated functions. Find (A) The instantaneous velocity function v = ’(x) (B) The velocity when x = 0
> If an object moves along the y axis (marked in feet) so that its position at time x (in seconds) is given by the indicated functions. Find (A) The instantaneous velocity function v = ’(x) (B) The velocity when x = 0
> Find (A) ï‚¦ï€ (x) (B) The slope of the graph of  at x = 2 and x = 4 (C) The equations of the tangent lines at x = 2 and x = 4 (D) The value(s) of x where the tangent line is horizontal
> Find (A) ï‚¦ï€ (x) (B) The slope of the graph of  at x = 2 and x = 4 (C) The equations of the tangent lines at x = 2 and x = 4 (D) The value(s) of x where the tangent line is horizontal
> Suppose that 6 people check their coats in a checkroom. If all claim checks are lost and the 6 coats are randomly returned, what is the probability that all the people will get their own coats back?
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Given the following probabilities for an event E, find the odds for and against E:
> Write the expression as a quotient of integers, reduced to lowest terms.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Refer to functions f and g that satisfy ’ (2) = 3 and g’ (2) = -1. In each problem, find h’ (2) for the indicated function h.
> Refer to functions f and g that satisfy ’ (2) = 3 and g’ (2) = -1. In each problem, find h’ (2) for the indicated function h.
> Refer to functions f and g that satisfy ’ (2) = 3 and g’ (2) = -1. In each problem, find h’ (2) for the indicated function h.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> Find the indicated derivatives.
> In a three-way race for the U.S. Senate, polls indicate that the two leading candidates are running neck-and-neck, while the third candidate is receiving half the support of either of the others. Registered voters are chosen at random and asked which of
> Find the indicated derivatives.
> Find the indicated derivatives. g′(x) for g(x) = x9
> Find the indicated derivatives. y′ for y = x8
> Find the indicated derivatives.
> Write the expression in the form a + b1n where a and b are reduced fractions and n is an integer
> Write the expression in the form a + b1n where a and b are reduced fractions and n is an integer
> Find the slope of the line through the given points. Write the slope as a reduced fraction, and also give its decimal form. Answer:
> Find the slope of the line through the given points. Write the slope as a reduced fraction, and also give its decimal form.
> The body temperature (in degrees Fahrenheit) of a patient t hours after taking a fever-reducing drug is given by (A) Use the four-step process to find F= (t). (B) Find F(3) and F= (3). Write a brief verbal interpretation of these results.
> Use the equally likely sample space in Example 2 to compute the probability of the following events: The number on the first die is even or the number on the second die is even.
> The U.S. consumption of refined copper (in thousands of metric tons) is given approximately by P(t) = 48t2 - 37t + 1,698 where t is time in years and t = 0 corresponds to 2010. (A) Use the four-step process to find p′(t). (B) Find the annual consumptio
> A company’s total sales (in millions of dollars) t months from now are given by S(t) = √t + 8 (A) Use the four-step process to find S′1t2. (B) Find S(9) and S′(9). Write a brief verbal interpretation of these results. (C) Use the results in part (B)
> The profit (in dollars) from the sale of x infant car seats is given by P(x) = 45x - 0.025x2 - 5,000 0 ≤ x ≤ 2,400 (A) Find the average change in profit if production is changed from 800 car seats to 850 car seats. (B) Use the four-step process to fin
> Repeat Problem 79 if the balloon is 1,024 feet above the ground when the ball is dropped. Data from Problem 79: A ball dropped from a balloon falls y = 16x2 feet in x seconds. If the balloon is 576 feet above the ground when the ball is dropped, when do
> Determine whether  is differentiable at x = 0 by considering
> Determine whether  is differentiable at x = 0 by considering (x) = x2/3
> Determine whether  is differentiable at x = 0 by considering (x) = 1 - | x |
> sketch the graph of  and determine where  is nondifferentiable.
> sketch the graph of  and determine where  is nondifferentiable.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If the graph of f has a sharp corner at x = a, then f is not continuous at x = a
> Refer to the description of a standard deck of 52 cards and Figure 4 on page 384. An experiment consists of dealing 5 cards from a standard 52-card deck. In Problems what is the probability of being dealt. Figure 4: 5 nonface cards.
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. if a function f is differentiable on the interval (a, b), then f is continuous on (a, b)
> Discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If (x) = mx + b is a linear function, then ’(x) = m.
> Let (x) = -x2 , g(x) = -x2 - 1, and h(x) = -x2 + 2. (A) How are the graphs of these functions related? How would you expect the derivatives of these functions to be related? (B) Use the four-step process to find the derivative of m(x) = -x2 + C, whe
> Repeat Problem 59 with (x) = 8x2 - 4x. Data from Problem 59: If an object moves along a line so that it is at y = (x) = 4x2 - 2x at time x (in seconds), find the instantaneous velocity function v = (x) and find the velocity at times x = 1, 3, and 5
> (A) Find = (x). (B) Find the slopes of the lines tangent to the graph of at x = 0, 2, and 4. (C) Graph and sketch in the tangent lines at x = 0, 2, and 4. (x) = 4x - x2 + 1
> Refer to the function F in the graph shown. Use the graph to determine whether F’(x) exists at each indicated value of x x = h
> Refer to the function F in the graph shown. Use the graph to determine whether F’(x) exists at each indicated value of x x = .
> Refer to the function F in the graph shown. Use the graph to determine whether F’(x) exists at each indicated value of x x = d
> Refer to the function F in the graph shown. Use the graph to determine whether F’(x) exists at each indicated value of x x = b
> suppose an object moves along the y axis so that its location is y = (x) = x2 + x at time x (y is in meters and x is in seconds). Find (A) The average velocity (the average rate of change of y with respect to x) for x changing from 2 to 4 seconds (B) T
> Use the equally likely sample space in Example 2 to compute the probability of the following events: A sum that is greater than 9.
> Refer to the graph of y = ( (x) = x2 + x shown. (A) Find the slope of the secant line joining (2, (2)) and (4, (4)). (B) Find the slope of the secant line joining (2, (2)) and (2 + h, ï&#
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Refer to the description of a standard deck of 52 cards and Figure 4 on page 384. An experiment consists of dealing 5 cards from a standard 52-card deck. what is the probability of being dealt. Figure 4: 5 Hearts?/
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> Use the four-step process to find ( = (x) and then find ( = (1) , ( = (2), and ( = (3).
> For ((x) = x4 , the instantaneous rate of change is known to be -4 at x = -1. Find the equation of the tangent line to the graph of y = ((x) at the point with x coordinate -1.
> For( (x) = 1 / + x2, the slope of the graph of y = ( (x) is known to be -0.16 at the point with x coordinate 2. Find the equation of the tangent line at that point.
> Four hours after the start of a 600-mile auto race, a driver’s velocity is 150 miles per hour as she completes the 352nd lap on a 1.5-mile track.
> Find the indicated quantities for f(x) = 3x2 .
>
> Use interval notation to specify the given interval.
> If the probability is .03 that an automobile tire fails in less than 50,000 miles, what is the probability that the tire does not fail in 50,000 miles?
> Use interval notation to specify the given interval.
> Use interval notation to specify the given interval.
> Use interval notation to specify the given interval. The set of all real numbers from -8 to -4, excluding -8 but including -4
> The graph shown represents the history of a person learning the material on limits and continuity in this book. At time t2, the student’s mind goes blank during a quiz. At time t4, the instructor explains a concept particularly well, th
> An office equipment rental and leasing company rents copiers for $10 per day (and any fraction thereof) or for $50 per 7-day week. Let C1x2 be the cost of renting a copier for x days.
> Table 2 shows the rates for natural gas charged by the Middle Tennessee Natural Gas Utility District during winter months. The base charge is a fixed monthly charge, independent of the amount of gas used per month. (A) Write a piecewise definition of t

