Question: Refer to the rectangles A, B, C,
Refer to the rectangles A, B, C, D, and E in the following figure.
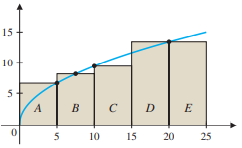
Which rectangles are both left and right rectangles?
> Use geometric formulas to find the unsigned area between the graph of y = (x) and the x axis over the indicated interval.
> Use geometric formulas to find the unsigned area between the graph of y = (x) and the x axis over the indicated interval.
> The number of children in a large city was found to increase and then decrease rather drastically. If the number of children (in millions) over a 6-year period was given by what was the average number of children in the city over the 6-year period? [Ass
> A drug is injected into the bloodstream of a patient through her right arm. The drug concentration in the blood stream of the left arm t hours after the injection is given by What is the average drug concentration in the bloodstream of the left arm dur
> The rate at which the area of a skin wound is increasing is given (in square centimeters per day) by A′(t) = -0.9e-0.1t . The initial wound has an area of 9 square centimeters. How much will the area change during the first 5 days? The second 5 days?
> In Problem 87, if the rate is found to be then approximately how many barrels of oil will the field produce during the first 5 years of production? The second 5Â years of production?
> Repeat Problem 85 with an order of 1,200 units every 4Â months. What is the average number of units on hand for a 4-month period? Data from Problem 85: A store orders 600 units of a product every 3 months. If the product is steadily depleted
> If the rate of labor use in Problem 83 is then approximately how many labor-hours will be required to assemble the 9th through the 27th control units? [Hint: Let a = 8 and b = 27.]
> Given the demand function find the average price (in dollars) over the demand interval [400, 600].
> Show that S = [x 1 - x 0 0], 0 ≤ x ≤ 1, is a stationary matrix for the transition matrix Discuss the generalization of this result to any absorbing Markov chain with two absorbing states and two nonadsorb
> The rate at which the total amount of money put into an advertising campaign is changing is given by A′(t) where t is the number of days since the campaign began. What does represent?
> The total cost (in dollars) of printing x dictionaries is C(x) = 20,000 + 10x. (A) Find the average cost per unit if 1,000 dictionaries are produced. (B) Find the average value of the cost function over the interval [0, 1,000]. (C) Discuss the differe
> The total accumulated costs C1t2 and revenues R1t2 (in thousands of dollars), respectively, for a coal mine satisfy C′(t) = 3 and R′(t) = 15e-0.1t where t is the number of years that the mine has been in operation. Find the useful life of the mine, to
> Refer to Problem 73. (A) Find a cubic regression equation for the data, and graph it and the data set in the same viewing window. (B) Use the regression equation and a numerical integration routine on a graphing calculator to approximate the number of
> Maintenance costs for an apartment house generally increase as the building gets older. From past records, the rate of increase in maintenance costs (in dollars per year) for a particular apartment complex is given approximately by where x is the age of
> Referring to Problem 69, compute the increase in cost going from a production level of 0 bikes per month to 600 bikes per month. Set up a definite integral and evaluate it. Data from Problem 69: A company manufactures mountain bikes. The research depart
> The fundamental theorem of calculus can be proved by showing that, for every positive integer n, there is a Riemann sum for f on [a, b] that is equal to F(b) – F(a). By the mean value theorem within each subinterval [xk - 1, xk] that be
> Use a numerical integration routine to evaluate each definite integral
> Use a numerical integration routine to evaluate each definite integral
> Evaluate the integrals.
> Verify the results in Problem 54 by computing Pk on a graphing calculator for large values of k.
> Evaluate the integrals.
> Evaluate the integrals.
> (A) Find the average value of each function over the indicated interval. (B) Use a graphing calculator to graph the function and its average value over the indicated interval in the same viewing window.
> (A) Find the average value of each function over the indicated interval. (B) Use a graphing calculator to graph the function and its average value over the indicated interval in the same viewing window.
> (A) Find the average value of each function over the indicated interval. (B) Use a graphing calculator to graph the function and its average value over the indicated interval in the same viewing window.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Repeat Problem 53 for Data From Problem 53: Step 1 Using a transition diagram, rearrange the columns and rows of P to produce a standard form for this chain. Step 2 Find the limiting matrix for this standard form. Step 3 Using a transition diagram, rev
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> Use a graphing calculator to approximate the limiting matrix for the indicated standard form.
> Evaluate the integrals in Problem.
> Evaluate the integrals in Problem.
> (A) Calculate the change in F(x) from x = 10 to x = 15. (B) Graph F′(x) and use geometric formulas (see the endpapers at the back of the book) to calculate the area between the graph of F′(x) and the x axis from x = 10 to x = 15. (C) Verify that your a
> (A) Calculate the change in F(x) from x = 10 to x = 15. (B) Graph F′(x) and use geometric formulas (see the endpapers at the back of the book) to calculate the area between the graph of F′(x) and the x axis from x = 10 to x = 15. (C) Verify that your a
> Use geometric formulas to find the unsigned area between the graph of y = (x) and the x axis over the indicated interval.
> Use geometric formulas to find the unsigned area between the graph of y = (x) and the x axis over the indicated interval.
> Refer to the rectangles A, B, C, D, and E in the following figure. Which rectangles are right rectangles?
> perform a mental calculation to find the answer and include the correct units. A square is circumscribed around a circle of radius 1 foot. Is the area inside the square but outside the circle less than 1 square foot?
> perform a mental calculation to find the answer and include the correct units. Find the total area enclosed by 5 non-overlapping rectangles, if each rectangle has width 3 feet and the heights of the rectangles are 2, 4, 6, 8, and 10 feet, respectively.
> Use a graphing calculator to approximate the limiting matrix for the indicated standard form.
> perform a mental calculation to find the answer and include the correct units. Find the total area enclosed by 6 non-overlapping rectangles, if each rectangle is 10 centimeters high and 3 centimeters wide.
> For the data in Problem 77, use left and right sums over three equal subintervals to approximate the area under the graph of N′(x) from x = 0 to x = 6. Replace the question marks with values of L3 and R3 as appropriate: Data from Probl
> Refer to Problem 75. Use left and right sums over five equal subintervals to approximate the area under the graph of A′(t) from t = 5 to t = 10. Calculate an error bound for this estimate. Data From Problem 75: The rate of healing, A&a
> For a new employee in Problem 73, use left and right sums to estimate the area under the graph of N(t) from t = 20 to t = 100. Use four equal subintervals for each. Replace the question marks with the values of L4 or R4 as appropriate:
> The left sum Ln or the right sum Rn is used to approximate the definite integral to the indicated accuracy. How large must n be chosen in each case? (Each function is increasing over the indicated interval.)
> the left sum Ln or the right sum Rn is used to approximate the definite integral to the indicated accuracy. How large must n be chosen in each case? (Each function is increasing over the indicated interval.)
> use derivatives to determine whether f is increasing or decreasing on the given interval. Use L4 or R4, whichever is appropriate, to give an overestimate of the signed area on the given interval.
> Use derivatives to determine whether  is increasing or decreasing on the given interval. Use L4 or R4, whichever is appropriate, to give an overestimate of the signed area on the given interval.
> Use L5 and R5 to approximate Compute error bounds for each. (Round answers to two decimal places.) Describe in geometric terms what the definite integral over the interval [1, 6] represents.
> Refer to the following figure showing two parcels of land along a river: Refer to Problem 61. Use R10 to estimate the combined area of both parcels, and calculate an error bound for this estimate. How many subdivisions of the baseline would
> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If a Markov chain is regular, then it is absorbing.
> could the given matrix be the transition matrix of an absorbing Markov chain?
> Discuss the validity of each statement. If the statement is always true, explain why. If it is not always true, give a counterexample. If ƒ is a decreasing function on [a, b], then the area under the graph of is greater than the left sum Ln and less th
> Discuss the validity of each statement. If the statement is always true, explain why. If it is not always true, give a counterexample. If (x)= 2x on [0, 10] and n is a positive integer, then there is some Riemann sum Sn that equals the exact area under
> Discuss the validity of each statement. If the statement is always true, explain why. If it is not always true, give a counterexample.
> calculate the definite integral, given that
> calculate the definite integral, given that
> calculate the definite integral, given that
> calculate the definite integral, given that
> calculate the definite integral, given that
> calculate the definite integral, given that
> calculate the definite integral by referring to the figure with the indicated areas.
> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If a Markov chain is absorbing, then it has a unique stationary matrix.
> calculate the definite integral by referring to the figure with the indicated areas.
> calculate the definite integral by referring to the figure with the indicated areas.
> calculate the definite integral by referring to the figure with the indicated areas.
> calculate the definite integral by referring to the figure with the indicated areas.
> calculate the definite integral by referring to the figure with the indicated areas.
> calculate the indicated Riemann sum Sn for the function (x) = x2 - 5x - 6. Partition 31, 74 into six subintervals of equal length, and let c1 = 2, c2 = 2, c3 = 4, c4 = 4, c5 = 6, and c6 = 6.
> calculate the indicated Riemann sum Sn for the function (x) = x2 - 5x - 6. Partition 30, 34 into three subintervals of equal length, and let c1 = 0.2, c2 = 1.5, and c3 = 2.8.
> calculate the indicated Riemann sum Sn for the function (x) = 25 - 3x2 . Partition 3-5, 54 into five subintervals of equal length, and for each subinterval [xk - 1, xk], let ck = (xk - 1 + xk) /2.
> calculate the indicated Riemann sum Sn for the function (x) = 25 - 3x2 . Partition 30, 124 into four subintervals of equal length, and for each subinterval [xk - 1, xk], let ck = (xk - 1 + 2xk) /3.
> Involve estimating the area under the curves in Figures A–D from x = 1 to x = 4. For each figure, divide the interval [1, 4] into three equal subintervals. Compute error bounds for L3 and R3 found in Problem 18 for both figures.
> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If a Markov chain has exactly three states, one nonabsorbing and two absorbing, then it is an absorbing chain.
> Involve estimating the area under the curves in Figures A–D from x = 1 to x = 4. For each figure, divide the interval [1, 4] into three equal subintervals. Replace the question marks with L3 and R3 as appropriate. Explain your choice.
> Involve estimating the area under the curves in Figures A–D from x = 1 to x = 4. For each figure, divide the interval [1, 4] into three equal subintervals. Using the results of Problem 16, compute L3 and R3 for Figure C and for Figure D.
> Involve estimating the area under the curves in Figures A–D from x = 1 to x = 4. For each figure, divide the interval [1, 4] into three equal subintervals. Draw in left and right rectangles for Figures C and D.
> Refer to the rectangles F, G, H, I, and J in the following figure. Which rectangles are neither left nor right rectangles?
> Refer to the rectangles F, G, H, I, and J in the following figure. Which rectangles are left rectangles?
> Find the general or particular solution, as indicated, for each first-order differential equation.
> Express the relationship between = (x) and (x) in words, and write a differential equation that (x) satisfies. For example, the derivative of (x) = e3x is 3 times ï
> Express the relationship between = (x) and (x) in words, and write a differential equation that (x) satisfies. For example, the derivative of (x) = e3x is 3 times ï
> Express the relationship between = (x) and (x) in words, and write a differential equation that (x) satisfies. For example, the derivative of (x) = e3x is 3 times ï
> Express the relationship between = (x) and (x) in words, and write a differential equation that (x) satisfies. For example, the derivative of (x) = e3x is 3 times ï
> discuss the validity of each statement. If the statement is always true, explain why. If not, give a counterexample. If a Markov chain has exactly two states and at least one absorbing state, then it is an absorbing chain.
> The Weber–Fechner law concerns a person’s sensed perception of various strengths of stimulation involving weights, sound, light, shock, taste, and so on. One form of the law states that the rate of change of sensed sen
> For a person learning to type, the number N of words per minute that the person could type after t hours of practice was given by the limited growth function N = 1001(1 - e-0.02t ) What is the rate of improvement after 10 hours of practice? After 40 ho

