Question: The director of a pharmacy department is
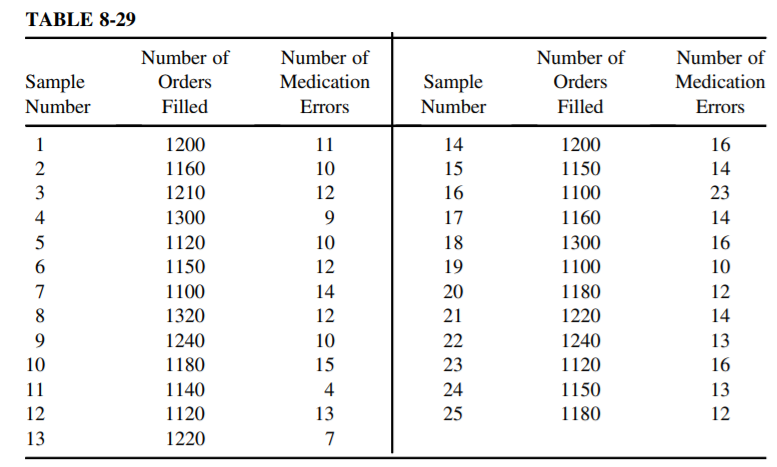
The director of a pharmacy department is interested in benchmarking the level of operations in the unit. The director has defined medication errors as being any one of the following: wrong medication; wrong dose; administered to the wrong patient; administered at the wrong time; incorrectly repeating the medication; or omitting the medication. The number of orders filled per day by the pharmacy varies. Table 8-29 shows the number of orders filled and the number of medication errors for 25 days. Construct an appropriate control chart and comment on the stability of the process.
> In solar cells, the exposed surface area is the characteristic of interest. The tolerances on the length and width of the cells are 4 ±0.06 cm and 5± 0.09 cm, respectively. Assuming these dimensions to be independent of each other and each normally distr
> A cylindrical piece is used in an assembly in which the weight is to be controlled. The tolerances on diameter and height, on the basis of five observations, are 2 ±0.06 cm and 6 ±0.06 cm, respectively. Assume that the dimensions are independent of each
> Consider the data on call waiting time of customers in a call center. The call center has set a goal of waiting time not to exceed 35 seconds. (a) Test to see (using α =0.05) if conducting capability analysis using normal distribution is appropriate. (b)
> Measurements on the pH values of a chemical compound are taken at random by two operators. Fifteen samples are randomly chosen, with each operator measuring each sample twice. The data are shown in Table 9-10. Specifications on pH are 6.5 ±
> A financial institution wants to improve proposal preparation time for its clients. Discuss the actions to be taken in reducing the average preparation time and the variability of preparation times.
> A logistics firm has identified four operations, which are to be conducted in succession, for an order to be processed. The tolerances (in hours) are shown in Table 9-9. Assume that the tolerances are independent of each other and that the time in each p
> In a piston assembly, the specifications for the piston diameter are 12 ±0.5 cm, and those for the cylinder diameter are 12.10 ±0.4 cm. Assume that the natural tolerance limits coincide with the specifications. A clearance fit is required for the assembl
> Refer to Exercise 9-30. If there is too much clearance between the hole and the shaft, a wobble will result. Clearances above 0.05 cm are not desirable and cause a wobble. Find the probability of a wobble. Data from Exercise 9-30: The specifications for
> The specifications for a shaft diameter in an assembly are 5± 0.03 cm, and those for the hole are 5.25 ±0.08 cm. If the assembly is to have a clearance of 0.18 ±0.05 cm, what proportion of the assemblies will be acceptable?
> Consider Figure 9-16, which shows the assembly of a shaft in a bearing. The specifications for the shaft diameter are 6± 0.06 cm, and those for the hole diameter are 6.2± 0.03 cm. (a) Find the probability of the assembly havin
> Four metal plates, each of thickness of 3 cm, are welded together to form a subassembly. The specifications for the thickness of each plate are 3± 0.2 cm. Assuming the weld thickness to be negligible, determine the tolerances for the assembly thickness.
> Consider the two-component assembly shown in Figure 9-15. Suppose that the mean lengths are given as μ1=14 cm and μ2=8 cm. Assuming that the specifications for Y are 6± 0.2 cm, what are the tolerances for X1 and
> Consider the two-component assembly shown in Figure 9-15. Suppose that the specifications for the dimension X2 are 5 ±0.05 cm and those for X1 are 12± 0.15 cm. Find the specifications for the dimension Y. Assume that the speci
> Refer to Exercise 9-24. Suppose that the specifications for the assembly length are 35± 0.3 cm and that the tolerances of A and C are equal but those for B and D are each twice as large as that for A. In addition, assume that the specificat
> Refer to the four-component assembly shown in Figure 9-14. Assume that the length of each component is normally and independently distributed with the means shown in Table 9-8. The specifications for the assembly length are 35 ±0.5 cm. Assu
> What are the advantages and disadvantages of using variables rather than attributes in control charts?
> In Exercise 9-22, suppose that the specifications for the gap are 1.05± 0.15 cm. An assembly with a gap exceeding the upper specification limit is scrapped, whereas that with a gap less than the lower specification limit can be reworked to
> Consider the assembly of three components shown in Figure 9-19. The tolerances for these three components are given in Table 9-7. Assume that the tolerances on the components are independent of each other and that the lengths of the components are normal
> The amount of a preservative added to dairy products should not exceed certain levels of 23 ±6 mg (set by the Food and Drug Administration). Samples of size 5 of processed cheese produced the values of the average and range shown in Table 9
> The waiting time in minutes before being served in a local post office is observed for 50 randomly chosen customers: (a) Test for normality using α= 0.05. What inferences can you draw? (b) Estimate the mean and standard deviation of the wai
> The diameter of a forged part has specifications of 120± 5 mm. A sample of 25 parts chosen from the process gives a sample mean of 122 mm with a sample standard deviation of 2 mm. (a) Find the Cpk index for the process and comment on its value. What is t
> Refer to Exercise 9-13. Find the process capability index Cpk and comment on process performance. If the target value is 47.5 ppm, find the Cpm and Cpmk indices and comment on their values. If the process center is shifted to the midpoint between the spe
> The emergency service unit in a hospital has a goal of 3.5 minutes for the waiting time of patients before being treated. A random sample of 20 patients is chosen and the sample average waiting time is found to be 2.3 minutes with a sample standard devia
> For Exercise 9-13, find the Cp index. Comment on the ability of the process to meet specifications. What proportion of the specification range is used up by the process? If it is easier to change the process mean than to change its variability, to what v
> How are rational samples selected? Explain the importance of this in the total quality systems approach.
> A pharmaceutical company producing vitamin capsules desires a proportion of calcium content between 40 and 55 ppm. A random sample of 20 capsules chosen from the output yields a sample mean calcium content of 44 ppm with a standard deviation of 3 ppm. Fi
> The marketing department of a large organization is interested in being responsive to its customers’ needs. Accordingly, each week it monitors the number of complaints received. It is possible for a customer to have more than one compla
> A major airline surveys passengers to monitor the satisfaction level of its customers. Even though the airline uses a five-point Likert scale (5: outstanding service; 4: good; 3: average; 2: below average; 1: poor), it wants to monitor the proportion tha
> An organization that accepts orders via the Web, processes it, and has delivery scheduled based on its inventory levels is known to be proficient in its service. Errors in orders received could be due to wrong shipment of product, incorrect quantity, or
> Data from a city bank showing monthly loan applications for home mortgage and the number rejected are given in Table 8-36. Construct an appropriate control chart and comment on the loan approval process.
> In a health care facility, it is known that nosocomial infections are rare. Table 8-35 shows the number of admissions between occurrences of nosocomial infections. Construct an appropriate control chart and comment on the performance of the health care t
> Mortality data for patients in the intensive care unit (ICU) in a health care facility, collected over 8-hour intervals, are shown in Table 8-34. Since patients have varying risks, the predicted mortality from a logistic regression model that uses the pa
> The JCAHO has standards pertaining to patient restraint use. A checklist has been developed that is to be used each time a restraint is used. The checklist contains five items, all of which should be checked. Table 8-33 shows data collected for 25 months
> Consider Exercise 8-38. However, now assume that the interval between complications follows an exponential distribution. Construct an appropriate control chart and comment on the process assuming a type I error rate of 0.005. Data from Exercise 8-38: Th
> Consider Exercise 8-38 under the assumption that the complication rate is 0.1% and a type I error of 0. 005.If you reduced the upper control limit to half of its previous value, what type of process complication rates, on average, will this new limit be
> Discuss the relationship between ARL and type I and II errors.
> Consider Exercise 8-38. Determine the sensitivity of the control limits complication rate using values of 0.2% and 0.5%. Data from Exercise 8-38: The number of heart surgery complications is rare. To monitor the effectiveness of such surgeries, data are
> Consider Exercise 8-38 under the assumption that the complication rate is 0.1%. If you were to construct a p-chart using two-sided 3σ limits, what would the minimum sample size be to detect an improvement in the process? Data from Exercise 8-
> Consider Exercise 8-38. If you were interested in detecting an improvement in the process using a one-sided limit, what should the minimum sample size be for an α of 0.005? What should it be for an α of 0.05? What conclusions
> The number of heart surgery complications is rare. To monitor the effectiveness of such surgeries, data are recorded on the number of such procedures until a complication occurs. These complications occur independently with a constant probability of occu
> Refer to Exercise 8-36. Set up 2σ control limits. What is the probability of detecting a change in the process average number of dietary errors per 100 trays to 8 on the first sample drawn after the change? Explain under what conditions you wo
> Refer to Exercise 8-27. Construct an OC curve for the c-chart. If the process average number of dietary errors per 100 trays increases to 10, what is the probability of detecting this on the first sample drawn after the change? Data from Exercise 8-27:
> Refer to Exercise 8-18. Construct an OC curve for the p-chart. If the process proportion of dissatisfied customers were to rise to 7%, what is the probability of not detecting this shift on the first sample drawn after the change has taken place? What is
> The Joint Commission on Accreditation of Healthcare Organizations (JCAHO) requires an accounting of significant medication errors. Data collected over the last 25 months, shown in Table 8-31, indicate the number of orders filled and the number of signifi
> Refer to Exercise 8-32. If the weighting systems were different (i.e., 10, 5, and 1), how would the centreline and the control limits change for the U-chart? Discuss changes, if any, in the inferences made about the process. Data from Exercise 8-32: Non
> Nonconformities in automobiles fall into three categories: serious, major, and minor. Twenty-five samples of five automobiles are chosen, and the total number of nonconformities in each category is reported. Table 8-30 shows the results. Assuming a weigh
> Describe the role of the average run length (ARL) in the selection of control chart parameters. Explain how ARL influences sample size.
> Refer to Exercise 8-29. If we want to control the number of imperfections per 100 m2, how would this affect the control chart? What would the control limits be? In terms of decision making, would there be a difference between this problem and Exercise 8-
> The number of imperfections in bond paper produced by a paper mill is observed over a period of several days. Table 8-28 shows the area inspected and the number of imperfections for 25 samples. Construct a control chart for the number of imperfections pe
> A building contractor subcontracts to a local merchant a job involving hanging wallpaper. To have an idea of the quality level of the merchant’s work, the contractor randomly selects 300 m2 and counts the number of blemishes. The total number of blemishe
> The number of dietary errors is found from a random sample of 100 trays chosen on a daily basis in a health care facility. The data for 25 such samples are shown in Table 8-27. (a) Construct an appropriate control chart and comment on the process. (b) Ho
> The number of processing errors per 100 purchase orders is monitored by a company with the objective of eliminating such errors totally. Table 8-26 shows samples that were selected randomly from all purchase orders. The company is in the process of testi
> Refer to Exercise 8-18 and the data shown in Table 8-21. Construct a control chart for the number of dissatisfied customers. Revise the chart, assuming special causes for points outside the control limits. Data from Exercise 8-18: The number of customer
> A health care facility is interested in monitoring the primary C-section rate. Monthly data on the number of primary C-sections collected over the last two and a half years is shown in Table 8-25. (a) Is the process in control? (b) There is pressure to m
> The quality of service in a hospital is tracked by determining the proportion of medication errors; this is done by dividing the number of medication errors by 1000 patient-days for each observation. The results of 25 such samples (in percentage of medic
> What is the utility of the operating characteristic curve? How can the discriminatory power of the curve be improved?
> Observations are taken from the output of a company making semiconductors. Table 8-23 shows the sample size and the number of nonconforming semiconductors for each sample. Construct a p-chart by setting up the exact control limits for each sample. Are an
> Health care facilities must conform to certain standards in submitting bills to Medicare/Medicaid for processing. The number of bills with errors and the number sampled are shown in Table 8-22. Construct an appropriate control chart and comment on the pe
> Refer to Exercise 8-18. Management believes that the dissatisfaction rate is 3%, so establish control limits based on this value. Comment on the ability of the store to meet this standard. If management were to set the standard at 2%, can the store meet
> The number of customers who are not satisfied with the service provided in a retail store is found for 20 samples of size 100 and is shown in Table 8-21. Construct a control chart for the proportion of dissatisfied customers. Revise the control limits, a
> Every employee in a check-processing department goes through a four-month training period, after which the employee is responsible for their operation. The work of one employee who has been on the job for eight months is being studied. Table 8-20 shows t
> For the patient data in Exercise 7-52 as shown in Table 7-32, construct a risk-adjusted exponentially weighted moving average (RAEWMA) chart using a weighting constant of 0.2 and a false-alarm rate of 0.10. Comment on the performance of the surgical team
> Refer to Exercise 7-52 and the data in Table 7-32 on cardiac surgery patients. Conduct a risk-adjusted sequential probability ratio test using a false-alarm rate of 0.005. Assume that the chance of failing to detect a decrease in the odds ratio of mortal
> The predicted mortality of cardiac surgery patients in an intensive care unit, based on their APACHE score, is found based on a logistic regression model applied to patients over a four-year period. Table 7-32 shows the predictive pre-operative mortality
> Refer to Exercise 7-49. Construct an exponentially weighted moving-average chart using a weighting constant of 0.2 for the weekly amount invested. Comment on the process. If it is desired to have a goal value of $1.5M for the standard deviation, using th
> Refer to Exercise 7-49 and the revised process. Is this process capable of meeting a goal value of $1.5M for the standard deviation of the weekly amount invested? Construct an appropriate chart and discuss. Data from Exercise 7-49: An investment bank is
> What are warning limits and what purpose do they serve?
> An investment bank is interested in monitoring the weekly amount (in millions of dollars) invested in volatile stocks so as to maintain a stable rate of return on a selected fund. Table 7-31 shows 25 consecutive weeks of the amount invested ($M) in the f
> Refer to Exercise 7-46. Construct an exponentially weighted moving-average chart using a weighting constant of 0.2 for the decision-making process and comment. Is the existing process capable of meeting a goal value of 12 weeks? Data from Exercise 7-46:
> Refer to Exercise 7-46. Demonstrate if the existing process in making a decision on loan applications is capable of meeting a goal value of 12 weeks. Assuming a normal distribution of the decision-making time, what proportion of the applications will not
> The time to evaluate and make a decision on mortgage loan applications is being examined in a financial institution. Twenty-five mortgage applications are selected from the previous month and the decision-making times, in days, are shown in Table 7-30. C
> Consider the data on 25 patients, of a certain diagnosis-related group, on systolic blood pressure, blood glucose level, and total cholesterol level as shown in Table 4-5. The table shows values on these variables before and after administration of a cer
> Consider the data on the parameters in a chemical process of temperature, pressure, proportion of catalyst, and pH value of mixture as indicated in Table 3-15. (a) Construct a Hotelling’s T2 -chart and comment on process stability. Whic
> It is desired to monitor project completion times by analysts in a consulting company. The magnitude and complexity of the project influence the completion time. It is also believed that the variability in completion time increases with the magnitude of
> A component to be used in the assembly of a transmission mechanism is manufactured in a process for which the two quality characteristics of tensile strength (X1) and diameter (X2) are of importance. Twenty samples, each of size 4, are obtained from the
> Refer to Exercise 7-38 and the data for the percentage of potassium content in a compound. Suppose that we wish to detect an out-of-control condition with a probability of 0.90 if the process is producing at a non-conformance rate of 4%. Determine the ac
> Define and explain type I and type II errors in the context of control charts. Are they related? How does the choice of control limits influence these two errors?
> The percentage of potassium in a compound is expected to be within the specification limits of 18–35%. Samples of size 4 are selected, and the mean and range of 25 such samples are shown in Table 7-27. It is desirable for the process no
> The time from product inception and design to marketing and sales is important for application software in mobile devices. For the last 20 products, Table 7-26 shows such times (in weeks) for a software organization. Construct an appropriate control char
> Consider the data on the waiting time (seconds) of customers before speaking to a representative at a call center, shown in Exercise 5-9. Data from Exercise 5-9: A random sample of 50 observations on the waiting time (in seconds) of customers before sp
> Consider the data on purchase order processing time for customers shown in Table 4-6. Construct an individuals and a moving-range chart on the data before process improvement changes are made and comment on process stability. Data from Table 4-6:
> The bending strength of the poles is a consideration to a manufacturer of fiberglass fishing rods. Samples are chosen from the process, and the average bending strength of four samples is found. The target mean bending strength is 30 kg, with a process s
> Automatic machines that fill packages of all-purpose flour to a desired standard weight need to be monitored closely. A random sample of four packages is selected and weighed. The average weight is then computed. Observations from 15 such samples are sho
> In a gasoline-blending plant, the quality of the output as indicated by its octane rating is measured for a sample taken from each batch. The observations from 20 such samples are shown in Table 7-23. Construct a chart for the moving range of two success
> Explain the rationale behind placing the control limits at three standard deviations from the mean.
> The level of dissolved oxygen in water was measured every 2 hours in a river where industrial plants discharge processed waste. Each observation consists of four samples, from which the sample mean and range of the amount of dissolved oxygen in parts per
> The bore size on a component to be used in assembly is a critical dimension. Samples of size 4 are collected and the sample average diameter and range are calculated. After 25 samples, we have The specifications on the bore size are 4.4 ±0.
> In a textile company, it is important that the acidity of the solution used to dye fabric be within certain acceptable values. Data values are gathered for a control chart by randomly taking four observations from the solution and determining the average
> Flight delays are of concern to passengers. An airline obtained observations on the average and range of delay times of flights (in minutes), each chosen from a sample of size 4, as shown in Table 7-20. Construct appropriate control charts and comment on
> Explain the difference between common causes and special causes. Give examples of each.
> Consider the hospitality industry. Describe special causes and common causes in this setting and discuss the role of quality control and quality improvement.
> The turnaround time (TAT) for a certain blood test in a laboratory in a health care facility is of interest. The mean and standard deviation of a random sample of 100 such tests are found to be 120 minutes and 20 minutes, respectively. Find the 3σ contro
> A health care facility is monitoring daily expenditures for a certain diagnosis-related group (DRG). Individual observations are selected. After 50 samples, the average and standard deviation of daily expenditures (in hundreds of dollars) are estimated t
> The time to deliver packaged containers by a logistics company is found from samples of size 4. The mean and standard deviation of delivery times is estimated to be 140 hours and 6 hours, respectively. (a) Find the 2σ and 3σ control limits for the averag
> A manager is contemplating using Rules 1 and 4 for determining out-of-control conditions. Suppose that the manager constructs 3σ limits. (a) What is the overall type I error probability assuming independence of the rules? (b) On average, how many samples
> Suppose 3σ control limits are constructed for the average temperature in a furnace. Samples of size 4 were selected with the average temperature being 5000°C and a standard deviation of 50°C. (a) Find the 3σ control charts. (b) Suppose that Rules 2 and 3
> The tensile strength of nonferrous pipes is of importance. Samples of size 5 are selected from the process output, and their tensile strength values are found. After 30 such samples, the process mean strength is estimated to be 3000 kg with a standard de
> The length of industrial filters is a quality characteristic of interest. Thirty samples, each of size 5, are chosen from the process. The data yield an average length of 110 mm, with the process standard deviation estimated to be 4 mm. (a) Find the warn
> The diameter of a part produced by an automatic machine is a characteristic of interest. Based on historical data, the process average diameter is 15 mm with a process standard deviation of 0.8 mm. If samples of size 4 are randomly selected from the proc
> What is meant by an overall type I error rate? If Rules 1, 2, and 3 of this chapter are used simultaneously, assuming independence, what is the probability of an overall type I error if 3σ control limits are used?

