Question: A lithium atom has three electrons, and
A lithium atom has three electrons, and the 2S1/2 ground-state electron configuration is 1s22s. The 1s22p excited state is split into two closely spaced levels, 2P3/2 and 2P1/2, by the spin-orbit interaction (see Example 41.7 in Section 41.5). A photon with wavelength 67.09608 mm is emitted in the 2P3/2 2S1/2 transition, and a photon with wavelength 67.09761 mm is emitted in the 2P1/2 2S1/2 transition. Calculate the effective magnetic field seen by the electron in the 1s22p state of the lithium atom. How does your result compare to that for the 3p level of sodium found in Example 41.7?
From Example 41.7
Transcribed Image Text:
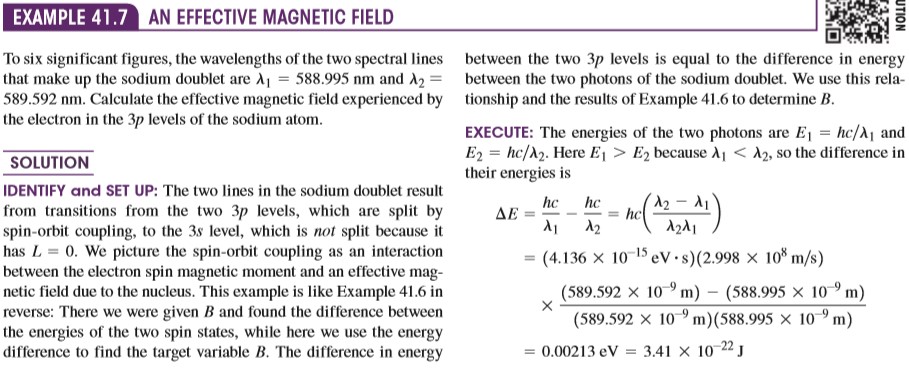
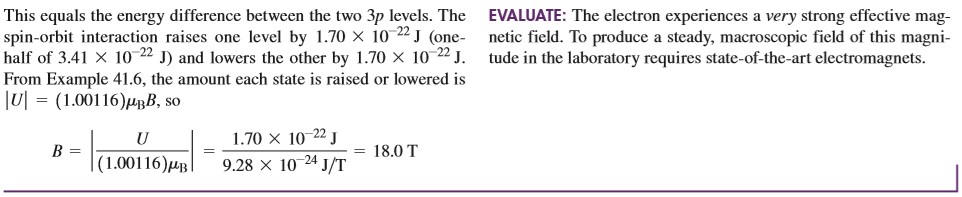
EXAMPLE 41.7 AN EFFECTIVE MAGNETIC FIELD To six significant figures, the wavelengths of the two spectral lines between the two 3p levels is equal to the difference in energy that make up the sodium doublet are A = 588.995 nm and A2 = between the two photons of the sodium doublet. We use this rela- 589.592 nm. Calculate the effective magnetic field experienced by tionship and the results of Example 41.6 to determine B. the electron in the 3p levels of the sodium atom. EXECUTE: The energies of the two photons are E hc/A1 and E2 = hc/A2. Here E, > E2 because A1 < ^2, so the difference in their energies is SOLUTION IDENTIFY and SET UP: The two lines in the sodium doublet result hc hc = hc 12 from transitions from the two 3p levels, which are split by spin-orbit coupling, to the 3s level, which is not split because it has L = 0. We picture the spin-orbit coupling as an interaction between the electron spin Magnetic Moment and an effective mag- netic field due to the nucleus. This example is like Example 41.6 in reverse: There we were given B and found the difference between the energies of the two spin states, while here we use the energy difference to find the target variable B. The difference in energy ΔΕ = (4.136 x 10-15eV•s)(2.998 × 1o* m/s) (589.592 x 109 m) - (588.995 x 109 m) (589.592 × 10-9 m)(588.995 × 109 m) = 0.00213 eV = 3.41 X 10 22 J UTION This equals the energy difference between the two 3p levels. The spin-orbit interaction raises one level by 1.70 X 10 22 J (one- half of 3.41 X 10 22 J) and lowers the other by 1.70 X 10 22 J. From Example 41.6, the amount each state is raised or lowered is |U| = (1.00116)µBB, so EVALUATE: The electron experiences a very strong effective mag- netic field. To produce a steady, macroscopic field of this magni- tude in the laboratory requires state-of-the-art electromagnets. 1.70 x 10 22 J 9.28 x 10 24 J/T U 18.0 T B = (1.00116)µB =
> Calculate the threshold kinetic energy for the reaction p + p → p + p + K+ + K- if a proton beam is incident on a stationary proton target.
> A proton and an antiproton collide head-on with equal kinetic energies. Two γ rays with wavelengths of 0.720 fm are produced. Calculate the kinetic energy of the incident proton.
> Beams of π- mesons are used in radiation therapy for certain cancers. The energy comes from the complete decay of the π- to stable particles. a. Write out the complete decay of a π- meson to stable particles. What are th
> In your job as a health physicist, you measure the activity of a mixed sample of radioactive elements. Your results are given in the table. Time (h)………….Decays,s 0………………7500 0.5……………4120 1.0…………….2570 1.5……………1790 2.0……………1350 2.5……………1070 3.0……………..872
> Your company develops radioactive isotopes for medical applications. In your work there, you measure the activity of a radioactive sample. Your results are given in the table. Time (h)…..Decays,s 0……………20,000 0.5………….14,800 1.0………….11,000 1.5…………….8130 2
> As a scientist in a nuclear physics research lab, you are conducting a photodisintegration experiment to verify the binding energy of a deuteron. A photon with wavelength l in air is absorbed by a deuteron, which breaks apart into a neutron and a proton.
> Consider the fusion reaction 1 2
> In the 1986 disaster at the Chernobyl reactor in eastern Europe, about 1/8 of the 137Cs present in the reactor was released. The isotope 137Cs has a half-life of 30.07 y for b decay, with the emission of a total of 1.17 MeV of energy per decay. Of this,
> A bone fragment found in a cave believed to have been inhabited by early humans contains 0.29 times as much 14C as an equal amount of carbon in the atmosphere when the organism containing the bone died. (See Example 43.9 in Section 43.4.) Find the approx
> An atom in its ground level absorbs a photon with energy equal to the K absorption edge. Does absorbing this photon ionize this atom? Explain.
> Nuclear weapons tests in the 1950s and 1960s released significant amounts of radioactive tritium ( 1 3
> A 60Co source with activity 2.6 * 10-4 Ci is embedded in a tumor that has mass 0.200 kg. The source emits g photons with average energy 1.25 MeV. Half the photons are absorbed in the tumor, and half escape. a. What energy is delivered to the tumor per s
> The nucleus 8 15
> Measurements indicate that 27.83% of all rubidium atoms currently on the earth are the radioactive 87Rb isotope. The rest are the stable 85Rb isotope. The half-life of 87Rb is 4.75 * 1010 y. Assuming that no rubidium atoms have been formed since, what pe
> A 70.0-kg person experiences a whole-body exposure to α radiation with energy 4.77 MeV. A total of 7.75 * 1012 α particles are absorbed. a. What is the absorbed dose in rad? b. What is the equivalent dose in rem? c. If the source is 0.0320 g of 226Ra
> In 1952 spectral lines of the element technetium-99 (99Tc) were discovered in a red giant star. Red giants are very old stars, often around 10 billion years old, and near the end of their lives. Technetium has no stable isotopes, and the half-life of 99T
> A person ingests an amount of a radioactive source that has a very long lifetime and activity 0.52 µCi. The radioactive material lodges in her lungs, where all of the emitted 4.0-MeV α particles are absorbed within a 0.50-kg mass of tissue. Calculate the
> Calculate the mass defect for the β+ decay of 6 11
> A neutral pion (π0) has a mass of 264 times the electron mass and decays with a lifetime of 8.4 * 10-17 s to
> The radiocarbon in our bodies is one of the naturally occurring sources of radiation. Let’s see how large a dose we receive. 14C decays via β- emission, and 18% of our body’s mass is carbon. a. Write out t
> a. Can you show that the orbital angular momentum of an electron in any given direction (e.g., along the z-axis) is always less than or equal to its total orbital angular momentum? In which cases would the two be equal to each other? b. Is the result in
> The polonium isotope 84 210
> The atomic mass of 12 25
> Thorium 90 230
> One of the problems of in-air testing of nuclear weapons (or, even worse, the use of such weapons!) is the danger of radioactive fallout. One of the most problematic nuclides in such fallout is strontium-90 (90Sr), which breaks down by β- decay with a ha
> The isotope 47 110
> a. Calculate the minimum energy required to remove one neutron from the nucleus 8 17
> a. Calculate the minimum energy required to remove one proton from the nucleus 6 12
> a. When gasoline is burned, it releases 1.3 * 108 J of energy per gallon (3.788 L). Given that the density of gasoline is 737 kg/m3, express the quantity of energy released in J/g of fuel. b. During fission, when a neutron is absorbed by a 235U nucleus,
> A p­n junction is part of the control mechanism for a wind turbine that is used to generate electricity. The turbine has been malfunctioning, so you are running diagnostics. You can remotely change the bias voltage V applied to the junction an
> The table gives the occupation probabilities f(E) as a function of the energy E for a solid conductor at a fixed temperature T To determine the Fermi energy of the solid material, you are asked to analyze this information in terms of the Fermiâ&
> The nucleus of a gold atom contains 79 protons. How does the energy required to remove a 1s electron completely from a gold atom compare with the energy required to remove the electron from the ground level in a hydrogen atom? In what region of the elect
> Why must the wave function of a particle be normalized?
> To determine the equilibrium separation of the atoms in the HCl molecule, you measure the rotational spectrum of HCl. You find that the spectrum contains these wavelengths (among others): 60.4 µm, 69.0 µm, 80.4 µm, 96.4 µm, and 120.4 µm. a. Use your mea
> Metallic lithium has a bcc crystal structure. Each unit cell is a cube of side length a = 0.35 nm. a. For a bcc lattice, what is the number of atoms per unit volume? Give your answer in terms of a. (Hint: How many atoms are there per unit cell?) b. Use
> The one­dimensional calculation of Example 42.4 (Section 42.3) can be extended to three dimensions. For the three dimensional fcc NaCl lattice, the result for the potential energy of a pair of Na+ and Cl- ions due to the electrostatic interact
> Compute the Fermi energy of potassium by making the simple approximation that each atom contributes one free electron. The density of potassium is 851 kg/m3, and the mass of a single potassium atom is 6.49 * 10-26 kg.
> Suppose the hydrogen atom in HF (see the Bridging Problem for this chapter) is replaced by an atom of deuterium, an isotope of hydrogen with a mass of 3.34 * 10-27 kg. The force constant is determined by the electron configuration, so it is the same as f
> The hydrogen iodide (HI) molecule has equilibrium separation 0.160 nm and vibrational frequency 6.93 * 1013 Hz. The mass of a hydrogen atom is 1.67 * 10-27 kg, and the mass of an iodine atom is 2.11 * 10-25 kg. a. Calculate the moment of inertia of HI a
> When an OH molecule undergoes a transition from the n = 0 to the n = 1 vibrational level, its internal vibrational energy increases by 0.463 eV. Calculate the frequency of vibration and the force constant for the interatomic force. (The mass of an oxygen
> The force constant for the internuclear force in a hydrogen molecule (H2) is k′ = 576 N / m. A hydrogen atom has mass 1.67 * 10-27 kg. Calculate the zeropoint vibrational energy for H2 (that is, the vibrational energy the molecule has in the n = 0 groun
> Our galaxy contains numerous molecular clouds, regions many light­years in extent in which the density is high enough and the temperature low enough for atoms to form into molecules. Most of the molecules are H2, but a small fraction of the mo
> The equilibrium separation for NaCl is 0.2361 nm. The mass of a sodium atom is 3.8176 * 10-26 kg. Chlorine has two stable isotopes, 35Cl and 37Cl, that have different masses but identical chemical properties. The atomic mass of 35Cl is 5.8068 * 10-26 kg,
> What are the most significant differences between the Bohr model of the hydrogen atom and the Schrödinger analysis? What are the similarities?
> Part (a) of Problem 42.39 gives an equation for the number of diatomic molecules in the lth rotational level to the number in the ground-state rotational level. a. Derive an expression for the value of l for which this ratio is the largest. b. For the
> Consider a gas of diatomic molecules (moment of inertia I) at an absolute temperature T. If Eg is a groundstate energy and Eex is the energy of an excited state, then the Maxwell–Boltzmann distribution (see Section 39.4) predicts that t
> Estimate the minimum and maximum wavelengths of the characteristic x rays emitted by a. vanadium (Z = 23) and b. rhenium (Z = 45). Discuss any approximations that you make.
> Electrons in the lower of two spin states in a magnetic field can absorb a photon of the right frequency and move to the higher state. a. Find the magnetic field magnitude B required for this transition in a hydrogen atom with n = 1 and l = 0 to be indu
> A hydrogen atom in an n = 2, l = 1, ml = -1 state emits a photon when it decays to an n = 1, l = 0, ml = 0 ground state. a. In the absence of an external magnetic field, what is the wavelength of this photon? b. If the atom is in a magnetic field in th
> In another universe, the electron is a spin-3/2 rather than a spin-1/2 particle, but all other physics are the same as in our universe. In this universe, a. what are the atomic numbers of the lightest two inert gases? b. What is the groundstate electro
> An electron in a hydrogen atom is in the 2p state. In a simple model of the atom, assume that the electron circles the proton in an orbit with radius r equal to the Bohr-model radius for n = 2. Assume that the speed v of the orbiting electron can be calc
> A large number of hydrogen atoms in 1s states are placed in an external magnetic field that is in the +z-direction. Assume that the atoms are in thermal equilibrium at room temperature, T = 300 K. According to the Maxwell–Boltzmann distribution (see Sect
> In a Stern–Gerlach experiment, the deflecting force on the atom is Fz = -µz(dBz/dz), where µz is given by Eq. (41.38) and dBz/dz is the magnetic-field gradient. In a particular experiment, the magnetic-field region is 50.0 cm long; assume the magnetic-fi
> What is the “central-field approximation” and why is it only an approximation?
> While studying the spectrum of a gas cloud in space, an astronomer magnifies a spectral line that results from a transition from a p state to an s state. She finds that the line at 575.050 nm has actually split into three lines, with adjacent lines 0.046
> An atom in a 3d state emits a photon of wavelength 475.082 nm when it decays to a 2p state. a. What is the energy (in electron volts) of the photon emitted in this transition? b. Use the selection rules described in Section 41.4 to find the allowed tra
> a. For an excited state of hydrogen, show that the smallest angle that the orbital angular momentum vector L can have with the z-axis is b. What is the corresponding expression for (θL)max, the largest possible angle between L and the z-ax
> Rydberg atoms are atoms whose outermost electron is in an excited state with a very large principal quantum number. Rydberg atoms have been produced in the laboratory and detected in interstellar space. a. Why do all neutral Rydberg atoms with the same n
> The normalized radial wave function for the 2p state of the hydrogen atom is R2p =(1/ 24a5 )re-r/2a. After we average over the angular variables, the radial probability function becomes P(r) dr =(R2p)2r2dr. At what value of r is P(r) for the 2p state a m
> For a hydrogen atom, the probability P(r) of finding the electron within a spherical shell with inner radius r and outer radius r + dr is given by Eq. (41.25). For a hydrogen atom in the 1s ground state, at what value of r does P(r) have its maximum valu
> Consider a hydrogen atom in the 1s state. a. For what value of r is the potential energy U(r) equal to the total energy E? Express your answer in terms of a. This value of r is called the classical turning point, since this is where a Newtonian particle
> a. What is the lowest possible energy (in electron volts) of an electron in hydrogen if its orbital angular momentum is 20 ħ ? b. What are the largest and smallest values of the z-component of the orbital angular momentum (in terms of ħ) for the elect
> a. Show that the total number of atomic states (including different spin states) in a shell of principal quantum number n is 2n2. [Hint: The sum of the first N integers 1 + 2 + 3 + …..+ N is equal to N(N + 1)/2.] b. Which shell has 50 states?
> A particle is described by the normalized wave function ψ(x, y, z)= Axe-αx2e-βy2e-γz2, where A, α, β, and γ are all real, positive constants. The probability that the particle will be found in the infinitesimal volume dx dy dz centered at the point (x0,
> For magnesium, the first ionization potential is 7.6 eV. The second ionization potential (additional energy required to remove a second electron) is almost twice this, 15 eV, and the third ionization potential is much larger, about 80 eV. How can these n
> An oscillator has the potential-energy function U(x, y, z)= 1/2 k′1(x2 + y2)+ 1/2 k′2z2, where k′1 > k′2. This oscillator is called anisotropic because the force constant is not the same in all three coordinate directions. a. Find a general expression f
> An isotropic harmonic oscillator has the potentialenergy function U(x, y, z)= 1/2 k′(x2 + y2 + z2). (Isotropic means that the force constant k′ is the same in all three coordinate directions.) a. Show that for this potential, a solution to Eq. (41.5) is
> A particle in the three-dimensional cubical box of Section 41.2 is in the ground state, where nX = nY = nZ = 1. a. Calculate the probability that the particle will be found somewhere between x = 0 and x = L/2. b. Calculate the probability that the part
> An electron is in a three-dimensional box. The x- and z-sides of the box have the same length, but the y-side has a different length. The two lowest energy levels are 2.24 eV and 3.47 eV, and the degeneracy of each of these levels (including the degenera
> While working in a magnetics lab, you conduct an experiment in which a hydrogen atom in the n = 1 state is in a magnetic field of magnitude B. A photon of wavelength λ (in air) is absorbed in a transition from the ms = - 1/2 to the ms = + 1/
> You are studying the absorption of electromagnetic radiation by electrons in a crystal structure. The situation is well described by an electron in a cubical box of side length L. The electron is initially in the ground state. a. You observe that the lo
> In studying electron screening in multielectron atoms, you begin with the alkali metals. You look up experimental data and find the results given in the table. The ionization energy is the minimum energy required to remove the least-bound electron from
> A hydrogen atom initially in an n = 3, l = 1 state makes a transition to the n = 2, l = 0, j = 1/2 state. Find the difference in wavelength between the following two photons: one emitted in a transition that starts in the n = 3, l = 1, j = 3/2 state and
> When low-energy electrons pass through an ionized gas, electrons of certain energies pass through the gas as if the gas atoms weren’t there and thus have transmission coefficients (tunneling probabilities) T equal to unity. The gas ions can be modeled ap
> As an intern at a research lab, you study the transmission of electrons through a potential barrier. You know the height of the barrier, 8.0 eV, but must measure the width L of the barrier. When you measure the tunneling probability T as a function of th
> The ionization energies of the alkali metals (that is, the lowest energy required to remove one outer electron when the atom is in its ground state) are about 4 or 5 eV, while those of the noble gases are in the range from 11 to 25 eV. Why is there a dif
> In your research on new solid-state devices, you are studying a solid-state structure that can be modeled accurately as an electron in a one-dimensional infinite potential well (box) of width L. In one of your experiments, electromagnetic radiation is ab
> Consider a potential well defined as U(x)= ∞ for x 0 for x > L (Fig. P40.60). Consider a particle with mass m and kinetic energy E a. The boundary condition at the infinite wall (x = 0) is ψ(0)= 0. What must the form of t
> a. The wave nature of particles results in the quantum-mechanical situation that a particle confined in a box can assume only wavelengths that result in standing waves in the box, with nodes at the box walls. Use this to show that an electron confined in
> a. Show by direct substitution in the Schrödinger equation for the one-dimensional harmonic oscillator that the wave function ψ1(x)= A1xe-α2x2/2, where α2 = mω/ħ, is a solution wit
> For small amplitudes of oscillation the motion of a pendulum is simple harmonic. For a pendulum with a period of 0.500 s, find the ground-level energy and the energy difference between adjacent energy levels. Express your results in joules and in electro
> A harmonic oscillator consists of a 0.020-kg mass on a spring. The oscillation frequency is 1.50 Hz, and the mass has a speed of 0.480 m/s as it passes the equilibrium position. a. What is the value of the quantum number n for its energy level? b. What
> a. For the finite potential well of Fig. 40.13, what relationships among the constants A and B of Eq. (40.38) and C and D of Eq. (40.40) are obtained by applying the boundary condition that c be continuous at x = 0 and at x = L? b. What relationships am
> An electron with initial kinetic energy 5.5 eV encounters a square potential barrier of height 10.0 eV. What is the width of the barrier if the electron has a 0.50% probability of tunneling through the barrier?
> A fellow student proposes that a possible wave function for a free particle with mass m (one for which the potential energy function U(x) is zero) is where κ is a positive constant. a. Graph this proposed wave function. b. Show that the p
> The penetration distance η in a finite potential well is the distance at which the wave function has decreased to 1/e of the wave function at the classical turning point: The penetration distance can be shown to be The probability of fi
> A small amount of magnetic-field splitting of spectral lines occurs even when the atoms are not in a magnetic field. What causes this?
> What is the probability of finding a particle in a box of length L in the region between x = L/4 and x = 3L/4 when the particle is in a. the ground level and b. the first excited level? (Hint: Integrate |ψ(x)|2 dx, where ψ is norm
> A particle is confined within a box with perfectly rigid walls at x = 0 and x = L. Although the magnitude of the instantaneous force exerted on the particle by the walls is infinite and the time over which it acts is zero, the impulse (that involves a pr
> Repeat Problem 40.48 for a particle in the first excited level. From Problem 40.48: Consider a particle in a box with rigid walls at x = 0 and x = L. Let the particle be in the ground level. Calculate the probability |ψ|2dx that the particle will be fo
> Consider a particle in a box with rigid walls at x = 0 and x = L. Let the particle be in the ground level. Calculate the probability |ψ|2dx that the particle will be found in the interval x to x + dx for a. x = L/4; b. x = L/2; c. x = 3L/4.
> An electron in a long, organic molecule used in a dye laser behaves approximately like a particle in a box with width 4.18 nm. What is the wavelength of the photon emitted when the electron undergoes a transition a. from the first excited level to the g
> A particle is in the ground level of a box that extends from x = 0 to x = L. a. What is the probability of finding the particle in the region between 0 and L/4? Calculate this by integrating |ψ(x)|2 dx, where ψ is normalized, from
> Consider a beam of free particles that move with velocity v = p/m in the x-direction and are incident on a potential- energy step U(x)= 0, for x < 0, and U(x)= U0 < E, for x > 0. The wave function for x < 0 is ψ(x)= Aeik1x + Be-ik1x, representing inciden
> a. Using the integral in Problem 40.42, determine the wave function ψ(x) for a function B(k) given by This represents an equal combination of all wave numbers between 0 and k0. Thus ψ(x) represents a particle with average wave num
> A particle of mass m in a one-dimensional box has the following wave function in the region x = 0 to x = L: Here ψ1(x) and ψ3(x) are the normalized stationary-state wave functions for the n = 1 and n = 3 levels, and E1 and E3 are
> Consider the wave packet defined by Let B(k)= e-a2k2. a. The function B(k) has its maximum value at k = 0. Let kh be the value of k at which B(k) has fallen to half its maximum value, and define the width of B(k) as wk = kh. In terms of α
> On the basis of the Pauli exclusion principle, the structure of the periodic table of the elements shows that there must be a fourth quantum number in addition to n, l, and ml. Explain.
> A particle is in the three-dimensional cubical box of Section 41.2. a. Consider the cubical volume defined by 0 ≤ x ≤ L/4, 0 ≤ y ≤ L/4, and 0 ≤ z ≤ L/4. What fraction of the total volume of the box is this cubical volume? b. If the particle is in the gr
> An electron is in a three-dimensional box with side lengths LX = 0.600 nm and LY = LZ = 2LX. What are the quantum numbers nX, nY, and nZ and the energies, in eV, for the four lowest energy levels? What is the degeneracy of each (including the degeneracy

