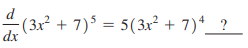[SOLVED] Replace ? with an expression that will make

> A point is moving on the graph of x3 + y2 = 1 in such a way that its y coordinate is always increasing at a rate of 2 units per second. At which point(s) is the x coordinate increasing at a rate of 1 unit per second?

> A point is moving along the x axis at a constant rate of 5 units per second. At which point is its distance from (0, 1) increasing at a rate of 2 units per second? At 4 units per second? At 5 units per second? At 10 units per second? Explain.

> Refer to Problem 27. At what rate is the person’s shadow growing when he is 20 feet from the pole? Data from Problem 27: A streetlight is on top of a 20-foot pole. A person who is 5 feet tall walks away from the pole at the rate of 5 feet per second. At

> (A) Is it possible to get 7 double 6’s in 10 rolls of a pair of fair dice? Explain. (B) If you rolled a pair of dice 36 times and got 11 double 6’s, would you suspect that the dice were unfair? Why or why not? If you suspect loaded dice, what empirical

> A weather balloon is rising vertically at the rate of 5 meters per second. An observer is standing on the ground 300 meters from where the balloon was released. At what rate is the distance between the observer and the balloon changing when the balloon i

> Boyle’s law for enclosed gases states that if the temperature is kept constant, the pressure P and volume V of a gas are related by the equation VP = k where k is a constant. If the volume is decreasing by 5 cubic inches per second, what is the rate of

> Refer to Problem 21. How fast is the surface area of the sphere increasing when the radius is 10 centimeters? Data from Problem 21: The radius of a spherical balloon is increasing at the rate of 3 centimeters per minute. How fast is the volume changing

> Refer to Problem 19. How fast is the circumference of a circular ripple changing when the radius is 10 feet? Data from Problem 19: A rock thrown into a still pond causes a circular ripple. If the radius of the ripple is increasing by 2 feet per second,

> Refer to Problem 17. Suppose that the distance between the boat and the dock is decreasing by 3.05 feet per second. How fast is the rope being pulled in when the boat is 10 feet from the dock? Data from Problem 17: A boat is being pulled toward a dock a

> A point is moving on the graph of 4x2 + 9y2 = 36. When the point is at (3, 0), its y coordinate is decreasing by 2 units per second. How fast is its x coordinate changing at that moment?

> Assume that x = x(t) and y = y(t). Find the indicated rate, given the other information. x2 - 2xy - y2 = 7; dy/dt = -1 when x = 2 and y = -1; find dx/dt

> Assume that x = x(t) and y = y(t). Find the indicated rate, given the other information. x2 + y2 = 4; dy/dt = 5 when x = 1.2 and y = -1.6; find dx/dt

> if it is possible to solve for y in terms of x, do so. If not, write “Impossible.” y2 + ex y + x3 = 0

> if it is possible to solve for y in terms of x, do so. If not, write “Impossible.” 2 ln y + y ln x = 3x

> What is the probability that a number selected at random from the first 60 positive integers is (exactly) divisible by 6 or 9?

> if it is possible to solve for y in terms of x, do so. If not, write “Impossible.” 4y2 - x2 = 36

> if it is possible to solve for y in terms of x, do so. If not, write “Impossible.” -4x2 + 3y + 12 = 0

> Refer to Problem 62. Find dF/dr and discuss the connection between dF/dr and dr/dF. Data from Problem 62: The equation is Newtonâs law of universal gravitation. G is a constant and F is the gravitational force between two objects havi

> The equation is Newtonâs law of universal gravitation. G is a constant and F is the gravitational force between two objects having masses m1 and m2 that are a distance r from each other. Use implicit differentiation to find dr/dF . Ass

> In Problem 59, find dV/dL by implicit differentiation. Data from problem 59: In biophysics, the equation (L + m) (V + n) = k is called the fundamental equation of muscle contraction, where m, n, and k are constants and V is the velocity of the shorteni

> The number x of compact refrigerators that an appliance chain is willing to sell per week at a price of $p is given by Use implicit differentiation to find dp/dx.

> The number x of fitness watches that people are willing to buy per week from an online retailer at a price of $p is given by x = 5,000 - 0.1p2 Use implicit differentiation to find dp/dx.

> Refer to the equation in Problem 53. Find the equation(s) of the tangent line(s) at the point(s) on the graph where y = -1. Round all approximate values to two decimal places. Equation from Problem 53:

> Find yâ² and the slope of the tangent line to the graph of each equation at the indicated point.

> In Problems a sample space S is described. Would it be reasonable to make the equally likely assumption? Explain. A wheel of fortune has seven sectors of equal area colored red, orange, yellow, red, orange, yellow, and red. We are interested in the color

> Find yâ² and the slope of the tangent line to the graph of each equation at the indicated point.

> Explain the difficulty that arises in solving x3 + y + xey = 1 for y as an explicit function of x. Find the slope of the tangent line to the graph of the equation at the point (0, 1).

> find the equation(s) of the tangent line(s) to the graphs of the indicated equations at the point(s) with the given value of x.

> Refer to the equation and graph shown in the figure. Find the slopes of the tangent lines at the points on the graph where x = 0.2. Check your answers by visually estimating the slopes on the graph in the figure.

> find x′ for x = x(t) defined implicitly by the given equation. Evaluate x′ at the indicated point. x3 - tx2 - 4 = 0; (-3, -2)

> Use implicit differentiation to find yâ² and evaluate yâ² at the indicated point.

> What is the probability of getting at least 1 black card in a 7-card hand dealt from a standard 52-card deck?

> Use implicit differentiation to find yâ² and evaluate yâ² at the indicated point.

> Use implicit differentiation to find y′ and evaluate y′ at the indicated point. 5x3 - y - 1 = 0; (1, 4)

> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. x + ln y = 1

> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. 4x2 - ey = 10

> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. x3 + y3 = 1

> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. 2x + 9y = 12

> In Problems a sample space S is described. Would it be reasonable to make the equally likely assumption? Explain. A nickel and dime are tossed. We are interested in the number of heads that appear, so an appropriate sample space is S = 50, 1, 26.

> Replace ? with an expression that will make the indicated equation valid.

> Find ï¦â²(x)

> Find ′(x) (x) = 5 - 6x5

> A yeast culture at room temperature (68°F) is placed in a refrigerator set at a constant temperature of 38°F. After t hours, the temperature T of the culture is given approximately by T = 30e-0.58t + 38 t ≥ 0 What is the rate of change of temperature o

> The use of iodine crystals is a popular way of making small quantities of water safe to drink. Crystals placed in a 1-ounce bottle of water will dissolve until the solution is saturated. After saturation, half of the solution is poured into a quart conta

> The number x of bicycle helmets people are willing to buy per week from a retail chain at a price of $p is given by (A) Find dx / dp. (B) Find the demand and the instantaneous rate of change of demand with respect to price when the price is $75. Write

> A single card is drawn from a standard 52-card deck. Calculate the probability of each event. A heart or a number less than 7 (count an ace as 1) is drawn.

> The total cost (in hundreds of dollars) of producing x cameras per week is (A) Find Câ²(x). (B) Find Câ²(15) and Câ²(24). Interpret the results.

> Find each derivative and simplify.

> Give the domain of ï¦, the domain of g, and the domain of m, where m(x) = ï¦ [g(x)].

> In Problems a sample space S is described. Would it be reasonable to make the equally likely assumption? Explain. A single fair coin is tossed. We are interested in whether the coin falls heads or tails, so an appropriate sample space is S = 5H, T6.

> Give the domain of ï¦, the domain of g, and the domain of m, where m(x) = ï¦ [g(x)].

> A student reasons that the functions ï¦(x) = (x + 1)ln (x + 1) - x and g(x) = (x + 1)1/3 must have the same derivative since she has entered ï¦ (x), g(x), ï¦ â²(x), and gâ&#128

> Find ï¦â²(x) and find the value(s) of x where the tangent line is horizontal.

> Find ï¦â²(x) and find the equation of the line tangent to the graph of ï¦ï at the indicated value of x.

> find the indicated derivative and simplify.

> A single card is drawn from a standard 52-card deck. Calculate the probability of each event. A king or a heart is drawn.

> find the indicated derivative and simplify.

> Find the indicated derivative and simplify.

> Find ï¦â²(x)and the equation of the line tangent to the graph of ï¦ at the indicated value of x. Find the value(s) of x where the tangent line is horizontal.

> Find ï¦â²(x) and simplify.

> An experiment consists of tossing three fair (not weighted) coins, except that one of the three coins has a head on both sides. Compute the probability of obtaining the indicated results. More than 1tail

> Find ï¦â²(x) and simplify.

> Replace ? with an expression that will make the indicated equation valid.

Question: Replace ? with an expression that will make