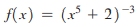Question: Find ′(x) and simplify. /
> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. 4x2 - ey = 10
> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. x3 + y3 = 1
> Find y′ in two ways: (A) Differentiate the given equation implicitly and then solve for y′. (B) Solve the given equation for y and then differentiate directly. 2x + 9y = 12
> In Problems a sample space S is described. Would it be reasonable to make the equally likely assumption? Explain. A nickel and dime are tossed. We are interested in the number of heads that appear, so an appropriate sample space is S = 50, 1, 26.
> Replace ? with an expression that will make the indicated equation valid.
> Replace ? with an expression that will make the indicated equation valid.
> Replace ? with an expression that will make the indicated equation valid.
> Find ′(x)
> Find ′(x)
> Find ′(x)
> Find ′(x) (x) = 5 - 6x5
> A yeast culture at room temperature (68°F) is placed in a refrigerator set at a constant temperature of 38°F. After t hours, the temperature T of the culture is given approximately by T = 30e-0.58t + 38 t ≥ 0 What is the rate of change of temperature o
> The use of iodine crystals is a popular way of making small quantities of water safe to drink. Crystals placed in a 1-ounce bottle of water will dissolve until the solution is saturated. After saturation, half of the solution is poured into a quart conta
> The number x of bicycle helmets people are willing to buy per week from a retail chain at a price of $p is given by (A) Find dx / dp. (B) Find the demand and the instantaneous rate of change of demand with respect to price when the price is $75. Write
> A single card is drawn from a standard 52-card deck. Calculate the probability of each event. A heart or a number less than 7 (count an ace as 1) is drawn.
> The total cost (in hundreds of dollars) of producing x cameras per week is (A) Find C′(x). (B) Find C′(15) and C′(24). Interpret the results.
> Find each derivative and simplify.
> Find each derivative and simplify.
> Find each derivative and simplify.
> Find each derivative and simplify.
> Find each derivative and simplify.
> Find each derivative and simplify.
> Give the domain of , the domain of g, and the domain of m, where m(x) =  [g(x)].
> Give the domain of , the domain of g, and the domain of m, where m(x) =  [g(x)].
> Give the domain of , the domain of g, and the domain of m, where m(x) =  [g(x)].
> In Problems a sample space S is described. Would it be reasonable to make the equally likely assumption? Explain. A single fair coin is tossed. We are interested in whether the coin falls heads or tails, so an appropriate sample space is S = 5H, T6.
> Give the domain of , the domain of g, and the domain of m, where m(x) =  [g(x)].
> A student reasons that the functions (x) = (x + 1)ln (x + 1) - x and g(x) = (x + 1)1/3 must have the same derivative since she has entered  (x), g(x),  ′(x), and gâ€
> Find ′(x) and find the value(s) of x where the tangent line is horizontal.
> Find ′(x) and find the value(s) of x where the tangent line is horizontal.
> Find ′(x) and find the value(s) of x where the tangent line is horizontal.
> Find ′(x) and find the equation of the line tangent to the graph of ï‚¦ï€ at the indicated value of x.
> Find ′(x) and find the equation of the line tangent to the graph of ï‚¦ï€ at the indicated value of x.
> Find ′(x) and find the equation of the line tangent to the graph of ï‚¦ï€ at the indicated value of x.
> find the indicated derivative and simplify.
> find the indicated derivative and simplify.
> A single card is drawn from a standard 52-card deck. Calculate the probability of each event. A king or a heart is drawn.
> find the indicated derivative and simplify.
> find the indicated derivative and simplify.
> find the indicated derivative and simplify.
> find the indicated derivative and simplify.
> find the indicated derivative and simplify.
> Find the indicated derivative and simplify.
> Find ′(x)and the equation of the line tangent to the graph of  at the indicated value of x. Find the value(s) of x where the tangent line is horizontal.
> Find ′(x)and the equation of the line tangent to the graph of  at the indicated value of x. Find the value(s) of x where the tangent line is horizontal.
> Find ′(x)and the equation of the line tangent to the graph of  at the indicated value of x. Find the value(s) of x where the tangent line is horizontal.
> Find ′(x) and simplify.
> An experiment consists of tossing three fair (not weighted) coins, except that one of the three coins has a head on both sides. Compute the probability of obtaining the indicated results. More than 1tail
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Find ′(x) and simplify.
> Replace ? with an expression that will make the indicated equation valid.
> Find (A) the derivative of T(x) / B(x) without using the quotient rule, and (B) T′(x) / B′(x). Note that the answer to part (B) is different from the answer to part (A).
> Use these frequencies to calculate the approximate empirical probabilities and odds for the events (A) The sum is a prime number or is exactly divisible by 4. (B) The sum is an odd number or exactly divisible by 3
> Exercises write the expression as a quotient of integers, reduced to lowest terms.
> Find (A) the derivative of F(x) S(x) without using the product rule, and (B) F′(x) S′(x). Note that the answer to part (B) is different from the answer to part (A)
> Find (A) the derivative of F(x) S(x) without using the product rule, and (B) F′(x) S′(x). Note that the answer to part (B) is different from the answer to part (A)
> One hour after a dose of x milligrams of a particular drug is administered to a person, the change in body temperature T(x), in degrees Fahrenheit, is given approximately by The rate T′(x) at which T changes with respect to the size of
> According to economic theory, the supply x of a quantity in a free market increases as the price p increases (see the figure). Suppose that the number x of DVD players a retail chain is willing to sell per week at a price of $p is given by (A) Find dx
> A communications company has installed a new cable television system in a city. The total number N (in thousands) of subscribers t months after the installation of the system is given by (A) Find N′(t). (B) Find N(16) and Nâ
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> An experiment consists of tossing three fair (not weighted) coins, except those one of the three coins has a head on both sides. Compute the probability of obtaining the indicated results. 0 Heads
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find each indicated derivative and simplify.
> Find ′(x) in two ways: (1) using the product or quotient rule and (2) simplifying first.
> Find ′(x) in two ways: (1) using the product or quotient rule and (2) simplifying first.
> Find ′ (x) and find the value(s) of x where ′ (x) = 0.
> Find ′ (x) and find the value(s) of x where ′ (x) = 0.
> Find  (x) and find the equation of the line tangent to the graph of  at x = 2.
> Find  (x) and find the equation of the line tangent to the graph of  at x = 2.
> (A) What are the odds for rolling a sum of 10 in a single roll of two fair dice? (B) If you bet $1 that a sum of 10 will turn up, what should the house pay (plus returning your $1 bet) if a sum of 10 turns up in order for the game to be fair? A pair of
> Find  (x) and find the equation of the line tangent to the graph of  at x = 2.
> (A) Find (x) using the quotient rule, and (B) Explain how (x) can be found easily without using the quotient rule.
> (A) Find (x) using the quotient rule, and (B) Explain how (x) can be found easily without using the quotient rule.
> Find the indicated derivatives and simplify.
> find the indicated derivatives and simplify.
> find the indicated derivatives and simplify.
> Find the indicated derivatives and simplify.
> Find the indicated derivatives and simplify.
> Find h′(x), where (x) is an unspecified differentiable function.
> Find h′(x), where (x) is an unspecified differentiable function.
> An experiment consists of tossing three fair (not weighted) coins, except those one of the three coins has a head on both sides. Compute the probability of obtaining the indicated results. 2 Heads
> Find h′(x), where (x) is an unspecified differentiable function.
> Find h′(x), where (x) is an unspecified differentiable function.
> Find h′(x), where (x) is an unspecified differentiable function.
> Find h′(x), where (x) is an unspecified differentiable function.
> Find (x) and simplify.
> Find (x) and simplify.
> Find (x) and simplify.
> Find (x) and simplify.